santeeset
esetnod32id 时间:2021-02-05 阅读:()
ContemporaryMathematicsVolume62,1987NON-STABLEK.
THEORYANDNON-CON'IMUTATIVETORIDedicatedtoHansBorclters,NicoHrtgenholtz,Richardl/'Kadisort,andDanielKastler,incelebrationoltheirContpletingSixtycircumttat'igatiottsoftheSunMarcA.
Rieffcl*DuringthclastfiveyearsthcrehasbecnmuchprogrcssinunderstandingandcalculatingtheK-groupsofvariousCx-algcbras.
Butoncethishrsbccnaccomplishcdinan1'givcnsiturtion,thcrcremainmanyintercstingqucstionsconcerningfincrstructurc,orwhatIcallnon-stableK-thcory.
Ir{ypurposehercistolistsomcolthcscquestions,andthentodiscusstheprogrcsswhichhasbccnmadcinansweringthcmfornon-commutativctoriandforafcwothcrcxamplcs.
l.
THEQUESTIONSIr{ostofthequestionswhichwcwillconsiderarconlyofintcrestforC*-algebraswithidentityelcment,andsowcwillassumetheprescnccofanidentityelcmentthroughout.
Actua1ly,asfarastheKoBroupisconCerncd,wecanusuall-vworkwithanyalgebrawithidentityelcmcnt.
Wcrecall[],l9]thatthcrcarctwoequivalentdcfinitionsoftheKO(A),ofanalgcbraA.
Inthcmostnaturalofthesctwodcfinitions,oneconsidcrsthesct,S(A),of*ThisrcsearchwasFoundationgrantDMSinpartbyNationalScicncc@1987AmericanMathematicalSociety027r-4r32187$1.
00+$.
25perPagesupported85-4l393.
267268MARCA.
RIEFFELisomorphismclasscsoffinitelygeneratedprojective(sayright)A-modules.
Underformationofdirectsumsofmodules,S(A)becomesacommutativesemigroup,withthe(classofthe)zeromodulcservingasidcntityelement.
ThenKo(A)isdefinedtobethcenveloping(orGrothcndieck)groupofthesemigroupS(A).
TheimageofS(A)inK0(A)providesKo(A)witha"positivecone",whichcanbebadlybehavedifAisnotfiniteinsomesense[3].
Thestudyandcalculationofthispositiveconecanbevcryintercsting,butweconsiderittobestillpartofthestableK-theoryofA.
RatheritisthestudyofS(A)itself,andofthepassagefromS(A)toKo(A),whichweconsidertoconstitutethenon-stablepartofKo-theory.
ThusforagivenalgebraAthefundamcntalqucstionofnon-stableI(o-theoryis:QUESTIONl.
'lVhatisthestructureofthesentigrottpS(A)Thisqucstionisusuallytoohardtoanswer,andsoonefirstconsidersspccialaspcctsofit.
Forexample:QUESTION2.
DoesS(A)satisfycancellation,thatis,ifIJ.
Yand\Nat'efinitell,generatedprojectiveA-ntodulessuchtl.
tatUeW=VeW.
doesilfollott'thatU=Ylfcancellationholds,thenthcmapfromS(A)toKo(A)isinjcctive.
ThusifoneknowswhatisthepositiveconeofKo(A),thcnoneknowsS(A).
Howevercancellationusuallyfails,andsooneinstcadasksweakerquestions.
Recall,forcxample,thetmodulesuandvrepresentthesameelementofKo(A)exactlyifthercissomeintegernsuchthatUoA"=V@An,whcreA.
dcnotesthefrecA-modulconngenerators.
Onccanthenask,foragivenalgcbra,whcthcrthercisanupperboundonthencededn's.
Thatis:QUESTION3.
IsthereapositiveintegerNsucftthatwhenet,erIJandYrepresentthesameelenzento/Ko(A),thenlJeAN=VeANThisquestioncanbcviewedasaskingwhethcrcancellationholdsassoonasthemodulcsinvolvedare"1argccnough".
Hcrc"largeenough"meansthatthemodulescontainANasasummand.
ForC*-algebrasthereisaclosclyrelatcdwayofdcfiningthesizeofamodule,namelybymeansolatraceonthcalgebra.
WerecallNON-STABLEK-THEORY,NON-COMMUTATIVETORI269thatthesecondequivalentdefinition[19]oftheK0-groupisintermsofprojectionsinmatrixalgebras,M.
(A),overA.
IfpisaprojectioninMn(A),thenpA"willbeafinitelygcneratcdprojcctivcrightA-module.
Ifrbca(finitc,positivc)traceonA,thcnrextcndsinanevidcntweytoatracconcachN{,.
,(A),andthusifpisaprojectioninanyM"(A)thenthepositivenumberr(p)isdefined.
OnecanshoweasilythatthisnumberdependsonlyontheisomorphismclassofthemodulepA".
ThusTdefinesahomomorphism(againdenotedbyr)ofS(A)intothcgroupofrealnumbcrs,whichthenfactorsthroughK"(A).
InanalogywithQuestion3onecanask:QUESTION4.
ForagiventraceTonA,isthereanumberNsircftthat,ifU@W=VeWandi/r(tul))N(soa/sor([W])7N),thertU=VInaslightlydifferentdirection,onecanaskaboutcancellationforspecialclasscsofmodules.
Themostcommonlydiscussedclassconsistsofthestablyfreemodules.
Specifically:QUESTION5.
ArestablyfreentodulesfreeThatis,ifthenodttleUissucltthatUeAn=Am+nforsomemandn,doesitahvaysfollowtlntU:A*Ifnot,thenonecanask,asbefore,whetherthcreisaboundonthcn'sthatareneeded,thatis:QUESTION6.
1sthereanintegerllsucltthattvlterteverUlsastablyfreemodttlethenU@ANisfreeThenextquestionisbestphrasedintermsofprojections,andismostappropriatetoaskforC*-algebraswherecancellationholds.
HcreandlaterweletU"(A)denotethegroupofunitaryelemcntsofMr,(A),andweletUf(A)denotetheconnectedcomponentoftheidcntityclementinU"(A).
270MARCA.
RIEFFELQUESTION7.
IfAsatisfiescancellatiort,andifpandqare(self-adioittt)proiectionsinM"(A)whichrepresenttl'tesanteclassittK.
(A),thenarepandqinthesanleconnectedcontponentoftlzesetofprojectionsinM"(A)Equivalently,isthereaunitaryuirlU;(A)strchtltatupu*=qAquestionaboutKn(A)whichgoesinaratherdifferentdirectionis:QUESTION8.
l{hatisthesntallestnsucllthattheprojectiortsirtM"(A)generateKo(A)WeremarkthattheanswcrstoQuestionsIand2areinvariantunderMoritaequivalenceofalgebras[12],whereasmostoftheotherquestionsaboveinvolve,insomesense,thepositionofthefreemoduleofrankoneasanordcrunitinS(A).
Werecall[9]thatK.
,(A)isdefinedasthelimitofthegroupsu"(A)/u;(A)'U.
,+1(A)/uf+1(A).
Thcrearetwoquiteevidentquestionstoaskaboutthenon-stablebehaviorforK'namely:QUESTION9.
lVhatisthesmallestnsucltthatthehontomorphisntfrontUu(A)/Uf(A)roKr(A)isinjectivelorallk)nQUESTION10.
IVhatistltesmallestnsucltthatthehontomorphisntfrontUn(A)/U;(A)loKr(A)issuriectiveThcrcisasubstantialliteratureinalgebraicK-theoryconcerningtheselasttwoquestions.
Sec[20]andthereferenccsthcrcin.
2.
TECHNIQUESANDINTERRELATIONSForacommutativeC*-algebra,offormA=C(X)forXacompactspace,thefinitelygeneratedprojcctivemodulescorrespondexactlytothecomplcxvectorbundles,byatheoremofSwan[8,121.
Thusinthiscasethevastapparatusofalgebraictopology,especiallytopologicalK-theory,canbebroughttobcaronansweringtheabovequestions.
Butitisknownthattheanswersareusuallycomplicated.
ThereareasmallnumberofgeneralNON-STABLEK-THEORY,NON-COMMUTATIVETORI27Lresults.
Forexample,fromTheorem1.
5ofChapter8of[8]oneobtainsimmcdiatelythefollowinganswertoQucstion4(whereonemaytakeasthetrace,evaluationoffunctionsatsomefixedpoint):THEOREMl.
LetXbeaconxpactconnectedCWcontplexofclimensiortd.
IfYandwarecontplexvectorbwtdlesoverXwhicltrepresentthesanteelemento7t.
df2,tltenY=W.
Butusually,evenwhenonecancomputeforspecificexamples,itisdifficulttofindgeneralpatterns.
Onemustthenexpectthatthiswillbeallthemorethesituationfornon-commutativcC*-algebras.
InthecaseofC*-algebraswhicharepostliminal(i.
e.
GCR),andsoarefairlycloselyrelatedtocommutativeC*-algebras,onecanhopethatresultsintopologicalK-theorywillprovidesomeguidanceastowhattoexpect,aswellasresultsuponwhichonecanbuildbyinductivearguments.
Forexample,AlbertJ.
Sheu[17]hasstudiedtheunitizedC*-algebra,6*(C)whereGisasimplyconncctednilpotentLiegroupofformR"rR.
TheseareGCR,andcanbecOnsidcredtobe"non-commutativeSpheres".
HeobtainsagoodanswertoQucstion4,whereaStracehcuscsevaluationatthcadjoined"pointofinfinity".
HealsoshowsthatcancellationholdsforcertainoftheGofarbitrarilyhighdimension.
Buthehasanexampleofafour-dimensionalGforwhichcancellationfails,thoughhecanneverthelcssdescribethestructureofitsscmigroupofprojectivemodules.
TheoremIabovesuggeststhatsomenotionofdimensioninthenon-commutativecontextmightplayaroleinnon-stableK-theory.
Itisfarfromclearwhetherthereshouldbeauniquenotionofdimensioninthiscontext,butonenotionhasalreadyplayedanimportantroleinalgebraicK-theory,namelythenotionofBassstablerank.
Weomitthedefinition,sincefortopologicalalgebrasitismoreconvenienttousethenotionoftopologicalstablcrank(tsr)whichwasintroducedin[13]andshowntheretodominatctheBassstablerank.
Subsequently,itwasshownbyHermanandvasersteintllthatforc*-algebrasthetopologicalstablerank272MARC.
A.
RIEFFELcoincideswiththeBassstablerank.
Todefinethetopologicalstablerank,weletGenu(v),foranymodulevandpositiveintegerk,denotethecollectionofk-tuplesofelements,{tj},inVkwhichcollectivelygenerateValgebraically,thatis,suchthatEvrA=V.
NotenextthatifAisatopologicalalgebra,thenanyfinitelygcncratedprojectiveA-module,beingrealizableasasummandofsomeA',isatopologicalmodule(independentlyoftherealization).
DEFINITION.
LetAbeatopologicalalgebra,andletYbeafinitelygeneratedprojectiveA-module.
Thentsr(Y)isdefinedtobetheleastintegerk,ifitexists,suchthatGenn(V)isdenseinYk.
Ittparticular,tsr(A)isdefinedtobethetsrofAasariglttA-module.
Motivationfortheabovedefinitioncanbefoundin[13].
ThereisasubstantiallitcratureinalgebraicK-theory(see[],20])relatingtheBassstableranktothenon-stablebehaviorofK'especiallyQuestions9and10.
AppliedtoC*-algebras,theseresultsyield,forexample:THEOREM2(Theorem10.
12of[13]).
I.
fn>tsr(A)+2,thenthemapfrontU"(A)/U;(A)roKr(A)isanisontorphism.
Warfield[21]seemstohavebeenthefirsttonoticeadirectgcneralrclationshipbetweentheBassstablcrankandthecancellationpropertyforprojectivemodules.
AdistinctivefeatureofhisrcsultsisthatitisnottheBassstablerankofthealgebrawhichisimportant,butratherthatoftheendomorphismalgebraofthemodulebeingcancelled.
HisresultsareinthespiritofQuestions3and4totheeffectthatcancellationholdsfor"sufficicntlylarge"modules,butnowsizeismeasuredintermsofthesizeofthemodulebeingcancelled.
Forexample,fromhisresultsoneobtains:THEOREM3.
LetWbeaprojectiveA-ntoduleandletnbetheBassstablerankofEndo(W).
IfUandYareprojectivemodtilessucltthat(U@Wn)@w=V@W,NON-STABLEK-THEORY,NON-COMN{UTATIVETORI273thenUoWn:V.
Toapplysuchstableranktechniques,oneneedstobeabletoestimatestableranks,andthisisoftenverydifficult.
ButSheu'sworkmentionedabovedependsheavilyonsuccessfulestimatesofstableranks.
andthesameistruefortheresultsaboutnon-commutativetoritobediscussedinthenextsection.
Acrucialtoolisprovidedbyanestimateinthecaseofcrossedproductsbytheintegers,whichcanbeconsideredthemostimportantresultof[3](seeTheorem7.
1).
Specifically:THEOREM4.
LetA*c(ZdenotethecrossedproductofaC*-algebraAbyanactiortaoftheirilegers.
Tlterttsr(AxoZ)identityelcmentweletC-denotethealgebraobtainedbyadjoininganidcntitytoC.
THEOREM6(Theorema.
lloftlTl).
ForanyC*-algebraAandanyn)l,cancellationholdsfor(Aa8,,)-.
Notablymissingaretechniquesforobtainingalowerboundfortsrintheabsenceofeitheradirectlyrelevantcompactspaceorofproperisometries.
Inparticular,nofinitesimpleC*-algebrasareknownforwhichonecanprovethattsr(A))2,althoughtherearcmanypossiblecandidatcs.
ThisisrclatedtothelackofanyexampleofafinitesimpleC*-algebraforu'hichcancellationfails'274MARCA.
RIEFFELsincetsr(A)=Iisequivalenttotheinvertibleelementsbeingdense,andonehas(seeIIL2.
4of[4]and4.
5.
2of[3]):THEOREM7.
IfinvertibleelementsaredenseinA,thenAsatisfiescancellation.
Notice,forexample,thatthisimpliesthatAFC*-algebrashavecancellation.
Thereiscorrespondinglyalackofanyexampleofafinitesimplec*-algebraforwhichonecanshowthattheinvertibleelementsarenotdense.
LetusdiscussnextthefactthatthevariousquestionsstatedinSlaresomewhatinterrelated.
Wegivetwoexamples,whoseproofswillappearin[6].
ThefirstinvolvesQuestions8and10.
THEOREM8.
Letq.
beanautontorphisntoftheunitalC*-algebraAtvltichisintheconnectedconxponentoftheidentityautontorphisntofA,andletc.
alsodenotethecorrespondingactiortofZonA.
SupposethatLEveryelemento/Kr(A)isrepresentedbyaninvertibleelementinAitself.
2.
TlteprojectiortsittAgenerateKo(A).
TheneveryelententinKr(A"oz)isrepresentedbyaninvertibleelententinAxdZ.
ForthenextresultweletTAdenotetheC*-algebraofcontinuousfunctionsfromthecircle,T,toA.
weremarkthatthenTA=AxoZforcrthetrivialaction,anditisaninterestingquestionastowhetherthenexttheoremcanbegeneralizedtothecaseofnon-triviala.
Thistheoreminvolveseuestions2and9.
THEOREM9.
ForaunitalC*-algebraAthefollowingareeqLtivarent:l.
TAsatisfiescancellation.
2.
Botlta)Asatisfiescancellation.
andb)ForeveryprojectiveA-moduleYthenaturalmapfrontAuto(V)/Autf,(V)roKr(A)isinjective.
Theproofofthislasttheoremcomesfromexaminingthefamiliar"clutching"constructionwhichtoanyautomorphismofanA-moduleassociatesaTA-module.
NON-STABLEI(-THEORY,NON-COMMUTATIVETORI2753.
NON-COMMUTATIYETORIBydefinitionanon-commutativetorus,Ag,isaC*-algebradefinedasfollows.
Let0beaskewbilinearformonR',anddefineaskewcocycleoonZnbyo(x,Y)=exP(ni0(x,Y))forx,yZn.
LetAgbethegroupC*-algebraofZ"twistedbyo,i.
e.
,C*(2",o).
ThustoeachxeZnthereisaunitary,ux,inAg,andtheseunitariessatisfythcrelationuyu*=o(x,Y)u**,.
Forn=2oneobtainsthemorefamiliarirrational(andrational)rotationC*-algebras[4].
BytheworkofPimsnerandVoiculescu[11]concerningthecomputationoftheK-groupsofcrossedproductswiththeintegers,onefindsthattheK-groupsofanAgarethesameasthoseforanordinaryn-torusTn(whichistheAOforwhichQ=0)'Inparticular,Ko(Ag)=Zzn-tThisstillleavesquiteopentheproblemofdetcrminingwhatisthcpositiveconeofKo(Ag).
ByusingtechniquesfromtopologicalK-theory,onecanshowthattheanswerforTtbecomescomplicatedforn=4and5(see[16]),andIdonotknowiftheanswerisknownfordimensionsmuchabovethat.
(Also,cancellationalreadyfailsforT5.
)Itturnsouthoweverthatthereisaniceanswerwhen0isnotentirelyrational,inthesensethattherangeof0ontheintegerlatticeZnCR'isnotentirelycontainedintherationalnumbcrs.
Noticethatthereisacanonicaltrace,T,onAg,CorrspondingtoevaluatingattheidentityelementofZn,withitsassociatedhomomorphism,T,fromKo(Ag)intothegroupofrealnumbers.
276MARCA.
RIEFFELThelatterispositiveonthepositiveconeofKo(Ag).
In[16]itisshownthat:THEOREMA.
If0isnotrational,thenthepositiveconeofKo(A6)consistsofexactlytheelententsonwhichtispositive.
weshouldmentionthattherangeofronKo(Ag)hasbeenelucidatedbyElliott[6],whoseworkisanimportantingredientoftheproofsofmostofthetheoremsstatedinthissection.
Muchoftheproofoftheabovetheoreminvolvesaspecificconstruction.
sketchedinU5l,offinitelygeneratedprojectivemodulesoverAg,togetherwithaclassificationofthemodulessoconstructed,bymeansofconnes'cherncharacterintroducedin[5].
Infact,onefindsthateveryelementofKo(Ag)withpositivetraceisrepresentedbyamoduleobtainedbytheconstruction.
Ifoneexaminestheconstructionfurthersoastoobtain,amongothcrthings,informationaboutthetopologicalstablerankoftheendomorphismalgebrasoftheconstructedmodules,onefindsthatonecanapplywarfield'stheorem(Theorem2above)toanswcrQuestion2:THEOREMB.
If0isnotrational,thens(Ag)satisfiescancellatiott.
ThusforsuchIonecananswereuestionl,thatis,onecandescribes(A6).
Evenmore,onehasanexplicitconstructionofallfinitelygeneratedprojectiveA6-modulesuptoisomorphism.
Forthespecialcasen=2theseresultswereobtainedearlierin[14].
Wealsoobtainin!
61ananswertoeuestion8:THEOREMc-If0isnotrational,thentlzeprojectionsinAsgenerateKo(Ag).
ByusingTheorem8oftheprevioussectionwithTheoremCaboveinaninductionargument,wethenobtainthefollowineanswertoQuestionl0:THEOREMD.
IfAisnotrational,theneveryelententofKr(Ag)isrepresentedbyaninvertibleelementolAe.
ByusingTheorem9oftheprevioussectionwithTheoremBwealsoobtainthefollowinganswertoQuestion9:THEOREME.
If0isnotrational,thenthenaturalnlaDfrontU1(A0)/U(Ag)toKr(Ag)isanisontorphisnt.
NON-STABLEK.
THEORY,NON-COMMUTATIVETORI277Fromthistheoremtogetherwithsomeadditionalargumcnt,oncobtainsthefollowinganswertoQuestion7:THEOREMF.
rf0isnotrational,thenanytwoprojectiortsinM-(Ag)whichrepresentthesanteelemento/K6(Ag)areintltesameconnectedcomponentofthesetofprojectionsirrM-(Ag)'Inclosing,letmementionthatJ.
A.
Packer[9,l0]hasstudiedthealgebrasc*(G,o)whereGisthediscreteHeisenberggroupandoisacocycleonG.
Amongmanyotherresults,shehasshownthatformanyo's,thesealgebrassatisfycancellation'Forthissheuses,inpart,thetechniquesof[13,l4].
tllt)1L-lt3lREFERENCESBass,H.
,AlgebraicK-theory,(Benjamin,NewYork,1968)'Blackadar,8.
,uAstablecancellationtheoremforsimpleC+-algebras,"Proc.
LondonMath.
Soc.
47(1983)'303-305'"NotesonthestructureofprojcctionsCTIlgEbras,"SemesterberichtFunktionalanalysis,insimpleTubingen,Winter-semester,1982/83.
l,4landHandelman,D.
,"DimensionfunctionsandtracesonT*-atgebras,"J.
FunctionalAnal.
45(1982),297-340't5lConnes,A.
,"C*-algbbresetgdomdtriediff6rentielle,"C'R'Acad.
Sci.
Paris290(1980),599-604.
t6lElliott,G.
A.
,"OntheK-theoryofthec*-algebrageneratedbyaprojectiverepresentationofatorsion-freediscreteabeliangroup,"pp.
19l'250inOperatorAlgebrasandGrottpRepresentations,vol.
I(Pitman,London,1984)'t7lHerman,R.
H.
andVaserstein,L.
N.
,"ThestablerangeofC*-algebras,"Invent.
Math'77(1984),553-555't8lHusemoller,D.
,FiberBtmdles(Springer-Vcrlag,NewYork'HeidelbergandBerlin,1966).
tl0]_,"C*-algcbrasgeneratedofthediscreteHeisenberggroupappear.
27819lPacker,associatedMARCA.
RIEFFELJ.
A.
.
"K-thcoretictotransformationsandinvariantsforC*-algebrasinduccdflows,"preprint.
byprojcctiverepresentationsI,II,"J.
FunctionalAnal.
,toovcrthedoctoraI"Trans.
Fl]Pimsner,M.
Y.
andVoiculescu,D.
,"ExactsequencesforK-groupsandExt-groupsofcertaincrossedproductC*-algebras,"J.
OperatorTheory4(1980),93-llg.
il2]Rieffel,M.
A.
,"Moritaequivalenceforoperatoralgebras,"inOperatorAlgebrasandApplicatiorts,(R.
V.
Kadison,ed.
),proc.
Symp.
PureMath.
38,pp.
285-298,(AmericanMathematicalSociety,Providence,1982).
[l3]-,''DimenSionandstabierankintheK.
theoryofC*-algebras,"Proc.
LondonMath.
Soc.
47(19g3),295-302.
[4]--,"Thecancellationtheoremforprojectivemodulesoverirrationalrotationc*-algebras,"proc.
LondonMath.
Soc.
47(1983),285-302.
II5]_,"'Yectorbundles'overhigherdimensional'non-commutativetori',"proc.
conferenceonoperatorAlgebras,ConnectionswithTopologyandErgodicTheory,LectttreNotesinMath.
,1r32,pp.
456-467(Springer-verlag,BerlinandHeidelberg,1985).
tl6l"Projcctivemodulesoverhigherdimensionalnon-commutativetori,"preprint.
[l7]Shcu,A.
J.
-L.
,"ThecancellationpropertyformodulesgroupC*-algebrasofcertainnilpotentLiegroups,"dissertation,IJniversityofCalifornia,Berkeley,19g5.
II8]Swan,R.
,"Vectorbundlesandprojectivemodulcs,Amer.
Math.
Soc.
105(1962),264-277.
[19]Taylor,J.
L.
,"Banachalgebrasandtopology,"iDAlgebrasinAnalysis,pp.
Il8-186,(Academicpress,Newyork,Igl5).
I20)vaserstein,L.
N.
,"ThefoundationsofalgebraicK-theory,"UspekhiMat.
Nauk.
3l(1976),87-149.
NON-STABLEK-THEORY,NON-CON{MUTATIVEToRI2T9[21]Warficld,R.
8.
,"Cancellationofmodulesandgroupsandstablerangeofendomorphismrings,"PacificJ'Math'91(1980),457-485.
DEPARTMENTOFMATHEMATICSUNIVERSITYOFCALIFORNIABERKELEY.
CALIFORNIA94'720
THEORYANDNON-CON'IMUTATIVETORIDedicatedtoHansBorclters,NicoHrtgenholtz,Richardl/'Kadisort,andDanielKastler,incelebrationoltheirContpletingSixtycircumttat'igatiottsoftheSunMarcA.
Rieffcl*DuringthclastfiveyearsthcrehasbecnmuchprogrcssinunderstandingandcalculatingtheK-groupsofvariousCx-algcbras.
Butoncethishrsbccnaccomplishcdinan1'givcnsiturtion,thcrcremainmanyintercstingqucstionsconcerningfincrstructurc,orwhatIcallnon-stableK-thcory.
Ir{ypurposehercistolistsomcolthcscquestions,andthentodiscusstheprogrcsswhichhasbccnmadcinansweringthcmfornon-commutativctoriandforafcwothcrcxamplcs.
l.
THEQUESTIONSIr{ostofthequestionswhichwcwillconsiderarconlyofintcrestforC*-algebraswithidentityelcment,andsowcwillassumetheprescnccofanidentityelcmentthroughout.
Actua1ly,asfarastheKoBroupisconCerncd,wecanusuall-vworkwithanyalgebrawithidentityelcmcnt.
Wcrecall[],l9]thatthcrcarctwoequivalentdcfinitionsoftheKO(A),ofanalgcbraA.
Inthcmostnaturalofthesctwodcfinitions,oneconsidcrsthesct,S(A),of*ThisrcsearchwasFoundationgrantDMSinpartbyNationalScicncc@1987AmericanMathematicalSociety027r-4r32187$1.
00+$.
25perPagesupported85-4l393.
267268MARCA.
RIEFFELisomorphismclasscsoffinitelygeneratedprojective(sayright)A-modules.
Underformationofdirectsumsofmodules,S(A)becomesacommutativesemigroup,withthe(classofthe)zeromodulcservingasidcntityelement.
ThenKo(A)isdefinedtobethcenveloping(orGrothcndieck)groupofthesemigroupS(A).
TheimageofS(A)inK0(A)providesKo(A)witha"positivecone",whichcanbebadlybehavedifAisnotfiniteinsomesense[3].
Thestudyandcalculationofthispositiveconecanbevcryintercsting,butweconsiderittobestillpartofthestableK-theoryofA.
RatheritisthestudyofS(A)itself,andofthepassagefromS(A)toKo(A),whichweconsidertoconstitutethenon-stablepartofKo-theory.
ThusforagivenalgebraAthefundamcntalqucstionofnon-stableI(o-theoryis:QUESTIONl.
'lVhatisthestructureofthesentigrottpS(A)Thisqucstionisusuallytoohardtoanswer,andsoonefirstconsidersspccialaspcctsofit.
Forexample:QUESTION2.
DoesS(A)satisfycancellation,thatis,ifIJ.
Yand\Nat'efinitell,generatedprojectiveA-ntodulessuchtl.
tatUeW=VeW.
doesilfollott'thatU=Ylfcancellationholds,thenthcmapfromS(A)toKo(A)isinjcctive.
ThusifoneknowswhatisthepositiveconeofKo(A),thcnoneknowsS(A).
Howevercancellationusuallyfails,andsooneinstcadasksweakerquestions.
Recall,forcxample,thetmodulesuandvrepresentthesameelementofKo(A)exactlyifthercissomeintegernsuchthatUoA"=V@An,whcreA.
dcnotesthefrecA-modulconngenerators.
Onccanthenask,foragivenalgcbra,whcthcrthercisanupperboundonthencededn's.
Thatis:QUESTION3.
IsthereapositiveintegerNsucftthatwhenet,erIJandYrepresentthesameelenzento/Ko(A),thenlJeAN=VeANThisquestioncanbcviewedasaskingwhethcrcancellationholdsassoonasthemodulcsinvolvedare"1argccnough".
Hcrc"largeenough"meansthatthemodulescontainANasasummand.
ForC*-algebrasthereisaclosclyrelatcdwayofdcfiningthesizeofamodule,namelybymeansolatraceonthcalgebra.
WerecallNON-STABLEK-THEORY,NON-COMMUTATIVETORI269thatthesecondequivalentdefinition[19]oftheK0-groupisintermsofprojectionsinmatrixalgebras,M.
(A),overA.
IfpisaprojectioninMn(A),thenpA"willbeafinitelygcneratcdprojcctivcrightA-module.
Ifrbca(finitc,positivc)traceonA,thcnrextcndsinanevidcntweytoatracconcachN{,.
,(A),andthusifpisaprojectioninanyM"(A)thenthepositivenumberr(p)isdefined.
OnecanshoweasilythatthisnumberdependsonlyontheisomorphismclassofthemodulepA".
ThusTdefinesahomomorphism(againdenotedbyr)ofS(A)intothcgroupofrealnumbcrs,whichthenfactorsthroughK"(A).
InanalogywithQuestion3onecanask:QUESTION4.
ForagiventraceTonA,isthereanumberNsircftthat,ifU@W=VeWandi/r(tul))N(soa/sor([W])7N),thertU=VInaslightlydifferentdirection,onecanaskaboutcancellationforspecialclasscsofmodules.
Themostcommonlydiscussedclassconsistsofthestablyfreemodules.
Specifically:QUESTION5.
ArestablyfreentodulesfreeThatis,ifthenodttleUissucltthatUeAn=Am+nforsomemandn,doesitahvaysfollowtlntU:A*Ifnot,thenonecanask,asbefore,whetherthcreisaboundonthcn'sthatareneeded,thatis:QUESTION6.
1sthereanintegerllsucltthattvlterteverUlsastablyfreemodttlethenU@ANisfreeThenextquestionisbestphrasedintermsofprojections,andismostappropriatetoaskforC*-algebraswherecancellationholds.
HcreandlaterweletU"(A)denotethegroupofunitaryelemcntsofMr,(A),andweletUf(A)denotetheconnectedcomponentoftheidcntityclementinU"(A).
270MARCA.
RIEFFELQUESTION7.
IfAsatisfiescancellatiort,andifpandqare(self-adioittt)proiectionsinM"(A)whichrepresenttl'tesanteclassittK.
(A),thenarepandqinthesanleconnectedcontponentoftlzesetofprojectionsinM"(A)Equivalently,isthereaunitaryuirlU;(A)strchtltatupu*=qAquestionaboutKn(A)whichgoesinaratherdifferentdirectionis:QUESTION8.
l{hatisthesntallestnsucllthattheprojectiortsirtM"(A)generateKo(A)WeremarkthattheanswcrstoQuestionsIand2areinvariantunderMoritaequivalenceofalgebras[12],whereasmostoftheotherquestionsaboveinvolve,insomesense,thepositionofthefreemoduleofrankoneasanordcrunitinS(A).
Werecall[9]thatK.
,(A)isdefinedasthelimitofthegroupsu"(A)/u;(A)'U.
,+1(A)/uf+1(A).
Thcrearetwoquiteevidentquestionstoaskaboutthenon-stablebehaviorforK'namely:QUESTION9.
lVhatisthesmallestnsucltthatthehontomorphisntfrontUu(A)/Uf(A)roKr(A)isinjectivelorallk)nQUESTION10.
IVhatistltesmallestnsucltthatthehontomorphisntfrontUn(A)/U;(A)loKr(A)issuriectiveThcrcisasubstantialliteratureinalgebraicK-theoryconcerningtheselasttwoquestions.
Sec[20]andthereferenccsthcrcin.
2.
TECHNIQUESANDINTERRELATIONSForacommutativeC*-algebra,offormA=C(X)forXacompactspace,thefinitelygeneratedprojcctivemodulescorrespondexactlytothecomplcxvectorbundles,byatheoremofSwan[8,121.
Thusinthiscasethevastapparatusofalgebraictopology,especiallytopologicalK-theory,canbebroughttobcaronansweringtheabovequestions.
Butitisknownthattheanswersareusuallycomplicated.
ThereareasmallnumberofgeneralNON-STABLEK-THEORY,NON-COMMUTATIVETORI27Lresults.
Forexample,fromTheorem1.
5ofChapter8of[8]oneobtainsimmcdiatelythefollowinganswertoQucstion4(whereonemaytakeasthetrace,evaluationoffunctionsatsomefixedpoint):THEOREMl.
LetXbeaconxpactconnectedCWcontplexofclimensiortd.
IfYandwarecontplexvectorbwtdlesoverXwhicltrepresentthesanteelemento7t.
df2,tltenY=W.
Butusually,evenwhenonecancomputeforspecificexamples,itisdifficulttofindgeneralpatterns.
Onemustthenexpectthatthiswillbeallthemorethesituationfornon-commutativcC*-algebras.
InthecaseofC*-algebraswhicharepostliminal(i.
e.
GCR),andsoarefairlycloselyrelatedtocommutativeC*-algebras,onecanhopethatresultsintopologicalK-theorywillprovidesomeguidanceastowhattoexpect,aswellasresultsuponwhichonecanbuildbyinductivearguments.
Forexample,AlbertJ.
Sheu[17]hasstudiedtheunitizedC*-algebra,6*(C)whereGisasimplyconncctednilpotentLiegroupofformR"rR.
TheseareGCR,andcanbecOnsidcredtobe"non-commutativeSpheres".
HeobtainsagoodanswertoQucstion4,whereaStracehcuscsevaluationatthcadjoined"pointofinfinity".
HealsoshowsthatcancellationholdsforcertainoftheGofarbitrarilyhighdimension.
Buthehasanexampleofafour-dimensionalGforwhichcancellationfails,thoughhecanneverthelcssdescribethestructureofitsscmigroupofprojectivemodules.
TheoremIabovesuggeststhatsomenotionofdimensioninthenon-commutativecontextmightplayaroleinnon-stableK-theory.
Itisfarfromclearwhetherthereshouldbeauniquenotionofdimensioninthiscontext,butonenotionhasalreadyplayedanimportantroleinalgebraicK-theory,namelythenotionofBassstablerank.
Weomitthedefinition,sincefortopologicalalgebrasitismoreconvenienttousethenotionoftopologicalstablcrank(tsr)whichwasintroducedin[13]andshowntheretodominatctheBassstablerank.
Subsequently,itwasshownbyHermanandvasersteintllthatforc*-algebrasthetopologicalstablerank272MARC.
A.
RIEFFELcoincideswiththeBassstablerank.
Todefinethetopologicalstablerank,weletGenu(v),foranymodulevandpositiveintegerk,denotethecollectionofk-tuplesofelements,{tj},inVkwhichcollectivelygenerateValgebraically,thatis,suchthatEvrA=V.
NotenextthatifAisatopologicalalgebra,thenanyfinitelygcncratedprojectiveA-module,beingrealizableasasummandofsomeA',isatopologicalmodule(independentlyoftherealization).
DEFINITION.
LetAbeatopologicalalgebra,andletYbeafinitelygeneratedprojectiveA-module.
Thentsr(Y)isdefinedtobetheleastintegerk,ifitexists,suchthatGenn(V)isdenseinYk.
Ittparticular,tsr(A)isdefinedtobethetsrofAasariglttA-module.
Motivationfortheabovedefinitioncanbefoundin[13].
ThereisasubstantiallitcratureinalgebraicK-theory(see[],20])relatingtheBassstableranktothenon-stablebehaviorofK'especiallyQuestions9and10.
AppliedtoC*-algebras,theseresultsyield,forexample:THEOREM2(Theorem10.
12of[13]).
I.
fn>tsr(A)+2,thenthemapfrontU"(A)/U;(A)roKr(A)isanisontorphism.
Warfield[21]seemstohavebeenthefirsttonoticeadirectgcneralrclationshipbetweentheBassstablcrankandthecancellationpropertyforprojectivemodules.
AdistinctivefeatureofhisrcsultsisthatitisnottheBassstablerankofthealgebrawhichisimportant,butratherthatoftheendomorphismalgebraofthemodulebeingcancelled.
HisresultsareinthespiritofQuestions3and4totheeffectthatcancellationholdsfor"sufficicntlylarge"modules,butnowsizeismeasuredintermsofthesizeofthemodulebeingcancelled.
Forexample,fromhisresultsoneobtains:THEOREM3.
LetWbeaprojectiveA-ntoduleandletnbetheBassstablerankofEndo(W).
IfUandYareprojectivemodtilessucltthat(U@Wn)@w=V@W,NON-STABLEK-THEORY,NON-COMN{UTATIVETORI273thenUoWn:V.
Toapplysuchstableranktechniques,oneneedstobeabletoestimatestableranks,andthisisoftenverydifficult.
ButSheu'sworkmentionedabovedependsheavilyonsuccessfulestimatesofstableranks.
andthesameistruefortheresultsaboutnon-commutativetoritobediscussedinthenextsection.
Acrucialtoolisprovidedbyanestimateinthecaseofcrossedproductsbytheintegers,whichcanbeconsideredthemostimportantresultof[3](seeTheorem7.
1).
Specifically:THEOREM4.
LetA*c(ZdenotethecrossedproductofaC*-algebraAbyanactiortaoftheirilegers.
Tlterttsr(AxoZ)identityelcmentweletC-denotethealgebraobtainedbyadjoininganidcntitytoC.
THEOREM6(Theorema.
lloftlTl).
ForanyC*-algebraAandanyn)l,cancellationholdsfor(Aa8,,)-.
Notablymissingaretechniquesforobtainingalowerboundfortsrintheabsenceofeitheradirectlyrelevantcompactspaceorofproperisometries.
Inparticular,nofinitesimpleC*-algebrasareknownforwhichonecanprovethattsr(A))2,althoughtherearcmanypossiblecandidatcs.
ThisisrclatedtothelackofanyexampleofafinitesimpleC*-algebraforu'hichcancellationfails'274MARCA.
RIEFFELsincetsr(A)=Iisequivalenttotheinvertibleelementsbeingdense,andonehas(seeIIL2.
4of[4]and4.
5.
2of[3]):THEOREM7.
IfinvertibleelementsaredenseinA,thenAsatisfiescancellation.
Notice,forexample,thatthisimpliesthatAFC*-algebrashavecancellation.
Thereiscorrespondinglyalackofanyexampleofafinitesimplec*-algebraforwhichonecanshowthattheinvertibleelementsarenotdense.
LetusdiscussnextthefactthatthevariousquestionsstatedinSlaresomewhatinterrelated.
Wegivetwoexamples,whoseproofswillappearin[6].
ThefirstinvolvesQuestions8and10.
THEOREM8.
Letq.
beanautontorphisntoftheunitalC*-algebraAtvltichisintheconnectedconxponentoftheidentityautontorphisntofA,andletc.
alsodenotethecorrespondingactiortofZonA.
SupposethatLEveryelemento/Kr(A)isrepresentedbyaninvertibleelementinAitself.
2.
TlteprojectiortsittAgenerateKo(A).
TheneveryelententinKr(A"oz)isrepresentedbyaninvertibleelententinAxdZ.
ForthenextresultweletTAdenotetheC*-algebraofcontinuousfunctionsfromthecircle,T,toA.
weremarkthatthenTA=AxoZforcrthetrivialaction,anditisaninterestingquestionastowhetherthenexttheoremcanbegeneralizedtothecaseofnon-triviala.
Thistheoreminvolveseuestions2and9.
THEOREM9.
ForaunitalC*-algebraAthefollowingareeqLtivarent:l.
TAsatisfiescancellation.
2.
Botlta)Asatisfiescancellation.
andb)ForeveryprojectiveA-moduleYthenaturalmapfrontAuto(V)/Autf,(V)roKr(A)isinjective.
Theproofofthislasttheoremcomesfromexaminingthefamiliar"clutching"constructionwhichtoanyautomorphismofanA-moduleassociatesaTA-module.
NON-STABLEI(-THEORY,NON-COMMUTATIVETORI2753.
NON-COMMUTATIYETORIBydefinitionanon-commutativetorus,Ag,isaC*-algebradefinedasfollows.
Let0beaskewbilinearformonR',anddefineaskewcocycleoonZnbyo(x,Y)=exP(ni0(x,Y))forx,yZn.
LetAgbethegroupC*-algebraofZ"twistedbyo,i.
e.
,C*(2",o).
ThustoeachxeZnthereisaunitary,ux,inAg,andtheseunitariessatisfythcrelationuyu*=o(x,Y)u**,.
Forn=2oneobtainsthemorefamiliarirrational(andrational)rotationC*-algebras[4].
BytheworkofPimsnerandVoiculescu[11]concerningthecomputationoftheK-groupsofcrossedproductswiththeintegers,onefindsthattheK-groupsofanAgarethesameasthoseforanordinaryn-torusTn(whichistheAOforwhichQ=0)'Inparticular,Ko(Ag)=Zzn-tThisstillleavesquiteopentheproblemofdetcrminingwhatisthcpositiveconeofKo(Ag).
ByusingtechniquesfromtopologicalK-theory,onecanshowthattheanswerforTtbecomescomplicatedforn=4and5(see[16]),andIdonotknowiftheanswerisknownfordimensionsmuchabovethat.
(Also,cancellationalreadyfailsforT5.
)Itturnsouthoweverthatthereisaniceanswerwhen0isnotentirelyrational,inthesensethattherangeof0ontheintegerlatticeZnCR'isnotentirelycontainedintherationalnumbcrs.
Noticethatthereisacanonicaltrace,T,onAg,CorrspondingtoevaluatingattheidentityelementofZn,withitsassociatedhomomorphism,T,fromKo(Ag)intothegroupofrealnumbers.
276MARCA.
RIEFFELThelatterispositiveonthepositiveconeofKo(Ag).
In[16]itisshownthat:THEOREMA.
If0isnotrational,thenthepositiveconeofKo(A6)consistsofexactlytheelententsonwhichtispositive.
weshouldmentionthattherangeofronKo(Ag)hasbeenelucidatedbyElliott[6],whoseworkisanimportantingredientoftheproofsofmostofthetheoremsstatedinthissection.
Muchoftheproofoftheabovetheoreminvolvesaspecificconstruction.
sketchedinU5l,offinitelygeneratedprojectivemodulesoverAg,togetherwithaclassificationofthemodulessoconstructed,bymeansofconnes'cherncharacterintroducedin[5].
Infact,onefindsthateveryelementofKo(Ag)withpositivetraceisrepresentedbyamoduleobtainedbytheconstruction.
Ifoneexaminestheconstructionfurthersoastoobtain,amongothcrthings,informationaboutthetopologicalstablerankoftheendomorphismalgebrasoftheconstructedmodules,onefindsthatonecanapplywarfield'stheorem(Theorem2above)toanswcrQuestion2:THEOREMB.
If0isnotrational,thens(Ag)satisfiescancellatiott.
ThusforsuchIonecananswereuestionl,thatis,onecandescribes(A6).
Evenmore,onehasanexplicitconstructionofallfinitelygeneratedprojectiveA6-modulesuptoisomorphism.
Forthespecialcasen=2theseresultswereobtainedearlierin[14].
Wealsoobtainin!
61ananswertoeuestion8:THEOREMc-If0isnotrational,thentlzeprojectionsinAsgenerateKo(Ag).
ByusingTheorem8oftheprevioussectionwithTheoremCaboveinaninductionargument,wethenobtainthefollowineanswertoQuestionl0:THEOREMD.
IfAisnotrational,theneveryelententofKr(Ag)isrepresentedbyaninvertibleelementolAe.
ByusingTheorem9oftheprevioussectionwithTheoremBwealsoobtainthefollowinganswertoQuestion9:THEOREME.
If0isnotrational,thenthenaturalnlaDfrontU1(A0)/U(Ag)toKr(Ag)isanisontorphisnt.
NON-STABLEK.
THEORY,NON-COMMUTATIVETORI277Fromthistheoremtogetherwithsomeadditionalargumcnt,oncobtainsthefollowinganswertoQuestion7:THEOREMF.
rf0isnotrational,thenanytwoprojectiortsinM-(Ag)whichrepresentthesanteelemento/K6(Ag)areintltesameconnectedcomponentofthesetofprojectionsirrM-(Ag)'Inclosing,letmementionthatJ.
A.
Packer[9,l0]hasstudiedthealgebrasc*(G,o)whereGisthediscreteHeisenberggroupandoisacocycleonG.
Amongmanyotherresults,shehasshownthatformanyo's,thesealgebrassatisfycancellation'Forthissheuses,inpart,thetechniquesof[13,l4].
tllt)1L-lt3lREFERENCESBass,H.
,AlgebraicK-theory,(Benjamin,NewYork,1968)'Blackadar,8.
,uAstablecancellationtheoremforsimpleC+-algebras,"Proc.
LondonMath.
Soc.
47(1983)'303-305'"NotesonthestructureofprojcctionsCTIlgEbras,"SemesterberichtFunktionalanalysis,insimpleTubingen,Winter-semester,1982/83.
l,4landHandelman,D.
,"DimensionfunctionsandtracesonT*-atgebras,"J.
FunctionalAnal.
45(1982),297-340't5lConnes,A.
,"C*-algbbresetgdomdtriediff6rentielle,"C'R'Acad.
Sci.
Paris290(1980),599-604.
t6lElliott,G.
A.
,"OntheK-theoryofthec*-algebrageneratedbyaprojectiverepresentationofatorsion-freediscreteabeliangroup,"pp.
19l'250inOperatorAlgebrasandGrottpRepresentations,vol.
I(Pitman,London,1984)'t7lHerman,R.
H.
andVaserstein,L.
N.
,"ThestablerangeofC*-algebras,"Invent.
Math'77(1984),553-555't8lHusemoller,D.
,FiberBtmdles(Springer-Vcrlag,NewYork'HeidelbergandBerlin,1966).
tl0]_,"C*-algcbrasgeneratedofthediscreteHeisenberggroupappear.
27819lPacker,associatedMARCA.
RIEFFELJ.
A.
.
"K-thcoretictotransformationsandinvariantsforC*-algebrasinduccdflows,"preprint.
byprojcctiverepresentationsI,II,"J.
FunctionalAnal.
,toovcrthedoctoraI"Trans.
Fl]Pimsner,M.
Y.
andVoiculescu,D.
,"ExactsequencesforK-groupsandExt-groupsofcertaincrossedproductC*-algebras,"J.
OperatorTheory4(1980),93-llg.
il2]Rieffel,M.
A.
,"Moritaequivalenceforoperatoralgebras,"inOperatorAlgebrasandApplicatiorts,(R.
V.
Kadison,ed.
),proc.
Symp.
PureMath.
38,pp.
285-298,(AmericanMathematicalSociety,Providence,1982).
[l3]-,''DimenSionandstabierankintheK.
theoryofC*-algebras,"Proc.
LondonMath.
Soc.
47(19g3),295-302.
[4]--,"Thecancellationtheoremforprojectivemodulesoverirrationalrotationc*-algebras,"proc.
LondonMath.
Soc.
47(1983),285-302.
II5]_,"'Yectorbundles'overhigherdimensional'non-commutativetori',"proc.
conferenceonoperatorAlgebras,ConnectionswithTopologyandErgodicTheory,LectttreNotesinMath.
,1r32,pp.
456-467(Springer-verlag,BerlinandHeidelberg,1985).
tl6l"Projcctivemodulesoverhigherdimensionalnon-commutativetori,"preprint.
[l7]Shcu,A.
J.
-L.
,"ThecancellationpropertyformodulesgroupC*-algebrasofcertainnilpotentLiegroups,"dissertation,IJniversityofCalifornia,Berkeley,19g5.
II8]Swan,R.
,"Vectorbundlesandprojectivemodulcs,Amer.
Math.
Soc.
105(1962),264-277.
[19]Taylor,J.
L.
,"Banachalgebrasandtopology,"iDAlgebrasinAnalysis,pp.
Il8-186,(Academicpress,Newyork,Igl5).
I20)vaserstein,L.
N.
,"ThefoundationsofalgebraicK-theory,"UspekhiMat.
Nauk.
3l(1976),87-149.
NON-STABLEK-THEORY,NON-CON{MUTATIVEToRI2T9[21]Warficld,R.
8.
,"Cancellationofmodulesandgroupsandstablerangeofendomorphismrings,"PacificJ'Math'91(1980),457-485.
DEPARTMENTOFMATHEMATICSUNIVERSITYOFCALIFORNIABERKELEY.
CALIFORNIA94'720
- santeeset相关文档
- resultantesetnod32id
- pantaesetnod32id
- stuckesetnod32id
- alarmsesetnod32id
- Examplesesetnod32id
- Useresetnod32id
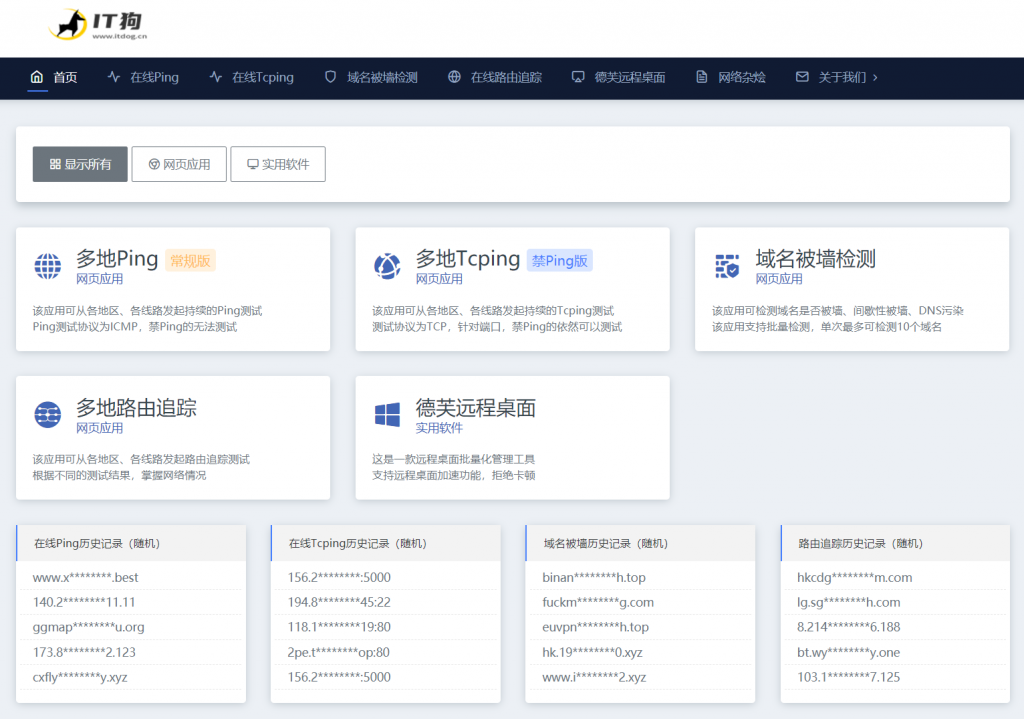
【IT狗】在线ping,在线tcping,路由追踪
IT狗为用户提供 在线ping、在线tcping、在线路由追踪、域名被墙检测、域名被污染检测 等实用工具。【工具地址】https://www.itdog.cn/【工具特色】1、目前同类网站中,在线ping 仅支持1次或少量次数的测试,无法客观的展现目标服务器一段时间的网络状况,IT狗Ping工具可持续的进行一段时间的ping测试,并生成更为直观的网络质量柱状图,让用户更容易掌握服务器在各地区、各线...
DogYun27.5元/月香港/韩国/日本/美国云服务器,弹性云主机
DogYun怎么样?DogYun是一家2019年成立的国人主机商,称为狗云,提供VPS及独立服务器租用,其中VPS分为经典云和动态云(支持小时计费及随时可删除),DogYun云服务器基于Kernel-based Virtual Machine(Kvm)硬件的完全虚拟化架构,您可以在弹性云中,随时调整CPU,内存,硬盘,网络,IPv4路线(如果该数据中心接入了多条路线)等。DogYun弹性云服务器优...

无忧云:洛阳BGP云服务器低至38.4元/月起;雅安高防云服务器/高防物理机优惠
无忧云怎么样?无忧云,无忧云是一家成立于2017年的老牌商家旗下的服务器销售品牌,现由深圳市云上无忧网络科技有限公司运营,是正规持证IDC/ISP/IRCS商家,主要销售国内、中国香港、国外服务器产品,线路有腾讯云国外线路、自营香港CN2线路等,都是中国大陆直连线路,非常适合免备案建站业务需求和各种负载较高的项目,同时国内服务器也有多个BGP以及高防节点。一、无忧云官网点击此处进入无忧云官方网站二...

esetnod32id为你推荐
-
abolishingios11Max163动设备管理解决买家googleDescriptionios5联通版iphone4s联通版iPhone4s 用联通3G卡好还是移动的好icloudiphone怎么利用iCloud使iPhone内存达到扩容目的micromediaMacromedia Fireworks 8是什么东西,怎么用?css3按钮如何在html添加一个搜索框和一个按钮重庆电信测速重庆电信对BT开始限制了?