numbers98成人网
98成人网 时间:2021-04-11 阅读:()
MoosaeiFixedPointTheoryandApplications2014,2014:98http://www.
fixedpointtheoryandapplications.
com/content/2014/1/98RESEARCHOpenAccessCommonxedpointsforsomegeneralizedcontractionpairsinconvexmetricspacesMohammadMoosaei**Correspondence:m.
moosaei@basu.
ac.
irDepartmentofMathematics,Bu-AliSinaUniversity,Hamedan,IranAbstractThepresentstudyfocusesonprovingtheexistenceofcoincidencepointsforself-mappingssatisfyingageneralizedcontractiveconditionwithintheframeworkofconvexmetricspaces.
Theexistenceofcommonxedpointsforweaklycompatibleself-mappingsaswellasBanachoperatorpairsundercertaingeneralizedcontractionsinaconvexmetricspaceisalsoestablished.
MSC:47H09;47H10;47H19;54H25Keywords:Banachoperatorpairs;coincidencepoints;commonxedpoints;compatiblemappings;convexmetricspaces;xedpoints;weaklycompatiblepair1IntroductionandpreliminariesIn,Takahashi[]introducedthenotionofconvexityinmetricspacesandprovedthatallnormedspacesandtheirconvexsubsetsareconvexmetricspaces.
Healsogavesomeexamplesoftheconvexmetricspaceswhicharenotembeddedinanynormed/Banachspaces.
AfterwardGuay,SinghandWhittield[],BegandAzam[],Beg,Azam,AliandMinhas[],ShimizuandTakahashi[],Ciric[],Beg[,],BegandAbbas[],andmanyotherauthorshavestudiedxedpointtheoremsinconvexmetricspaces.
Inthispaper,weintroduce(α,β,γ,η)-generalizedcontractionpairsandstudytheexis-tenceofacoincidencepointforsuchpairsinaconvexmetricspaceundercertaincondi-tions(seeTheorem.
).
Consequently,weprovetheexistenceofacommonxedpointforweaklycompatiblemappingsandalsoBanachoperatorpairswhichare(α,β,γ,η)-generalizedcontractionpairs(seeTheorem.
andTheorem.
).
Wenowreviewnotationsanddenitionsneeded.
WedenotebyNandRthesetofnaturalnumbersandthesetofrealnumbers,respectively.
WealsodenotebyItheidentitymapping.
Inwhatfollows,(X,d)isametricspace,andCisanonemptysubsetofX.
Denition.
LetSandTbetwoself-mappingsofC.
ApointxofCiscalled(i)axedpointofTifTx=x,(ii)acommonxedpointofthepair(S,T)ifSx=Tx=x,and(iii)acoincidencepointofthepair(S,T)ifSx=Tx.
ThesetofxedpointsofTisdenotedbyF(T).
Thesetofcommonxedpoints(respec-tively,coincidencepoints)ofthepair(S,T)isdenotedbyF(S,T)(respectively,C(S,T)).
NotethatC(I,T)=F(T).
Denition.
LetSandTbetwoself-mappingsofC.
ThemappingTiscalled2014Moosaei;licenseeSpringer.
ThisisanOpenAccessarticledistributedunderthetermsoftheCreativeCommonsAttribu-tionLicense(http://creativecommons.
org/licenses/by/2.
0),whichpermitsunrestricteduse,distribution,andreproductioninanymedium,providedtheoriginalworkisproperlycited.
MoosaeiFixedPointTheoryandApplications2014,2014:98Page2of8http://www.
fixedpointtheoryandapplications.
com/content/2014/1/98(i)acontractionifthereexistsk∈[,)suchthatd(Tx,Ty)≤kd(x,y)forallx,y∈C,(ii)anS-contractionifthereexistsk∈[,)suchthatd(Tx,Ty)≤kd(Sx,Sy)forallx,y∈C,(iii)nonexpansiveifd(Tx,Ty)≤d(x,y)forallx,y∈C,and(iv)S-nonexpansiveifd(Tx,Ty)≤d(Sx,Sy)forallx,y∈C.
Denition.
LetSandTbetwoself-mappingsofC.
Thepair(S,T)issaidtobe(i)commutingifSTx=TSxforallx∈C,(ii)R-weaklycommuting[]ifthereexistsR>suchthatd(STx,TSx)≤Rd(Sx,Tx)forallx∈C.
IfR=,thenthemappingsarecalledweaklycommuting[],(iii)compatible[]iflimn→∞d(STxn,TSxn)=,whenever{xn}∞n=isasequenceinCsuchthatlimn→∞Sxn=limn→∞Txn=xforsomex∈C,and(iv)weaklycompatibleiftheycommuteonC(S,T)i.
e.
STx=TSxforallx∈C(S,T)(see[,]formoredetails).
Itiswellknownthatcommutingmappingsareweaklycommuting,andweaklycommut-ingmappingsareR-weaklymappings.
Moreover,R-weaklymappingsarecompatible,andcompatiblemappingsareweaklycompatible.
Thefollowingexampleshowsthattheconversesoftheaboveresultsarenottrueingeneral.
Example.
LetX=Rwiththeusualmetricd(x,y)=|x–y|forallx,y∈X,wehave:()LetC=[,].
LetSx=xandTx=xforallx∈C.
ItistrivialthatSandTareweaklycommutingbutarenotcommuting.
()LetC=[,∞].
ConsiderSx=x–andTx=xforallx∈C.
ThenSandTare-weaklycommutingbutarenotweaklycommuting(see[]).
()LetC=X,Sx=x,Tx=x,x∈C.
ThenSandTarecompatiblebutarenotR-weaklycommuting(see[,,]formoredetails).
()LetC=[,],anddeneself-mappingsSandTofCbyS()=,S(x)=iforηorη,thenηorηorη<α+γholds.
CompetinginterestsTheauthordeclaresthattheyhavenocompetinginterests.
AcknowledgementsTheauthorisgratefultothereviewersfortheirvaluablecommentswhichimprovedthecontentsofthemanuscript.
Received:28November2013Accepted:27March2014Published:16Apr2014MoosaeiFixedPointTheoryandApplications2014,2014:98Page8of8http://www.
fixedpointtheoryandapplications.
com/content/2014/1/98References1.
Takahashi,T:AconvexityinmetricspacesandnonexpansivemappingI.
KodaiMath.
Semin.
Rep.
22,142-149(1970)2.
Guay,MD,Singh,KL,Whiteld,JHM:Fixedpointtheoremsfornonexpansivemappingsinconvexmetricspaces.
In:ProceedingsofConferenceonNonlinearAnalysis.
LectureNotesinPureandAppliedMathematics,vol.
80,pp.
179-189.
Dekker,NewYork(1982)3.
Beg,I,Azam,A:Fixedpointonstar-shapedsubsetsofconvexmetricspaces.
IndianJ.
PureAppl.
Math.
18,594-596(1987)4.
Beg,I,Azam,A,Ali,F,Minhas,T:Somexedpointtheoremsinconvexmetricspaces.
Rend.
Circ.
Mat.
PalermoXL,307-315(1991)5.
Shimizu,T,Takahashi,W:Fixedpointtheoremsincertainconvexmetricspaces.
Math.
Jpn.
37,855-859(1992)6.
Ciric,L:Onsomediscontinuousxedpointtheoremsinconvexmetricspaces.
Czechoslov.
Math.
J.
43(188),319-326(1993)7.
Beg,I:Structureofthesetofxedpointsofnonexpansivemappingsonconvexmetricspaces.
Ann.
Univ.
MariaeCurie-Skodowska,Sect.
ALII,7-14(1998)8.
Beg,I:Inequalitiesinmetricspaceswithapplications.
Topol.
MethodsNonlinearAnal.
17,183-190(2001)9.
Beg,I,Abbas,M:FixedpointsandbestapproximationinMengerconvexmetricspaces.
Arch.
Math.
41,389-397(2005)10.
Pant,RP:Commonxedpointsofnoncommutingmappings.
J.
Math.
Anal.
Appl.
188,436-440(1994)11.
Sessa,S:Onaweakcommutativityconditionofmappingsinxedpointconsiderations.
Publ.
Inst.
Math.
32,149-153(1982)12.
Jungck,G:Compatiblemappingsandcommonxedpoints.
Int.
J.
Math.
Math.
Sci.
9,771-779(1986)13.
Jungck,G,Rhoades,BE:Fixedpointforsetvaluedfunctionswithoutcontinuity.
IndianJ.
PureAppl.
Math.
29(3),227-238(1998)14.
Chugh,R,Kumar,S:Commonxedpointsforweaklycompatiblemaps.
Proc.
IndianAcad.
Sci.
Math.
Sci.
111,241-247(2001)15.
Jungck,G:Commonxedpointsforcommutingandcompatiblemapsoncompacta.
Proc.
Am.
Math.
Soc.
103,978-983(1988)16.
Jungck,G:CommonxedpointtheoremsforcompatibleselfmapsofHausdortopologicalspaces.
FixedPointTheoryAppl.
3,355-363(2005)17.
Chen,J,Li,Z:Commonxed-pointsforBanachoperatorpairsinbestapproximation.
J.
Math.
Anal.
Appl.
336,1466-1475(2007)18.
Hussain,N:CommonxedpointsinbestapproximationforBanachoperatorpairswithCirictypeI-contractions.
J.
Math.
Anal.
Appl.
338,1351-1363(2008)19.
Agarwal,RP,O'Regan,D,Sahu,DR:FixedPointTheoryforLipschitzian-TypeMappingswithApplications.
Springer,Heidelberg(2009)20.
Hussain,N,Abbas,M,Kim,JK:CommonxedpointandinvariantapproximationinMengerconvexmetricspaces.
Bull.
KoreanMath.
Soc.
48,671-680(2008)21.
Moosaei,M:Fixedpointtheoremsinconvexmetricspaces.
FixedPointTheoryAppl.
2012,ArticleID164(2012).
doi:10.
1186/1687-1812-2012-16410.
1186/1687-1812-2014-98Citethisarticleas:Moosaei:Commonxedpointsforsomegeneralizedcontractionpairsinconvexmetricspaces.
FixedPointTheoryandApplications2014,2014:98
fixedpointtheoryandapplications.
com/content/2014/1/98RESEARCHOpenAccessCommonxedpointsforsomegeneralizedcontractionpairsinconvexmetricspacesMohammadMoosaei**Correspondence:m.
moosaei@basu.
ac.
irDepartmentofMathematics,Bu-AliSinaUniversity,Hamedan,IranAbstractThepresentstudyfocusesonprovingtheexistenceofcoincidencepointsforself-mappingssatisfyingageneralizedcontractiveconditionwithintheframeworkofconvexmetricspaces.
Theexistenceofcommonxedpointsforweaklycompatibleself-mappingsaswellasBanachoperatorpairsundercertaingeneralizedcontractionsinaconvexmetricspaceisalsoestablished.
MSC:47H09;47H10;47H19;54H25Keywords:Banachoperatorpairs;coincidencepoints;commonxedpoints;compatiblemappings;convexmetricspaces;xedpoints;weaklycompatiblepair1IntroductionandpreliminariesIn,Takahashi[]introducedthenotionofconvexityinmetricspacesandprovedthatallnormedspacesandtheirconvexsubsetsareconvexmetricspaces.
Healsogavesomeexamplesoftheconvexmetricspaceswhicharenotembeddedinanynormed/Banachspaces.
AfterwardGuay,SinghandWhittield[],BegandAzam[],Beg,Azam,AliandMinhas[],ShimizuandTakahashi[],Ciric[],Beg[,],BegandAbbas[],andmanyotherauthorshavestudiedxedpointtheoremsinconvexmetricspaces.
Inthispaper,weintroduce(α,β,γ,η)-generalizedcontractionpairsandstudytheexis-tenceofacoincidencepointforsuchpairsinaconvexmetricspaceundercertaincondi-tions(seeTheorem.
).
Consequently,weprovetheexistenceofacommonxedpointforweaklycompatiblemappingsandalsoBanachoperatorpairswhichare(α,β,γ,η)-generalizedcontractionpairs(seeTheorem.
andTheorem.
).
Wenowreviewnotationsanddenitionsneeded.
WedenotebyNandRthesetofnaturalnumbersandthesetofrealnumbers,respectively.
WealsodenotebyItheidentitymapping.
Inwhatfollows,(X,d)isametricspace,andCisanonemptysubsetofX.
Denition.
LetSandTbetwoself-mappingsofC.
ApointxofCiscalled(i)axedpointofTifTx=x,(ii)acommonxedpointofthepair(S,T)ifSx=Tx=x,and(iii)acoincidencepointofthepair(S,T)ifSx=Tx.
ThesetofxedpointsofTisdenotedbyF(T).
Thesetofcommonxedpoints(respec-tively,coincidencepoints)ofthepair(S,T)isdenotedbyF(S,T)(respectively,C(S,T)).
NotethatC(I,T)=F(T).
Denition.
LetSandTbetwoself-mappingsofC.
ThemappingTiscalled2014Moosaei;licenseeSpringer.
ThisisanOpenAccessarticledistributedunderthetermsoftheCreativeCommonsAttribu-tionLicense(http://creativecommons.
org/licenses/by/2.
0),whichpermitsunrestricteduse,distribution,andreproductioninanymedium,providedtheoriginalworkisproperlycited.
MoosaeiFixedPointTheoryandApplications2014,2014:98Page2of8http://www.
fixedpointtheoryandapplications.
com/content/2014/1/98(i)acontractionifthereexistsk∈[,)suchthatd(Tx,Ty)≤kd(x,y)forallx,y∈C,(ii)anS-contractionifthereexistsk∈[,)suchthatd(Tx,Ty)≤kd(Sx,Sy)forallx,y∈C,(iii)nonexpansiveifd(Tx,Ty)≤d(x,y)forallx,y∈C,and(iv)S-nonexpansiveifd(Tx,Ty)≤d(Sx,Sy)forallx,y∈C.
Denition.
LetSandTbetwoself-mappingsofC.
Thepair(S,T)issaidtobe(i)commutingifSTx=TSxforallx∈C,(ii)R-weaklycommuting[]ifthereexistsR>suchthatd(STx,TSx)≤Rd(Sx,Tx)forallx∈C.
IfR=,thenthemappingsarecalledweaklycommuting[],(iii)compatible[]iflimn→∞d(STxn,TSxn)=,whenever{xn}∞n=isasequenceinCsuchthatlimn→∞Sxn=limn→∞Txn=xforsomex∈C,and(iv)weaklycompatibleiftheycommuteonC(S,T)i.
e.
STx=TSxforallx∈C(S,T)(see[,]formoredetails).
Itiswellknownthatcommutingmappingsareweaklycommuting,andweaklycommut-ingmappingsareR-weaklymappings.
Moreover,R-weaklymappingsarecompatible,andcompatiblemappingsareweaklycompatible.
Thefollowingexampleshowsthattheconversesoftheaboveresultsarenottrueingeneral.
Example.
LetX=Rwiththeusualmetricd(x,y)=|x–y|forallx,y∈X,wehave:()LetC=[,].
LetSx=xandTx=xforallx∈C.
ItistrivialthatSandTareweaklycommutingbutarenotcommuting.
()LetC=[,∞].
ConsiderSx=x–andTx=xforallx∈C.
ThenSandTare-weaklycommutingbutarenotweaklycommuting(see[]).
()LetC=X,Sx=x,Tx=x,x∈C.
ThenSandTarecompatiblebutarenotR-weaklycommuting(see[,,]formoredetails).
()LetC=[,],anddeneself-mappingsSandTofCbyS()=,S(x)=iforηorη,thenηorηorη<α+γholds.
CompetinginterestsTheauthordeclaresthattheyhavenocompetinginterests.
AcknowledgementsTheauthorisgratefultothereviewersfortheirvaluablecommentswhichimprovedthecontentsofthemanuscript.
Received:28November2013Accepted:27March2014Published:16Apr2014MoosaeiFixedPointTheoryandApplications2014,2014:98Page8of8http://www.
fixedpointtheoryandapplications.
com/content/2014/1/98References1.
Takahashi,T:AconvexityinmetricspacesandnonexpansivemappingI.
KodaiMath.
Semin.
Rep.
22,142-149(1970)2.
Guay,MD,Singh,KL,Whiteld,JHM:Fixedpointtheoremsfornonexpansivemappingsinconvexmetricspaces.
In:ProceedingsofConferenceonNonlinearAnalysis.
LectureNotesinPureandAppliedMathematics,vol.
80,pp.
179-189.
Dekker,NewYork(1982)3.
Beg,I,Azam,A:Fixedpointonstar-shapedsubsetsofconvexmetricspaces.
IndianJ.
PureAppl.
Math.
18,594-596(1987)4.
Beg,I,Azam,A,Ali,F,Minhas,T:Somexedpointtheoremsinconvexmetricspaces.
Rend.
Circ.
Mat.
PalermoXL,307-315(1991)5.
Shimizu,T,Takahashi,W:Fixedpointtheoremsincertainconvexmetricspaces.
Math.
Jpn.
37,855-859(1992)6.
Ciric,L:Onsomediscontinuousxedpointtheoremsinconvexmetricspaces.
Czechoslov.
Math.
J.
43(188),319-326(1993)7.
Beg,I:Structureofthesetofxedpointsofnonexpansivemappingsonconvexmetricspaces.
Ann.
Univ.
MariaeCurie-Skodowska,Sect.
ALII,7-14(1998)8.
Beg,I:Inequalitiesinmetricspaceswithapplications.
Topol.
MethodsNonlinearAnal.
17,183-190(2001)9.
Beg,I,Abbas,M:FixedpointsandbestapproximationinMengerconvexmetricspaces.
Arch.
Math.
41,389-397(2005)10.
Pant,RP:Commonxedpointsofnoncommutingmappings.
J.
Math.
Anal.
Appl.
188,436-440(1994)11.
Sessa,S:Onaweakcommutativityconditionofmappingsinxedpointconsiderations.
Publ.
Inst.
Math.
32,149-153(1982)12.
Jungck,G:Compatiblemappingsandcommonxedpoints.
Int.
J.
Math.
Math.
Sci.
9,771-779(1986)13.
Jungck,G,Rhoades,BE:Fixedpointforsetvaluedfunctionswithoutcontinuity.
IndianJ.
PureAppl.
Math.
29(3),227-238(1998)14.
Chugh,R,Kumar,S:Commonxedpointsforweaklycompatiblemaps.
Proc.
IndianAcad.
Sci.
Math.
Sci.
111,241-247(2001)15.
Jungck,G:Commonxedpointsforcommutingandcompatiblemapsoncompacta.
Proc.
Am.
Math.
Soc.
103,978-983(1988)16.
Jungck,G:CommonxedpointtheoremsforcompatibleselfmapsofHausdortopologicalspaces.
FixedPointTheoryAppl.
3,355-363(2005)17.
Chen,J,Li,Z:Commonxed-pointsforBanachoperatorpairsinbestapproximation.
J.
Math.
Anal.
Appl.
336,1466-1475(2007)18.
Hussain,N:CommonxedpointsinbestapproximationforBanachoperatorpairswithCirictypeI-contractions.
J.
Math.
Anal.
Appl.
338,1351-1363(2008)19.
Agarwal,RP,O'Regan,D,Sahu,DR:FixedPointTheoryforLipschitzian-TypeMappingswithApplications.
Springer,Heidelberg(2009)20.
Hussain,N,Abbas,M,Kim,JK:CommonxedpointandinvariantapproximationinMengerconvexmetricspaces.
Bull.
KoreanMath.
Soc.
48,671-680(2008)21.
Moosaei,M:Fixedpointtheoremsinconvexmetricspaces.
FixedPointTheoryAppl.
2012,ArticleID164(2012).
doi:10.
1186/1687-1812-2012-16410.
1186/1687-1812-2014-98Citethisarticleas:Moosaei:Commonxedpointsforsomegeneralizedcontractionpairsinconvexmetricspaces.
FixedPointTheoryandApplications2014,2014:98
Friendhosting,美国迈阿密机房新上线,全场45折特价优惠,100Mbps带宽不限流量,美国/荷兰/波兰/乌兰克/瑞士等可选,7.18欧元/半年
近日Friendhosting发布了最新的消息,新上线了美国迈阿密的云产品,之前的夏季优惠活动还在进行中,全场一次性45折优惠,最高可购买半年,超过半年优惠力度就不高了,Friendhosting商家的优势就是100Mbps带宽不限流量,有需要的朋友可以尝试一下。Friendhosting怎么样?Friendhosting服务器好不好?Friendhosting服务器值不值得购买?Friendho...

819云互联 香港 日本 美国 2核4G 18元 8核8G 39元 免费空间 免费CDN 香港 E3 16G 20M 230元/月
819云互联是海外领先的互联网业务平台服务提供商。专注为用户提供低价高性能云计算产品,致力于云计算应用的易用性开发,并引导云计算在国内普及。目前平台研发以及运营云服务基础设施服务平台(IaaS),面向全球客户提供基于云计算的IT解决方案与客户服务,拥有丰富的海外资源、香港,日本,美国等各国优质的IDC资源。官方网站:https://www.819yun.com香港特价物理服务器:地区CPU内存带宽...
华为云(69元)828促销活动 2G1M云服务器
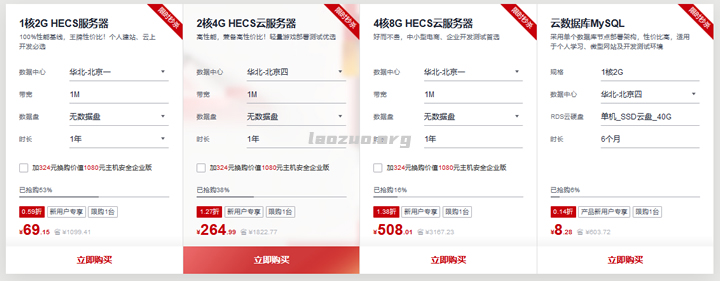
华为云818上云活动活动截止到8月31日。1、秒杀限时区优惠仅限一单!云服务器秒杀价低至0.59折,每日9点开抢秒杀抢购活动仅限早上9点开始,有限量库存的。2G1M云服务器低至首年69元。2、新用户折扣区优惠仅限一单!购云服务器享3折起加购主机安全及数据库。企业和个人的优惠力度和方案是不同的。比如还有.CN域名首年8元。华为云服务器CPU资源正常没有扣量。3、抽奖活动在8.4-8.31日期间注册并...
98成人网为你推荐
-
toupianaoeiu后面是什么?phpcms模板phpcms模板下载后如何安装重庆网络公司一九互联网络公司做一个网站大概需要多少钱苹果appstore宕机apple id登陆不了app store怎么办新iphone也将禁售iPhone8plus在2020年还会有货吗什么是支付宝支付宝是什么意思?360免费建站怎样给360免费自助建站制作的企业网站做一级域名解析绑定?www.topit.me提供好的图片网站密码cuteftp资费标准中国移动4g18元套餐介绍