U1kkk755.com
kkk755.com 时间:2021-04-07 阅读:()
AmethodtoextractinstantaneousfeaturesoflowfrequencyoscillationbasedontrajectorysectioneigenvaluesZijunBIN1,2,YushengXUE1,2AbstractAffectedbythenonlineartime-varyingfactorsduetofaultscenarios,protectionrelaying,andcontrolmeasures,thedynamicbehaviorsofapowersystemmaybesignicantlydifferentfromtheresultsofpreviousmethods.
Inordertoanalyzetheoscillationcharacteristicsofcomplexpowersystemsmoreaccuratelyandsuppressthelowfrequencyoscillationmoreeffectively,thispaperimprovesthetrajectorysectioneigenvaluemethod.
Firstly,thetimeresponseofasystemisobtainedbynumericalsimulationinagivenfaultscenario.
Secondly,thealge-braicvariablesaresubstitutedtothedifferentialequationsalongthetrajectory.
Thus,theoriginaltime-varyingdif-ferential-algebraicequationsareapproximatedbyasetoflinearordinarydifferentialequations,whichcanbeupdatedalongthetrajectory.
Onthisbasis,thispaperproposesamethodtoextractinstantaneousfeaturesoftheoscillationfromthemicroperspective.
Thenon-equilibriumpointswithstrongnonlinearityorcriticaleigenmodesareidenti-edbytheproposedmethod.
ThesimulationtestresultsoftheIEEE3-machine9-bussystemandtheNewEnglandsystemillustratethevalidityoftheproposedmethod.
KeywordsLowfrequencyoscillation,Nonlinearfactor,Time-varyingfactor,Trajectorysectioneigenvalue,Instantaneousfeature1IntroductionInrecentyears,thelowfrequencyoscillation(LFO)hasbecomeincreasinglyintricate,withtheenlargementofpowergrids[1],theintegrationofrenewableenergyandthedevelopmentofhighvoltagedirect-currenttransmis-sion[2,3].
Theuniversalityandaccuracyofpreviousanalysisapproachesforsmalldisturbancestabilitycanhardlysatisfytherequirementsofmodernpowersystems.
Inuencedbytheessentialnonlinearfactorsandtime-varyingfactorsduetofaultscenarios,protectionrelaying,andcontrolmeasures,theresultsobtainedbytraditionalmethodsmaybesignicantlydifferentwiththedynamicbehaviorsofapowersystem[4].
Hence,itisurgenttoputforwardanLFOanalysismethodthatcandescribethedynamicbehaviorsofapowersystemmoreaccuratelywithacompleteconsiderationofthenonlineartime-varyingfactors.
Generally,LFOscanbedividedintothreeformsbasedonthecauses:steadyincreaseingeneratorrotorangleduetolackofsynchronizingtorque;`rotoroscillationwithincreasingamplitudeduetolackofsufcientdampingtorque[5];sustainedoscillationduetoperiodicdistur-banceresultingfromfailuresormalfunctioningequipment[6].
Theeigen-analysismethodismostwidelyusedinpractice.
Itdescribesthedynamicsystembehaviorsneartheequilibriumpointbyalineartime-invariantsystem.
However,thenonlinearityofapowersystemmayleadtobifurcationandblow-upofsolutions[7,8].
Reference[9]addressesthenonlinearsingularityphenomenonbyHopfCrossCheckdate:21May2019Received:3December2018/Accepted:21May2019/Publishedonline:10July2019TheAuthor(s)2019&ZijunBINbinzijun@gmail.
comYushengXUExueyusheng@sgepri.
sgcc.
com.
cn1SchoolofElectricalEngineering,ShandongUniversity,Jinan250061,China2StateGridElectricPowerResearchInstitute,Nanjing211000,China123J.
Mod.
PowerSyst.
CleanEnergy(2019)7(4):753–766https://doi.
org/10.
1007/s40565-019-0556-zbifurcationtheory,whichdemonstratesthatthedynamicbehaviorsofasimplepowersystemcouldbedifferentfromtheresultsofeigen-analysis.
However,limitedbytheheavycomputationinlarge-scalesystems,thismethodisstillunderinvestigationaswellinmethodologicalaspectsasinconcreteapplications.
Inordertoconsidertheeffectsofnonlinearfactors,thenormalformtheory[10]andthemodalseriesmethod[11]areproposed.
Moredetailedinformationofeigenmodesisextractedbysolvingthehigh-orderapproximateequations.
Onemajordrawbackoftheseapproachesisthatthesolutionsareobtainedattheequilibriumpoint,neglectingtime-varyingfactors.
Forthesakeofconsideringtheeffectsofrandomfactors,proba-bilitymodelsareusedtoimprovetheuniversalityoftra-ditionalmethods[12].
Allofthesemethodsreviewedthusfar,however,sufferfromthelimitationofdisturbancescenarios.
Asaresult,somecriticalfactorsofapowersystemsuchasthenon-differentiablecomponentsandcontrolmeasuresareignored[13].
Toentirelyanalyzetheinuenceofnonlineartime-varyingfactorsonpowersystemoscillation,itisquitenecessarytoextractinformationfromtrajectories.
Somesignalanalysistechniquesareusedtoidentifytheoscilla-tionmodals.
Commonapproachesliketime-frequencytransformationstakethedisturbedtrajectoryasresearchobject.
Thefrequencyfeaturesareidentiedbydecouplingfrequencycomponents,andthedampingfeaturesareextractedbydifferentiatingmodeamplitudeswithrespecttotime.
Asaclassicalmethod,thePronyanalysisreplacesthetrajectorybyasetofexponentialcomponents[14].
Thismethodcandescribethedynamicbehaviorsinanintervalapproximately,thoughtheinuencesoftime-varyingfac-torsareaveraged.
Basedonwide-areameasurementsignalsandtheproperorthogonaldecomposition(POD)method,[15]usesdynamicmodedecoupling(DMD)methodtoachieveoscillationmodesdecouplingandfeaturesextracting.
Nevertheless,thesemethodscanhardlydescribetheinstantaneouscharacteristics,whicharetime-varying.
Theempiricalmodedecoupling(EMD)methodextractsinstantaneousfeaturesbydecomposingtheorigi-nalsignalintolocallysymmetriccomponentsonthebasisofenvelopecharacteristicsandtheHilberttransform[16].
However,theprecisionofEMDmethodisstronglyaffec-tedbythefrequenciesofadjacentcomponents.
Toextractinstantaneousfeaturesmoreaccurately,windowPronymethod[17],andwindowfastFouriertransformation(ridges)method[18]useslidewindowstodividetheentiretimetoasetofoverlappingperiodsalongthetrajectory.
Thefeaturesequenceiscomposedofthemodalsofallwindows.
Despiteofthat,theaccuracyofthemethodsislimitedbythewidthofthewindow.
Moreover,thetrun-catederrorcausedbytheedgesonbothsidesofthesignalisinevitable.
Toimprovetheadaptiveabilityofthetime-frequencytransformationapproaches,wavelet(ridge)methodisintroducedtoassistanalyzingLFOs[19].
Researchesshowthatthemethodcanidentifythemodalsofanactualtrajectorywithnoiseseffectively[20],althoughthechoiceofwaveletbasisreliesonempiricalparameters.
Reference[21]approximatestheobservedprocessasalinearsystemformoreinformationofmodels.
Thestabilitymarginsareobtainedasposteriorprobabilitiesthatthepolesoftheestimatedsystemareunstable.
Aconsiderablenumberofresearcheshavebeendonetoachievetheextractionofinstantaneousfeaturesbysignalanalysistechniquesduringthelastdecades.
Tobeobjec-tive,theseapproachesareinstrumentalinidentifyingmodalsonline.
However,allthesementionedmethodssufferfromaseriouslimitation.
Theessenceofsignalanalysistechniquesisidenticationoftheaveragechar-acteristicsinatimewindow.
Thetimescaleoftheoscil-lationanalysisislimitedbytheresolutionofthemethods.
Ontheotherhand,themajorgoalofmodalidenticationofLFOistosuppresstheoscillation.
Tolocatecriticalgen-eratorsorparameters,thesignalanalysistechniqueshavetobeuniedwiththemodelsattheequilibriumpoint,whichcanhardlyreecttherealdynamicbehaviorsofanonlineartime-varyingsystem.
Attemptstoresolvethisdilemmahaveresultedintheinventionoftrajectorysectioneigenvalue(TSE)theory,whichextendstheconceptofeigenvaluefromtheequi-libriumpointtoothernon-equilibriumpointsofthetra-jectory.
Thepioneerworkcanbetracedto[22].
Reference[23]attemptstouseTSEmethodtoanalyzetransientsta-bility.
Themethodisfurtherdevelopedtoestimatetheenergyofasystembeforethevisualfarendpointorthevisualdynamicsaddlepoint[24].
Reference[25]concludesthattheglobalstabilityoflineartime-varyingsystemscannotbedeterminedbyTSEs.
Themajorreasonisthattheglobaldynamicsoftime-varyingsystemsareaffectedbythekineticenergyatnon-equilibriumpoints,whichcouldnotbeconsideredbyTSEmethod.
However,[26]hasprovedthattheeffectsofthekineticenergycanbeignoredwhenanalyzingtheinstantaneouscharacteristicsinoneintegrationstep.
Meanwhile,themathematicalandphysicalfoundationsofTSEmethodhavebeengiveninthisliterature.
Thecontributionsofthispaperarethreefold.
Firstly,itimprovesthemathematicsofTSEmethodtogiveabetterexplanationofthephysicalmeaningoftheeigenvaluesatnon-equilibriumpoints.
Basedontheimprovedtheory,thispaperproposesamethodtoextracttheinstantaneousfea-turesofLFOs.
Secondly,thepaperproposesthecriteriontoidentifythenon-equilibriumpointswithstrongnonlinear-ity,andillustratesthemechanismofnonlinearoscillationsinpowersystem.
Thirdly,itproposesthecriteriontoidentifythedangerousnon-equilibriumpointsandthe754ZijunBIN,YushengXUE123criticaleigenmodes.
Comparedwithpreviousmethods,thecriticalgeneratorsandparametersidentiedatabovenon-equilibriumpointscanachievebetterinhibitioneffects.
2Mathematicsoftrajectorysectioneigenvalues2.
1Piecewise-linearizedsystemmodelsandsolutionintimedomainThebehaviorsofadynamicsystem,suchasapowersystem,maybedescribedbyasetofnrst-ordernonlineardifferential-algebraicequations(DAEs)asthefollowingform(thederivativesofthestatevariablesarenotexplicitfunctionsoftime)[5]:_XfX;Y0gX;Y&1ThecolumnvectorXisreferredasthestatevector,anditsentriesxiasthestatevariables,suchasrotoranglesandangularvelocitiesoftherotors.
ThecolumnvectorYisreferredasthealgebraicvector,anditsentriesyiasthealgebraicvariables,suchasnodevoltagesandnodecurrentinjections.
ThesolutionsofXandYinthetimedomaincanbeobtainedbynumericalsimulationingivenfaultscenarios.
BysubstitutingYintothedifferentialequations_XfX;Yandlinearizingthenonlinearcomponentsofthefunctionsalongthetrajectory,theDAEsarereplacedbyasetoflinearordinarydifferentialequations(ODEs).
Foranillustrativepurpose,thelinearODEattkistakenasanexampletointroduceTSEmethod.
D_XkDtAkDXkDtBk2whereAkofoXXtkistheJacobimatrixofthenonlinearfunctionf;BkfXtk;Ytkistheinitialvaluesoftherightsideofthedifferentialequationattk.
SupposingthatUkistherightmodalmatrixofAk.
NewstatevectorisdenedbyZkDtU1kDXkDt.
Bothsidesof(2)areleft-multipliedbyU1k:_ZkDtU1kAkUkZkDtU1kBk3Solve(3)withtheinitialvaluesoftheelementsofZkDtrespecttoDt(Zk00),whichmeanstheincrementaltimeintk;tk1.
ZkDtCkEkDtK1kU1kBk4whereEkDtekk;1Dt;ekk;2Dtekk;nDtT;KkU1kAkUkdiag(kk;1;kk;2;kk;nistheeigenvaluesofthestatematrixAkattk.
TwoprocedureparametermatricesDkandCkaredenedasfollows:Dkdk;1dk;2.
.
.
dk;n26664377751kk;1vk;11f1vk;12f2vk;1nfn1kk;2vk;21f1vk;22f2vk;2nfn.
.
.
1kk;nvk;n1f1vk;n2f2vk;nnfn2666666666643777777777755Ckdk;1000dk;20.
.
.
.
.
.
.
.
.
00dk;n26664377756wheredk,iistheithelementofDk;vk;ijistheelementintheithrowandthejthcolumnofU1k;fiistheithelementofBk.
Bothsidesof(4)areleft-multipliedbyUk:DXkDtUkCkEkDtA1kBk7Obviously,(7)describesthedynamicbehaviorsofthechangesofstatevariablesintk;tk1.
ThevaluesofUkCkindicatethedegreesofeigenmodesexcitation[27].
2.
2PhysicalmeaningoftrajectorysectioneigenvaluesThechangesofthestatevariablesrespecttoDthavebeenobtainedby(7)inthetimedomain.
ConsideringthattheinitialvaluesofZk(Dt)arezero,wehaveZk0CkEk0K1kU1kBk0.
Equation(7)canberearrangedto:DXkDtUkCkEkDtUkCkEk08Assumingtheexistenceofanewsystem,ttkDt,thestatevectorofthissystemXk,new(t)satises:Xk;newtUkCkEkttk9Therefore,thechangesofthenewstatevectorintk;tk1canbeexpressedby:DXk;newDtXk;newtXk;newtkUkCkEkttkUkCkEk0DXkDt10Accordingto(10),thedynamicbehaviorofthenewsystemisthesamewiththeoriginalsystem,ignoringtheunbalancedpowerintk;tk1.
Thestateequationofthenewsystemcanbederivedfrom(9).
Amethodtoextractinstantaneousfeaturesoflowfrequencyoscillationbasedontrajectory…755123_Xk;newtUkCkKkEkttkUkCkKkUkCk1Xk;newt11CkandKkarebothdiagonalmatrices,andtheexpressionin(11)mustsatisfyUkCkKkUkCk1UkKkU1kAk.
Equation(11)canberearrangedas:_Xk;newtAkXk;newt12Thus,theoriginalsystemwithoutregardtounbalancedpowerisapproximatedbyalinearsystemintk;tk1.
AlthoughXk,new(t)maybedifferentfromXk(t),thechangesoftheirstatevectorsarethesameintk;tk1.
Thesectioneigenvalueskk;1;kk;2;kk;ndescribethedynamicbehavioroftheimaginarysystemintk;tk1.
Besides,theparametermatricesUkandCkareupdatedalongthetrajectoryaccordingtotheresultsofnumericalsimulation.
Theeffectoftheunbalancedpowerisreectedinfollowingtimesections.
Furthermore,theaccumulateerrorisavoidedbyupdatingtheparametersof(7)and(12)alongthetrajectory.
Consequently,theeffectsofnonlinearfactorsandtime-varyingfactorsareconsideredbythesectionstatematrixchangingwithtime.
3ExtractionmethodofinstantaneousfeaturesofLFOToextracttheinstantaneousfeaturesofLFO,theeigenvaluesofthesectionstatematrixarecalculated.
Ingeneral,ann-ordermatrixhasneigenvalues,whichmayconsistofcouplesofcomplexeigenmodesandrealeigenmodes.
Acoupleofcomplexeigenmodesrepresentasinusoidalcomponent,whilearealeigenmoderepresentsanexponentialcomponent.
Inthispaperfk;i;insisusedtorepresenttheimaginarypartoftheitheigenvalueattk,dividedbyaconstant2p.
rk;i;insisusedtorepresenttherealpartoftheitheigenvalueattk.
Asforacomplexroot,fk;i;insrepresentstheinstantaneousfrequencycharacteristicoftheitheigenmodeattk.
rk;i;insdescribesthetrendoftheamplitudeoftheitheigenvalueattk.
Asforarealroot,fk;i;insiszero.
rk;i;insrepresentstherateofatrendchangewithinoneintegrationstep.
3.
1IdenticationofpointswithstrongnonlinearityInanoscillation,acoupleofcomplexeigenmodesmayreversiblytransformintotworealeigenmodesoccasion-ally.
Aone-machineinnitebus(OMIB)systemisusedtoilluminatethemechanismofthevariationofTSEs.
ThestructureoftheOMIBsystemisshowninFig.
1.
Vrepresentsthevoltageoftheinnitebus.
Peistheinputpower.
ThesummationofreactanceisdenotedasXR.
E0anddarereferredasthemoduleandangleofthetransientelectromotanceafterthetransientreactanceofthegener-ator,respectively.
Theclassicalmodelisusedtorepresentthegenerator.
ThedynamicequationsofthegeneratorcanbefoundinAppendixA(A1).
Whenadisturbanceoccurs,theTSEscanbeobtainedalongthetrajectory:kk;1;2D2MD24MKkp2M13whereDisthedampingtorquecoefcientofthegenerator;KkE0Vcosdk=XRrepresentstheslopeoftheelectro-magneticpower-rotoranglecurveattk,geometrically;dkisthevalueoftherotorangleattkwithavaluerangeof0;p.
Ascanbeexpected,ifdk20;arccosD2XR=4ME0V,kk;1;2wouldbeacoupleofcomplexroots.
Withtheincreaseofdk,theimaginarypartsofkk;1;2decrease.
Ifdk2arccosD2XR=4ME0V;p,kk;1;2wouldbetwodifferentrealroots.
Withtheincreaseofdk,oneofthemdecreases,andtheotherincreases.
Therefore,thenon-equilibriumpointswithstrongnonlinearitycanbeidenti-edbrieybelow:aggregatingthecomplexeigenmodesateachtimesection;`comparingthenumbersofthecomplexeigenmodesatnon-equilibriumpointstotheequilibriumpoint;thosenon-equilibriumpointsaresigned,thenumberofwhichisinconsistentwiththeequilibriumpoint.
Theconceptofthenon-equilibriumpointswithstrongnonlinearitycanbeusedtoexplainsomecomplexphe-nomena.
Forinstance,thedistortionofarotorangletra-jectoryisinducednearfarendpoints(FEPs)asshowninFig.
2a,c.
TheangularaccelerationandvelocityapproachtozerowhenFEPsareclosetothedynamicsaddlepoint(DSP).
Figure2bdepictstheevolutionoftheimaginarypartsoftheTSEs,theeigenmodesofwhichtransfertotheexponentialcomponentsfromtheoscillationcomponents.
3.
2IdenticationofcriticalpointsAtwo-machinesystemispresentedinFig.
3tointro-ducethecriticalnon-equilibriumpointsingeneralsystem.
TheTSEsofthistwo-machinesystemafterbeingdis-turbedcanbeoftwoforms:acoupleofcomplexroots,arealroot(canbezero),andazeroroot;`threerealrootsGV0°EPeXΣδFig.
1One-machineinnitebussystem756ZijunBIN,YushengXUE123(canbezero)andazeroroot.
Equation(12)suggeststhatthepositivesignsoftherealpartsofTSEsrepresenttheinjectionofdangerousenergy.
Thus,thecriticalnon-equilibriumpointsareidentiedbythesignsoftherealpartsoftheeigenvaluesalongthetrajectory.
Forthesakeofillustratingtherelationsbetweeninstantaneousfeaturesandglobaldynamics,Fig.
4ana-lyzesatypicalconvergentoscillationandatypicaldiver-gentoscillationinthetwo-machinesystem.
AsshowninFig.
4a,inaconvergentoscillation,therealpartsofmode3couldbepositiveperiodically.
AsshowninFig.
4b,inadivergentoscillation,therealpartsofmode1andmode2arenotalwayspositive.
Intheneighborhoodoftheequilibriumpoint,theTSEsareconsistentwiththeeigenvaluesattheequilibriumpoint.
However,powersystemisanessentialnonlineardynamicsystem.
Oncetheoperatingpointofthesystemrunsawayfromtheequilibriumpoint,thedynamicbehaviorsmaybedifferentwiththeeigenvaluesattheequilibriumpoint.
Inalinearsystem,theacceleratedpowergraduallyincreaseswiththedecreasingrelativeangularvelocityfromDCPtoFEP.
However,affectedbythenonlinearityofthePe–dcurve,theacceleratedpowerofapowersystemislowerthanthelinearsystembeforetheFEP.
Asaresult,thedampingcharacteristicsnearFEPswouldbeworsethantheneighborhoodoftheequilibriumpoint.
TheclosertheFEPistotheDSP,thelowerthestabilitymarginofthesystemsuffers,andthetrajectoriesoftherealpartsofTSEschangecorrespondingly.
Figure4a,bdepictstheevolutionofrk;i;insinconvergentanddivergentoscillation,-10121.
01.
52.
02.
5(a)Trajectoryofrotorangle1.
01.
52.
02.
500.
51.
5(b)Trajectoryoffk,i,ins000.
51.
01.
52.
02.
5-0.
50.
51.
01.
52.
0(c)Pe-δcurveThefaultoccursThefaultiscleanedDistortionofthetrajectoryt(s)3δ(rad)ImaginarypartsoftheTSEsarezero1.
02.
0t(s)fk,i,ins(Hz)-1.
0δ(rad)-0.
5Pe(p.
u.
)FEP2FEP1PeDSPPmFig.
2DistortionphenomenonofrotoranglenearFEPsG1G2LoadFig.
3Two-machinesystem123456-2-1012(a)Trajectoriesofσk,i,insinconvergentrotorangleoscillation123450-3-1132-2(b)Trajectoriesofσk,i,insindivergentrotorangleoscillation0Mode3Mode2;Mode1;σk,i,inst(s)60t(s)σk,i,insFig.
4RealpartsofTSEsinatwo-machinesystemAmethodtoextractinstantaneousfeaturesoflowfrequencyoscillationbasedontrajectory…757123respectively.
Thevaluesofrk;i;inshaveobviouslypositivecorrelationwiththedampingcharacteristics.
Therefore,thetrajectoriesoftherealpartsofTSEsindicatetheinstantaneousdampingcharacteristics.
Impor-tanceshouldbeattachedtothecriticalnon-equilibriumpointswithpositiverealpartsofTSEs.
Tosuppresspowersystemoscillation,itisnecessarytooptimizetheparametersofcriticalgeneratorsorcon-trollers,whichcanbelocatedbytheeigenmodeswithpositiverealparts.
Theparticipationfactorandtheelec-tromechanicalmodecorrelationratiocanalsobeextendedfromtheequilibriumpointtonon-equilibriumpoints.
Section4demonstratestheeffectivenessoftheinstanta-neousfeaturesinlocatingcriticalgeneratorandguidingtheoptimization.
Inaddition,therelativepositionsofeigenvaluesbetweenadjacenttimesectionsarerandom,whichlimitstheapplicationofTSEtheoryinidentifyingcriticaleigenmodes.
Foranillustrativepurpose,assumingthattheeigenvaluesattaandtbareka;1;ka;2;ka;nandkb;1;kb;2;kb;n,respectively.
ka;1mayrelatetokb;2orevenkb;n.
Theeigen-polynomialisacontinuousfunctionoftheparametersofstatematrix,andthezeropointsoftheeigen-polynomialarecontinuousfunctionsoftheparame-tersofeigen-polynomial.
Consequently,theeigenvaluesofamatrixarecontinuousfunctionsoftheparametersofthematrix.
TheTSEsbetweenadjacenttimesectionscanmatchwiththesimilarityoftheirvalues.
3.
3ProcessofinstantaneousfeaturesextractionFigure5showstheproceduretoextractinstantaneousfeatures.
Themethodincludessixstepsasfollows.
Step1:thetime-domainresponsesofstatevariablesareobtainedbynumericalsimulationorphasemeasurementunits.
Step2:thealgebraicvariablesaresubstitutedtothedifferentialequationsalongthetrajectoryandthesectionstatematricesareobtainedbylinearizingthenonlinearcomponentsofthedifferentialequations.
Inthispaper,thesizeofanalysisstepisequaltotheintegrationstep.
Step3:theTSEsarecalculatedfromthestatematrixandtheinstantaneousfeaturesareextractedalongthetrajectory.
Step4:theeigenvaluesbetweenadjacenttimesectionsarematched.
Step5:thepointswithstrongnonlinearityareidentiedbycorrespondingcriterion.
RnandInrepresenttheeldsofrealnumberandimaginarynumber,respectively.
Step6:criticalpointsareidentiedbycorrespondingcriterion.
4Casestudy4.
1IEEE3-machine9-bussystemInthissection,a3-machine9-bussystemisusedtoillustratethelimitationofthetraditionaleigen-analysismethod.
First-orderTaylorseriesexpansionSimplesystemmodelingIsstepsizelargeLinearfitIsanalysisprecisionhighFull-OrdermodelingSectionstatematricescalculationEigenmodesmatchingIsthereeigenmodetransformbetweenRnandInPointwithstrongnonlinearityPointwithweaknonlinearityIsthereanyRealpartofTSEspositiveCriticalPointWell-BehavedPointYNStartEndNYNYNYNumericalsimulatonStepsizedeterminationStep1Step2Step5Step6Step3Step4Fig.
5Flowdiagramofinstantaneousfeaturesextraction758ZijunBIN,YushengXUE123ForthestructureshowninFig.
6,thegeneratorsareallequippedwithgovernors.
Theclassicalmodelisusedtorepresentthegenerator,thetransientelectromotanceE0afterX0disconstant.
ThedynamicequationsofgeneratorandgovernorarelistedinAppendixA.
Theconstantimpedancemodelisusedtorepresenttheload.
Thelinedata,thegeneratordata,andthegovernordataarelistedinAppendixB.
TheeigenvaluesattheequilibriumpointarelistedinTable1andtherealpartsofalleigenvaluesarenegative.
Intheviewofthetraditionaleigen-analysismethod,thetrajectoriesofstatevariablesshouldbeconvergentoscil-lationwhenthesystemisdisturbed.
Ontheotherside,themostdangerouseigenmodeidentiedbythetraditionaleigen-analysismethodshouldbethe3rdand4theigenvalueswiththeminimumrealparts.
Moreover,thecorrespondingclusterofgeneratorsis{G2,G3}vs.
{G1}.
Theoscillationcharacteristicsofthissystemareana-lyzedindifferentfaultscenarios.
Thenumericalintegrationisusedtoassessthevalidityoftheproposedmethod.
4.
1.
1Scenario1:weaknonlineartime-varyingscenarioThedisturbanceisathree-phasetransientfaultinbus6,andthefaultdurationis0.
09s.
Thetrajectoriesoftherotoranglesareconvergentoscillationswithweakdamping,asshowninFig.
7.
Todemonstratethattheinstantaneousfeaturesoftheoscillationareconsistentwiththetraditionaleigen-analysismethodinweaknonlineartime-varyingscenarios,theinstantaneousfeaturesareobtainedasfollows.
AsshowninFig.
8a,afterthefaultdisappears(laterthan0.
09s),inmostofthetime,therearefourcouplesofcomplexeigenmodes,whichrepresentfouroscillationcomponentswithdifferentfrequency.
Accordingtothecriterionforpointswithstrongnonlinearity,therearenononlinearpointsinthetrajectoriesofk1;2,k3;4,andk5;6.
Instead,thenonlinearpointsappearinthetrajectoriesofk7;8,whicharesignedbypurpleblocksinFig.
8c.
Thestrongnonlineareigenmodesandthedangerouseigen-modesmaybedifferent.
AsshowninFig.
8b,thesignsoftherealpartsofk7;8arealwaysnegative,andthesignsoftherealpartsofk3;4arepositiveinsometimesections,whicharesignedbyredblocksinFig.
8c.
Ascanbeexpected,thecriticalpointsarerelatedtothepointswithstrongnonlinearity.
G2G3G1213456789Fig.
6IEEE3-machine9-bussystemTable1EigenvaluesofIEEE3-machine9-bussystematequilibriumpointNumberRealpartImaginarypartClusterofgenerators1-0.
104214.
4300{G1,G2}vs.
{G3}2-0.
104214.
4300{G1,G2}vs.
{G3}3-0.
02568.
7149{G2,G3}vs.
{G1}4-0.
02568.
7149{G2,G3}vs.
{G1}5-0.
24820.
6203{G2}vs.
{G1,G3}6-0.
24820.
6203{G2}vs.
{G1,G3}7-1.
19600.
0763{G1,G2}vs.
{G3}8-1.
19600.
0763{G1,G2}vs.
{G3}9-4.
04470.
0000–10-19.
10850.
0000–11-19.
37790.
0000–12-19.
39790.
0000–13-5.
79040.
0000–14-0.
75610.
0000–15-0.
23430.
0000–160.
00000.
0000–17-0.
02030.
0000–18-0.
01490.
0000–-101-10100.
51.
01.
52.
02.
53.
03.
54.
04.
5t(s)-0.
500.
5δ1(rad)δ2(rad)δ3(rad)Fig.
7Trajectoriesofrotoranglesofsysteminscenario1Amethodtoextractinstantaneousfeaturesoflowfrequencyoscillationbasedontrajectory…7591234.
1.
2Scenario2:strongnonlineartime-varyingscenarioThedisturbanceisathree-phasetransientfaultinbus6,andthefaultdurationis0.
15s.
Thetrajectoriesoftherotoranglesaredivergentoscillations,asshowninFig.
9.
Theinconsistencybetweenthetrajectoriesofrotoranglesandtheeigenvaluesattheequilibriumpointrevealsthelimitationoftraditionaleigen-analysismethodinstrongnonlineartime-varyingscenarios.
Toillustratethemecha-nismoftheoscillationfromthemicropointofview,theinstantaneousfeaturesareobtainedasfollows.
AsshowninFig.
10a,b,afterthefaultdisappears(laterthan0.
15s),pointswithstrongnonlinearityappearinthetrajectoriesofk3;4,k5;6andk7;8.
Thecriticaltimesectionsappearinthetrajectoriesofk3;4andk5;6.
ThepointswithstrongnonlinearityandcriticaleigenmodesaresignedinFig.
10cbypurpleandredblocks,respectively.
Obviously,themostdangerouseigenmodeisk5;6withthemaximumpositivevaluesintheoscillation,asshowninFig.
10b.
Theclusterofthegeneratorsisthesameastheactualinstabilitymode:{G2},{G1,G3}.
Therefore,thecharacteristicsofinstantaneousfeaturescanbesummarizedasfollows:inweaknonlineartime-varyingscenarios,theoperatingpointofthesystemrunsneartheequilibriumpoint,andtheinstantaneousfeaturesaresimilartothetraditionaleigen-analysismethod;`instrongnonlineartime-varyingscenario,theoperatingpointofthesystemrunsfarawayfromtheequilibriumpointperiodically,andtheinstantaneousfeaturesmaybetotallydifferentfromthetraditionaleigen-analysismethod.
4.
2IEEE10-machine39-busNewEnglandsystemIntheviewofthetraditionaleigen-analysismethod,theeigenmodeswithrealpartsgreaterthanzeroaredangerous.
Theeigenmodeswithrealpartsapproximatetozeroaredenedasweakdampingeigenmodes.
ForthesakeofsuppressingLFO,theseeigenmodesshouldbecontrolled.
However,Section4.
1hasrevealedthatthetraditionaleigen-analysismethodcannotdescribethedynamicbehaviorsaccurately.
Moreover,theactuallydangerousorweakdampingeigenmodesmaybeignored.
TheNewEnglandsystemisusedtoilluminatethesuperiorityoftheproposedmethodinlocatingcriticaleigenmodes.
Thestructureofthesystem,thelinedataandthegen-eratordatacanbefoundin[28].
Thesetengeneratorsareallequippedwithgovernors.
Theclassicalmodelisusedtorepresentthegenerator,thetransientelectromotanceE0afterX0dareconstant.
Thedynamicequationsofgenerators-1.
0-0.
500.
5-4.
5-4.
0-6.
0-19.
5-19.
0-18.
500.
51.
52.
53.
54.
5(c)Locationsofpointswithstrongnonlinearityandcriticalpointsinscenario100.
51.
01.
52.
53.
54.
50.
51.
52.
5(a)Trajectoriesoffk,i,insinscenario11.
02.
02.
03.
04.
0t(s)λ7,8λ5,6λ1,2λ3,4fk,i,ins(Hz)λ5,6λ3,4λ1,2λ7,800.
51.
01.
52.
53.
54.
5(b)Trajectoriesofσk,i,insinscenario12.
03.
04.
0t(s)σk,i,insNonlineartimesection;Negativedampingtimesection1.
02.
03.
04.
0t(s)λ9λ14λ13λ10λ11λ12Fig.
8TrajectoriesofTSEsinscenario1-202δ1(rad)-20200.
51.
52.
53.
54.
5t(s)-202δ2(rad)δ3(rad)1.
02.
03.
04.
0Fig.
9Trajectoriesofrotoranglesofsysteminscenario2760ZijunBIN,YushengXUE123andgovernorsareshowninAppendixA.
Theconstantimpedancemodelisusedtorepresenttheload.
Thegov-ernordataarelistedinAppendixC.
Asacomparison,theeigenvaluesattheequilibriumpointarelistedinAppendixD.
Itisobviousthattherealpartsofalleigenvaluesarenegative.
Thedisturbanceisathree-phasetransientfaultinbus15andthefaultdurationis0.
39s.
ThetrajectoriesoftherotoranglesareshowninFig.
11.
Beforethe3rdsecond,theamplitudesoftherotoranglesaredecreasingandthedominantoscillationmodeis{G39}tothesetoftheothergenerators.
Afterthe3rdsecond,theamplitudesoftherotoranglesareincreasingandnallythesystemlosesstability.
Thecriticalmodeis{G34}tothesetoftheothergenerators.
4.
2.
1RealpartsoftrajectorysectioneigenvaluesInstantaneousfeaturesareextractedandtherealpartsoftheTSEsareshowninFig.
12.
Inordertohighlightthecriticaleigenmodes,theothereigenmodesarehidden,thesignsoftherealpartsofwhicharenegativeinalltime.
Inintervals1and2,thecriticalpointsmainlyappearineigenmodek29;30,thesamewiththeeigenvaluesattheequilibriumpoint.
Inintervals3to6,thecriticalpointsmainlyappearinthetrajectoriesofacoupleofneweigenmodes.
Thus,thecriticaleigenmodesthatmainlyaffectthedynamicbehaviorsoftheoscillationshouldbethenewcouple.
4.
2.
2LocationofcriticalgeneratorInordertolocatethecriticalgenerator,itisnecessarytocalculatetheparticipationfactorsofcriticaleigenmodesatcriticaltimesections.
Thegeneratorwithhighestpartici-pationfactorinoneintervalisinvariant.
Foranillustrativepurpose,theresultsofeigenmodesanalysesintypicaltimesectionsareshowninTable2.
Inintervals1and2,k29andk30arethemostdominanteigenmodeswiththehighestparticipationfactorsandthecriticalgeneratorisG39.
Inintervals3to6,theneweigenmodesarethemostdominanteigenmodeswiththehighestparticipationfactor,andthecriticalgeneratorisG34.
Thedescriptionsoftheinstantaneousfeaturesarethe00.
51.
52.
53.
54.
5(c)Locationsofpointswithstrongnonlinearityandcriticalpointsinscenario2t(s)1.
02.
03.
04.
0Nonlineartimesection;Negativedampingtimesection00.
51.
52.
53.
54.
5-20-15-10-5051015(b)Trajectoryofσk,i,insinscenario2σk,i,ins1.
02.
03.
04.
0λ7,8λ3,4λ5,6λ1,200.
51.
52.
53.
54.
50.
51.
52.
5(a)Trajectoryoffk,i,insinscenario21.
02.
03.
04.
0fk,i,ins(Hz)1.
02.
0λ1,2λ3,4λ5,6λ7,8t(s)λ9λ13λ10λ11,12λ14t(s)Fig.
10TrajectoriesofTSEsinscenario2-3-2-10123123456780δ1(rad)t(s)G30;G32;G33;G34G35;G36;G37;G38;G39G31;Fig.
11TrajectoriesofrotoranglesofIEEE10-machine39-bussystemAmethodtoextractinstantaneousfeaturesoflowfrequencyoscillationbasedontrajectory…761123sameastheactualtrajectoriesoftherotorangles,asshowninFig.
11.
Consideringthattheelectromechanicalmodecorrelationratiosoftheseeigenmodesaremuchlargerthan1,thestatevariablesparticipatingintheeigenmodesaremainlyrotoranglesandangularvelocities.
Tosuppresstheoscillation,thedampingtorquecoef-cientofG34isup-regulatedby10%,andthenumericalsimulationshowsthatthetrajectoriesoftherotoranglesturntobeconvergent,asshowninthelefthalfofFig.
13.
Bycomparison,thedampingtorquecoefcientofG39isup-regulatedby50%andtrajectoriesarestilldivergentaftertheoptimization,asshownintherighthalfofFig.
13.
5ConclusionBasedonTSEs,thispaperproposesamethodtoextractinstantaneousoscillationfeaturesfromthemicroperspec-tive.
TobetterillustratethemechanismofpowersystemoscillationandeffectivelysuppresstheLFO,thecriteriafornon-equilibriumpointswithstrongnonlinearityandcriticaleigenmodesareproposed.
Atnon-equilibriumpointswithstrongnonlinearity,thedynamicbehaviorsmaybetotallydifferentfromtheeigenvaluesattheequilibriumpoint.
Thecriticalpointsrarelyappearinweaknonlineartime-varyingscenarios.
Eventhoughtheoscillationisconvergent,therealpartsofTSEsmaybepositiveatsomenon-equilibriumpointswithstrongnonlinearity.
Theinstabilityofoscilla-tionsmaybecausedbytheaccumulationeffectsofthecriticalnon-equilibriumpoints,whichmassivelyappearinstrongnonlineartime-varyingscenarios.
Moreover,simu-lationresultsclearlyindicatetheenhancedaccuracyofcriticalgeneratorslocation.
6DiscussionOverthelast3decades,therotoranglestabilityhasbeencharacterizedindividuallyintotwosubcategories:small-disturbancestabilityandtransientstability.
Asaresult,itisimpossibletoanalyzetherelationshipbetweenoscillationstabilityandsynchronousstabilitywithinthecurrentframework.
Thedisturbancesinsomecasestudiesofthispaperbelongtothetraditionaltransientstabilitycategory,forthreereasons:1)Itisdifculttodistinguishsmalldisturbanceandlargedisturbance.
Inanegativedampingoscillationdenedbythetraditionalmethod,acomplexdynamicprocessisinevitablebeforesynchronousinstability.
Thus,thisstudybeginswiththeaimofstudyingthemechanismandcharacteristicsoftheevolutionofLFO,nomatterthedisturbanceislargeorsmall.
AlthoughTSEmethodcannotbeusedtoanalyzetheglobalstability-4-2024607.
0Interval1Interval5σk,i,ins8t(s)7.
56.
06.
55.
05.
54.
04.
53.
03.
52.
02.
51.
01.
50.
5λ40(neweigenmode);λ41(neweigenmode)λ29(originaleigenmode);λ30(originaleigenmode);Fig.
12TrajecotiesofrealpartsofcriticaleigenmodesinIEEE10-machine39-bussystemTable2Analysesofeigenmodesattypicaltimesectionsfromintervals1to6IntervalnumberTypicaltimesection(s)HighestparticipatinggeneratorParticipationfactorElectromechanicalmodecorrelationratio11.
00G390.
139789.
593422.
20G390.
84089.
367033.
50G340.
50481102.
774045.
20G340.
4824709.
611855.
80G340.
4781717.
336766.
20G340.
4774623.
5736762ZijunBIN,YushengXUE123ofapowersystem,simulationtestresultsindicateastrongcorrelationbetweentheoscillationcharacteris-ticsandtheproposedfeatures.
2)Inordertostudythestabilityofapowersystem,auniedframeworkhasbeenproposedby[29]tounifytheanalysisapproachesoftheoscillationstabilityandthetransientstabilityintermsofenergy.
TheimprovedTSEmethodandtheachievementsofthispapercontributetotheuniedframework.
ThispaperisatentativestudyonunifyingsmalldisturbanceanalysisandlargedisturbanceanalysisthroughTSEmethod.
3)Theeigenvaluescanonlydescribethedynamicbehaviorsintheneighboringregionofonepoint,whetherequilibriumpointornon-equilibriumpointitis.
Toanalyzetheoscillationcharacteristicsinstrongnonlineartime-varyingscenarios,thestatematrixandthevariablesareupdatedalongthetrajectory,accord-ingtothenumericalresults.
Finally,theproposedmethodwouldbeusedinengi-neeringonlywhenthetraditionaleigen-analysiscannotdescribethedynamicbehaviorsaccurately,ordetailedanalysisisrequiredinsomecomplexscenarios.
Thus,comparedwiththetraditionaleigen-analysis,theincreasingcomputationisacceptable.
Thequantityofinformationandcomputationcanbebalancedbydeterminingthestepsizemoreintelligently.
Moreover,maturemethodshavebeenusedtoreducethedimensioncursewhencalculatingeigenvaluesattheequilibriumpoint.
Thetheoremoftheinverseoperatorcanbeusedtodeterminethecirclewheretheeigenvalueslie.
Theradiusofthecirclecanbeesti-matedbyGerschgorintheory.
Manyresultshavebeenmadeinthiseld[30].
Theseachievementscanalsobeusedtoestimatethedistributionoftheeigenvaluesatthenon-equilibriumpoints.
AcknowledgementsThisworkwassupportedbyScienceandTechnologyProgramofStateGridCorporationofChina(TheoreticalBasis,AlgorithmandApplicationofTrajectoryEigenvalueMethod).
OpenAccessThisarticleisdistributedunderthetermsoftheCreativeCommonsAttribution4.
0InternationalLicense(http://creativecommons.
org/licenses/by/4.
0/),whichpermitsunrestricteduse,distribution,andreproductioninanymedium,providedyougiveappropriatecredittotheoriginalauthor(s)andthesource,providealinktotheCreativeCommonslicense,andindicateifchangesweremade.
AppendixAdddtxdxdt1MPmPeDx8>:A1wheredrepresentstheangleoftherotors,xrepresentstheanglevelocityoftherotor;Mrepresentstheinertialofgenerator;Pmrepresentsthemechanicaltorque;andPerepresentstheelectromagnetictorque.
InthedatalistsofAppendixesBandC,thedirect-axistransientreactanceofgeneratorisdenotedbyX0d,thequadratureaxissyn-chronousreactanceisdenotedbyXq,thedampingtorquecoefcientisdenotedbyD.
12345678-3-2-101-3-2-101230δ(rad)t(s)δ(rad)G30;G32;G33;G34G31;G35;G36;G37;G38;G392Fig.
13TrajectoriesofrotoranglesofIEEE10G39NsystemafterparameteradjustmentsofG34andG39,respectivelyAmethodtoextractinstantaneousfeaturesoflowfrequencyoscillationbasedontrajectory…763123dldtrdrdt1TGTplnTGrd[nRDdldt1TdRlnd(Pm2ldt2TwlPm8>>>>>>>>>>>>>>>>>:A2wherel,r,nrepresentthestatevariablesofgovernorsandwaterturbines,asshowninFig.
A1;Kprepresentsthegainofgovernor;Rrepresentstheregulationcoefcientofgovernor;TGandTprepresenttheservotimeconstantandthepilotvalvetimeconstantofgovernor;TdandDdrep-resentsthesoftfeedbacktimeconstantandcoefcientofgovernors;andTwrepresentsthewaterstartingtimeoftheturbine,AppendixBThelinedata,generatordataandgovernordataforIEEE3-machine9-bussystemaregiveninTablesA1,A2andA3,whereVlimitrepresentsthelimitspeedsofwatergate.
AppendixCThegovernordataforIEEE10-machine39-bussystemaregiveninTableA4.
P0R+Kpωωref++ηξ1++PUP.
1TG(1+sTp)PDOWN.
σ1sμ1sTw1+sTw/2PmRsDdTd1+sTdξ2Fig.
A1DiagramsofgovernorandwaterturbineTableA1LinedataforIEEE3-machine9-bussystemTypeStartpointTerminationpointResistance(p.
u.
)Reactance(p.
u.
)Groundcapacitance(p.
u.
)Line450.
03000.
25500.
2640Line460.
01700.
09200.
0790Line570.
09600.
48300.
4590Line690.
09780.
33200.
1290Line780.
02550.
21600.
2235Line890.
03570.
30240.
3135Transformer140.
00000.
0576–Transformer270.
00000.
0625–Transformer390.
00000.
0586–TableA2GeneratordataforIEEE3-machine9-bussystemGeneratornumberInertia(s)X0d(p.
u.
)Xq(p.
u.
)D(p.
u.
)G123.
64000.
06080.
06080.
8000G26.
40000.
11980.
11980.
4000G33.
01000.
18130.
18130.
7000TableA3GovernordataforIEEE3-machine9-bussystemGovernornumberKp(p.
u.
)R(p.
u.
)TG(s)Tp(s)GH10.
50000.
03000.
40000.
0500GH23.
50000.
03000.
15000.
0460GH30.
50000.
03000.
40000.
0500GovernornumberTd(s)Tw(s)Dd(p.
u.
)Vlimit(p.
u.
)GH15.
00000.
50000.
2000±0.
0500GH25.
00000.
50000.
2000±0.
0500GH37.
50001.
50000.
2000±0.
0500TableA4GovernordataforIEEE10-machine39-bussystemGovernornumberKp(p.
u.
)R(p.
u.
)TG(s)Tp(s)GH301.
00000.
03000.
40000.
0500GH311.
00000.
03000.
40000.
0500GH3218.
00000.
03000.
40000.
0500GH3315.
00000.
03000.
40000.
0500GH3413.
00000.
03000.
40000.
0500GH3515.
00000.
03000.
40000.
0500GH3620.
00000.
03000.
10000.
0800GH3720.
00000.
03000.
10000.
0800GH3812.
00000.
03000.
40000.
0500GH391.
00000.
03000.
40000.
0500GovernornumberTd(s)Tw(s)Dd(p.
u.
)Vlimit(p.
u.
)GH305.
00000.
50000.
2000±0.
0500GH315.
00000.
50000.
2000±0.
0500GH325.
00000.
50000.
2000±0.
0500GH335.
00000.
50000.
2000±0.
0500GH345.
00000.
50000.
2000±0.
0500GH355.
00000.
50000.
2000±0.
0500GH365.
00000.
50000.
1000±0.
0500GH375.
00000.
50000.
1000±0.
0500GH385.
00000.
50000.
2000±0.
0500GH395.
00000.
50000.
2000±0.
0500764ZijunBIN,YushengXUE123AppendixDTheeigenvaluesofIEEE10-machine39-bussystematequilibriumpointaregiveninTableA5.
References[1]XueY,YuX(2017)Beyondsmartgrid-cyber-physical-socialsysteminenergyfuture.
ProcIEEE105(12):2290–2292[2]WeiS,ZhouY,HuangY(2017)Synchronousmotor-generatorpairtoenhancesmallsignalandtransientstabilityofpowersystemwithhighpenetrationofrenewableenergy.
IEEEAccess5:11505–11512[3]AnZ,ShenC,ZhengZTetal(2019)Scenario-basedanalysisandprobabilityassessmentofsub-synchronousoscillationcausedbywindfarmswithdirect-drivenwindgenerators.
JModPowerSystCleanEnergy7(2):243-253[4]AbedEH,VaraiyaPP(1984)Nonlinearoscillationsinpowersystems.
IntJElectrPowerEnergySyst6(1):37–43[5]KundurP(1994)Powersystemstabilityandcontrol.
McGrawHill,NewYork,pp699–736[6]YeH,LiuY,ZhangPetal(2017)Analysisanddetectionofforcedoscillationinpowersystem.
IEEETransPowerSyst32(2):1149–1160[7]AjjarapuV,LeeB(1992)Bifurcationtheoryanditsapplicationtononlineardynamicalphenomenainanelectricalpowersys-tem.
IEEETransPowerSyst7(1):424–431[8]SidorovN,SidorovD,LiY(2018)Basinsofattractionofnonlinearsystems'equilibriumpoints:stability,branchingandblow-up.
ActaMathSci.
https://arxiv.
org/pdf/1807.
05487.
pdf.
Accessed10October2018[9]AyasunS,NwankpaCO,KwatnyHG(2004)Computationofsingularandsingularityinducedbifurcationpointsofdifferen-tial-algebraicpowersystemmodel.
IEEETransCircuitsSyst51(8):1525–1538[10]LiuS,MessinaAR,VittalV(2005)Assessingplacementofcontrollersandnonlinearbehaviorusingnormalformanalysis.
IEEETransPowerSyst20(3):1486–1495[11]RodriguezO,MedinaA,MessinaARetal(2009)Themodalseriesmethodandmulti-dimensionalLaplacetransformsfortheanalysisofnonlineareffectsinpowersystemsdynamics.
In:ProceedingsofIEEEPESgeneralmeeting,Calgary,Canada,26–30July2009,pp1–8[12]WangKW,ChungCY,TsangCTetal(2001)ProbabilisticeigenvaluesensitivityindicesforrobustPSSsiteselection.
IEEEProcGenerTransmDistrib148(6):603–609[13]YeH,MouQ,LiuY(2017)Calculationofcriticaloscillationmodesforlargedelayedcyber-physicalpowersystemusingpseudo-spectraldiscretizationofsolutionoperator.
IEEETransPowerSyst32(6):4464–4476[14]GrundCE,PaserbaJJ,HauerJFetal(1993)ComparisonofPronyandeigen-analysisforpowersystemcontroldesign.
IEEETransPowerSyst8(3):964–971[15]BarocioE,PalBC,ThornhillNFetal(2015)Adynamicmodedecompositionframeworkforglobalpowersystemoscillationanalysis.
IEEETransPowerSyst30(6):2902–2912[16]HanS,XuZ,SunBetal(2010)DynamiccharacteristicanalysisofpowersysteminterareaoscillationsusingHHT.
IntJElectrPowerEnergySyst32(10):1085–1090[17]BracaleA,CaramiaP,CarpinelliG(2007)AdaptivePronymethodforwaveformdistortiondetectioninpowersystems.
IntJElectrPowerEnergySyst29(5):371–379[18]PeterOS(2000)Theuseofslidingspectralwindowsforparameterestimationinpowersystemdisturbancemonitoring.
IEEETransPowerSyst15(4):1261–1267[19]RuedaJL,JuarezCA,ErlichI(2011)Wavelet-basedanalysisofpowersystemlow-frequencyelectromechanicaloscillations.
IEEETransPowerSyst26(3):1733–1743[20]JinT,LiuS,FleschRCCetal(2017)Amethodfortheiden-ticationoflowfrequencyoscillationmodesinpowersystemssubjectedtonoise.
ApplEnergy206:1379–1392[21]SidoroyD,PanasetskyD,SˇmadlV(2010)Non-stationaryautoregressivemodelforon-linedetectionofinter-areaoscil-lationsinpowersystems.
In:ProceedingsofIEEEPESISGTEurope,Gothenburg,Sweden,11–13October2010,pp1–5[22]PanX,XueY,ZhangXetal(2008)Analyticalcalculationofpowersystemtrajectoryeigenvaluesanditserroranalysis.
AutomElectricPowerSyst32(19):10–14[23]TanW,ShenC,LiuFetal(2012)Apracticalcriterionfortrajectoryeigenvaluesbasedtransientstabilityanalysis.
AutomElectricPowerSyst36(16):14–19TableA5EigenvaluesofIEEE10-machine39-bussystematequi-libriumpointNumberRealpartImaginarypartNumberRealpartImaginarypart1-19.
40150.
000031-4.
33000.
00002-19.
3980.
000032-4.
22410.
00003-19.
39610.
000033-4.
18980.
00004-19.
33860.
000034-4.
15800.
00005-19.
32760.
000035-4.
13770.
00006-19.
30810.
000036-4.
01380.
00007-19.
3130.
000037-4.
00740.
00008-19.
31550.
000038-4.
00210.
00009-9.
53350.
000039-1.
31160.
000010-9.
24140.
000040-0.
30800.
542711-0.
13268.
563341-0.
3080-0.
542712-0.
1326-8.
563342-1.
05050.
000013-0.
21248.
632643-0.
19880.
000014-0.
2124-8.
632644-0.
75840.
000015-0.
23668.
278445-0.
77790.
000016-0.
2366-8.
278446-0.
77750.
000017-0.
09217.
096247-0.
7770.
000018-0.
0921-7.
096248-0.
76260.
000019-0.
21307.
202349-0.
76570.
000020-0.
2130-7.
202350-0.
76850.
000021-0.
12906.
2769510.
00000.
000022-0.
1290-6.
276952-0.
02890.
000023-0.
15305.
758653-0.
02430.
000024-0.
1530-5.
758654-0.
01980.
000025-0.
10655.
247555-0.
01990.
000026-0.
1065-5.
247556-0.
01990.
000027-6.
78670.
000057-0.
01990.
000028-6.
15960.
000058-0.
01980.
000029-0.
01323.
506059-0.
01980.
000030-0.
0132-3.
506060-0.
01980.
0000Amethodtoextractinstantaneousfeaturesoflowfrequencyoscillationbasedontrajectory…765123[24]XueY,HaoL,WuQHetal(2010)AnnotationforFEPandDSPintermsoftrajectorysectioneigenvalues.
AutomElectricPowerSyst34(12):1–7[25]PanX,XueY,JuP(2013)Reconsiderationoftrajectoryeigenvaluemethod.
AutomElectricPowerSyst37(23):37–44[26]XueY,BinZ(2019)Trajectorysectioneigenvaluemethodfornonlineartime-varyingpowersystem.
IntJElectrPowerEnergySyst107:321–331[27]NiJ,ShenC,TanWetal(2010)Acoherenceidentifyingmethodbasedonlinearizationatnon-equilibriumpoint.
AutomElectricPowerSyst34(20):7–12[28]PaiMA(1989)Energyfunctionanalysisforpowersystemstability.
KluwerAcademicPublishersGroup,Boston,pp223–226[29]XueY(2018)AUniedenergy-domaintheoryofrotor-anglestabilities.
https://dwz.
cn/Ee9i5GnQ.
Accessed20August2018[30]ShestopalVE(1998)StrengtheningoflocalizationtheoremsofGersˇgorinandBrauer.
FundamentalnayaIPrikladnayaMatematika4(1):345–358ZijunBINreceivedhisB.
E.
degreefromHuazhongUniversityofScienceandTechnology,Wuhan,China,in2013.
Currently,heispursuinghisPh.
D.
degreeinShandongUniversity,Jinan,China.
Hisresearchinterestsincludepowersystemstabilityandcontrol.
YushengXUEreceivedtheB.
E.
degreefromShandongUniversity,Jinan,China,in1963,andM.
S.
degreefromStateGridElectricPowerResearchInstitute,Nanjing,China,in1981,respectively.
HereceivedthePh.
D.
degreeinelectricalengineeringfromUniversityofLie`ge,Lie`ge,Belgium,in1987.
Dr.
XueisamemberofChineseAcademyofEngineering,HonoraryPresidentofStateGridElectricPowerResearchInstitute(NARIGroupCorporation),andprofessorintheElectricalEngineeringDepartment,ShandongUniversity.
Hisresearchinterestispowersystemautomation.
766ZijunBIN,YushengXUE123
Inordertoanalyzetheoscillationcharacteristicsofcomplexpowersystemsmoreaccuratelyandsuppressthelowfrequencyoscillationmoreeffectively,thispaperimprovesthetrajectorysectioneigenvaluemethod.
Firstly,thetimeresponseofasystemisobtainedbynumericalsimulationinagivenfaultscenario.
Secondly,thealge-braicvariablesaresubstitutedtothedifferentialequationsalongthetrajectory.
Thus,theoriginaltime-varyingdif-ferential-algebraicequationsareapproximatedbyasetoflinearordinarydifferentialequations,whichcanbeupdatedalongthetrajectory.
Onthisbasis,thispaperproposesamethodtoextractinstantaneousfeaturesoftheoscillationfromthemicroperspective.
Thenon-equilibriumpointswithstrongnonlinearityorcriticaleigenmodesareidenti-edbytheproposedmethod.
ThesimulationtestresultsoftheIEEE3-machine9-bussystemandtheNewEnglandsystemillustratethevalidityoftheproposedmethod.
KeywordsLowfrequencyoscillation,Nonlinearfactor,Time-varyingfactor,Trajectorysectioneigenvalue,Instantaneousfeature1IntroductionInrecentyears,thelowfrequencyoscillation(LFO)hasbecomeincreasinglyintricate,withtheenlargementofpowergrids[1],theintegrationofrenewableenergyandthedevelopmentofhighvoltagedirect-currenttransmis-sion[2,3].
Theuniversalityandaccuracyofpreviousanalysisapproachesforsmalldisturbancestabilitycanhardlysatisfytherequirementsofmodernpowersystems.
Inuencedbytheessentialnonlinearfactorsandtime-varyingfactorsduetofaultscenarios,protectionrelaying,andcontrolmeasures,theresultsobtainedbytraditionalmethodsmaybesignicantlydifferentwiththedynamicbehaviorsofapowersystem[4].
Hence,itisurgenttoputforwardanLFOanalysismethodthatcandescribethedynamicbehaviorsofapowersystemmoreaccuratelywithacompleteconsiderationofthenonlineartime-varyingfactors.
Generally,LFOscanbedividedintothreeformsbasedonthecauses:steadyincreaseingeneratorrotorangleduetolackofsynchronizingtorque;`rotoroscillationwithincreasingamplitudeduetolackofsufcientdampingtorque[5];sustainedoscillationduetoperiodicdistur-banceresultingfromfailuresormalfunctioningequipment[6].
Theeigen-analysismethodismostwidelyusedinpractice.
Itdescribesthedynamicsystembehaviorsneartheequilibriumpointbyalineartime-invariantsystem.
However,thenonlinearityofapowersystemmayleadtobifurcationandblow-upofsolutions[7,8].
Reference[9]addressesthenonlinearsingularityphenomenonbyHopfCrossCheckdate:21May2019Received:3December2018/Accepted:21May2019/Publishedonline:10July2019TheAuthor(s)2019&ZijunBINbinzijun@gmail.
comYushengXUExueyusheng@sgepri.
sgcc.
com.
cn1SchoolofElectricalEngineering,ShandongUniversity,Jinan250061,China2StateGridElectricPowerResearchInstitute,Nanjing211000,China123J.
Mod.
PowerSyst.
CleanEnergy(2019)7(4):753–766https://doi.
org/10.
1007/s40565-019-0556-zbifurcationtheory,whichdemonstratesthatthedynamicbehaviorsofasimplepowersystemcouldbedifferentfromtheresultsofeigen-analysis.
However,limitedbytheheavycomputationinlarge-scalesystems,thismethodisstillunderinvestigationaswellinmethodologicalaspectsasinconcreteapplications.
Inordertoconsidertheeffectsofnonlinearfactors,thenormalformtheory[10]andthemodalseriesmethod[11]areproposed.
Moredetailedinformationofeigenmodesisextractedbysolvingthehigh-orderapproximateequations.
Onemajordrawbackoftheseapproachesisthatthesolutionsareobtainedattheequilibriumpoint,neglectingtime-varyingfactors.
Forthesakeofconsideringtheeffectsofrandomfactors,proba-bilitymodelsareusedtoimprovetheuniversalityoftra-ditionalmethods[12].
Allofthesemethodsreviewedthusfar,however,sufferfromthelimitationofdisturbancescenarios.
Asaresult,somecriticalfactorsofapowersystemsuchasthenon-differentiablecomponentsandcontrolmeasuresareignored[13].
Toentirelyanalyzetheinuenceofnonlineartime-varyingfactorsonpowersystemoscillation,itisquitenecessarytoextractinformationfromtrajectories.
Somesignalanalysistechniquesareusedtoidentifytheoscilla-tionmodals.
Commonapproachesliketime-frequencytransformationstakethedisturbedtrajectoryasresearchobject.
Thefrequencyfeaturesareidentiedbydecouplingfrequencycomponents,andthedampingfeaturesareextractedbydifferentiatingmodeamplitudeswithrespecttotime.
Asaclassicalmethod,thePronyanalysisreplacesthetrajectorybyasetofexponentialcomponents[14].
Thismethodcandescribethedynamicbehaviorsinanintervalapproximately,thoughtheinuencesoftime-varyingfac-torsareaveraged.
Basedonwide-areameasurementsignalsandtheproperorthogonaldecomposition(POD)method,[15]usesdynamicmodedecoupling(DMD)methodtoachieveoscillationmodesdecouplingandfeaturesextracting.
Nevertheless,thesemethodscanhardlydescribetheinstantaneouscharacteristics,whicharetime-varying.
Theempiricalmodedecoupling(EMD)methodextractsinstantaneousfeaturesbydecomposingtheorigi-nalsignalintolocallysymmetriccomponentsonthebasisofenvelopecharacteristicsandtheHilberttransform[16].
However,theprecisionofEMDmethodisstronglyaffec-tedbythefrequenciesofadjacentcomponents.
Toextractinstantaneousfeaturesmoreaccurately,windowPronymethod[17],andwindowfastFouriertransformation(ridges)method[18]useslidewindowstodividetheentiretimetoasetofoverlappingperiodsalongthetrajectory.
Thefeaturesequenceiscomposedofthemodalsofallwindows.
Despiteofthat,theaccuracyofthemethodsislimitedbythewidthofthewindow.
Moreover,thetrun-catederrorcausedbytheedgesonbothsidesofthesignalisinevitable.
Toimprovetheadaptiveabilityofthetime-frequencytransformationapproaches,wavelet(ridge)methodisintroducedtoassistanalyzingLFOs[19].
Researchesshowthatthemethodcanidentifythemodalsofanactualtrajectorywithnoiseseffectively[20],althoughthechoiceofwaveletbasisreliesonempiricalparameters.
Reference[21]approximatestheobservedprocessasalinearsystemformoreinformationofmodels.
Thestabilitymarginsareobtainedasposteriorprobabilitiesthatthepolesoftheestimatedsystemareunstable.
Aconsiderablenumberofresearcheshavebeendonetoachievetheextractionofinstantaneousfeaturesbysignalanalysistechniquesduringthelastdecades.
Tobeobjec-tive,theseapproachesareinstrumentalinidentifyingmodalsonline.
However,allthesementionedmethodssufferfromaseriouslimitation.
Theessenceofsignalanalysistechniquesisidenticationoftheaveragechar-acteristicsinatimewindow.
Thetimescaleoftheoscil-lationanalysisislimitedbytheresolutionofthemethods.
Ontheotherhand,themajorgoalofmodalidenticationofLFOistosuppresstheoscillation.
Tolocatecriticalgen-eratorsorparameters,thesignalanalysistechniqueshavetobeuniedwiththemodelsattheequilibriumpoint,whichcanhardlyreecttherealdynamicbehaviorsofanonlineartime-varyingsystem.
Attemptstoresolvethisdilemmahaveresultedintheinventionoftrajectorysectioneigenvalue(TSE)theory,whichextendstheconceptofeigenvaluefromtheequi-libriumpointtoothernon-equilibriumpointsofthetra-jectory.
Thepioneerworkcanbetracedto[22].
Reference[23]attemptstouseTSEmethodtoanalyzetransientsta-bility.
Themethodisfurtherdevelopedtoestimatetheenergyofasystembeforethevisualfarendpointorthevisualdynamicsaddlepoint[24].
Reference[25]concludesthattheglobalstabilityoflineartime-varyingsystemscannotbedeterminedbyTSEs.
Themajorreasonisthattheglobaldynamicsoftime-varyingsystemsareaffectedbythekineticenergyatnon-equilibriumpoints,whichcouldnotbeconsideredbyTSEmethod.
However,[26]hasprovedthattheeffectsofthekineticenergycanbeignoredwhenanalyzingtheinstantaneouscharacteristicsinoneintegrationstep.
Meanwhile,themathematicalandphysicalfoundationsofTSEmethodhavebeengiveninthisliterature.
Thecontributionsofthispaperarethreefold.
Firstly,itimprovesthemathematicsofTSEmethodtogiveabetterexplanationofthephysicalmeaningoftheeigenvaluesatnon-equilibriumpoints.
Basedontheimprovedtheory,thispaperproposesamethodtoextracttheinstantaneousfea-turesofLFOs.
Secondly,thepaperproposesthecriteriontoidentifythenon-equilibriumpointswithstrongnonlinear-ity,andillustratesthemechanismofnonlinearoscillationsinpowersystem.
Thirdly,itproposesthecriteriontoidentifythedangerousnon-equilibriumpointsandthe754ZijunBIN,YushengXUE123criticaleigenmodes.
Comparedwithpreviousmethods,thecriticalgeneratorsandparametersidentiedatabovenon-equilibriumpointscanachievebetterinhibitioneffects.
2Mathematicsoftrajectorysectioneigenvalues2.
1Piecewise-linearizedsystemmodelsandsolutionintimedomainThebehaviorsofadynamicsystem,suchasapowersystem,maybedescribedbyasetofnrst-ordernonlineardifferential-algebraicequations(DAEs)asthefollowingform(thederivativesofthestatevariablesarenotexplicitfunctionsoftime)[5]:_XfX;Y0gX;Y&1ThecolumnvectorXisreferredasthestatevector,anditsentriesxiasthestatevariables,suchasrotoranglesandangularvelocitiesoftherotors.
ThecolumnvectorYisreferredasthealgebraicvector,anditsentriesyiasthealgebraicvariables,suchasnodevoltagesandnodecurrentinjections.
ThesolutionsofXandYinthetimedomaincanbeobtainedbynumericalsimulationingivenfaultscenarios.
BysubstitutingYintothedifferentialequations_XfX;Yandlinearizingthenonlinearcomponentsofthefunctionsalongthetrajectory,theDAEsarereplacedbyasetoflinearordinarydifferentialequations(ODEs).
Foranillustrativepurpose,thelinearODEattkistakenasanexampletointroduceTSEmethod.
D_XkDtAkDXkDtBk2whereAkofoXXtkistheJacobimatrixofthenonlinearfunctionf;BkfXtk;Ytkistheinitialvaluesoftherightsideofthedifferentialequationattk.
SupposingthatUkistherightmodalmatrixofAk.
NewstatevectorisdenedbyZkDtU1kDXkDt.
Bothsidesof(2)areleft-multipliedbyU1k:_ZkDtU1kAkUkZkDtU1kBk3Solve(3)withtheinitialvaluesoftheelementsofZkDtrespecttoDt(Zk00),whichmeanstheincrementaltimeintk;tk1.
ZkDtCkEkDtK1kU1kBk4whereEkDtekk;1Dt;ekk;2Dtekk;nDtT;KkU1kAkUkdiag(kk;1;kk;2;kk;nistheeigenvaluesofthestatematrixAkattk.
TwoprocedureparametermatricesDkandCkaredenedasfollows:Dkdk;1dk;2.
.
.
dk;n26664377751kk;1vk;11f1vk;12f2vk;1nfn1kk;2vk;21f1vk;22f2vk;2nfn.
.
.
1kk;nvk;n1f1vk;n2f2vk;nnfn2666666666643777777777755Ckdk;1000dk;20.
.
.
.
.
.
.
.
.
00dk;n26664377756wheredk,iistheithelementofDk;vk;ijistheelementintheithrowandthejthcolumnofU1k;fiistheithelementofBk.
Bothsidesof(4)areleft-multipliedbyUk:DXkDtUkCkEkDtA1kBk7Obviously,(7)describesthedynamicbehaviorsofthechangesofstatevariablesintk;tk1.
ThevaluesofUkCkindicatethedegreesofeigenmodesexcitation[27].
2.
2PhysicalmeaningoftrajectorysectioneigenvaluesThechangesofthestatevariablesrespecttoDthavebeenobtainedby(7)inthetimedomain.
ConsideringthattheinitialvaluesofZk(Dt)arezero,wehaveZk0CkEk0K1kU1kBk0.
Equation(7)canberearrangedto:DXkDtUkCkEkDtUkCkEk08Assumingtheexistenceofanewsystem,ttkDt,thestatevectorofthissystemXk,new(t)satises:Xk;newtUkCkEkttk9Therefore,thechangesofthenewstatevectorintk;tk1canbeexpressedby:DXk;newDtXk;newtXk;newtkUkCkEkttkUkCkEk0DXkDt10Accordingto(10),thedynamicbehaviorofthenewsystemisthesamewiththeoriginalsystem,ignoringtheunbalancedpowerintk;tk1.
Thestateequationofthenewsystemcanbederivedfrom(9).
Amethodtoextractinstantaneousfeaturesoflowfrequencyoscillationbasedontrajectory…755123_Xk;newtUkCkKkEkttkUkCkKkUkCk1Xk;newt11CkandKkarebothdiagonalmatrices,andtheexpressionin(11)mustsatisfyUkCkKkUkCk1UkKkU1kAk.
Equation(11)canberearrangedas:_Xk;newtAkXk;newt12Thus,theoriginalsystemwithoutregardtounbalancedpowerisapproximatedbyalinearsystemintk;tk1.
AlthoughXk,new(t)maybedifferentfromXk(t),thechangesoftheirstatevectorsarethesameintk;tk1.
Thesectioneigenvalueskk;1;kk;2;kk;ndescribethedynamicbehavioroftheimaginarysystemintk;tk1.
Besides,theparametermatricesUkandCkareupdatedalongthetrajectoryaccordingtotheresultsofnumericalsimulation.
Theeffectoftheunbalancedpowerisreectedinfollowingtimesections.
Furthermore,theaccumulateerrorisavoidedbyupdatingtheparametersof(7)and(12)alongthetrajectory.
Consequently,theeffectsofnonlinearfactorsandtime-varyingfactorsareconsideredbythesectionstatematrixchangingwithtime.
3ExtractionmethodofinstantaneousfeaturesofLFOToextracttheinstantaneousfeaturesofLFO,theeigenvaluesofthesectionstatematrixarecalculated.
Ingeneral,ann-ordermatrixhasneigenvalues,whichmayconsistofcouplesofcomplexeigenmodesandrealeigenmodes.
Acoupleofcomplexeigenmodesrepresentasinusoidalcomponent,whilearealeigenmoderepresentsanexponentialcomponent.
Inthispaperfk;i;insisusedtorepresenttheimaginarypartoftheitheigenvalueattk,dividedbyaconstant2p.
rk;i;insisusedtorepresenttherealpartoftheitheigenvalueattk.
Asforacomplexroot,fk;i;insrepresentstheinstantaneousfrequencycharacteristicoftheitheigenmodeattk.
rk;i;insdescribesthetrendoftheamplitudeoftheitheigenvalueattk.
Asforarealroot,fk;i;insiszero.
rk;i;insrepresentstherateofatrendchangewithinoneintegrationstep.
3.
1IdenticationofpointswithstrongnonlinearityInanoscillation,acoupleofcomplexeigenmodesmayreversiblytransformintotworealeigenmodesoccasion-ally.
Aone-machineinnitebus(OMIB)systemisusedtoilluminatethemechanismofthevariationofTSEs.
ThestructureoftheOMIBsystemisshowninFig.
1.
Vrepresentsthevoltageoftheinnitebus.
Peistheinputpower.
ThesummationofreactanceisdenotedasXR.
E0anddarereferredasthemoduleandangleofthetransientelectromotanceafterthetransientreactanceofthegener-ator,respectively.
Theclassicalmodelisusedtorepresentthegenerator.
ThedynamicequationsofthegeneratorcanbefoundinAppendixA(A1).
Whenadisturbanceoccurs,theTSEscanbeobtainedalongthetrajectory:kk;1;2D2MD24MKkp2M13whereDisthedampingtorquecoefcientofthegenerator;KkE0Vcosdk=XRrepresentstheslopeoftheelectro-magneticpower-rotoranglecurveattk,geometrically;dkisthevalueoftherotorangleattkwithavaluerangeof0;p.
Ascanbeexpected,ifdk20;arccosD2XR=4ME0V,kk;1;2wouldbeacoupleofcomplexroots.
Withtheincreaseofdk,theimaginarypartsofkk;1;2decrease.
Ifdk2arccosD2XR=4ME0V;p,kk;1;2wouldbetwodifferentrealroots.
Withtheincreaseofdk,oneofthemdecreases,andtheotherincreases.
Therefore,thenon-equilibriumpointswithstrongnonlinearitycanbeidenti-edbrieybelow:aggregatingthecomplexeigenmodesateachtimesection;`comparingthenumbersofthecomplexeigenmodesatnon-equilibriumpointstotheequilibriumpoint;thosenon-equilibriumpointsaresigned,thenumberofwhichisinconsistentwiththeequilibriumpoint.
Theconceptofthenon-equilibriumpointswithstrongnonlinearitycanbeusedtoexplainsomecomplexphe-nomena.
Forinstance,thedistortionofarotorangletra-jectoryisinducednearfarendpoints(FEPs)asshowninFig.
2a,c.
TheangularaccelerationandvelocityapproachtozerowhenFEPsareclosetothedynamicsaddlepoint(DSP).
Figure2bdepictstheevolutionoftheimaginarypartsoftheTSEs,theeigenmodesofwhichtransfertotheexponentialcomponentsfromtheoscillationcomponents.
3.
2IdenticationofcriticalpointsAtwo-machinesystemispresentedinFig.
3tointro-ducethecriticalnon-equilibriumpointsingeneralsystem.
TheTSEsofthistwo-machinesystemafterbeingdis-turbedcanbeoftwoforms:acoupleofcomplexroots,arealroot(canbezero),andazeroroot;`threerealrootsGV0°EPeXΣδFig.
1One-machineinnitebussystem756ZijunBIN,YushengXUE123(canbezero)andazeroroot.
Equation(12)suggeststhatthepositivesignsoftherealpartsofTSEsrepresenttheinjectionofdangerousenergy.
Thus,thecriticalnon-equilibriumpointsareidentiedbythesignsoftherealpartsoftheeigenvaluesalongthetrajectory.
Forthesakeofillustratingtherelationsbetweeninstantaneousfeaturesandglobaldynamics,Fig.
4ana-lyzesatypicalconvergentoscillationandatypicaldiver-gentoscillationinthetwo-machinesystem.
AsshowninFig.
4a,inaconvergentoscillation,therealpartsofmode3couldbepositiveperiodically.
AsshowninFig.
4b,inadivergentoscillation,therealpartsofmode1andmode2arenotalwayspositive.
Intheneighborhoodoftheequilibriumpoint,theTSEsareconsistentwiththeeigenvaluesattheequilibriumpoint.
However,powersystemisanessentialnonlineardynamicsystem.
Oncetheoperatingpointofthesystemrunsawayfromtheequilibriumpoint,thedynamicbehaviorsmaybedifferentwiththeeigenvaluesattheequilibriumpoint.
Inalinearsystem,theacceleratedpowergraduallyincreaseswiththedecreasingrelativeangularvelocityfromDCPtoFEP.
However,affectedbythenonlinearityofthePe–dcurve,theacceleratedpowerofapowersystemislowerthanthelinearsystembeforetheFEP.
Asaresult,thedampingcharacteristicsnearFEPswouldbeworsethantheneighborhoodoftheequilibriumpoint.
TheclosertheFEPistotheDSP,thelowerthestabilitymarginofthesystemsuffers,andthetrajectoriesoftherealpartsofTSEschangecorrespondingly.
Figure4a,bdepictstheevolutionofrk;i;insinconvergentanddivergentoscillation,-10121.
01.
52.
02.
5(a)Trajectoryofrotorangle1.
01.
52.
02.
500.
51.
5(b)Trajectoryoffk,i,ins000.
51.
01.
52.
02.
5-0.
50.
51.
01.
52.
0(c)Pe-δcurveThefaultoccursThefaultiscleanedDistortionofthetrajectoryt(s)3δ(rad)ImaginarypartsoftheTSEsarezero1.
02.
0t(s)fk,i,ins(Hz)-1.
0δ(rad)-0.
5Pe(p.
u.
)FEP2FEP1PeDSPPmFig.
2DistortionphenomenonofrotoranglenearFEPsG1G2LoadFig.
3Two-machinesystem123456-2-1012(a)Trajectoriesofσk,i,insinconvergentrotorangleoscillation123450-3-1132-2(b)Trajectoriesofσk,i,insindivergentrotorangleoscillation0Mode3Mode2;Mode1;σk,i,inst(s)60t(s)σk,i,insFig.
4RealpartsofTSEsinatwo-machinesystemAmethodtoextractinstantaneousfeaturesoflowfrequencyoscillationbasedontrajectory…757123respectively.
Thevaluesofrk;i;inshaveobviouslypositivecorrelationwiththedampingcharacteristics.
Therefore,thetrajectoriesoftherealpartsofTSEsindicatetheinstantaneousdampingcharacteristics.
Impor-tanceshouldbeattachedtothecriticalnon-equilibriumpointswithpositiverealpartsofTSEs.
Tosuppresspowersystemoscillation,itisnecessarytooptimizetheparametersofcriticalgeneratorsorcon-trollers,whichcanbelocatedbytheeigenmodeswithpositiverealparts.
Theparticipationfactorandtheelec-tromechanicalmodecorrelationratiocanalsobeextendedfromtheequilibriumpointtonon-equilibriumpoints.
Section4demonstratestheeffectivenessoftheinstanta-neousfeaturesinlocatingcriticalgeneratorandguidingtheoptimization.
Inaddition,therelativepositionsofeigenvaluesbetweenadjacenttimesectionsarerandom,whichlimitstheapplicationofTSEtheoryinidentifyingcriticaleigenmodes.
Foranillustrativepurpose,assumingthattheeigenvaluesattaandtbareka;1;ka;2;ka;nandkb;1;kb;2;kb;n,respectively.
ka;1mayrelatetokb;2orevenkb;n.
Theeigen-polynomialisacontinuousfunctionoftheparametersofstatematrix,andthezeropointsoftheeigen-polynomialarecontinuousfunctionsoftheparame-tersofeigen-polynomial.
Consequently,theeigenvaluesofamatrixarecontinuousfunctionsoftheparametersofthematrix.
TheTSEsbetweenadjacenttimesectionscanmatchwiththesimilarityoftheirvalues.
3.
3ProcessofinstantaneousfeaturesextractionFigure5showstheproceduretoextractinstantaneousfeatures.
Themethodincludessixstepsasfollows.
Step1:thetime-domainresponsesofstatevariablesareobtainedbynumericalsimulationorphasemeasurementunits.
Step2:thealgebraicvariablesaresubstitutedtothedifferentialequationsalongthetrajectoryandthesectionstatematricesareobtainedbylinearizingthenonlinearcomponentsofthedifferentialequations.
Inthispaper,thesizeofanalysisstepisequaltotheintegrationstep.
Step3:theTSEsarecalculatedfromthestatematrixandtheinstantaneousfeaturesareextractedalongthetrajectory.
Step4:theeigenvaluesbetweenadjacenttimesectionsarematched.
Step5:thepointswithstrongnonlinearityareidentiedbycorrespondingcriterion.
RnandInrepresenttheeldsofrealnumberandimaginarynumber,respectively.
Step6:criticalpointsareidentiedbycorrespondingcriterion.
4Casestudy4.
1IEEE3-machine9-bussystemInthissection,a3-machine9-bussystemisusedtoillustratethelimitationofthetraditionaleigen-analysismethod.
First-orderTaylorseriesexpansionSimplesystemmodelingIsstepsizelargeLinearfitIsanalysisprecisionhighFull-OrdermodelingSectionstatematricescalculationEigenmodesmatchingIsthereeigenmodetransformbetweenRnandInPointwithstrongnonlinearityPointwithweaknonlinearityIsthereanyRealpartofTSEspositiveCriticalPointWell-BehavedPointYNStartEndNYNYNYNumericalsimulatonStepsizedeterminationStep1Step2Step5Step6Step3Step4Fig.
5Flowdiagramofinstantaneousfeaturesextraction758ZijunBIN,YushengXUE123ForthestructureshowninFig.
6,thegeneratorsareallequippedwithgovernors.
Theclassicalmodelisusedtorepresentthegenerator,thetransientelectromotanceE0afterX0disconstant.
ThedynamicequationsofgeneratorandgovernorarelistedinAppendixA.
Theconstantimpedancemodelisusedtorepresenttheload.
Thelinedata,thegeneratordata,andthegovernordataarelistedinAppendixB.
TheeigenvaluesattheequilibriumpointarelistedinTable1andtherealpartsofalleigenvaluesarenegative.
Intheviewofthetraditionaleigen-analysismethod,thetrajectoriesofstatevariablesshouldbeconvergentoscil-lationwhenthesystemisdisturbed.
Ontheotherside,themostdangerouseigenmodeidentiedbythetraditionaleigen-analysismethodshouldbethe3rdand4theigenvalueswiththeminimumrealparts.
Moreover,thecorrespondingclusterofgeneratorsis{G2,G3}vs.
{G1}.
Theoscillationcharacteristicsofthissystemareana-lyzedindifferentfaultscenarios.
Thenumericalintegrationisusedtoassessthevalidityoftheproposedmethod.
4.
1.
1Scenario1:weaknonlineartime-varyingscenarioThedisturbanceisathree-phasetransientfaultinbus6,andthefaultdurationis0.
09s.
Thetrajectoriesoftherotoranglesareconvergentoscillationswithweakdamping,asshowninFig.
7.
Todemonstratethattheinstantaneousfeaturesoftheoscillationareconsistentwiththetraditionaleigen-analysismethodinweaknonlineartime-varyingscenarios,theinstantaneousfeaturesareobtainedasfollows.
AsshowninFig.
8a,afterthefaultdisappears(laterthan0.
09s),inmostofthetime,therearefourcouplesofcomplexeigenmodes,whichrepresentfouroscillationcomponentswithdifferentfrequency.
Accordingtothecriterionforpointswithstrongnonlinearity,therearenononlinearpointsinthetrajectoriesofk1;2,k3;4,andk5;6.
Instead,thenonlinearpointsappearinthetrajectoriesofk7;8,whicharesignedbypurpleblocksinFig.
8c.
Thestrongnonlineareigenmodesandthedangerouseigen-modesmaybedifferent.
AsshowninFig.
8b,thesignsoftherealpartsofk7;8arealwaysnegative,andthesignsoftherealpartsofk3;4arepositiveinsometimesections,whicharesignedbyredblocksinFig.
8c.
Ascanbeexpected,thecriticalpointsarerelatedtothepointswithstrongnonlinearity.
G2G3G1213456789Fig.
6IEEE3-machine9-bussystemTable1EigenvaluesofIEEE3-machine9-bussystematequilibriumpointNumberRealpartImaginarypartClusterofgenerators1-0.
104214.
4300{G1,G2}vs.
{G3}2-0.
104214.
4300{G1,G2}vs.
{G3}3-0.
02568.
7149{G2,G3}vs.
{G1}4-0.
02568.
7149{G2,G3}vs.
{G1}5-0.
24820.
6203{G2}vs.
{G1,G3}6-0.
24820.
6203{G2}vs.
{G1,G3}7-1.
19600.
0763{G1,G2}vs.
{G3}8-1.
19600.
0763{G1,G2}vs.
{G3}9-4.
04470.
0000–10-19.
10850.
0000–11-19.
37790.
0000–12-19.
39790.
0000–13-5.
79040.
0000–14-0.
75610.
0000–15-0.
23430.
0000–160.
00000.
0000–17-0.
02030.
0000–18-0.
01490.
0000–-101-10100.
51.
01.
52.
02.
53.
03.
54.
04.
5t(s)-0.
500.
5δ1(rad)δ2(rad)δ3(rad)Fig.
7Trajectoriesofrotoranglesofsysteminscenario1Amethodtoextractinstantaneousfeaturesoflowfrequencyoscillationbasedontrajectory…7591234.
1.
2Scenario2:strongnonlineartime-varyingscenarioThedisturbanceisathree-phasetransientfaultinbus6,andthefaultdurationis0.
15s.
Thetrajectoriesoftherotoranglesaredivergentoscillations,asshowninFig.
9.
Theinconsistencybetweenthetrajectoriesofrotoranglesandtheeigenvaluesattheequilibriumpointrevealsthelimitationoftraditionaleigen-analysismethodinstrongnonlineartime-varyingscenarios.
Toillustratethemecha-nismoftheoscillationfromthemicropointofview,theinstantaneousfeaturesareobtainedasfollows.
AsshowninFig.
10a,b,afterthefaultdisappears(laterthan0.
15s),pointswithstrongnonlinearityappearinthetrajectoriesofk3;4,k5;6andk7;8.
Thecriticaltimesectionsappearinthetrajectoriesofk3;4andk5;6.
ThepointswithstrongnonlinearityandcriticaleigenmodesaresignedinFig.
10cbypurpleandredblocks,respectively.
Obviously,themostdangerouseigenmodeisk5;6withthemaximumpositivevaluesintheoscillation,asshowninFig.
10b.
Theclusterofthegeneratorsisthesameastheactualinstabilitymode:{G2},{G1,G3}.
Therefore,thecharacteristicsofinstantaneousfeaturescanbesummarizedasfollows:inweaknonlineartime-varyingscenarios,theoperatingpointofthesystemrunsneartheequilibriumpoint,andtheinstantaneousfeaturesaresimilartothetraditionaleigen-analysismethod;`instrongnonlineartime-varyingscenario,theoperatingpointofthesystemrunsfarawayfromtheequilibriumpointperiodically,andtheinstantaneousfeaturesmaybetotallydifferentfromthetraditionaleigen-analysismethod.
4.
2IEEE10-machine39-busNewEnglandsystemIntheviewofthetraditionaleigen-analysismethod,theeigenmodeswithrealpartsgreaterthanzeroaredangerous.
Theeigenmodeswithrealpartsapproximatetozeroaredenedasweakdampingeigenmodes.
ForthesakeofsuppressingLFO,theseeigenmodesshouldbecontrolled.
However,Section4.
1hasrevealedthatthetraditionaleigen-analysismethodcannotdescribethedynamicbehaviorsaccurately.
Moreover,theactuallydangerousorweakdampingeigenmodesmaybeignored.
TheNewEnglandsystemisusedtoilluminatethesuperiorityoftheproposedmethodinlocatingcriticaleigenmodes.
Thestructureofthesystem,thelinedataandthegen-eratordatacanbefoundin[28].
Thesetengeneratorsareallequippedwithgovernors.
Theclassicalmodelisusedtorepresentthegenerator,thetransientelectromotanceE0afterX0dareconstant.
Thedynamicequationsofgenerators-1.
0-0.
500.
5-4.
5-4.
0-6.
0-19.
5-19.
0-18.
500.
51.
52.
53.
54.
5(c)Locationsofpointswithstrongnonlinearityandcriticalpointsinscenario100.
51.
01.
52.
53.
54.
50.
51.
52.
5(a)Trajectoriesoffk,i,insinscenario11.
02.
02.
03.
04.
0t(s)λ7,8λ5,6λ1,2λ3,4fk,i,ins(Hz)λ5,6λ3,4λ1,2λ7,800.
51.
01.
52.
53.
54.
5(b)Trajectoriesofσk,i,insinscenario12.
03.
04.
0t(s)σk,i,insNonlineartimesection;Negativedampingtimesection1.
02.
03.
04.
0t(s)λ9λ14λ13λ10λ11λ12Fig.
8TrajectoriesofTSEsinscenario1-202δ1(rad)-20200.
51.
52.
53.
54.
5t(s)-202δ2(rad)δ3(rad)1.
02.
03.
04.
0Fig.
9Trajectoriesofrotoranglesofsysteminscenario2760ZijunBIN,YushengXUE123andgovernorsareshowninAppendixA.
Theconstantimpedancemodelisusedtorepresenttheload.
Thegov-ernordataarelistedinAppendixC.
Asacomparison,theeigenvaluesattheequilibriumpointarelistedinAppendixD.
Itisobviousthattherealpartsofalleigenvaluesarenegative.
Thedisturbanceisathree-phasetransientfaultinbus15andthefaultdurationis0.
39s.
ThetrajectoriesoftherotoranglesareshowninFig.
11.
Beforethe3rdsecond,theamplitudesoftherotoranglesaredecreasingandthedominantoscillationmodeis{G39}tothesetoftheothergenerators.
Afterthe3rdsecond,theamplitudesoftherotoranglesareincreasingandnallythesystemlosesstability.
Thecriticalmodeis{G34}tothesetoftheothergenerators.
4.
2.
1RealpartsoftrajectorysectioneigenvaluesInstantaneousfeaturesareextractedandtherealpartsoftheTSEsareshowninFig.
12.
Inordertohighlightthecriticaleigenmodes,theothereigenmodesarehidden,thesignsoftherealpartsofwhicharenegativeinalltime.
Inintervals1and2,thecriticalpointsmainlyappearineigenmodek29;30,thesamewiththeeigenvaluesattheequilibriumpoint.
Inintervals3to6,thecriticalpointsmainlyappearinthetrajectoriesofacoupleofneweigenmodes.
Thus,thecriticaleigenmodesthatmainlyaffectthedynamicbehaviorsoftheoscillationshouldbethenewcouple.
4.
2.
2LocationofcriticalgeneratorInordertolocatethecriticalgenerator,itisnecessarytocalculatetheparticipationfactorsofcriticaleigenmodesatcriticaltimesections.
Thegeneratorwithhighestpartici-pationfactorinoneintervalisinvariant.
Foranillustrativepurpose,theresultsofeigenmodesanalysesintypicaltimesectionsareshowninTable2.
Inintervals1and2,k29andk30arethemostdominanteigenmodeswiththehighestparticipationfactorsandthecriticalgeneratorisG39.
Inintervals3to6,theneweigenmodesarethemostdominanteigenmodeswiththehighestparticipationfactor,andthecriticalgeneratorisG34.
Thedescriptionsoftheinstantaneousfeaturesarethe00.
51.
52.
53.
54.
5(c)Locationsofpointswithstrongnonlinearityandcriticalpointsinscenario2t(s)1.
02.
03.
04.
0Nonlineartimesection;Negativedampingtimesection00.
51.
52.
53.
54.
5-20-15-10-5051015(b)Trajectoryofσk,i,insinscenario2σk,i,ins1.
02.
03.
04.
0λ7,8λ3,4λ5,6λ1,200.
51.
52.
53.
54.
50.
51.
52.
5(a)Trajectoryoffk,i,insinscenario21.
02.
03.
04.
0fk,i,ins(Hz)1.
02.
0λ1,2λ3,4λ5,6λ7,8t(s)λ9λ13λ10λ11,12λ14t(s)Fig.
10TrajectoriesofTSEsinscenario2-3-2-10123123456780δ1(rad)t(s)G30;G32;G33;G34G35;G36;G37;G38;G39G31;Fig.
11TrajectoriesofrotoranglesofIEEE10-machine39-bussystemAmethodtoextractinstantaneousfeaturesoflowfrequencyoscillationbasedontrajectory…761123sameastheactualtrajectoriesoftherotorangles,asshowninFig.
11.
Consideringthattheelectromechanicalmodecorrelationratiosoftheseeigenmodesaremuchlargerthan1,thestatevariablesparticipatingintheeigenmodesaremainlyrotoranglesandangularvelocities.
Tosuppresstheoscillation,thedampingtorquecoef-cientofG34isup-regulatedby10%,andthenumericalsimulationshowsthatthetrajectoriesoftherotoranglesturntobeconvergent,asshowninthelefthalfofFig.
13.
Bycomparison,thedampingtorquecoefcientofG39isup-regulatedby50%andtrajectoriesarestilldivergentaftertheoptimization,asshownintherighthalfofFig.
13.
5ConclusionBasedonTSEs,thispaperproposesamethodtoextractinstantaneousoscillationfeaturesfromthemicroperspec-tive.
TobetterillustratethemechanismofpowersystemoscillationandeffectivelysuppresstheLFO,thecriteriafornon-equilibriumpointswithstrongnonlinearityandcriticaleigenmodesareproposed.
Atnon-equilibriumpointswithstrongnonlinearity,thedynamicbehaviorsmaybetotallydifferentfromtheeigenvaluesattheequilibriumpoint.
Thecriticalpointsrarelyappearinweaknonlineartime-varyingscenarios.
Eventhoughtheoscillationisconvergent,therealpartsofTSEsmaybepositiveatsomenon-equilibriumpointswithstrongnonlinearity.
Theinstabilityofoscilla-tionsmaybecausedbytheaccumulationeffectsofthecriticalnon-equilibriumpoints,whichmassivelyappearinstrongnonlineartime-varyingscenarios.
Moreover,simu-lationresultsclearlyindicatetheenhancedaccuracyofcriticalgeneratorslocation.
6DiscussionOverthelast3decades,therotoranglestabilityhasbeencharacterizedindividuallyintotwosubcategories:small-disturbancestabilityandtransientstability.
Asaresult,itisimpossibletoanalyzetherelationshipbetweenoscillationstabilityandsynchronousstabilitywithinthecurrentframework.
Thedisturbancesinsomecasestudiesofthispaperbelongtothetraditionaltransientstabilitycategory,forthreereasons:1)Itisdifculttodistinguishsmalldisturbanceandlargedisturbance.
Inanegativedampingoscillationdenedbythetraditionalmethod,acomplexdynamicprocessisinevitablebeforesynchronousinstability.
Thus,thisstudybeginswiththeaimofstudyingthemechanismandcharacteristicsoftheevolutionofLFO,nomatterthedisturbanceislargeorsmall.
AlthoughTSEmethodcannotbeusedtoanalyzetheglobalstability-4-2024607.
0Interval1Interval5σk,i,ins8t(s)7.
56.
06.
55.
05.
54.
04.
53.
03.
52.
02.
51.
01.
50.
5λ40(neweigenmode);λ41(neweigenmode)λ29(originaleigenmode);λ30(originaleigenmode);Fig.
12TrajecotiesofrealpartsofcriticaleigenmodesinIEEE10-machine39-bussystemTable2Analysesofeigenmodesattypicaltimesectionsfromintervals1to6IntervalnumberTypicaltimesection(s)HighestparticipatinggeneratorParticipationfactorElectromechanicalmodecorrelationratio11.
00G390.
139789.
593422.
20G390.
84089.
367033.
50G340.
50481102.
774045.
20G340.
4824709.
611855.
80G340.
4781717.
336766.
20G340.
4774623.
5736762ZijunBIN,YushengXUE123ofapowersystem,simulationtestresultsindicateastrongcorrelationbetweentheoscillationcharacteris-ticsandtheproposedfeatures.
2)Inordertostudythestabilityofapowersystem,auniedframeworkhasbeenproposedby[29]tounifytheanalysisapproachesoftheoscillationstabilityandthetransientstabilityintermsofenergy.
TheimprovedTSEmethodandtheachievementsofthispapercontributetotheuniedframework.
ThispaperisatentativestudyonunifyingsmalldisturbanceanalysisandlargedisturbanceanalysisthroughTSEmethod.
3)Theeigenvaluescanonlydescribethedynamicbehaviorsintheneighboringregionofonepoint,whetherequilibriumpointornon-equilibriumpointitis.
Toanalyzetheoscillationcharacteristicsinstrongnonlineartime-varyingscenarios,thestatematrixandthevariablesareupdatedalongthetrajectory,accord-ingtothenumericalresults.
Finally,theproposedmethodwouldbeusedinengi-neeringonlywhenthetraditionaleigen-analysiscannotdescribethedynamicbehaviorsaccurately,ordetailedanalysisisrequiredinsomecomplexscenarios.
Thus,comparedwiththetraditionaleigen-analysis,theincreasingcomputationisacceptable.
Thequantityofinformationandcomputationcanbebalancedbydeterminingthestepsizemoreintelligently.
Moreover,maturemethodshavebeenusedtoreducethedimensioncursewhencalculatingeigenvaluesattheequilibriumpoint.
Thetheoremoftheinverseoperatorcanbeusedtodeterminethecirclewheretheeigenvalueslie.
Theradiusofthecirclecanbeesti-matedbyGerschgorintheory.
Manyresultshavebeenmadeinthiseld[30].
Theseachievementscanalsobeusedtoestimatethedistributionoftheeigenvaluesatthenon-equilibriumpoints.
AcknowledgementsThisworkwassupportedbyScienceandTechnologyProgramofStateGridCorporationofChina(TheoreticalBasis,AlgorithmandApplicationofTrajectoryEigenvalueMethod).
OpenAccessThisarticleisdistributedunderthetermsoftheCreativeCommonsAttribution4.
0InternationalLicense(http://creativecommons.
org/licenses/by/4.
0/),whichpermitsunrestricteduse,distribution,andreproductioninanymedium,providedyougiveappropriatecredittotheoriginalauthor(s)andthesource,providealinktotheCreativeCommonslicense,andindicateifchangesweremade.
AppendixAdddtxdxdt1MPmPeDx8>:A1wheredrepresentstheangleoftherotors,xrepresentstheanglevelocityoftherotor;Mrepresentstheinertialofgenerator;Pmrepresentsthemechanicaltorque;andPerepresentstheelectromagnetictorque.
InthedatalistsofAppendixesBandC,thedirect-axistransientreactanceofgeneratorisdenotedbyX0d,thequadratureaxissyn-chronousreactanceisdenotedbyXq,thedampingtorquecoefcientisdenotedbyD.
12345678-3-2-101-3-2-101230δ(rad)t(s)δ(rad)G30;G32;G33;G34G31;G35;G36;G37;G38;G392Fig.
13TrajectoriesofrotoranglesofIEEE10G39NsystemafterparameteradjustmentsofG34andG39,respectivelyAmethodtoextractinstantaneousfeaturesoflowfrequencyoscillationbasedontrajectory…763123dldtrdrdt1TGTplnTGrd[nRDdldt1TdRlnd(Pm2ldt2TwlPm8>>>>>>>>>>>>>>>>>:A2wherel,r,nrepresentthestatevariablesofgovernorsandwaterturbines,asshowninFig.
A1;Kprepresentsthegainofgovernor;Rrepresentstheregulationcoefcientofgovernor;TGandTprepresenttheservotimeconstantandthepilotvalvetimeconstantofgovernor;TdandDdrep-resentsthesoftfeedbacktimeconstantandcoefcientofgovernors;andTwrepresentsthewaterstartingtimeoftheturbine,AppendixBThelinedata,generatordataandgovernordataforIEEE3-machine9-bussystemaregiveninTablesA1,A2andA3,whereVlimitrepresentsthelimitspeedsofwatergate.
AppendixCThegovernordataforIEEE10-machine39-bussystemaregiveninTableA4.
P0R+Kpωωref++ηξ1++PUP.
1TG(1+sTp)PDOWN.
σ1sμ1sTw1+sTw/2PmRsDdTd1+sTdξ2Fig.
A1DiagramsofgovernorandwaterturbineTableA1LinedataforIEEE3-machine9-bussystemTypeStartpointTerminationpointResistance(p.
u.
)Reactance(p.
u.
)Groundcapacitance(p.
u.
)Line450.
03000.
25500.
2640Line460.
01700.
09200.
0790Line570.
09600.
48300.
4590Line690.
09780.
33200.
1290Line780.
02550.
21600.
2235Line890.
03570.
30240.
3135Transformer140.
00000.
0576–Transformer270.
00000.
0625–Transformer390.
00000.
0586–TableA2GeneratordataforIEEE3-machine9-bussystemGeneratornumberInertia(s)X0d(p.
u.
)Xq(p.
u.
)D(p.
u.
)G123.
64000.
06080.
06080.
8000G26.
40000.
11980.
11980.
4000G33.
01000.
18130.
18130.
7000TableA3GovernordataforIEEE3-machine9-bussystemGovernornumberKp(p.
u.
)R(p.
u.
)TG(s)Tp(s)GH10.
50000.
03000.
40000.
0500GH23.
50000.
03000.
15000.
0460GH30.
50000.
03000.
40000.
0500GovernornumberTd(s)Tw(s)Dd(p.
u.
)Vlimit(p.
u.
)GH15.
00000.
50000.
2000±0.
0500GH25.
00000.
50000.
2000±0.
0500GH37.
50001.
50000.
2000±0.
0500TableA4GovernordataforIEEE10-machine39-bussystemGovernornumberKp(p.
u.
)R(p.
u.
)TG(s)Tp(s)GH301.
00000.
03000.
40000.
0500GH311.
00000.
03000.
40000.
0500GH3218.
00000.
03000.
40000.
0500GH3315.
00000.
03000.
40000.
0500GH3413.
00000.
03000.
40000.
0500GH3515.
00000.
03000.
40000.
0500GH3620.
00000.
03000.
10000.
0800GH3720.
00000.
03000.
10000.
0800GH3812.
00000.
03000.
40000.
0500GH391.
00000.
03000.
40000.
0500GovernornumberTd(s)Tw(s)Dd(p.
u.
)Vlimit(p.
u.
)GH305.
00000.
50000.
2000±0.
0500GH315.
00000.
50000.
2000±0.
0500GH325.
00000.
50000.
2000±0.
0500GH335.
00000.
50000.
2000±0.
0500GH345.
00000.
50000.
2000±0.
0500GH355.
00000.
50000.
2000±0.
0500GH365.
00000.
50000.
1000±0.
0500GH375.
00000.
50000.
1000±0.
0500GH385.
00000.
50000.
2000±0.
0500GH395.
00000.
50000.
2000±0.
0500764ZijunBIN,YushengXUE123AppendixDTheeigenvaluesofIEEE10-machine39-bussystematequilibriumpointaregiveninTableA5.
References[1]XueY,YuX(2017)Beyondsmartgrid-cyber-physical-socialsysteminenergyfuture.
ProcIEEE105(12):2290–2292[2]WeiS,ZhouY,HuangY(2017)Synchronousmotor-generatorpairtoenhancesmallsignalandtransientstabilityofpowersystemwithhighpenetrationofrenewableenergy.
IEEEAccess5:11505–11512[3]AnZ,ShenC,ZhengZTetal(2019)Scenario-basedanalysisandprobabilityassessmentofsub-synchronousoscillationcausedbywindfarmswithdirect-drivenwindgenerators.
JModPowerSystCleanEnergy7(2):243-253[4]AbedEH,VaraiyaPP(1984)Nonlinearoscillationsinpowersystems.
IntJElectrPowerEnergySyst6(1):37–43[5]KundurP(1994)Powersystemstabilityandcontrol.
McGrawHill,NewYork,pp699–736[6]YeH,LiuY,ZhangPetal(2017)Analysisanddetectionofforcedoscillationinpowersystem.
IEEETransPowerSyst32(2):1149–1160[7]AjjarapuV,LeeB(1992)Bifurcationtheoryanditsapplicationtononlineardynamicalphenomenainanelectricalpowersys-tem.
IEEETransPowerSyst7(1):424–431[8]SidorovN,SidorovD,LiY(2018)Basinsofattractionofnonlinearsystems'equilibriumpoints:stability,branchingandblow-up.
ActaMathSci.
https://arxiv.
org/pdf/1807.
05487.
pdf.
Accessed10October2018[9]AyasunS,NwankpaCO,KwatnyHG(2004)Computationofsingularandsingularityinducedbifurcationpointsofdifferen-tial-algebraicpowersystemmodel.
IEEETransCircuitsSyst51(8):1525–1538[10]LiuS,MessinaAR,VittalV(2005)Assessingplacementofcontrollersandnonlinearbehaviorusingnormalformanalysis.
IEEETransPowerSyst20(3):1486–1495[11]RodriguezO,MedinaA,MessinaARetal(2009)Themodalseriesmethodandmulti-dimensionalLaplacetransformsfortheanalysisofnonlineareffectsinpowersystemsdynamics.
In:ProceedingsofIEEEPESgeneralmeeting,Calgary,Canada,26–30July2009,pp1–8[12]WangKW,ChungCY,TsangCTetal(2001)ProbabilisticeigenvaluesensitivityindicesforrobustPSSsiteselection.
IEEEProcGenerTransmDistrib148(6):603–609[13]YeH,MouQ,LiuY(2017)Calculationofcriticaloscillationmodesforlargedelayedcyber-physicalpowersystemusingpseudo-spectraldiscretizationofsolutionoperator.
IEEETransPowerSyst32(6):4464–4476[14]GrundCE,PaserbaJJ,HauerJFetal(1993)ComparisonofPronyandeigen-analysisforpowersystemcontroldesign.
IEEETransPowerSyst8(3):964–971[15]BarocioE,PalBC,ThornhillNFetal(2015)Adynamicmodedecompositionframeworkforglobalpowersystemoscillationanalysis.
IEEETransPowerSyst30(6):2902–2912[16]HanS,XuZ,SunBetal(2010)DynamiccharacteristicanalysisofpowersysteminterareaoscillationsusingHHT.
IntJElectrPowerEnergySyst32(10):1085–1090[17]BracaleA,CaramiaP,CarpinelliG(2007)AdaptivePronymethodforwaveformdistortiondetectioninpowersystems.
IntJElectrPowerEnergySyst29(5):371–379[18]PeterOS(2000)Theuseofslidingspectralwindowsforparameterestimationinpowersystemdisturbancemonitoring.
IEEETransPowerSyst15(4):1261–1267[19]RuedaJL,JuarezCA,ErlichI(2011)Wavelet-basedanalysisofpowersystemlow-frequencyelectromechanicaloscillations.
IEEETransPowerSyst26(3):1733–1743[20]JinT,LiuS,FleschRCCetal(2017)Amethodfortheiden-ticationoflowfrequencyoscillationmodesinpowersystemssubjectedtonoise.
ApplEnergy206:1379–1392[21]SidoroyD,PanasetskyD,SˇmadlV(2010)Non-stationaryautoregressivemodelforon-linedetectionofinter-areaoscil-lationsinpowersystems.
In:ProceedingsofIEEEPESISGTEurope,Gothenburg,Sweden,11–13October2010,pp1–5[22]PanX,XueY,ZhangXetal(2008)Analyticalcalculationofpowersystemtrajectoryeigenvaluesanditserroranalysis.
AutomElectricPowerSyst32(19):10–14[23]TanW,ShenC,LiuFetal(2012)Apracticalcriterionfortrajectoryeigenvaluesbasedtransientstabilityanalysis.
AutomElectricPowerSyst36(16):14–19TableA5EigenvaluesofIEEE10-machine39-bussystematequi-libriumpointNumberRealpartImaginarypartNumberRealpartImaginarypart1-19.
40150.
000031-4.
33000.
00002-19.
3980.
000032-4.
22410.
00003-19.
39610.
000033-4.
18980.
00004-19.
33860.
000034-4.
15800.
00005-19.
32760.
000035-4.
13770.
00006-19.
30810.
000036-4.
01380.
00007-19.
3130.
000037-4.
00740.
00008-19.
31550.
000038-4.
00210.
00009-9.
53350.
000039-1.
31160.
000010-9.
24140.
000040-0.
30800.
542711-0.
13268.
563341-0.
3080-0.
542712-0.
1326-8.
563342-1.
05050.
000013-0.
21248.
632643-0.
19880.
000014-0.
2124-8.
632644-0.
75840.
000015-0.
23668.
278445-0.
77790.
000016-0.
2366-8.
278446-0.
77750.
000017-0.
09217.
096247-0.
7770.
000018-0.
0921-7.
096248-0.
76260.
000019-0.
21307.
202349-0.
76570.
000020-0.
2130-7.
202350-0.
76850.
000021-0.
12906.
2769510.
00000.
000022-0.
1290-6.
276952-0.
02890.
000023-0.
15305.
758653-0.
02430.
000024-0.
1530-5.
758654-0.
01980.
000025-0.
10655.
247555-0.
01990.
000026-0.
1065-5.
247556-0.
01990.
000027-6.
78670.
000057-0.
01990.
000028-6.
15960.
000058-0.
01980.
000029-0.
01323.
506059-0.
01980.
000030-0.
0132-3.
506060-0.
01980.
0000Amethodtoextractinstantaneousfeaturesoflowfrequencyoscillationbasedontrajectory…765123[24]XueY,HaoL,WuQHetal(2010)AnnotationforFEPandDSPintermsoftrajectorysectioneigenvalues.
AutomElectricPowerSyst34(12):1–7[25]PanX,XueY,JuP(2013)Reconsiderationoftrajectoryeigenvaluemethod.
AutomElectricPowerSyst37(23):37–44[26]XueY,BinZ(2019)Trajectorysectioneigenvaluemethodfornonlineartime-varyingpowersystem.
IntJElectrPowerEnergySyst107:321–331[27]NiJ,ShenC,TanWetal(2010)Acoherenceidentifyingmethodbasedonlinearizationatnon-equilibriumpoint.
AutomElectricPowerSyst34(20):7–12[28]PaiMA(1989)Energyfunctionanalysisforpowersystemstability.
KluwerAcademicPublishersGroup,Boston,pp223–226[29]XueY(2018)AUniedenergy-domaintheoryofrotor-anglestabilities.
https://dwz.
cn/Ee9i5GnQ.
Accessed20August2018[30]ShestopalVE(1998)StrengtheningoflocalizationtheoremsofGersˇgorinandBrauer.
FundamentalnayaIPrikladnayaMatematika4(1):345–358ZijunBINreceivedhisB.
E.
degreefromHuazhongUniversityofScienceandTechnology,Wuhan,China,in2013.
Currently,heispursuinghisPh.
D.
degreeinShandongUniversity,Jinan,China.
Hisresearchinterestsincludepowersystemstabilityandcontrol.
YushengXUEreceivedtheB.
E.
degreefromShandongUniversity,Jinan,China,in1963,andM.
S.
degreefromStateGridElectricPowerResearchInstitute,Nanjing,China,in1981,respectively.
HereceivedthePh.
D.
degreeinelectricalengineeringfromUniversityofLie`ge,Lie`ge,Belgium,in1987.
Dr.
XueisamemberofChineseAcademyofEngineering,HonoraryPresidentofStateGridElectricPowerResearchInstitute(NARIGroupCorporation),andprofessorintheElectricalEngineeringDepartment,ShandongUniversity.
Hisresearchinterestispowersystemautomation.
766ZijunBIN,YushengXUE123
- U1kkk755.com相关文档
- Nascentkkk755.com
- "买家名称","电子邮件","联系人","公司网址","公司电话","公司传真","公司地址","所在国家","经营产品"
- Moldingkkk755.com
- grownkkk755.com
- devourkkk755.com
- 开封市kkk755.com
Spinservers:美国圣何塞机房少量补货/双E5/64GB DDR4/2TB SSD/10Gbps端口月流量10TB/$111/月
Chia矿机,Spinservers怎么样?Spinservers好不好,Spinservers大硬盘服务器。Spinservers刚刚在美国圣何塞机房补货120台独立服务器,CPU都是双E5系列,64-512GB DDR4内存,超大SSD或NVMe存储,数量有限,机器都是预部署好的,下单即可上架,无需人工干预,有需要的朋友抓紧下单哦。Spinservers是Majestic Hosting So...
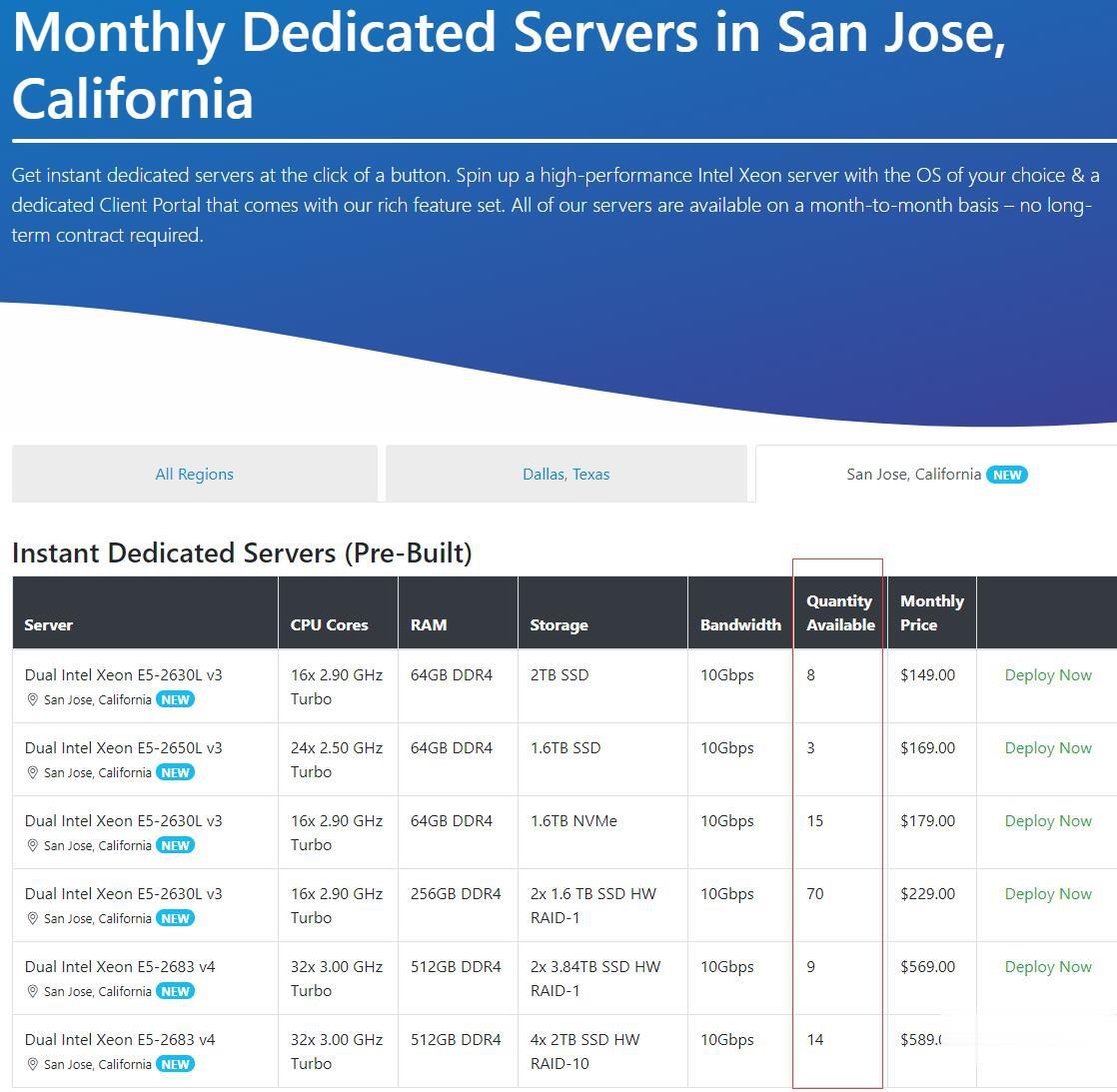
LOCVPS新上日本软银线路VPS,原生IP,8折优惠促销
LOCVPS在农历新年之后新上架了日本大阪机房软银线路VPS主机,基于KVM架构,配备原生IP,适用全场8折优惠码,最低2GB内存套餐优惠后每月仅76元起。LOCVPS是一家成立于2012年的国人VPS服务商,提供中国香港、韩国、美国、日本、新加坡、德国、荷兰、俄罗斯等地区VPS服务器,基于KVM或XEN架构(推荐选择KVM),线路方面均选择国内直连或优化方案,访问延迟低,适合建站或远程办公使用。...

VirMach:$27.3/月-E3-1240v1/16GB/1TB/10TB/洛杉矶等多机房
上次部落分享过VirMach提供的End of Life Plans系列的VPS主机,最近他们又发布了DEDICATED MIGRATION SPECIALS产品,并提供6.5-7.5折优惠码,优惠后最低每月27.3美元起。同样的这些机器现在订购,将在2021年9月30日至2022年4月30日之间迁移,目前这些等待迁移机器可以在洛杉矶、达拉斯、亚特兰大、纽约、芝加哥等5个地区机房开设,未来迁移的时...
kkk755.com为你推荐
-
12306崩溃12306网站显示异常,什么原因啊硬盘工作原理高人指点:电子存储器(U盘,储存卡,硬盘等)的工作原理地图应用什么地图导航最好用最准确比肩工场比肩接踵的意思xyq.163.cbg.comhttp://xyq.cbg.163.com/cgi-bin/equipquery.py?act=buy_show_equip_info&equip_id=475364&server_id=625 有金鱼贵吗?同ip网站12306怎么那么多同IP网站啊?这么重要的一个网站我感觉应该是超强配置的独立服务器才对啊,求高人指点seo优化工具SEO优化要用到什么软件?同ip站点同ip站点很多有没有影响?777k7.comwww 地址 777rv怎么打不开了,还有好看的吗>com同一服务器网站同一服务器上的域名/网址无法访问