naturalspublishingb2t
b2t 时间:2021-02-21 阅读:()
J.
Ana.
Num.
Theor.
2,No.
2,59-63(2014)59JournalofAnalysis&NumberTheoryAnInternationalJournalhttp://dx.
doi.
org/10.
12785/jant/020206NewGeneralizationofEulerianPolynomialsandtheirApplicationsSerkanAraci1,,MehmetAcikgoz1,andErdoganSen2,1DepartmentofMathematics,FacultyofScienceandArts,UniversityofGaziantep,27310Gaziantep,Turkey2DepartmentofMathematics,FacultyofScienceandLetters,NamkKemalUniversity,59030Tekirdag,TurkeyReceived:14Feb.
2014,Revised:20Apr.
2014,Accepted:22Apr.
2014Publishedonline:1Jul.
2014Abstract:Inthepresentpaper,weintroduceEulerianpolynomialswithparametersaandbandgivethedenitionofthem.
Byusingthedenitionofgeneratingfunctionforourpolynomials,wederivesomenewidentitiesinAnalyticNumbersTheory.
Also,wegiverelationsbetweenEulerianpolynomialswithparametersaandb,Bernsteinpolynomials,Poly-logarithmfunctions,BernoulliandEulernumbers.
Moreover,weseethatourpolynomialsata=1arerelatedtoEuler-Zetafunctionatnegativeinetegers.
Finally,wegetWitt'sformulafornewgeneralizationofEulerianpolynomialswhichweexpressinthispaper.
Keywords:Eulerianpolynomials,Poly-logarithmfunctions,Stirlingnumbersofthesecondkind,Bernsteinpolynomials,Bernoullinumbers,EulernumbersandEuler-Zetafunction,p-adicfermionicintegralonZp.
2010MATHEMATICSSUBJECTCLASSIFICATION.
Primary05A10,11B65;Secondary11B68,11B73.
1IntroductionTheBernoullinumbersandpolynomials,Eulernumbersandpolynomials,Genocchinumbersandpolynomials,Stirlingnumbersofthesecondkind,BernsteinpolynomialsandEulerianpolynomialspossessmanyinterestingpropertiesnotonlyincomplexanalysis,andanalyticnumberstheorybutalsoinmathematicalphysicsrelatedtoknottheoryandζ-function,andp-adicanalysis.
Thesepolynomialshavebeenstudiedbymanymathematiciansforalongtime(fordetails,see[1-30]).
Eulerianpolynomialsequence{An(x)}n≥0isgivenbythefollowingsummation:∞∑l=0lnxl=An(x)(1x)n+1,|x|0in(15),becomesAn(a,b)=1a1n1∑k=0nkAk(a,b)(1a)nk(lnb)nk.
(16)Wewanttonotethattakinga=xandb=ein(16)reducestoAn(x)=1x1n1∑k=0nkAk(x)(1x)nk(17)(see[5]and[25]).
Weseethat(17)isproportionalwithBernsteinpolynomialswhichwestateinthefollowingtheorem:c2014NSPNaturalSciencesPublishingCor.
J.
Ana.
Num.
Theor.
2,No.
2,59-63(2014)/www.
naturalspublishing.
com/Journals.
asp61Theorem2.
ThefollowingidentityAn(x)=n1∑k=0Ak(x)Bk,n(x)xk+1xkistrue.
Letusnowconsiderlimt→0dkdtkin(14),thenwereadilyarriveatthefollowingtheorem.
Theorem3.
Letb∈R+anda∈C,thenwehaveAk(a,b)=limt→0dkdtk1abt(1a)a.
(18)By(18),weeasilyconcludethefollowingcorollary.
Corollary1.
ThefollowingCauchy-typeintegralholdstrue:11aAk(a,b)=k!
2πiCtk1bt(1a)adtwhereCisaloopwhichstartsat∞,encirclestheoriginonceinthepositivedirection,andthereturns∞.
By(14),wediscoverthefollowing:∞∑n=0Ana2,b2tnn!
=1abt(1+a)(1a)a1+abt(1a)(1+a)a=∞∑n=0(1+a)nAn(a,b)tnn!
∞∑n=0(1a)nAn(a,b)tnn!
.
ByusingCauchyproductontheaboveequality,thenwegetthefollowingtheorem.
Theorem4.
ThefollowingequalityAna2,b2=∑nk=0nk(1+a)kAk(a,b)Ank(a,b)(1a)nk(19)istrue.
Afterthebasicoperationsin(19),wediscoverthefollowingcorollary.
Corollary2.
Thefollowingpropertyholds:Ana2,b2=n∑k=01+1akBk,n(a)Ak(a,b)Ank(a,b).
Nowalso,weconsidergeometricseriesin(14),thenwecomputeasfollows:∞∑n=0An(a,b)tnn!
=1aet(1a)lnba=1a11a1et(1a)lnb=11a∞∑j=0ajejt(1a)lnb=11a∞∑j=0aj∞∑n=0jn(1a)n(lnb)ntnn!
=∞∑n=011a∞∑j=0ajjn(1a)n(lnb)ntnn!
.
Bycomparingthecoefcientsoftnn!
ontheaboveequation,thenwereadilyderivethefollowingtheorem.
Theorem5.
Thefollowing1a1nAn(a,b)=(lnb)na(lnb)n∞∑j=1ajjnistrue.
TheabovetheoremisrelatedtoPoly-logarithmfunction,asfollows:1a1nAn(a,b)=(lnb)na(lnb)nLina1.
(20)In[27],itiswell-knownthatLin(x)=xddxnx1x=∑nk=0k!
S(n+1,k+1)x1xk+1(21)whereS(n,k)aretheStirlingnumbersofthesecondkind.
By(20)and(21),wehavethefollowinginterestingtheorem.
Theorem6.
Thefollowingholdstrue:aAn(a,b)=(lnb)nn∑k=0k!
S(n+1,k+1)1a1kn.
3FurtherRemarksNow,weconsider(14)forevaluatingata=1,asfollows:∞∑n=0An(1,b)tnn!
=2b2t+1(22)whereAn(1,b)arecalledEulerianpolynomialswithparameterb.
By(22),wederivethefollowingequalityincomplexplane:∞∑n=0inAn(1,b)tnn!
=2b2it+1=2e2itlnb+1.
Fromthis,wediscoverthefollowing:∞∑n=0inAn(1,b)tnn!
=∞∑n=0En2nin(lnb)tnn!
(23)whereEnaren-thEulernumberswhicharedenedbythefollowingexponentialgeneratingfunction:∞∑n=0Entnn!
=2et+1,|t|0,thenwehaveAn(1,b)=2n+1(lnb)n∞∑j=1(1)jjn.
(26)Asiswellknown,Euler-zetafunctionisdenedbyζE(s)=2∞∑j=1(1)jjs,s∈C(see[3]).
(27)From(26)and(27),weobtaintheinterpolationfunctionofnewgeneralizationofEulerianpolynomialsata=1,asfollow:An(1,b)=2n(lnb)nζE(n).
(28)Equation(28)seemstobeinterpolationfunctionatnegativeintegersforEulerianpolynomialswithparameterb.
LetusnowconsiderWitt'sformulaforourpolynomialsata=1,soweneedthefollowingnotations:Imaginethatpbeaxedoddprimenumber.
Throughoutthispaper,weusethefollowingnotations.
ByZp,wedenotetheringofp-adicrationalintegers,Qdenotestheeldofrationalnumbers,Qpdenotestheeldofp-adicrationalnumbers,andCpdenotesthecompletionofalgebraicclosureofQp.
LetNbethesetofnaturalnumbersandN=N∪{0}.
Thenormalizedp-adicabsolutevalueisdenedby|p|p=1p.
Letqbeanindeterminatewith|q1|pb2t+1=∞∑n=0An(1,b)tnn!
.
(31)By(31)andusingTaylorexpansionofe2tυlnb,weobtainWitt'sformulaforourpolynomialsata=1,asfollows:Theorem11.
Thefollowingholdstrue:An(1,b)=(lnb)n2nXυnd1(υ).
(32)Equation(32)seemstobeinterestingforourfurtherworksintheconceptofp-adicintegrals.
References[1]T.
Kim,IdentitiesinvolvingFrobenius-Eulerpolynomialsarisingfromnon-lineardifferentialequations,JournalofNumberTheory,132,2854-2865(2012).
[2]T.
Kim,Someidentitiesontheq-Eulerpolynomialsofhigherorderandq-stirlingnumbersbythefermionicp-adicintegralonZp,RussianJ.
Math.
Phys.
,16,484–491(2009).
[3]T.
Kim,Eulernumbersandpolynomialsassociatedwithzetafunctions,AbstractandAppliedAnalysis,vol.
2008,ArticleID581582,11pages,2008.
[4]T.
Kim,SomeidentitiesfortheBernoulli,theEulerandtheGenocchinumbersandpolynomials,AdvStudContempMath.
,20,23–28(2010).
[5]D.
S.
Kim,T.
Kim,W.
J.
KimandD.
V.
Dolgy,AnoteonEulerianpolynomials,AbstractandAppliedAnalysis,Volume2012(2012),ArticleID269640,10pages.
[6]D.
S.
Kim,T.
Kim,Y.
H.
Kim,andD.
V.
Dolgy,AnoteonEulerianpolynomialsassociatedwithBernoulliandEulernumbersandpolynomials,AdvancedStudiesinContemporaryMathematics,22,342–353(2012).
[7]M.
AcikgozandY.
Simsek,OnmultipleinterpolationfunctionsoftheN¨orlund-typeq-Eulerpolynomials,AbstractandAppliedAnalysis,2009,ArticleID382574,14pages.
[8]M.
AcikgozandS.
Araci,OnthegeneratingfunctionsforBernsteinpolynomials,NumericalAnalysisandAppliedMathematics,Amer.
Inst.
Phys.
Conf.
Proc.
CP1281,1141-1143(2010).
[9]S.
Araci,M.
AcikgozandD.
Gao,OntheDirichlet'stypeofEulerianpolynomials,arXiv:1207.
1834[math.
NT][10]S.
AraciandM.
Acikgoz,Dirichlet'stypeoftwistedEulerianpolynomialsinconnectionwithtwistedDirichlet'stype-L-function,arXiv:1208.
0589[math.
NT][11]S.
Araci,D.
ErdalandJ.
J.
Seo,Astudyonthefermionicp-adicq-integralrepresentationonZpassociatedwithweightedq-Bernsteinandq-Genocchipolynomials,AbstractandAppliedAnalysis,2011,ArticleID649248,10pages.
[12]S.
Araci,M.
Acikgoz,andJ.
J.
Seo,Explicitformulasinvolvingq-Eulernumbersandpolynomials,AbstractandAppliedAnalysis,2012,ArticleID298531,11pages.
[13]E.
Cetin,M.
Acikgoz,I.
N.
Cangul,andS.
Araci,Anoteonthe(h,q)-Zeta-typefunctionwithweightα,JournalofInequalitiesandApplications,2013,2013:100.
[14]S.
Araci,M.
Acikgoz,andA.
Kilicman,Extendedp-adicq-invariantintegralsonZpassociatedwithapplicationsofumbralcalculus,AdvancesinDifferenceEquations2013,2013:96.
[15]S.
Araci,M.
Acikgoz,andF.
Qi,Ontheq-Genocchinumbersandpolynomialswithweightzeroandtheirinterpolationfunctions,NonlinearFunctionalAnalysisandApplications,18,193-203(2013).
[16]G.
Birkhoff,C.
deBoor,Piecewisepolynomialinterpolationandapproximation,Proc.
Sympos.
GeneralMotorsRes.
Lab.
,,ElsevierPubl.
Co.
,Amsterdam,1965,164–190(1964).
[17]I.
N.
Cangul,H.
Ozden,andY.
Simsek,Generatingfunctionsofthe(h,q)extensionoftwistedEulerpolynomialsandnumbers,ActaMathematicaHungarica,120,281–299(2008).
[18]L.
Carlitz,Euleriannumbersandpolynomials,MathematicsMagazine,32,247-260.
[19]L.
Carlitz,q-BernoulliandEuleriannumbers,TransactionsoftheAmericanMathematicalSociety,76,332-350(1954).
[20]L.
Carlitz,Acombinatorialpropertyofq-Euleriannumbers,Amer.
Math.
Monthly,82,51–54(1975).
[21]F.
Hirzebruch,Eulerianpolynomials,M¨unsterJ.
ofMath.
,1,9–14(2008).
[22]L.
C.
Jang,V.
Kurt,Y.
Simsek,andS.
H.
Rim,q-analogueofthep-adictwistedl-function,JournalofConcreteandApplicableMathematics,6,169–176,(2008).
[23]H.
Jolany,R.
E.
AlikelayeandS.
S.
Mohamad,SomeresultsonthegeneralizationofBernoulli,EulerandGenocchipolynomials,ActaUniversitatisApulensis,299-306(2011).
[24]H.
JolanyandH.
Shari,SomeresultsfortheApostol-Genocchipolynomialsofhigherorder,Bull.
Malays.
Math.
Sci.
Soc.
,36,465-479(2013).
[25]D.
Foata,Eulerianpolynomials:fromEuler'stimetothepresent,ThelegacyofAlladiRamakrishnaninthemathematicalsciences,253–273,Springer,NewYork,2010.
[26]J.
ChoiandH.
M.
Srivastava,ThemultipleHurwitzZetafunctionandthemultipleHurwitz-Eulerzetafunction,TaiwaneseJournalofMathematics,15,501-522(2011).
[27]L.
Lewin,Polylogarithmsandassociatedfunctions,NorthHolland,(1981).
[28]Q.
M.
Luo,F.
Qi,andL.
Debnath,GeneralizationsofEulernumbersandpolynomials,IJMMS.
2003,3893-3901(2003).
[29]Q.
M.
Luo,B.
N.
Guo,F.
Qi,andL.
Debnath,GeneralizationofBernoullinumbersandpolynomials,IJMMS,2003,3769-3776(2003).
[30]H.
M.
SrivastavaandJ.
Choi,SeriesAssociatedwiththeZetaandRelatedFunctions,KluwerAcademicPublishers,Dordrecht,BostonandLondon,(2001).
c2014NSPNaturalSciencesPublishingCor.
Ana.
Num.
Theor.
2,No.
2,59-63(2014)59JournalofAnalysis&NumberTheoryAnInternationalJournalhttp://dx.
doi.
org/10.
12785/jant/020206NewGeneralizationofEulerianPolynomialsandtheirApplicationsSerkanAraci1,,MehmetAcikgoz1,andErdoganSen2,1DepartmentofMathematics,FacultyofScienceandArts,UniversityofGaziantep,27310Gaziantep,Turkey2DepartmentofMathematics,FacultyofScienceandLetters,NamkKemalUniversity,59030Tekirdag,TurkeyReceived:14Feb.
2014,Revised:20Apr.
2014,Accepted:22Apr.
2014Publishedonline:1Jul.
2014Abstract:Inthepresentpaper,weintroduceEulerianpolynomialswithparametersaandbandgivethedenitionofthem.
Byusingthedenitionofgeneratingfunctionforourpolynomials,wederivesomenewidentitiesinAnalyticNumbersTheory.
Also,wegiverelationsbetweenEulerianpolynomialswithparametersaandb,Bernsteinpolynomials,Poly-logarithmfunctions,BernoulliandEulernumbers.
Moreover,weseethatourpolynomialsata=1arerelatedtoEuler-Zetafunctionatnegativeinetegers.
Finally,wegetWitt'sformulafornewgeneralizationofEulerianpolynomialswhichweexpressinthispaper.
Keywords:Eulerianpolynomials,Poly-logarithmfunctions,Stirlingnumbersofthesecondkind,Bernsteinpolynomials,Bernoullinumbers,EulernumbersandEuler-Zetafunction,p-adicfermionicintegralonZp.
2010MATHEMATICSSUBJECTCLASSIFICATION.
Primary05A10,11B65;Secondary11B68,11B73.
1IntroductionTheBernoullinumbersandpolynomials,Eulernumbersandpolynomials,Genocchinumbersandpolynomials,Stirlingnumbersofthesecondkind,BernsteinpolynomialsandEulerianpolynomialspossessmanyinterestingpropertiesnotonlyincomplexanalysis,andanalyticnumberstheorybutalsoinmathematicalphysicsrelatedtoknottheoryandζ-function,andp-adicanalysis.
Thesepolynomialshavebeenstudiedbymanymathematiciansforalongtime(fordetails,see[1-30]).
Eulerianpolynomialsequence{An(x)}n≥0isgivenbythefollowingsummation:∞∑l=0lnxl=An(x)(1x)n+1,|x|0in(15),becomesAn(a,b)=1a1n1∑k=0nkAk(a,b)(1a)nk(lnb)nk.
(16)Wewanttonotethattakinga=xandb=ein(16)reducestoAn(x)=1x1n1∑k=0nkAk(x)(1x)nk(17)(see[5]and[25]).
Weseethat(17)isproportionalwithBernsteinpolynomialswhichwestateinthefollowingtheorem:c2014NSPNaturalSciencesPublishingCor.
J.
Ana.
Num.
Theor.
2,No.
2,59-63(2014)/www.
naturalspublishing.
com/Journals.
asp61Theorem2.
ThefollowingidentityAn(x)=n1∑k=0Ak(x)Bk,n(x)xk+1xkistrue.
Letusnowconsiderlimt→0dkdtkin(14),thenwereadilyarriveatthefollowingtheorem.
Theorem3.
Letb∈R+anda∈C,thenwehaveAk(a,b)=limt→0dkdtk1abt(1a)a.
(18)By(18),weeasilyconcludethefollowingcorollary.
Corollary1.
ThefollowingCauchy-typeintegralholdstrue:11aAk(a,b)=k!
2πiCtk1bt(1a)adtwhereCisaloopwhichstartsat∞,encirclestheoriginonceinthepositivedirection,andthereturns∞.
By(14),wediscoverthefollowing:∞∑n=0Ana2,b2tnn!
=1abt(1+a)(1a)a1+abt(1a)(1+a)a=∞∑n=0(1+a)nAn(a,b)tnn!
∞∑n=0(1a)nAn(a,b)tnn!
.
ByusingCauchyproductontheaboveequality,thenwegetthefollowingtheorem.
Theorem4.
ThefollowingequalityAna2,b2=∑nk=0nk(1+a)kAk(a,b)Ank(a,b)(1a)nk(19)istrue.
Afterthebasicoperationsin(19),wediscoverthefollowingcorollary.
Corollary2.
Thefollowingpropertyholds:Ana2,b2=n∑k=01+1akBk,n(a)Ak(a,b)Ank(a,b).
Nowalso,weconsidergeometricseriesin(14),thenwecomputeasfollows:∞∑n=0An(a,b)tnn!
=1aet(1a)lnba=1a11a1et(1a)lnb=11a∞∑j=0ajejt(1a)lnb=11a∞∑j=0aj∞∑n=0jn(1a)n(lnb)ntnn!
=∞∑n=011a∞∑j=0ajjn(1a)n(lnb)ntnn!
.
Bycomparingthecoefcientsoftnn!
ontheaboveequation,thenwereadilyderivethefollowingtheorem.
Theorem5.
Thefollowing1a1nAn(a,b)=(lnb)na(lnb)n∞∑j=1ajjnistrue.
TheabovetheoremisrelatedtoPoly-logarithmfunction,asfollows:1a1nAn(a,b)=(lnb)na(lnb)nLina1.
(20)In[27],itiswell-knownthatLin(x)=xddxnx1x=∑nk=0k!
S(n+1,k+1)x1xk+1(21)whereS(n,k)aretheStirlingnumbersofthesecondkind.
By(20)and(21),wehavethefollowinginterestingtheorem.
Theorem6.
Thefollowingholdstrue:aAn(a,b)=(lnb)nn∑k=0k!
S(n+1,k+1)1a1kn.
3FurtherRemarksNow,weconsider(14)forevaluatingata=1,asfollows:∞∑n=0An(1,b)tnn!
=2b2t+1(22)whereAn(1,b)arecalledEulerianpolynomialswithparameterb.
By(22),wederivethefollowingequalityincomplexplane:∞∑n=0inAn(1,b)tnn!
=2b2it+1=2e2itlnb+1.
Fromthis,wediscoverthefollowing:∞∑n=0inAn(1,b)tnn!
=∞∑n=0En2nin(lnb)tnn!
(23)whereEnaren-thEulernumberswhicharedenedbythefollowingexponentialgeneratingfunction:∞∑n=0Entnn!
=2et+1,|t|0,thenwehaveAn(1,b)=2n+1(lnb)n∞∑j=1(1)jjn.
(26)Asiswellknown,Euler-zetafunctionisdenedbyζE(s)=2∞∑j=1(1)jjs,s∈C(see[3]).
(27)From(26)and(27),weobtaintheinterpolationfunctionofnewgeneralizationofEulerianpolynomialsata=1,asfollow:An(1,b)=2n(lnb)nζE(n).
(28)Equation(28)seemstobeinterpolationfunctionatnegativeintegersforEulerianpolynomialswithparameterb.
LetusnowconsiderWitt'sformulaforourpolynomialsata=1,soweneedthefollowingnotations:Imaginethatpbeaxedoddprimenumber.
Throughoutthispaper,weusethefollowingnotations.
ByZp,wedenotetheringofp-adicrationalintegers,Qdenotestheeldofrationalnumbers,Qpdenotestheeldofp-adicrationalnumbers,andCpdenotesthecompletionofalgebraicclosureofQp.
LetNbethesetofnaturalnumbersandN=N∪{0}.
Thenormalizedp-adicabsolutevalueisdenedby|p|p=1p.
Letqbeanindeterminatewith|q1|pb2t+1=∞∑n=0An(1,b)tnn!
.
(31)By(31)andusingTaylorexpansionofe2tυlnb,weobtainWitt'sformulaforourpolynomialsata=1,asfollows:Theorem11.
Thefollowingholdstrue:An(1,b)=(lnb)n2nXυnd1(υ).
(32)Equation(32)seemstobeinterestingforourfurtherworksintheconceptofp-adicintegrals.
References[1]T.
Kim,IdentitiesinvolvingFrobenius-Eulerpolynomialsarisingfromnon-lineardifferentialequations,JournalofNumberTheory,132,2854-2865(2012).
[2]T.
Kim,Someidentitiesontheq-Eulerpolynomialsofhigherorderandq-stirlingnumbersbythefermionicp-adicintegralonZp,RussianJ.
Math.
Phys.
,16,484–491(2009).
[3]T.
Kim,Eulernumbersandpolynomialsassociatedwithzetafunctions,AbstractandAppliedAnalysis,vol.
2008,ArticleID581582,11pages,2008.
[4]T.
Kim,SomeidentitiesfortheBernoulli,theEulerandtheGenocchinumbersandpolynomials,AdvStudContempMath.
,20,23–28(2010).
[5]D.
S.
Kim,T.
Kim,W.
J.
KimandD.
V.
Dolgy,AnoteonEulerianpolynomials,AbstractandAppliedAnalysis,Volume2012(2012),ArticleID269640,10pages.
[6]D.
S.
Kim,T.
Kim,Y.
H.
Kim,andD.
V.
Dolgy,AnoteonEulerianpolynomialsassociatedwithBernoulliandEulernumbersandpolynomials,AdvancedStudiesinContemporaryMathematics,22,342–353(2012).
[7]M.
AcikgozandY.
Simsek,OnmultipleinterpolationfunctionsoftheN¨orlund-typeq-Eulerpolynomials,AbstractandAppliedAnalysis,2009,ArticleID382574,14pages.
[8]M.
AcikgozandS.
Araci,OnthegeneratingfunctionsforBernsteinpolynomials,NumericalAnalysisandAppliedMathematics,Amer.
Inst.
Phys.
Conf.
Proc.
CP1281,1141-1143(2010).
[9]S.
Araci,M.
AcikgozandD.
Gao,OntheDirichlet'stypeofEulerianpolynomials,arXiv:1207.
1834[math.
NT][10]S.
AraciandM.
Acikgoz,Dirichlet'stypeoftwistedEulerianpolynomialsinconnectionwithtwistedDirichlet'stype-L-function,arXiv:1208.
0589[math.
NT][11]S.
Araci,D.
ErdalandJ.
J.
Seo,Astudyonthefermionicp-adicq-integralrepresentationonZpassociatedwithweightedq-Bernsteinandq-Genocchipolynomials,AbstractandAppliedAnalysis,2011,ArticleID649248,10pages.
[12]S.
Araci,M.
Acikgoz,andJ.
J.
Seo,Explicitformulasinvolvingq-Eulernumbersandpolynomials,AbstractandAppliedAnalysis,2012,ArticleID298531,11pages.
[13]E.
Cetin,M.
Acikgoz,I.
N.
Cangul,andS.
Araci,Anoteonthe(h,q)-Zeta-typefunctionwithweightα,JournalofInequalitiesandApplications,2013,2013:100.
[14]S.
Araci,M.
Acikgoz,andA.
Kilicman,Extendedp-adicq-invariantintegralsonZpassociatedwithapplicationsofumbralcalculus,AdvancesinDifferenceEquations2013,2013:96.
[15]S.
Araci,M.
Acikgoz,andF.
Qi,Ontheq-Genocchinumbersandpolynomialswithweightzeroandtheirinterpolationfunctions,NonlinearFunctionalAnalysisandApplications,18,193-203(2013).
[16]G.
Birkhoff,C.
deBoor,Piecewisepolynomialinterpolationandapproximation,Proc.
Sympos.
GeneralMotorsRes.
Lab.
,,ElsevierPubl.
Co.
,Amsterdam,1965,164–190(1964).
[17]I.
N.
Cangul,H.
Ozden,andY.
Simsek,Generatingfunctionsofthe(h,q)extensionoftwistedEulerpolynomialsandnumbers,ActaMathematicaHungarica,120,281–299(2008).
[18]L.
Carlitz,Euleriannumbersandpolynomials,MathematicsMagazine,32,247-260.
[19]L.
Carlitz,q-BernoulliandEuleriannumbers,TransactionsoftheAmericanMathematicalSociety,76,332-350(1954).
[20]L.
Carlitz,Acombinatorialpropertyofq-Euleriannumbers,Amer.
Math.
Monthly,82,51–54(1975).
[21]F.
Hirzebruch,Eulerianpolynomials,M¨unsterJ.
ofMath.
,1,9–14(2008).
[22]L.
C.
Jang,V.
Kurt,Y.
Simsek,andS.
H.
Rim,q-analogueofthep-adictwistedl-function,JournalofConcreteandApplicableMathematics,6,169–176,(2008).
[23]H.
Jolany,R.
E.
AlikelayeandS.
S.
Mohamad,SomeresultsonthegeneralizationofBernoulli,EulerandGenocchipolynomials,ActaUniversitatisApulensis,299-306(2011).
[24]H.
JolanyandH.
Shari,SomeresultsfortheApostol-Genocchipolynomialsofhigherorder,Bull.
Malays.
Math.
Sci.
Soc.
,36,465-479(2013).
[25]D.
Foata,Eulerianpolynomials:fromEuler'stimetothepresent,ThelegacyofAlladiRamakrishnaninthemathematicalsciences,253–273,Springer,NewYork,2010.
[26]J.
ChoiandH.
M.
Srivastava,ThemultipleHurwitzZetafunctionandthemultipleHurwitz-Eulerzetafunction,TaiwaneseJournalofMathematics,15,501-522(2011).
[27]L.
Lewin,Polylogarithmsandassociatedfunctions,NorthHolland,(1981).
[28]Q.
M.
Luo,F.
Qi,andL.
Debnath,GeneralizationsofEulernumbersandpolynomials,IJMMS.
2003,3893-3901(2003).
[29]Q.
M.
Luo,B.
N.
Guo,F.
Qi,andL.
Debnath,GeneralizationofBernoullinumbersandpolynomials,IJMMS,2003,3769-3776(2003).
[30]H.
M.
SrivastavaandJ.
Choi,SeriesAssociatedwiththeZetaandRelatedFunctions,KluwerAcademicPublishers,Dordrecht,BostonandLondon,(2001).
c2014NSPNaturalSciencesPublishingCor.
- naturalspublishingb2t相关文档
- addedb2t
- 丁基b2t
- 英文版b2t
- 王淦昌b2t
- simulationsb2t
- metricsb2t
WHloud Date鲸云数据($9.00/月), 韩国,日本,香港
WHloud Date(鲸云数据),原做大数据和软件开发的团队,现在转变成云计算服务,面对海内外用户提供中国大陆,韩国,日本,香港等多个地方节点服务。24*7小时的在线支持,较为全面的虚拟化构架以及全方面的技术支持!官方网站:https://www.whloud.com/WHloud Date 韩国BGP云主机少量补货随时可以开通,随时可以用,两小时内提交退款,可在工作日期间全额原路返回!支持pa...

sharktech:老牌高防服务器商,跳楼价,1G独享$70、10G共享$240、10G独享$800
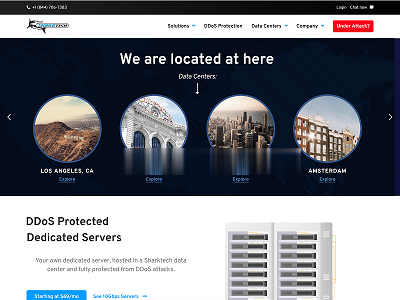
不知道大家是否注意到sharktech的所有服务器的带宽价格全部跳楼跳水,降幅简直不忍直视了,还没有见过这么便宜的独立服务器。根据不同的机房,价格也是不一样的。大带宽、不限流量比较适合建站、数据备份、做下载、做流媒体、做CDN等多种业务。 官方网站:https://www.sharktech.net 付款方式:比特币、信用卡、PayPal、支付宝、西联汇款 以最贵的洛杉矶机器为例,配置表如...
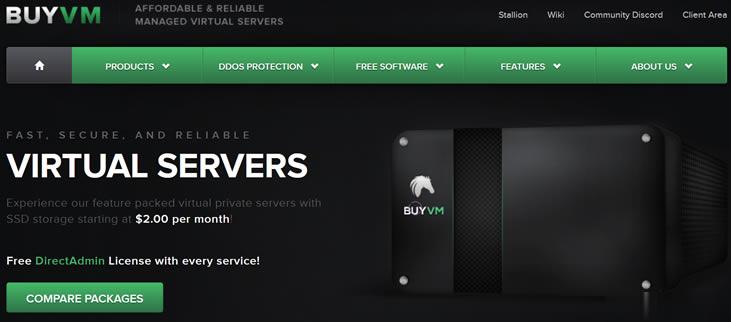
BuyVM($5/月)不限流量流媒体优化VPS主机 1GB内存
BuyVM商家属于比较老牌的服务商,早年有提供低价年付便宜VPS主机还记得曾经半夜的时候抢购的。但是由于这个商家风控非常严格,即便是有些是正常的操作也会导致被封账户,所以后来陆续无人去理睬,估计被我们风控的抢购低价VPS主机已经手足无措。这两年商家重新调整,而且风控也比较规范,比如才入手他们新上线的流媒体优化VPS主机也没有不适的提示。目前,BuyVM商家有提供新泽西、迈阿密等四个机房的VPS主机...
b2t为你推荐
-
苏州商标注册苏州商标局在哪里windows优化大师怎么用如何用Windows优化大师??深圳公交车路线深圳公交车路线查询bluestacks安卓模拟器BlueStacks如何安装使用?自助建站自助建站到底好还是不好网站运营网络运营主管的主要工作职责是什么?网站联盟网络联盟是什么意思镜像文件是什么系统镜像是什么qq空间装扮qq空间怎么装扮qq空间打扮如何打扮QQ空间?