dominated腾讯qq好友恢复
腾讯qq好友恢复 时间:2021-05-25 阅读:()
Exploringpossiblyexistingqqbbtetraquarkstateswithqq=ud,ss,ccAntjePetersa,PedroBicudob,KrzysztofCichya,c,BjrnWagenbacha,MarcWagneraaGoethe-UniversittFrankfurtamMain,InstitutfürTheoretischePhysik,Max-von-Laue-Strae1,D-60438FrankfurtamMain,GermanybCFTP,DepartamentodeFísica,InstitutoSuperiorTécnico,UniversidadedeLisboa,AvenidaRoviscoPais,1049-001Lisboa,PortugalcAdamMickiewiczUniversity,FacultyofPhysics,Umultowska85,61-614Poznan,PolandE-mail:peters@th.
physik.
uni-frankfurt.
de,bicudo@tecnico.
ulisboa.
pt,kcichy@th.
physik.
uni-frankfurt.
de,wagenbach@th.
physik.
uni-frankfurt.
de,mwagner@th.
physik.
uni-frankfurt.
deWecomputepotentialsoftwostaticantiquarksinthepresenceoftwoquarksqqofnitemassusinglatticeQCD.
InasecondstepwesolvetheSchrdingerequation,todetermine,whethertheresultingpotentialsaresufcientlyattractivetohostaboundstate,whichwouldindicatetheexistenceofastableqqbbtetraquark.
Wendaboundstateforqq=(uddu)/√2withcorrespondingquantumnumbersI(JP)=0(1+)andevidenceagainsttheexistenceofboundstateswithisospinI=1orqq∈{cc,ss}.
The33rdInternationalSymposiumonLatticeFieldTheory14-18July2015KobeInternationalConferenceCenter,Kobe,JapanSpeaker.
cCopyrightownedbytheauthor(s)underthetermsoftheCreativeCommonsAttribution-NonCommercial-ShareAlikeLicence.
http://pos.
sissa.
it/arXiv:1508.
00343v1[hep-lat]3Aug2015Exploringpossiblyexistingqqbbtetraquarkstateswithqq=ud,ss,ccAntjePeters1.
MotivationAnumberofmesonsobservedinexperimentslikeLHCborBellearenotwellunderstood.
Thosemesonshavemassesandquantumnumbers,whicharenottypicalforstandardquark-antiquarkstates,butindicateanexoticfour-quarkstructure.
Prominentexamplesarethechargedcharmonium-likeandbottomonium-likestatesZc±andZb±(cf.
e.
g.
[1]).
Theirmassesanddecayproductssuggestthepresenceofaccorbbpair,respectively.
Ontheotherhandtheirelectricchargeindicatesadditionallyalightquark-antiquarkpairudordu.
Thosefour-quarksystems,inthefol-lowingalsoreferredtoastetraquarks,areexpectedtobestudiedinmoredetailinthenearfuturebyexperimentalcollaborations.
Therefore,asoundtheoreticalunderstandingofthosesystemsiscrucialandofgreatinterest.
Herewesummarizethemainresultsofourrecentlypublishedwork[2],wherewehavestudiedfour-quarksystemswithtwoheavyantiquarksbbandtwolighterquarksqqusinglatticeQCDandtheBorn-Oppenheimerapproximation.
Firstbbpotentialsinthepresenceoflighterquarksqqarecomputed.
ThentheSchrdingerequationissolvedusingthesepotentials,wherepossiblyexistingboundstatesindicatestabletetraquarks.
Otherpapersstudyingthesamesystemswithsimilarmethodsinclude[3,4,5,6,7,8,9,10,11,12,13,14].
2.
QualitativediscussionofqqbbsystemsAtsmallbbseparationsthebbinteractionisdominatedby1-gluonexchange.
Foraboundstatethebbpairmust,therefore,beinanattractivecolortriplet.
DuetothePauliprincipleandbecauseweassumeaspatiallysymmetrics-wave,bbhastoformanantisymmetriccolor-spin-avorcombinationand,hence,asymmetricspincombination,i.
e.
bbspinjb=1.
Sincethecompletefour-quarksystemiscolorneutral,thelightquarksqqmustbeinanantisymmetriccolorantitriplet.
AgainduetothePauliprincipleqqhastoformanantisymmetriccolor-spin-avorcombinationand,hence,asymmetricspin-avorcombination.
Candidatesfortetraquarksare,therefore,the(spin)scalarisosinglet(i.
e.
aqqspinsingletj=0withantisymmetricavor,e.
g.
qq∈{(uddu)/√2,(s(1)s(2)s(2)s(1))/√2,(c(1)c(2)c(2)c(1))/√2}1)andthe(spin)vectorisotriplet(i.
e.
aqqspintripletj=1withsymmetricavor,e.
g.
qq∈{uu,(ud+du)/√2,dd,ss,cc}).
TheoverallquantumnumbersofaboundqqbbsystemareI(JP)=0(1+)forthescalarisosingletchannelandI(JP)∈{1(0+),1(1+),1(2+)}forthevectorisotripletchannel.
Atlargebbseparationsthebbinteractionisscreenedbythelightquarksqq,i.
e.
thefourquarksformasystemoftwoheavy-lightmesons.
Oneexpectsstrongerscreeningforincreasingquarkmassmq,becausethewavefunctionsofthecorrespondingmesonsqbarethenmorecompact.
3.
LatticeQCDcomputationofstaticantiquark-antiquarkpotentialsWeextractpotentialsoftwostaticantiquarksQQ(approximatingthetwobquarksoftheqqbbsystem)inthepresenceoftwolightquarksqqfromcorrelationfunctionsC(t,r)=|O(t)O(0)|∝t→∞exp(V(r)t).
(3.
1)1Tobeabletostudyavorantisymmetricqqcombinationswithq=s,weconsidertwohypotheticaldegenerateavorswiththemassofthesquark,s(1)ands(2),andsimilarlyforq=c,c(1)andc(2).
2Exploringpossiblyexistingqqbbtetraquarkstateswithqq=ud,ss,ccAntjePetersOdenotesafour-quarkcreationoperator,O=(CΓ)AB(CΓ)CDQC(r1)q(1)A(r1)QD(r2)q(2)B(r2),r=|r1r2|,(3.
2)whereΓisanappropriatecombinationofγmatricesaccountingfordenedquantumnumberslightquarkspin|jz|,parityPandPx(cf.
[8]fordetails).
Γ∈{(1γ0)γ5,(1γ0)γj}doesnotaffecttheresultingpotentialV(r),sincethestaticquarkspinisirrelevant.
C=γ0γ2denotesthechargeconjugationmatrix.
Notethatoperatorslike(3.
2)generateoverlapnotonlytomesonicmoleculestructures,butalsotodiquark-antidiquarkstructures[15,16].
Theasymptoticvalueofapotentialandwhetheritisattractiveorrepulsivedependsonthequantumnumbers(|jz|,P,Px)and,hence,onΓ.
Inthefollowingweareexclusivelyinterestedinat-tractivepotentialsbetweentwogroundstatestatic-lightmesons:thescalarisosingletcorrespondingtoΓ=(1+γ0)γ5andthevectorisotripletcorrespondingtoΓ=(1+γ0)γj.
Computationshavebeenperformedusingtwoensemblesofgaugelinkcongurationsgener-atedbytheEuropeanTwistedMassCollaboration(ETMC)withdynamicalu/dquarks.
Informa-tionontheseensemblescanbefoundinTable1and[17,18].
βlatticesizelainfmmπinMeV#congurations3.
90243*480.
004000.
0793404804.
35323*640.
001750.
042352100Table1:Ensemblesofgaugelinkcongurations(β:inversegaugecoupling;l:bareu/dquarkmassinlatticeunits;a:latticespacing;mπ:pionmass).
4.
qqbbtetraquarksintheBorn-OppenheimerapproximationTodetermineananalyticalexpressionfortheQQpotentialorequivalentlybbpotential,wettheansatzV(r)=αrexprd2+V0(4.
1)withrespecttoα,dandV0tothelatticeQCDresultsobtainedintheprevioussection.
TheconstantV0accountsfortwicethemassofthegroundstatestatic-lightmeson.
Weinsertthetheanalyticalexpression(4.
1)intheSchrdingerequationfortheradialcoordi-nateofthetwobquarks(whichweassumetobeinans-wave),12d2dr2+U(r)R(r)=EBR(r)(4.
2)withU(r)=V(r)|V0=0and=mb/2anddeterminethelowesteigenvalueEB.
IfEB0,thereisnobinding,i.
e.
thefour-quarksystemwillalwaysbeasystemoftwounboundBmesons.
Noticethatthisso-calledBorn-Oppenheimerapproximationisvalidformqmb,whichiscertainlythecaseforq∈{u,d,s}andatleastcrudelyfullledforq=c.
Toquantifythesystematicerrorsofdifferentchannels(scalarisosingletandvectorisotriplet,differentlightavorsq∈{u,d,s,c}),weperformalargenumberoftsvaryingtherangeof3Exploringpossiblyexistingqqbbtetraquarkstateswithqq=ud,ss,ccAntjePeterstemporalseparationstmin≤t≤tmaxofthecorrelationfunctionC(t,r)(cf.
eq.
(3.
1)),atwhichthelatticepotentialisreadoff,aswellastherangeofspatialbbseparationsrmin≤r≤rmaxconsideredintheχ2minimizingtofeq.
(4.
1)tothelatticepotential.
Detailsonthisparametervariationcanbefoundin[2].
Foreachsetofinputparameters(tmin,tmax,rmin,rmax)wedetermineα,dandEB.
Thenwegeneratehistogramsforα,dandEBweightedaccordingtothecorrespondingχ2/dof.
Thewidthsofthesehistogramsaretakenassystematicerrorsofα,dandEB[19],whilethestatisticalerrorsareobtainedviaajackknifeanalysis.
InFigure1examplehistogramsforthescalarisosingletforqq=udareshown.
Figure1:Histogramsforthescalarisosingletforqq=ud.
Thered/green/bluebarsindicatethestatistical/systematic/combinederrors.
Theresultingpotentialstsfordifferentchannels,i.
e.
eq.
(4.
1)withcorrespondingvaluesforαandd,arecollectedinFigure2.
Theerrorbandsrepresentthecombinedsystematicandstatisticalerrors.
Onecanobservethatthepotentialsarewideranddeeperforlighterqqquarkmasses.
Moreover,thescalarchannelsaremoreattractivethantherespectivevectorchannels.
Correspondingly,itturnsoutthatthereisaboundstateonlyforthescalarisosingletwithqq=udwithbindingenergyEB=93+4743MeV,i.
e.
aboundstatewitharound2σcondencelevel.
InFigure3wepresentourresultsinanalternativegraphicalway.
Thethreeplotscorrespondtou/d,sandclightquarksqq,respectively.
Eachtofeq.
(4.
1)tolatticepotentialresultsisrepresentedbyadot(red:scalarchannels;green:vectorchannels;crosses:rmin=2a;boxes:rmin=3a).
Theextensionsofthepointcloudsrepresentthesystematicuncertaintieswithrespecttoαandd.
IfapointcloudislocalizedaboveorleftoftheisolinewithEB=0.
1MeV(essentiallythebindingthreshold),thecorrespondingfourquarksqqbbcannotformaboundstate.
Alocalizationbeloworrightofthatisolineisastrongindicationfortheexistenceofatetraquark.
Againthereisclearevidenceforatetraquarkstateinthescalaru/dchannel.
Thescalarschannelisslightlyabove,butratherclosetothebindingthreshold.
Thescalarcandallvectorchannelsclearlydonothostboundfour-quarkstates.
5.
SummaryandoutlookWehavefoundaudbbtetraquarkwithquantumnumbersI(JP)=0(1+)(i.
e.
inthescalarisosingletchannelwithqq=ud)withacondencelevelofaround2σ.
Thereseemtoexistno4Exploringpossiblyexistingqqbbtetraquarkstateswithqq=ud,ss,ccAntjePetersFigure2:Potentialstsfordifferentchannels(upperline:scalarisosinglet;lowerline:vectorisotriplet).
Thecurveswithoutanerrorbandarecopiedfromtherespectiveotherplotsinthesamelineforeasycomparison.
Verticallinesindicatetheavailablelatticebbseparations.
tetraquarksfortheotherchannels.
InthisworklatticeQCDcomputationshavebeenperformedforlightu/dquarkscorrespond-ingtomπ≈340MeV.
Weplantorepeattheanalysisforatleastanotherpionmassandthenextrapolatetothephysicalpoint.
Itwillthenbemostinterestingtocheck,whetheraboundstatewillalsoappearinthevectorisotripletchannelwithqq=ud.
AnotheraspectistoinvestigatethestructureofthefoundI(JP)=0(1+)tetraquark,i.
e.
toexplore,whetheritisratheramesonicmoleculeoradiquark-antidiquarkpair.
Wealsoplantoincludecorrectionsduetotheheavyquarkspins(forrstpreliminaryresultscf.
[14]).
Finally,oneshouldstudytheexperimentallymoreaccessible,buttheoreticallymorechallengingcaseofqqbbsystems.
AcknowledgmentsP.
B.
thanksIFTforhospitalityandCFTP,grantFCTUID/FIS/00777/2013,forsupport.
M.
W.
andA.
P.
acknowledgesupportbytheEmmyNoetherProgrammeoftheDFG(GermanResearchFoundation),grantWA3000/1-1.
ThisworkwassupportedinpartbytheHelmholtzInternationalCenterforFAIRwithintheframeworkoftheLOEWEprogramlaunchedbytheStateofHesse.
5Exploringpossiblyexistingqqbbtetraquarkstateswithqq=ud,ss,ccAntjePetersFigure3:BindingenergyisolinesEB=constantintheα-d-planeforu/d,sandclightquarksqqtogetherwiththetresultsofeq.
(4.
1)tolatticepotentials.
6Exploringpossiblyexistingqqbbtetraquarkstateswithqq=ud,ss,ccAntjePetersCalculationsontheLOEWE-CSChigh-performancecomputerofJohannWolfgangGoethe-UniversityFrankfurtamMainwereconductedforthisresearch.
WewouldliketothankHPC-Hessen,fundedbytheStateMinistryofHigherEducation,ResearchandtheArts,forprogrammingadvice.
References[1]A.
Bondaretal.
[BelleCollaboration],Phys.
Rev.
Lett.
108,122001(2012)[arXiv:1110.
2251[hep-ex]].
[2]P.
Bicudo,K.
Cichy,A.
Peters,B.
WagenbachandM.
Wagner,Phys.
Rev.
D92,014507(2015)[arXiv:1505.
00613[hep-lat]].
[3]C.
StewartandR.
Koniuk,Phys.
Rev.
D57,5581(1998)[arXiv:hep-lat/9803003].
[4]C.
MichaelandP.
Pennanen[UKQCDCollaboration],Phys.
Rev.
D60,054012(1999)[arXiv:hep-lat/9901007].
[5]M.
S.
CookandH.
R.
Fiebig,arXiv:hep-lat/0210054.
[6]T.
Doi,T.
T.
TakahashiandH.
Suganuma,AIPConf.
Proc.
842,246(2006)[arXiv:hep-lat/0601008].
[7]W.
Detmold,K.
OrginosandM.
J.
Savage,Phys.
Rev.
D76,114503(2007)[arXiv:hep-lat/0703009].
[8]M.
Wagner[ETMCollaboration],PoSLATTICE2010,162(2010)[arXiv:1008.
1538[hep-lat]].
[9]G.
BaliandM.
Hetzenegger,PoSLATTICE2010,142(2010)[arXiv:1011.
0571[hep-lat]].
[10]M.
Wagner[ETMCollaboration],ActaPhys.
Polon.
Supp.
4,747(2011)[arXiv:1103.
5147[hep-lat]].
[11]P.
BicudoandM.
Wagner,Phys.
Rev.
D87,114511(2013)[arXiv:1209.
6274[hep-ph]].
[12]Z.
S.
BrownandK.
Orginos,Phys.
Rev.
D86,114506(2012)[arXiv:1210.
1953[hep-lat]].
[13]B.
Wagenbach,P.
BicudoandM.
Wagner,J.
Phys.
Conf.
Ser.
599,012006(2015)[arXiv:1411.
2453[hep-lat]].
[14]J.
Scheunert,P.
Bicudo,A.
UenverandM.
Wagner,arXiv:1505.
03496[hep-ph].
[15]C.
Alexandrou,J.
O.
Daldrop,M.
DallaBrida,M.
Gravina,L.
Scorzato,C.
UrbachandM.
Wagner,[ETMCollaboration],JHEP1304,137(2013)[arXiv:1212.
1418].
[16]A.
Abdel-Rehim,C.
Alexandrou,J.
Berlin,M.
DallaBrida,M.
GravinaandM.
Wagner,PoSLATTICE2014,104(2014)[arXiv:1410.
8757[hep-lat]].
[17]P.
Boucaudetal.
[ETMCollaboration],Comput.
Phys.
Commun.
179,695(2008)[arXiv:0803.
0224[hep-lat]].
[18]R.
Baronetal.
[ETMCollaboration],JHEP1008,097(2010)[arXiv:0911.
5061[hep-lat]].
[19]K.
Cichy,V.
Drach,E.
Garcia-Ramos,G.
HerdoizaandK.
Jansen,Nucl.
Phys.
B869,131(2013)[arXiv:1211.
1605[hep-lat]].
7
physik.
uni-frankfurt.
de,bicudo@tecnico.
ulisboa.
pt,kcichy@th.
physik.
uni-frankfurt.
de,wagenbach@th.
physik.
uni-frankfurt.
de,mwagner@th.
physik.
uni-frankfurt.
deWecomputepotentialsoftwostaticantiquarksinthepresenceoftwoquarksqqofnitemassusinglatticeQCD.
InasecondstepwesolvetheSchrdingerequation,todetermine,whethertheresultingpotentialsaresufcientlyattractivetohostaboundstate,whichwouldindicatetheexistenceofastableqqbbtetraquark.
Wendaboundstateforqq=(uddu)/√2withcorrespondingquantumnumbersI(JP)=0(1+)andevidenceagainsttheexistenceofboundstateswithisospinI=1orqq∈{cc,ss}.
The33rdInternationalSymposiumonLatticeFieldTheory14-18July2015KobeInternationalConferenceCenter,Kobe,JapanSpeaker.
cCopyrightownedbytheauthor(s)underthetermsoftheCreativeCommonsAttribution-NonCommercial-ShareAlikeLicence.
http://pos.
sissa.
it/arXiv:1508.
00343v1[hep-lat]3Aug2015Exploringpossiblyexistingqqbbtetraquarkstateswithqq=ud,ss,ccAntjePeters1.
MotivationAnumberofmesonsobservedinexperimentslikeLHCborBellearenotwellunderstood.
Thosemesonshavemassesandquantumnumbers,whicharenottypicalforstandardquark-antiquarkstates,butindicateanexoticfour-quarkstructure.
Prominentexamplesarethechargedcharmonium-likeandbottomonium-likestatesZc±andZb±(cf.
e.
g.
[1]).
Theirmassesanddecayproductssuggestthepresenceofaccorbbpair,respectively.
Ontheotherhandtheirelectricchargeindicatesadditionallyalightquark-antiquarkpairudordu.
Thosefour-quarksystems,inthefol-lowingalsoreferredtoastetraquarks,areexpectedtobestudiedinmoredetailinthenearfuturebyexperimentalcollaborations.
Therefore,asoundtheoreticalunderstandingofthosesystemsiscrucialandofgreatinterest.
Herewesummarizethemainresultsofourrecentlypublishedwork[2],wherewehavestudiedfour-quarksystemswithtwoheavyantiquarksbbandtwolighterquarksqqusinglatticeQCDandtheBorn-Oppenheimerapproximation.
Firstbbpotentialsinthepresenceoflighterquarksqqarecomputed.
ThentheSchrdingerequationissolvedusingthesepotentials,wherepossiblyexistingboundstatesindicatestabletetraquarks.
Otherpapersstudyingthesamesystemswithsimilarmethodsinclude[3,4,5,6,7,8,9,10,11,12,13,14].
2.
QualitativediscussionofqqbbsystemsAtsmallbbseparationsthebbinteractionisdominatedby1-gluonexchange.
Foraboundstatethebbpairmust,therefore,beinanattractivecolortriplet.
DuetothePauliprincipleandbecauseweassumeaspatiallysymmetrics-wave,bbhastoformanantisymmetriccolor-spin-avorcombinationand,hence,asymmetricspincombination,i.
e.
bbspinjb=1.
Sincethecompletefour-quarksystemiscolorneutral,thelightquarksqqmustbeinanantisymmetriccolorantitriplet.
AgainduetothePauliprincipleqqhastoformanantisymmetriccolor-spin-avorcombinationand,hence,asymmetricspin-avorcombination.
Candidatesfortetraquarksare,therefore,the(spin)scalarisosinglet(i.
e.
aqqspinsingletj=0withantisymmetricavor,e.
g.
qq∈{(uddu)/√2,(s(1)s(2)s(2)s(1))/√2,(c(1)c(2)c(2)c(1))/√2}1)andthe(spin)vectorisotriplet(i.
e.
aqqspintripletj=1withsymmetricavor,e.
g.
qq∈{uu,(ud+du)/√2,dd,ss,cc}).
TheoverallquantumnumbersofaboundqqbbsystemareI(JP)=0(1+)forthescalarisosingletchannelandI(JP)∈{1(0+),1(1+),1(2+)}forthevectorisotripletchannel.
Atlargebbseparationsthebbinteractionisscreenedbythelightquarksqq,i.
e.
thefourquarksformasystemoftwoheavy-lightmesons.
Oneexpectsstrongerscreeningforincreasingquarkmassmq,becausethewavefunctionsofthecorrespondingmesonsqbarethenmorecompact.
3.
LatticeQCDcomputationofstaticantiquark-antiquarkpotentialsWeextractpotentialsoftwostaticantiquarksQQ(approximatingthetwobquarksoftheqqbbsystem)inthepresenceoftwolightquarksqqfromcorrelationfunctionsC(t,r)=|O(t)O(0)|∝t→∞exp(V(r)t).
(3.
1)1Tobeabletostudyavorantisymmetricqqcombinationswithq=s,weconsidertwohypotheticaldegenerateavorswiththemassofthesquark,s(1)ands(2),andsimilarlyforq=c,c(1)andc(2).
2Exploringpossiblyexistingqqbbtetraquarkstateswithqq=ud,ss,ccAntjePetersOdenotesafour-quarkcreationoperator,O=(CΓ)AB(CΓ)CDQC(r1)q(1)A(r1)QD(r2)q(2)B(r2),r=|r1r2|,(3.
2)whereΓisanappropriatecombinationofγmatricesaccountingfordenedquantumnumberslightquarkspin|jz|,parityPandPx(cf.
[8]fordetails).
Γ∈{(1γ0)γ5,(1γ0)γj}doesnotaffecttheresultingpotentialV(r),sincethestaticquarkspinisirrelevant.
C=γ0γ2denotesthechargeconjugationmatrix.
Notethatoperatorslike(3.
2)generateoverlapnotonlytomesonicmoleculestructures,butalsotodiquark-antidiquarkstructures[15,16].
Theasymptoticvalueofapotentialandwhetheritisattractiveorrepulsivedependsonthequantumnumbers(|jz|,P,Px)and,hence,onΓ.
Inthefollowingweareexclusivelyinterestedinat-tractivepotentialsbetweentwogroundstatestatic-lightmesons:thescalarisosingletcorrespondingtoΓ=(1+γ0)γ5andthevectorisotripletcorrespondingtoΓ=(1+γ0)γj.
Computationshavebeenperformedusingtwoensemblesofgaugelinkcongurationsgener-atedbytheEuropeanTwistedMassCollaboration(ETMC)withdynamicalu/dquarks.
Informa-tionontheseensemblescanbefoundinTable1and[17,18].
βlatticesizelainfmmπinMeV#congurations3.
90243*480.
004000.
0793404804.
35323*640.
001750.
042352100Table1:Ensemblesofgaugelinkcongurations(β:inversegaugecoupling;l:bareu/dquarkmassinlatticeunits;a:latticespacing;mπ:pionmass).
4.
qqbbtetraquarksintheBorn-OppenheimerapproximationTodetermineananalyticalexpressionfortheQQpotentialorequivalentlybbpotential,wettheansatzV(r)=αrexprd2+V0(4.
1)withrespecttoα,dandV0tothelatticeQCDresultsobtainedintheprevioussection.
TheconstantV0accountsfortwicethemassofthegroundstatestatic-lightmeson.
Weinsertthetheanalyticalexpression(4.
1)intheSchrdingerequationfortheradialcoordi-nateofthetwobquarks(whichweassumetobeinans-wave),12d2dr2+U(r)R(r)=EBR(r)(4.
2)withU(r)=V(r)|V0=0and=mb/2anddeterminethelowesteigenvalueEB.
IfEB0,thereisnobinding,i.
e.
thefour-quarksystemwillalwaysbeasystemoftwounboundBmesons.
Noticethatthisso-calledBorn-Oppenheimerapproximationisvalidformqmb,whichiscertainlythecaseforq∈{u,d,s}andatleastcrudelyfullledforq=c.
Toquantifythesystematicerrorsofdifferentchannels(scalarisosingletandvectorisotriplet,differentlightavorsq∈{u,d,s,c}),weperformalargenumberoftsvaryingtherangeof3Exploringpossiblyexistingqqbbtetraquarkstateswithqq=ud,ss,ccAntjePeterstemporalseparationstmin≤t≤tmaxofthecorrelationfunctionC(t,r)(cf.
eq.
(3.
1)),atwhichthelatticepotentialisreadoff,aswellastherangeofspatialbbseparationsrmin≤r≤rmaxconsideredintheχ2minimizingtofeq.
(4.
1)tothelatticepotential.
Detailsonthisparametervariationcanbefoundin[2].
Foreachsetofinputparameters(tmin,tmax,rmin,rmax)wedetermineα,dandEB.
Thenwegeneratehistogramsforα,dandEBweightedaccordingtothecorrespondingχ2/dof.
Thewidthsofthesehistogramsaretakenassystematicerrorsofα,dandEB[19],whilethestatisticalerrorsareobtainedviaajackknifeanalysis.
InFigure1examplehistogramsforthescalarisosingletforqq=udareshown.
Figure1:Histogramsforthescalarisosingletforqq=ud.
Thered/green/bluebarsindicatethestatistical/systematic/combinederrors.
Theresultingpotentialstsfordifferentchannels,i.
e.
eq.
(4.
1)withcorrespondingvaluesforαandd,arecollectedinFigure2.
Theerrorbandsrepresentthecombinedsystematicandstatisticalerrors.
Onecanobservethatthepotentialsarewideranddeeperforlighterqqquarkmasses.
Moreover,thescalarchannelsaremoreattractivethantherespectivevectorchannels.
Correspondingly,itturnsoutthatthereisaboundstateonlyforthescalarisosingletwithqq=udwithbindingenergyEB=93+4743MeV,i.
e.
aboundstatewitharound2σcondencelevel.
InFigure3wepresentourresultsinanalternativegraphicalway.
Thethreeplotscorrespondtou/d,sandclightquarksqq,respectively.
Eachtofeq.
(4.
1)tolatticepotentialresultsisrepresentedbyadot(red:scalarchannels;green:vectorchannels;crosses:rmin=2a;boxes:rmin=3a).
Theextensionsofthepointcloudsrepresentthesystematicuncertaintieswithrespecttoαandd.
IfapointcloudislocalizedaboveorleftoftheisolinewithEB=0.
1MeV(essentiallythebindingthreshold),thecorrespondingfourquarksqqbbcannotformaboundstate.
Alocalizationbeloworrightofthatisolineisastrongindicationfortheexistenceofatetraquark.
Againthereisclearevidenceforatetraquarkstateinthescalaru/dchannel.
Thescalarschannelisslightlyabove,butratherclosetothebindingthreshold.
Thescalarcandallvectorchannelsclearlydonothostboundfour-quarkstates.
5.
SummaryandoutlookWehavefoundaudbbtetraquarkwithquantumnumbersI(JP)=0(1+)(i.
e.
inthescalarisosingletchannelwithqq=ud)withacondencelevelofaround2σ.
Thereseemtoexistno4Exploringpossiblyexistingqqbbtetraquarkstateswithqq=ud,ss,ccAntjePetersFigure2:Potentialstsfordifferentchannels(upperline:scalarisosinglet;lowerline:vectorisotriplet).
Thecurveswithoutanerrorbandarecopiedfromtherespectiveotherplotsinthesamelineforeasycomparison.
Verticallinesindicatetheavailablelatticebbseparations.
tetraquarksfortheotherchannels.
InthisworklatticeQCDcomputationshavebeenperformedforlightu/dquarkscorrespond-ingtomπ≈340MeV.
Weplantorepeattheanalysisforatleastanotherpionmassandthenextrapolatetothephysicalpoint.
Itwillthenbemostinterestingtocheck,whetheraboundstatewillalsoappearinthevectorisotripletchannelwithqq=ud.
AnotheraspectistoinvestigatethestructureofthefoundI(JP)=0(1+)tetraquark,i.
e.
toexplore,whetheritisratheramesonicmoleculeoradiquark-antidiquarkpair.
Wealsoplantoincludecorrectionsduetotheheavyquarkspins(forrstpreliminaryresultscf.
[14]).
Finally,oneshouldstudytheexperimentallymoreaccessible,buttheoreticallymorechallengingcaseofqqbbsystems.
AcknowledgmentsP.
B.
thanksIFTforhospitalityandCFTP,grantFCTUID/FIS/00777/2013,forsupport.
M.
W.
andA.
P.
acknowledgesupportbytheEmmyNoetherProgrammeoftheDFG(GermanResearchFoundation),grantWA3000/1-1.
ThisworkwassupportedinpartbytheHelmholtzInternationalCenterforFAIRwithintheframeworkoftheLOEWEprogramlaunchedbytheStateofHesse.
5Exploringpossiblyexistingqqbbtetraquarkstateswithqq=ud,ss,ccAntjePetersFigure3:BindingenergyisolinesEB=constantintheα-d-planeforu/d,sandclightquarksqqtogetherwiththetresultsofeq.
(4.
1)tolatticepotentials.
6Exploringpossiblyexistingqqbbtetraquarkstateswithqq=ud,ss,ccAntjePetersCalculationsontheLOEWE-CSChigh-performancecomputerofJohannWolfgangGoethe-UniversityFrankfurtamMainwereconductedforthisresearch.
WewouldliketothankHPC-Hessen,fundedbytheStateMinistryofHigherEducation,ResearchandtheArts,forprogrammingadvice.
References[1]A.
Bondaretal.
[BelleCollaboration],Phys.
Rev.
Lett.
108,122001(2012)[arXiv:1110.
2251[hep-ex]].
[2]P.
Bicudo,K.
Cichy,A.
Peters,B.
WagenbachandM.
Wagner,Phys.
Rev.
D92,014507(2015)[arXiv:1505.
00613[hep-lat]].
[3]C.
StewartandR.
Koniuk,Phys.
Rev.
D57,5581(1998)[arXiv:hep-lat/9803003].
[4]C.
MichaelandP.
Pennanen[UKQCDCollaboration],Phys.
Rev.
D60,054012(1999)[arXiv:hep-lat/9901007].
[5]M.
S.
CookandH.
R.
Fiebig,arXiv:hep-lat/0210054.
[6]T.
Doi,T.
T.
TakahashiandH.
Suganuma,AIPConf.
Proc.
842,246(2006)[arXiv:hep-lat/0601008].
[7]W.
Detmold,K.
OrginosandM.
J.
Savage,Phys.
Rev.
D76,114503(2007)[arXiv:hep-lat/0703009].
[8]M.
Wagner[ETMCollaboration],PoSLATTICE2010,162(2010)[arXiv:1008.
1538[hep-lat]].
[9]G.
BaliandM.
Hetzenegger,PoSLATTICE2010,142(2010)[arXiv:1011.
0571[hep-lat]].
[10]M.
Wagner[ETMCollaboration],ActaPhys.
Polon.
Supp.
4,747(2011)[arXiv:1103.
5147[hep-lat]].
[11]P.
BicudoandM.
Wagner,Phys.
Rev.
D87,114511(2013)[arXiv:1209.
6274[hep-ph]].
[12]Z.
S.
BrownandK.
Orginos,Phys.
Rev.
D86,114506(2012)[arXiv:1210.
1953[hep-lat]].
[13]B.
Wagenbach,P.
BicudoandM.
Wagner,J.
Phys.
Conf.
Ser.
599,012006(2015)[arXiv:1411.
2453[hep-lat]].
[14]J.
Scheunert,P.
Bicudo,A.
UenverandM.
Wagner,arXiv:1505.
03496[hep-ph].
[15]C.
Alexandrou,J.
O.
Daldrop,M.
DallaBrida,M.
Gravina,L.
Scorzato,C.
UrbachandM.
Wagner,[ETMCollaboration],JHEP1304,137(2013)[arXiv:1212.
1418].
[16]A.
Abdel-Rehim,C.
Alexandrou,J.
Berlin,M.
DallaBrida,M.
GravinaandM.
Wagner,PoSLATTICE2014,104(2014)[arXiv:1410.
8757[hep-lat]].
[17]P.
Boucaudetal.
[ETMCollaboration],Comput.
Phys.
Commun.
179,695(2008)[arXiv:0803.
0224[hep-lat]].
[18]R.
Baronetal.
[ETMCollaboration],JHEP1008,097(2010)[arXiv:0911.
5061[hep-lat]].
[19]K.
Cichy,V.
Drach,E.
Garcia-Ramos,G.
HerdoizaandK.
Jansen,Nucl.
Phys.
B869,131(2013)[arXiv:1211.
1605[hep-lat]].
7
- dominated腾讯qq好友恢复相关文档
- 支持腾讯qq好友恢复
- 胶州市民政系统信息平台(QQ群)联系表
- 引流腾讯qq好友恢复
- 腾讯qq好友恢复QQ好友如何恢复?
- 腾讯qq好友恢复QQ安全中心怎么恢复好友
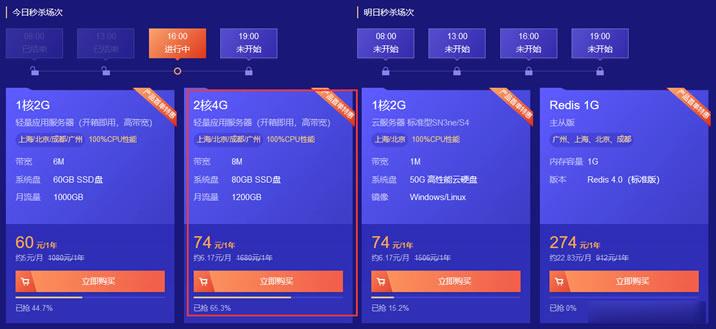
腾讯云轻量服务器两款低价年付套餐 2核4GB内存8M带宽 年74元
昨天,有在"阿里云秋季促销活动 轻量云服务器2G5M配置新购年60元"文章中记录到阿里云轻量服务器2GB内存、5M带宽一年60元的活动,当然这个也是国内机房的。我们很多人都清楚备案是需要接入的,如果我们在其他服务商的域名备案的,那是不能解析的。除非我们不是用来建站,而是用来云端的,是可以用的。这不看到其对手腾讯云也有推出两款轻量服务器活动。其中一款是4GB内存、8M带宽,这个比阿里云还要狠。这个真...
NameSilo域名优惠码活动
NameSilo是通过之前的感恩节优惠活动中认识到这家注册商的,于是今天早上花了点时间专门了解了NameSilo优惠码和商家的详细信息。该商家只销售域名,他们家的域名销售价格还是中规中矩的,没有像godaddy域名标价和使用优惠之后的价格悬殊很大,而且其特色就是该域名平台提供免费的域名停放、免费隐私保护等功能。namesilo新注册域名价格列表,NameSilo官方网站:www.namesilo....

RackNerd 2022春节促销提供三款年付套餐 低至年付10.88美元
RackNerd 商家我们应该是比较熟悉的商家,速度一般,但是人家便宜且可选机房也是比较多的,较多集中在美国机房。包括前面的新年元旦促销的时候有提供年付10美元左右的方案,实际上RackNerd商家的营销策略也是如此,每逢节日都有活动,配置简单变化,价格基本差不多,所以我们网友看到没有必要囤货,有需要就选择。RackNerd 商家这次2022农历新年也是有几款年付套餐。低至RackNerd VPS...

腾讯qq好友恢复为你推荐
-
回收卡巴斯基股份一卡通系统人才ipad买家googlegetIntjavatcpip上的netbiostcpip上的netbios是什么用的,有安全隐患吗?开启还是关上重庆电信宽带测速重庆电信光纤宽带网络,内外网络和电脑等设备都没发生变化的情况下,办理了从20M提速到100M业务。win7关闭135端口win7系统 怎么关闭135 445 端口 修改注册表 创建IP安全策略 也试过 就是关不了 还望高手指教micromediawww.macromedia.com 是什么网站morphvoxpro怎么用MorphVOX Pro变声器声音怎样调试