cancellingxvm
xvm 时间:2021-01-24 阅读:()
RepresentationTheoryCT,Lent20051WhatisRepresentationTheoryGroupsariseinnatureas"setsofsymmetries(ofanobject),whichareclosedundercompo-sitionandundertakinginverses".
Forexample,thesymmetricgroupSnisthegroupofallpermutations(symmetries)of{1,n};thealternatinggroupAnisthesetofallsymmetriespreservingtheparityofthenumberoforderedpairs(didyoureallyrememberthatone);thedihedralgroupD2nisthegroupofsymmetriesoftheregularn-gonintheplane.
TheorthogonalgroupO(3)isthegroupofdistance-preservingtransformationsofEuclideanspacewhichxtheorigin.
Thereisalsothegroupofalldistance-preservingtransformations,whichincludesthetranslationsalongwithO(3).
1Theocialdenitionisofcoursemoreabstract,agroupisasetGwithabinaryoperationwhichisassociative,hasaunitelementeandforwhichinversesexist.
Associativityallowsaconvenientabuseofnotation,wherewewriteghforgh;wehaveghk=(gh)k=g(hk)andparenthesesareunnecessary.
Iwilloftenwrite1fore,butthisisdangerousonrareoccasions,suchaswhenstudyingthegroupZunderaddition;inthatcase,e=0.
Theabstractdenitionnotwithstanding,theinterestingsituationinvolvesagroup"acting"onaset.
Formally,anactionofagroupGonasetXisan"actionmap"a:G*X→Xwhichiscompatiblewiththegrouplaw,inthesensethata(h,a(g,x))=a(hg,x)anda(e,x)=x.
Thisjustiestheabusivenotationa(g,x)=g.
xorevengx,forwehaveh(gx)=(hg)x.
Fromthispointofview,geometryasks,"GivenageometricobjectX,whatisitsgroupofsymmetries"Representationtheoryreversesthequestionto"GivenagroupG,whatobjectsXdoesitacton"andattemptstoanswerthisquestionbyclassifyingsuchXuptoisomorphism.
Beforerestrictingtothelinearcase,ourmainconcern,letusrememberanotherwaytodescribeanactionofGonX.
Everyg∈Gdenesamapa(g):X→Xbyx→gx.
Thismapisabijection,withinversemapa(g1):indeed,a(g1)a(g)(x)=g1gx=ex=xfromthepropertiesoftheaction.
Hencea(g)belongstothesetPerm(X)ofbijectiveself-mapsofX.
Thissetformsagroupundercomposition,andthepropertiesofanactionimplythat1.
1Proposition.
AnactionofGonX"isthesameas"agrouphomomorphismα:G→Perm(X).
1.
2Remark.
Thereisalogicalabusehere,clearlyanaction,denedasamapa:G*X→XisnotthesameasthehomomorphismαintheProposition;youaremeanttoreadthatspecifyingoneiscompletelyequivalenttospecifyingtheother,unambiguously.
Butthedenitionsaredesignedtoallowsuchabusewithoutmuchdanger,andIwillfrequentlyindulgeinthat(infactIdenotedαbyainlecture).
21Thisgroupisisomorphictothesemi-directproductO(3)R3—butifyoudonotknowwhatthismeans,donotworry.
2Withrespecttoabuse,youmaywishtoerronthesideofcautionwhenwritingupsolutionsinyourexam!
1ThereformulationofProp.
1.
1leadstothefollowingobservation.
ForanyactionaHonXandgrouphomomorphism:G→H,thereisdenedarestrictedorpulled-backactionaofGonX,asa=a.
Intheoriginaldenition,theactionsends(g,x)to(g)(x).
(1.
3)Example:TautologicalactionofPerm(X)onXThisistheobviousaction,callitT,sending(f,x)tof(x),wheref:X→Xisabijectionandx∈X.
Checkthatitsatisesthepropertiesofanaction!
Inthislanguage,theactionaofGonXisαT,withthehomomorphismαoftheproposition—thepull-backunderαofthetautologicalaction.
(1.
4)Linearity.
ThequestionofclassifyingallpossibleXwithactionofGishopelessinsuchgenerality,butoneshouldrecallthat,inrstapproximation,mathematicsislinear.
SoweshalltakeourXtoavectorspaceoversomegroundeld,andaskthattheactionofGbelinear,aswell,inotherwords,thatitshouldpreservethevectorspacestructure.
OurinterestismostlyconnedtothecasewhentheeldofscalarsisC,althoughweshalloccasionalmentionhowthepicturechangeswhenothereldsarestudied.
1.
5Denition.
AlinearrepresentationρofGonacomplexvectorspaceVisaset-theoreticactiononVwhichpreservesthelinearstructure,thatis,ρ(g)(v1+v2)=ρ(g)v1+ρ(g)v2,v1,2∈V,ρ(g)(kv)=k·ρ(g)v,k∈C,v∈VUnlessotherwisementioned,representationwillmeannite-dimensionalcomplexrepresentation.
(1.
6)Example:ThegenerallineargroupLetVbeacomplexvectorspaceofdimensionnm.
Moregenerally,dimΛmV=1anddimΛkV=mk.
Notation:Inthesymmetricpower,weoftenuseu1untodenotethesymmetrisedvectorsinProp.
7.
4,whileintheexteriorpower,oneusesu1un.
8ThecharacterofarepresentationWenowturntothecoreresultsofthecourse,thecharactertheoryofgrouprepresentations.
Thisallowsaneectivecalculuswithgrouprepresentations,includingtheirtensorproducts,andtheirdecompositionintoirreducibles.
WewanttoattachinvariantstoarepresentationρofanitegroupGonV.
Thematrixcoecientsofρ(g)arebasis-dependent,hencenottrueinvariants.
Observe,however,thatggeneratesanitecyclicsubgroupofG;thisimpliesthefollowing(seeLecture2).
8.
1Proposition.
IfGanddimVarenite,theneveryρ(g)isdiagonalisable.
Moreprecisely,alleigenvaluesofρ(g)willberootsofunityofordersdividingthatofG.
(ApplyLagrange'stheoremtothecyclicsubgroupgeneratedbyg.
)Toeachg∈G,wecanassignitseigenvaluesonV,uptoorder.
Numericalinvariantsresultfromtheelementarysymmetricfunctionsoftheseeigenvalues,whichyoualsoknowasthecoecientsofthecharacteristicpolynomialdetV[λρ(g)].
Especiallymeaningfularetheconstantterm,detρ(g)(uptosign);thesub-leadingterm,Trρ(g)(uptosign).
Thefollowingisclearfrommultiplicativityofthedeterminant:8.
2Proposition.
Themapg→detVρ(g)∈Cdenesa1-dimensionalrepresentationofG.
Thisisanice,but"weak"invariant.
Forinstance,youmayknowthatthealternatinggroupA5issimple,thatis,ithasnopropernormalsubgroups.
Becauseitisnotabelian,anyhomomorphismtoCmustbetrivial,sothedeterminantofanyofitsrepresentationsis1.
Evenforabeliangroups,thedeterminantistooweaktodistinguishisomorphismclassesofrepresentations.
Thewinnerturnsouttobetheotherinterestinginvariant.
8.
3Denition.
Thecharacteroftherepresentationρisthecomplex-valuedfunctiononGdenedbyχρ(g):=TrV(ρ(g)).
TheBigTheoremofthecourseisthatthisisacompleteinvariant,inthesensethatitdeterminesρuptoisomorphism.
Forarepresentationρ:G→GL(V),wehavedenedχV:G→CbyχV(g)=TrV(ρ(g)).
8.
4Theorem(Firstproperties).
1.
χVisconjugation-invariant,χV(hgh1)=χV(g),g,h∈G;2.
χV(1)=dimV;3.
χV(g1)=χV(g);4.
FortworepresentationsV,W,χVW=χV+χW,andχVW=χV·χW;5.
ForthedualrepresentationV,χV(g)=χV(g1).
17Proof.
Parts(1)and(2)areclear.
Forpart(3),chooseaninvariantinnerproductonV;unitarityofρ(g)impliesthatρ(g1)=ρ(g)1=ρ(g)T,whence(3)followsbytakingthetrace.
4Part(4)isclearfromTrA00B=TrA+TrB,andρVW=ρV00ρW.
TheproductformulafollowsformtheidentityTr(AB)=TrA·TrBfromLecture6.
Finally,Part(5)followsformthefactthattheactionofgonalinearmapL∈Hom(V;C)=Viscompositionwithρ(g)1(Lecture5).
8.
5Remark.
conjugation-invariantfunctionsonGarealsocalledcentralfunctionsorclassfunc-tions.
Theirvalueatagroupelementgdependsonlyontheconjugacyclassofg.
Wecanthereforeviewthemasfunctionsonthesetofconjugacyclasses.
(8.
6)TherepresentationringWecanrewriteproperty(4)moreprofessionallybyintroducinganalgebraicconstruct.
8.
7Denition.
TherepresentationringRGofthenitegroupGisthefreeabeliangroupbasedonthesetofisomorphismclassesofirreduciblerepresentationsofG,withmultiplicationreectingthetensorproductdecompositionofirreducibles:[V]·[W]=knk·[Vk]iVW=kVnkk,wherewewrite[V]fortheconjugacyclassofanirreduciblerepresentationV,andthetensorproductVWhasbeendecomposedintoirreduciblesVk,withmultiplicitiesnk.
Thusdened,therepresentationringisassociativeandcommutative,withidentitythetrivial1-dimensionalrepresentation:[C]·[W]=[W]foranyW,becauseCW=W.
Example:G=Cn,thecyclicgroupofordernOurirreduciblesareL0,Ln1,wherethegeneratorofCnactsonLkasexp2πikn.
WehaveLpLq=Lp+q,subscriptsbeingtakenmodulon.
ItfollowsthatRCn=Z[X]/(Xn1),identifyingLkwithXk.
Theprofessionalrestatementofproperty(4)inTheorem8.
4isthefollowing.
8.
8Proposition.
ThecharacterisaringhomomorphismfromRGtotheclassfunctionsonG.
IttakestheinvolutionVVtocomplexconjugation.
Later,weshallreturntothishomomorphismandlistsomeothergoodproperties.
(8.
9)OrthogonalityofcharactersFortwocomplexfunctions,ψonG,welet|ψ:=1|G|g∈G(g)·ψ(g).
Inparticular,thisdenesaninnerproductonclassfunctions,ψ,wherewesumoverconjugacyclassesCG:|ψ=1|G||C|·(C)ψ(C).
Wearenowreadyfortherstpartofourmaintheorem.
Therewillbeacomplementinthenextlecture.
4Foranalternativeargument,recallthattheeigenvaluesofρ(g)arerootsofunity,andthoseofρ(g)1willbetheirinverses;butthesearealsotheirconjugates.
Recallnowthatthetraceisthesumoftheeigenvalues.
188.
10Theorem(Orthogonalityofcharacters).
1.
IfVisirreducible,thenχV2=1.
2.
IfV,Wareirreducibleandnotisomorphic,thenχV|χW=0.
Beforeprovingthetheorem,letuslistsomeconsequencestoillustrateitspower.
8.
11Corollary.
ThenumberoftimesanirreduciblerepresentationVappearsinanirreducibledecompositionofsomeWisχV|χW.
8.
12Corollary.
Theabovenumber(calledthemultiplicityofVinW)isindependentoftheirreducibledecompositionWehadalreadyprovedthisinLecture4,butwenowhaveasecondproof.
8.
13Corollary.
Tworepresentationsareisomorphicitheyhavethesamecharacter.
(Usecompletereducibilityandtherstcorollaryabove).
8.
14Corollary.
ThemultiplicityofthetrivialrepresentationinWis1|G|g∈GχW(g).
8.
15Corollary(Irreducibilitycriterion).
VisirreducibleiχV2=1.
Proof.
DecomposeVintoirreduciblesaskVnkk;then,χV=knk·χk,andχV2=kn2k.
SoχV2=1iallthenk'svanishbutone,whosevaluemustbe1.
Inpreparationfortheproofoforthogonality,weestablishthefollowingLemma.
FortworepresentationsV,WofGandanylinearmapφ:V→W,deneφ0=1|G|g∈GρW(g)φρV(g)1.
8.
16Lemma.
1.
φ0intertwinestheactionsG.
2.
IfVandWareirreducibleandnotisomorphic,thenφ0=0.
3.
IfV=WandρV=ρW,thenφ0=TrφdimV·Id.
Proof.
Forpart(1),wejustexaminetheresultofconjugatingbyh∈G:ρW(h)φ0ρV(h)1=1|G|g∈GρW(h)ρW(g)φρV(g)1ρV(h)1=1|G|g∈GρW(hg)φρV(hg)1=1|G|g∈GρW(g)φρV(g)1=φ0.
Part(2)nowfollowsfromSchur'slemma.
Forpart(3),Schur'slemmaagaintellsusthatφ0isascalar;tondit,itsucestotakethetraceoverV:then,φ0=Tr(φ0)/dimV.
ButwehaveTr(φ0)=1|G|g∈GTrρV(g)φρV(g)1=Tr(φ),asclaimed.
19Proofoforthogonality.
ChooseinvariantinnerproductsonV,Wandorthonormalbases{vi}and{wj}forthetwospaces.
WhenV=Wweshallusethesameinnerproductandbasisinboth.
Weshallusethe"bra·ket"vectornotationexplainedinthehandout.
ThenwehaveχW|χV=1|G|g∈GχW(g)χV(g)=1|G|g∈GχW(g1)χV(g)=1|G|i,jg∈Gwi|ρW(g)1|wivj|ρV(g)|vj.
Wenowinterpreteachsummandasfollows.
Recallrstthat|wivj|designatesthelinearmapV→Wwhichsendsavectorvtothevectorwi·vj|v.
Theproductwi|ρW(g)1|wivj|ρV(g)|vjistheninterpretableastheresultofapplyingthelinearmapρW(g)1|wivj|ρV(g)tothevector|vj,andthentakingdotproductwithwj.
Fixnowiandjandsumoverg∈G.
Lemma8.
16thenshowsthatthesumoflinearmapsρW(g)1|wivj|ρV(g)=0ifVW,Tr(|wivj|)/dimVifρV=ρW.
(8.
17)Intherstcase,summingoveri,jstillleadstozero,andwehaveprovedthatχV⊥χW.
Inthesecondcase,Tr(|wivj|)=1ifi=j,0otherwise,andsummingoveri,jleadstoafactorofdimV,cancellingthedenominatorinthesecondlineof(8.
17)andcompletingourproof.
8.
18Remark.
Theinnerproductofcharacterscanbedenedoveranygroundeldkofcharac-teristicnotdividing|G|,byusingχ(g1)inlieuofχ(g).
WehaveusedSchur'sLemmaoverCinestablishingPart(3)ofLemma8.
16,althoughnotforParts1and2.
Hence,theorthogonal-ityofcharactersofnon-isomorphicirreduciblesholdsoveranysucheldk,butorthonormalityχ2=1canfailifkisnotalgebraicallyclosed.
Thevalueofthesquaredependsonthedecompositionoftherepresentationinthealgebraicclosurek.
ThiscanbedeterminedfromthedivisionalgebraDV=EndGk(V)andtheGaloistheoryofitscentre,whichwillbeaniteextensioneldofk.
See,forinstance,Serre'sbookformoredetail.
(8.
19)TherepresentationringagainWedenedtherepresentationringRGofGasthefreeabeliangroupbasedontheisomorphismclassesofirreduciblerepresentationsofG.
Usingcompletereducibility,wecanidentifythelinearcombinationsofthesebasiselementswithnon-negativecoecientswithisomorphismclassesofG-representations:wesimplysendarepresentationiVnii,decomposedintoirreducibles,tothelinearcombinationini[Vi]∈RG(thebracketdenotestheisomorphismclass).
GeneralelementsofRGaresometimescalledvirtualrepresentationsofG.
WedenedthemultiplicationinRGusingthetensorproductofrepresentations.
Wesometimesdenotetheproductby,torememberitsorigin.
Theone-dimensionaltrivialrepresentation1istheunit.
WethendenedthecharacterχVofarepresentationρonVtobethecomplex-valuedfunctiononGwithχV(g)=TrVρ(g),theoperatortraceintherepresentationρ.
Thisfunctionisconjugation-invariant,oraclassfunctiononG.
WedenotethespaceofcomplexclassfunctionsonGbyC[G]G.
Bylinearity,χbecomesamapχ:RG→C[G]G.
WethencheckedthatχVW=χV+χW,χVW=χV·χW.
Thus,χ:RG→C[G]Gisaringhomomorphism.
Dualitycorrespondstocomplexconjugation:χ(V)=χV.
20ThelinearfunctionalInv:RG→Z,sendingavirtualrepresentationtothemultiplicity(=coecient)ofthetrivialrepresentation1correspondstoaveragingoverG:Inv(V)=1|G|g∈GχV(g).
WecandeneapositivedeniteinnerproductonRGbyV|W:=Inv(VW).
Thiscorrespondstotheobviousinnerproductoncharacters,1|G|g∈GχV(g)χW(g).
8.
20Remark.
ThestructuresdetailedaboveforRGisthatofaFrobeniusringwithinvolution.
(Inaringwithoutinvolution,wewouldjustrequirethatthebilinearpairingV*W→Inv(VW)shouldbenon-degenerate).
Similarly,C[G]GisacomplexFrobeniusalgebrawithinvolution,andwearesayingthatthecharacterχisahomomorphismofsuchstructures.
Itfailstobeanisomorphism"onlyintheobviousway":thetworingsshareabasisofirreduciblerepresentations(resp.
characters),buttheRGcoecientsareintegral,notcomplex.
9TheRegularRepresentationRecallthattheregularrepresentationofGhasbasis{eg},labelledbyg∈G;weletGpermutethebasisvectorsaccordingtoitsleftaction,λ(h)(eg)=ehg.
Thisdenesalinearactiononthespanoftheeg.
WecanidentifythespanoftheegwiththespaceC[G]ofcomplexfunctionsonG,bymatchingegwiththefunctionsendinggto1∈Candallothergroupelementstozero.
Underthiscorrespondence,elementsh∈Gact("ontheleft")onC[G],bysendingthefunctiontothefunctionλ(h)()(g):=(h1g).
Wecanseethatthecharacteroftheregularrepresentationisχreg(g)=|G|ifg=10otherwiseinparticular,χreg2=|G|2/|G|=|G|,sothisisfarfromirreducible.
Indeed,everyirreduciblerepresentationappearsintheregularrepresentation,asthefollowingpropositionshows.
9.
1Proposition.
Themultiplicityofanyirreduciblerepresentationintheregularrepresentationequalsitsdimension.
Proof.
χV|χreg=1|G|χV(1)χreg(1)=χV(1)=dimV.
Forinstance,thetrivialrepresentationappearsexactlyonce,whichwecanalsoconrmdirectly:theonlytranslation-invariantfunctionsonGareconstant.
9.
2Proposition.
Vdim2V=|G|,thesumrangingoverallirreducibleisomorphismclasses.
Proof.
Fromχreg=dimV·χVwegetχreg2=dim2V,asdesired.
Wenowproceedtocompleteourmainorthogonalitytheorem.
9.
3Theorem(Completenessofcharacters).
Theirreduciblecharactersformabasisforthespaceofcomplexclassfunctions.
Thus,theyformanorthonormalbasisforthatspace.
Theproofconsistsinshowingthatifaclassfunctionisorthogonaltoeveryirreduciblecharacter,thenitsvalueateverygroupelementiszero.
Forthis,weneedthefollowing.
21Construction.
Toeveryfunction:G→C,andtoeveryrepresentation(V,ρ)ofG,weassignthefollowinglinearself-mapρ()ofV:ρ()=g∈G(g)ρ(g).
Wenote,byapplyingρ(h)(h∈G)andrelabellingthesum,thatρ(h)ρ()=ρ(λ(h)).
9.
4Lemma.
isaclassfunctioniρ()commuteswithG,ineveryrepresentationV.
Proof.
Ifisaclassfunction,then(h1gh)=(g)forallg,handsoρ(h)ρ()ρ(h1)=g∈G(g)ρ(hgh1)=k∈G(h1kh)ρ(k)=k∈G(k)ρ(k).
Intheotherdirection,letVbetheregularrepresentation;thenρ()(e1)=g∈G(g)eg,andλ(h)ρ()(e1)=g∈G(g)ehg=g∈G(h1g)eg,whereasρ()λ(h)(e1)=ρ()(eh)=g∈G(g)egh=g∈G(gh1)eg;equatingthetwoshowsthat(h1g)=(gh1),asclaimed.
WhenisaclassfunctionandVisirreducible,Schur'sLemmaandthepreviousresultshowthatρ()isascalar.
Tondit,wecomputethetrace:Tr[ρ()]=g∈G(g)χV(g)=|G|·χV|(recallthatχV=χV).
Weobtainthat,whenVisirreducible,ρ()=|G|dimV·χV|·Id.
(9.
5)ProofofCompleteness.
Assumethattheclassfunctionisorthogonaltoallirreduciblechar-acters.
By(9.
5),ρ()=0ineveryirreduciblerepresentationV,hence(bycompletereducibil-ity)alsointheregularrepresentation.
But,aswesawintheproofofLemma9.
4,wehaveρ()(e1)=g∈G(g)egintheregularrepresentation;so=0,asdesired.
(9.
6)TheGroupalgebraWenowstudytheregularrepresentationingreaterdepth.
DeneamultiplicationonC[G]bysettingeg·eh=eghonthebasiselementsandextendingbylinearity:ggeg·hψheh=g,hgψhegh=ghgh1ψheg.
Thisisassociativeanddistributiveforaddition,butitisnotcommutativeunlessGwasso.
(Associativityfollowsfromthesamepropertyinthegroup.
)Itcontainse1asthemultiplicativeidentity,andthecopyCe1ofCcommuteswitheverything.
ThismakesC[G]intoaC-algebra.
ThegroupGembedsintothegroupofmultiplicativelyinvertibleelementsofC[G]byg→eg.
Forthisreason,wewilloftenreplaceegbyginournotation,andthinkofelementsofC[G]aslinearcombinationsofgroupelements,withtheobviousmultiplication.
229.
7Proposition.
ThereisanaturalbijectionbetweenmodulesoverC[G]andcomplexrepre-sentationsofG.
Proof.
Oneachrepresentation(V,ρ)ofG,weletthegroupalgebraactintheobviousway,ρgg·g(v)=ggρ(g)(v).
Conversely,givenaC[G]-moduleM,theactionofCe1makesitintoacomplexvectorspaceandagroupactionisdenedbyembeddingGinsideC[G]asexplainedabove.
Wecanreformulatethediscussionabove(perhapsmoreobscurely)bysayingthatagrouphomomorphismρ:G→GL(V)extendsnaturallytoanalgebrahomomorphismfromC[G]toEnd(V);VisamoduleoverEnd(V)andtheextendedhomomorphismmakesitintoaC[G]-module.
Conversely,anysuchhomomorphismdenesbyrestrictionarepresentationofG,fromwhichtheoriginalmapcanthenberecoveredbylinearity.
Choosingacompletelist(uptoisomorphism)ofirreduciblerepresentationsVofGgivesahomomorphismofalgebras,ρV:C[G]→VEnd(V),→(ρV()).
(9.
8)NoweachspaceEnd(V)carriestwocommutingactionsofG:theseareleftcompositionwithρV(g),andrightcompositionwithρV(g)1.
Foranalternativerealisation,End(V)isisomorphictoVVandGactsseparatelyonthetwofactors.
TherearealsotwocommutingactionsofGonC[G],bymultiplicationontheleftandontheright,respectively:(λ(h))(g)=(h1g),(ρ(h))(g)=(gh).
ItisclearfromourconstructionthatthetwoactionsofG*Gontheleftandrightsidesof(9.
8)correspond,henceouralgebrahomomorphismisalsoamapofG*G-representations.
ThemainresultofthissubsectionisthefollowingmuchmorepreciseversionofProposition9.
1.
9.
9Theorem.
Thehomomorphism(9.
8)isanisomorphismofalgebras,andhenceofG*G-representations.
Inparticular,wehaveanisomorphismofG*G-representations,C[G]=VVV,withthesumrangingovertheirreduciblerepresentationsofG.
Fortheproof,werequirethefollowing9.
10Lemma.
LetV,WbecomplexirreduciblerepresentationsofthenitegroupsGandH.
Then,VWisanirreduciblerepresentationofG*H.
Moreover,everyirrepofG*Hisofthatform.
Thereadershouldbecautionedthatthestatementcanfailiftheeldisnotalgebraicallyclosed.
Indeed,theproofusestheorthonormalityofcharacters.
Proof.
Directcalculationofthesquarenormshowsthatthecharacterχv(g)*χW(h)ofG*Hisirreducible.
(Homework:dothis!
).
Moreover,IclaimthatthesecharactersspantheclassfunctionsonG*H.
Indeed,conjugacyclassesinG*HareCartesianproductsofclassesinthefactors,andwecanwriteeveryindicatorclassfunctioninGandHasalinearcombinationofcharacters;sowecanwriteeveryindicatorclassfunctiononG*HasalinearcombinationoftheχV*χW.
23Inparticular,itfollowsthattherepresentationVVofG*Gisirreducible.
Moreover,notwosuchrepsfordistinctVcanbeisomorphic;indeed,onpairs(g,1)∈G*G,thecharacterreducestothatofV.
ProofofTheorem9.
8.
Bydimension-count,itsucestoshowthatourmapρVissurjective.
TheG*G-structurewillbeessentialforthis.
NotefromourLemmathatthedecompositionS:=VVVsplitsthesumSintopairwisenon-isomorphic,irreduciblerepresentations.
FromtheresultsinLecture4,weknowthatanyG*G-mapintoSmustbeblock-diagonalfortheisotypicaldecompositions.
Inparticular,ifamapsurjectsontoeachfactorseparately,itsurjectsontothesumS.
Sincethesummandsareirreducible,itsucesthentoshowthateachprojectiontoEnd(V)isnon-zero.
Butthegroupidentitye1mapstotheidentityineachEnd(V).
9.
11Remark.
(i)ItcanbeshownthattheinversemaptoρVassignstoφ∈End(V)thefunctiong→TrV(ρV(g)φ)/dimV.
(OptionalHomework.
)(ii)Schur'sLemmaensuresthattheinvariantsontheright-handsidefortheactionofthediagonalcopyGG*Garethemultiplesoftheidentityineachsummand.
Ontheleftside,wegettheconjugation-invariantfunctions,orclassfunctions.
10ThecharactertableInviewofourtheoremaboutcompletenessandorthogonalityofcharacters,thegoalofcomplexrepresentationtheoryforanitegroupistoproducethecharactertable.
Thisisthesquarematrixwhoserowsarelabelledbytheirreduciblecharacters,andwhosecolumnsarelabelledbytheconjugacyclassesinthegroup.
Theentriesinthetablearethevaluesofthecharacters.
Arepresentationcanberegardedas"known"assoonasitscharacterisknown.
Indeed,onecanextracttheirreduciblemultiplicitiesfromthedotproductswiththerowsofthechar-actertable.
Youwillseeinthehomework(Q.
2.
5)thatyoucanevenconstructtheisotypicaldecompositionofyourrepresentation,oncetheirreduciblecharactersareknown.
Orthonormalityofcharactersimpliesacertainorthonormalityoftherowsofthecharactertable,whenviewedasamatrixA.
"Acertain"reectstothefactthattheinnerproductbetweencharactersisnotthedotproductoftherows;rather,thecolumnofaclassCmustbeweighedbyafactor|C|/|G|inthedotproduct(asthesumgoesovergroupelementsandnotconjugacyclasses).
Inotherwords,ifBisthematrixobtainedfromAbymultiplyingeachcolumnby|C|/|G|,thentheorthonormalityrelationsimplythatBisaunitarymatrix.
Orthonormalityofitscolumnsleadstothe10.
1Proposition(Thesecondorthogonalityrelations).
ForanyconjugacyclassesC,C,wehave,summingovertheirreduciblecharactersχofG,χχ(C)·χ(C)=|G|/|C|ifC=C,0otherwise.
10.
2Remark.
Theorbit-stabilisertheorem,appliedtotheconjugationactionofGonitselfandsomeelementc∈C,showsthattheprefactor|G|/|C|istheorderofZG(c),thecentraliserofcinG(thesubgroupofG-elementscommutingwithc).
Asareformulation,wecanexpresstheindicatorfunctionECofaconjugacyclassCGintermsofcharacters:EC(g)=|C||G|χχ(C)·χ(g).
Notethatthecoecientsneednotbeintegral,sotheindicatorfunctionofaconjugacyclassisnotthecharacterofarepresentation.
24(10.
3)Example:ThecyclicgroupCnofordernCallgagenerator,andletLkbetheone-dimensionalrepresentationwheregactsasωk,whereω=exp2πin.
ThenweknowthatL0,Ln1arealltheirreduciblesandthecharactertableisthefollowing:{1}{g}gq}.
.
.
111.
.
.
1.
.
.
L01ω.
.
.
ωq.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
Lp1ωp.
.
.
ωpq.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
MovingontoD6,forwhichwehadfoundtheone-dimensionalirreducibles1,Sandthe2-dimensionalirreducibleV,wenotethat1+1+22=6sotherearenootherirreducibles.
Wethusexpect3conjugacyclassesand,indeed,wend{1},{g,g2},{r,rg,rg2},thethreereectionsbeingallconjugate(g1rg=rg2,etc).
Fromourmatrixconstructionoftherepresentationwellintheentriesofthecharactertable:{1}{g,g2}{rg,.
.
.
}1111S111V210Letuscheckoneoftheorthogonalityrelations:χ2V=(22+12)/6=5/6,whichdoesnotmatchthetheoremverywell;thatis,ofcourse,becauseweforgottoweightheconjugacyclassesbytheirsize.
Itishelpfultomakeanoteoftheweightssomewhereinthecharactertable.
Thecorrectcomputationisχ2V=(22+2·12)/6=1.
ThegeneraldihedralgroupD2nworksverysimilarly,butthereisadistinction,accordingtotheparityofn.
Letusrsttaken=2m+1,odd,andletω=exp(2πi/n).
Wecanagainseethetrivialandsignrepresentations1andS;additionally,wehavemtwo-dimensionalrepresentationsVk,labelledby0xvmus=0,wherexmodpisaxed,non-zeroresidueandmandsinthesumrangeoverallpossiblechoices.
12ThealternatinggroupA5InthislectureweconstructthecharactertableofthealternatinggroupA5ofevenpermutationsonveletters.
Unlikethepreviousexamples,wewillnowexploitthepropertiesofcharactersinndingrepresentationsandprovingtheirirreducibility.
Westartwiththeconjugacyclasses.
Recallthateverypermutationisaproductofdisjointcycles,uniquelyuptotheirorder,andthattwopermutationsareconjugateinthesymmetric27groupitheyhavethesamecycletype,meaningthecyclelengths(withtheirmultiplicities).
Thus,acompletesetofrepresentativesfortheconjugacyclassesinS5isId,(12),(12)(34),(123),(123)(45),(1234),(12345),sevenintotal.
Ofthese,onlyId,(12)(34),(123)and(12345)areinA5.
Now,aconjugacyclassinS5mayormaynotbreakupintoseveralclassesinA5.
ThereasonisthatwemayormaynotbeabletorelatetwogivenpermutationsinA5whichareconjugateinA5byanevenpermutation.
Nowtherstthreepermutationsinourlistallcommutewithsometransposition.
ThisensuresthatwecanimplementtheeectofanyconjugationinS5byaconjugationinA5:conjugatebytheextratranspositionifnecessary.
Wedonothavesuchanargumentforthe5-cycle,sothereisthepossibilitythatthecycles(12345)and(21345)arenotconjugateinA5.
Tomakesure,wecountthesizeoftheconjugacyclassbytheorbit-stabilisertheorem.
Thesizeoftheconjugacyclasscontainingg∈Gis|G|dividedbytheorderofthecentraliserofg.
Thecentraliserof(12345)inS5isthecyclicsubgroupoforder5generatedbythiscycle,andisthereforethesameasthecentraliserinA5.
SotheorderoftheconjugacyclassinS5is24,butinA5only12.
Sooursuspicionsareconrmed,andtherearetwoclassesof5-cyclesinA5.
12.
1Remark.
Thesameorbit-stabiliserargumentshowsthattheconjugacyclassinSnofanevenpermutationσisasingleconjugacyclassinAnpreciselywhenσcommuteswithsomeoddpermutation;else,itbreaksupintotwoclassesofequalsize.
Foruseincomputations,wenotethattheordersoftheconjugacyclassesare1forId,15for(12)(34),20for(123)and12eachfor(12345)and(21345).
Wehave,asweshould,1+15+20+12+12=60=|A5|.
Wenowstartproducingtherowsofthecharactertable,listingtheconjugacyclassesintheorderabove.
Thetrivialrep1givestherow1,1,1,1,1.
Next,weobservethatA5actsnaturallyonC5bypermutingthebasisvectors.
Thisrepresentationisnotirreducible;indeed,thelinespannedbythevectore1e5isinvariant.
ThecharacterχC5is5,1,2,0,0anditsdotproductχC5|χ1=5/60+1/4+2/3=1withthetrivialrepshowsthat1appearsinC5withmultiplicityone.
ThecomplementVhascharacterχV=4,0,1,1,1,whosesquarenormisχV2=1660+13+15+15=1,showingthatVisirreducible.
Weseekmorerepresentations,andconsiderforthatthetensorsquareV2.
Wealreadyknowthisisreducible,becauseitsplitsasSym2VΛ2V,butwetryextractirreduciblecomponents.
ForthisweneedthecharacterformulaeforSym2VandΛ2V.
12.
2Proposition.
Foranyg∈G,χSym2V(g)=χV(g)2+χV(g2)/2,χΛ2V(g)=χV(g)2χV(g2)/2.
Proof.
Havingxedg,wechooseabasisofVonwhichgactsdiagonally,witheigenvaluesλ1,λd,repeatedasnecessary.
FromourbasesofSym2V,Λ2V,weseethattheeigenvaluesofgonthetwospacesareλpλq,with1≤p≤q≤donSym2andwith1≤p1).
ProofoftheLemma.
Ifq∈U(1)isnotarootofunity,thenitspowersaredenseinU(1)(exercise,usingthepigeonholeprinciple).
So,anyclosed,propersubgroupofU(1)consistsonlyofrootsof1.
Amongthose,theremustbeoneofsmallestargument(inabsolutevalue)orelsetherewouldbeasequenceconvergingto1;thesewouldagaingenerateadensesubgroupofU(1).
Therootofunityofsmallestargumentisthenthegenerator.
ProofofTheorem19.
10.
Acontinuousmap:U(1)→C*hascompact,henceboundedimage.
Theimagemustlieontheunitcircle,becausetheintegralpowersofanyothercomplexnumberformanunboundedsequence.
SoisacontinuoushomomorphismfromU(1)itself.
Now,kerisaclosedsubgroupofU(1).
Ifkeristheentiregroup,then=1andthetheoremholdswithn=0.
Ifker=n(n≥1),wewillnowshowthat(z)=z±n,withthesamechoiceofsignforallz.
Toseethis,deneacontinuousfunctionψ:[0,2π/n]→R,ψ(0)=0,ψ(θ)=arg(eiθ);inotherwords,weparametriseU(1)bytheargumentθ,startwithψ(0)=0,whichisonevalueoftheargumentof(1)=1,choosetheargumentsoastomakethefunctioncontinuous.
Becauseker=n,ψmustbeinjectiveon[0,2π/n).
Bycontinuity,itmustbemonotonicallyincreasingordecreasing(IntermediateValueTheorem),andwemusthaveψ(2π/n)=±2π:thevaluezeroisruledoutbymonotonicityandanyothermultipleof2πwouldleadtoanintermediatevalueofθwith(eiθ)=1.
Henceforth,±denotesthesignofψ(2π/n).
Becauseisahomomorphismand(e2πi/n)=1,(e2πi/mn)mustbeanmthrootofunity,andsoψ({2πk/mn}){±2πk/m},k=0,m.
Bymonotonicity,thesem+1valuesmustbetakenexactlyonceandinthenaturalorder,soψ(2πk/mn)=±2πk/m,forallmandallk=0,m.
Butthen,ψ(θ)=±n·θ,bycontinuity,and(z)=z±n,asclaimed.
19.
12Remark.
Completereducibilitynowshowsthatcontinuousnite-dimensionalrepresenta-tionsofU(1)areinfactalgebraic:thatis,theentriesinamatrixrepresentationsareLaurentpolynomialsinz.
Thisisnotanaccident;itholdsforalargeclassoftopologicalgroups(thecompactLiegroups).
45(19.
13)CharactertheoryThehomomorphismsρn:U(1)→C*,ρn(z)=znformthecompletelistofirreduciblerepre-sentationsofU(1).
Clearly,theircharacters(whichweabusivelydenotebythesamesymbol)arelinearlyindependent;infact,theyareorthonormalintheinnerproduct|ψ=12π2π0(θ)ψ(θ)dθ,whichcorrespondsto"averagingoverU(1)"(z=eiθ).
ThefunctionsρnaresometimescalledFouriermodes,andtheirnitelinearcombinationsaretheFourierpolynomials.
AsU(1)isabelian,itcoincideswiththespaceofitsconjugacyclasses.
Wecannowstateourmaintheorem.
19.
14Theorem.
(i)ThefunctionsρnformacompletelistofirreduciblecharactersofU(1).
(ii)Everynite-dimensionalrepresentationVofU(1)isisomorphictoasumoftheρn.
ItscharacterχVisaFourierpolynomial.
ThemultiplicityofρninVistheinnerproductρn|χV.
RecallthatcompletereducibilityofrepresentationsfollowsbyWeyl'sunitarytrick,averaginganygiveninnerproductbyintegrationonU(1).
Asthespaceof(continuous)classfunctionsisinnite-dimensional,itrequiresabitofcaretostatethenalpartofourmaintheorem,thatthecharactersforma"basis"ofthespaceofclassfunctions.
ThegoodsettingforthatistheHilbertspaceofsquare-integrablefunctions,whichwediscussbelow.
Fornow,letusjustnoteanalgebraicversionoftheresult.
19.
15Proposition.
TheρnformabasisofthepolynomialfunctionsonU(1)R2.
Indeed,ontheunitcircle,z=z1sothetheFourierpolynomialsarealsothepolynomialsinzandz,whichcanalsobeexpressedaspolynomialsinx,y.
(19.
16)Digression:FourierseriesTheFouriermodesρnformacompleteorthonormalset(orthonormalbasis)oftheHilbertspaceL2(U(1)).
Thismeansthateverysquare-integrablefunctionf∈L2hasa(Fourier)seriesexpansionf(θ)=∞∞fn·einθ=∞∞fn·ρn,(*)whichconvergesinmeansquare;theFouriercoecientsfnaregivenbytheformulafn=ρn|f=12π2π0einθf(θ)dθ.
(**)Recallthatmean-squareconvergencesigniesthatthepartialsumsapproachf,inthedistancedenedbytheinnerproduct.
Theproofofmostofthisisfairlyeasy.
OrthonormalityoftheρnimpliestheFourierexpansionformulafortheFourierpolynomials,thenitelinearcombinationsoftheρn.
(Ofcourse,inthiscasetheFourierseriesisanitesum,andnoanalysisisneeded.
)Furthermore,foranygivennitecollectionSofindexesn,andforanyf∈L2,thesumoftermsin(*)withn∈Sistheorthogonalprojectionoffontothethespanoftheρn,n∈S.
Hence,anynitesumofnormsoftheFouriercoecientsfn2in(*)isboundedbyf2,andtheFourierseriesconverges.
Moreover,thelimitghasthepropertythatfgisorthogonaltoeachρn;inotherwords,gistheprojectionoffontothespanofalltheρn.
Themoredicultpartistoshowthatanyf∈L2whichisorthogonaltoallρnvanishes.
OnemethodistoderiveitfromapowerfultheoremofWeierstra',whichsaysthattheFourierpolynomialsaredenseinthespaceofcontinuousfunctions,inthesenseofuniformconvergence.
46(TheresultholdsforcontinuousfunctionsonanycompactsubsetofRN;here,N=2.
)Approx-imatingacandidatef,orthogonaltoallρn,byasequenceofFourierpolynomialspkleadstoacontradiction,becausefpk2=f2+pk2≥f2,byorthogonality,andyetuniformconvergencecertainlyimpliesconvergenceinmean-square.
Wesummarisethebasicfactsinthefollowing19.
17Theorem.
(i)AnycontinuousfunctiononU(1)canbeuniformlyapproximatedbynitelinearcombinationsoftheρn.
(ii)Anysquare-integrablefunctionf∈L2(U(1))hasaseriesexpansionf=fn·ρn,withFouriercoecientsfngivenby(**).
20ThegroupSU(2)WemoveontothegroupSU(2).
Wewilldescribeitsconjugacyclasses,ndthebi-invariantvolumeform,whoseexistenceimpliestheunitarisabilityandcompletereducibilityofitscon-tinuousnite-dimensionalrepresentations.
Inthenextlecture,wewilllisttheirreducibleswiththeircharacters.
Givingawaytheplot,observethatSU(2)actsonthespacePofpolynomialsintwovariablesz1,z2,byitsnaturallinearactiononthecoordinates.
WecandecomposePintothehomogeneouspiecesPn,n=0,1,2,preservedbytheactionofSU(2):thus,thespaceP0ofconstantsisthetrivialone-dimensionalrepresentation,thespaceP1oflinearfunctionsthestandard2-dimensionalone,etc.
Then,P0,P1,P2,.
.
.
isthecompletelistofirreduciblerepresentationsofSU(2),uptoisomorphism,andeverycontinuousnite-dimensionalrepresentationisisomorphictoadirectsumofthose.
(20.
1)SU(2)andthequaternionsBydenition,SU(2)isthegroupofcomplex2*2matricespreservingthecomplexinnerproductandwithdeterminant1;thegroupoperationismatrixmultiplication.
Explicitly,SU(2)=uvvu:u,v∈C,|u|2+|v|2=1.
Geometrically,thiscanbeidentiedwiththethree-dimensionalunitsphereinC2.
ItisusefultoreplaceC2withHamilton'squaternionsH,generatedoverRbyelementsi,jsatisfyingtherelationsi2=j2=1,ij=ji.
Thus,Hisfour-dimensional,spannedby1,i,jandk:=ij.
Theconjugateofaquaternionq=a+bi+cj+dkisq:=abicjdk,andthequaternionnormisq2=qq=qq=a2+b2+c2+d2.
Therelationq1q2=q2q1showsthat:H→Rismultiplicative,q1q2=q1q2·q1q2=q1q2·q2q1=q1q2inparticular,the"unitquaternions"(thequaternionsofunitnorm)formagroupundermulti-plication.
Directcalculationestablishesthefollowing.
20.
2Proposition.
Sendinguvvutoq=u+vjgivesanisomorphismofSU(2)withthemultiplicativegroupofunitquaternions.
47(20.
3)ConjugacyclassesAtheoremfromlinearalgebraassertsthatunitarymatricesarediagonalisableinanorthonormaleigenbasis.
Thediagonalisedmatrixisthenalsounitary,soitsdiagonalelementsarecomplexnumbersofunitnorm.
Thesenumbers,ofcourse,aretheeigenvaluesofthematrix.
Theyareonlydetermineduptoreordering.
WethereforegetabijectionofconjugacyclassesintheunitarygroupwithunorderedN-tuplesofcomplexnumbersofunitnorm:U(N)/U(N)U(1)N/SN,wherethesymmetricgroupSNactsbypermutingtheNfactorsofU(1)N.
ThemapfromrighttoleftisdenedbytheinclusionU(1)NU(N)andisthereforecontinuous;ageneraltheoremfromtopologyensuresthatitisinfactahomeomorphism.
7Clearly,restrictingtoSU(N)imposedthedet=1restrictionontherightsideU(1)N.
However,thereisapotentialprobleminsight,becauseitmighthappen,inprinciple,thattwomatricesinSU(N)willbeconjugateinU(N),butnotinSU(N).
ForageneralsituationofasubgroupHGthiswarrantsdeservescarefulconsideration.
Inthecaseathand,however,wearelucky.
ThescalarmatricesU(1)·IdU(N)arecentral,thatis,theirconjugationactionistrivial.
Now,foranymatrixA∈U(N)andanyNthrootrofdetA,wehaver1A∈SU(N),andconjugatingbythelatterhasthesameeectasconjugationbyA.
WethengetabijectionSU(N)/SU(N)SU(1)N/SN,whereU(1)NisidentiedwiththegroupofdiagonalunitaryN*NmatricesandtheSontheright,standingfor"special",indicatesitssubgroupofdeterminant1matrices.
20.
4Proposition.
Thenormalisedtrace12Tr:SU(2)→CgivesahomomorphismsofthesetofconjugacyclassesinSU(2)withtheinterval[1,1].
Thesetofconjugacyclassesisgiventhequotienttopology.
Proof.
Asdiscussed,matricesareconjugateinSU(2)itheireigenvaluesagreeuptoorder.
Theeigenvaluesformapair{z,z1},withzontheunitcircle,sotheyaretherootsofX2(z+z1)X+1,inwhichthemiddlecoecientrangesover[2,2].
Thequaternionpictureprovidesagoodgeometricmodelofthismap:thehalf-tracebecomestheprojectionq→aontherealaxisRH.
Theconjugacyclassesarethereforethe2-dimensionalspheresofconstantlatitudeontheunitsphere,plusthetwopoles,thesingletons{±1}.
Thelattercorrespondtothecentralelements±I2∈SU(2).
(20.
5)CharactersasLaurentpolynomialsTheprecedingdiscussionimpliesthatthecharactersofcontinuousSU(2)-representationsarecontinuousfunctionson[1,1].
Itwillbemoreprotable,however,toparametrisethatintervalbythe"latitude"φ∈[0,π],ratherthantherealpartcosφ.
Wecaninfactletφrangefrom[0,2π],providewerememberourfunctionsareinvariantunderφφandperiodicwithperiod2π.
WecallsuchfunctionsofφWeyl-invariant,oreven.
Functionswhichchangesignunderthatsamesymmetryareanti-invariantorodd.
7Weusethequotienttopologiesonbothsides.
TheresultIamalludingtoassertsthatacontinuousbijectionfromacompactspacetoaHausdorspaceisahomeomorphism.
48Noticethatφparametrisesthesubgroupofdiagonalmatrices,isomorphictoU(1),eiφ00eiφ=z00z1∈SU(2),andthetransformationφφisinducedbyconjugationbythematrix0ii0∈SU(2).
ThecharacterofanSU(2)-representationVwillbethefunctionofz∈U(1)χV(z)=TrVz00z1,invariantunderzz1.
Whenconvenient,weshallre-expressitintermsofφandabusivelywriteχV(φ).
ArepresentationofSU(2)restrictstooneofourU(1),andweknowfromlastlecturethatthecharactersofthelatterarepolynomialsinzandz1.
SuchfunctionsarecalledLaurentpolynomials.
Wethereforeobtainthe20.
6Proposition.
CharactersofSU(2)-representationsareevenLaurentpolynomialsinz.
(20.
7)ThevolumeformandWeyl'sIntegrationformulaforSU(2)Fornitegroups,averagingoverthegroupwasusedtoprovecompletereducibility,todenetheinnerproductofcharactersandprovetheorthogonalitytheorems.
Forcompactgroups(suchasU(1)),integrationoverthegroupmustbeusedinstead.
Inbothcases,theessentialpropertyoftheoperationisitsinvarianceunderleftandrighttranslationsonthegroup.
Tostatethismoreprecisely:thelinearfunctionalsendingacontinuousfunctiononGtoG(g)dgisinvariantunderleftandrighttranslationsof.
Asecondaryproperty(whichisarrangedbyappropriatescaling)isthattheaverageorintegraloftheconstantfunction1is1.
WethusneedavolumeformoverSU(2)whichisinvariantunderleftandrighttranslations.
OurgeometricmodelforSU(2)astheunitsphereinR4allowsustonditdirectly,withoutappealingtoHaar's(dicult)generalresult.
Note,indeed,thattheactionsofSU(2)onHbyleftandrightmultiplicationpreservethequaternionnorm,hencetheEuclideandistance.
Inparticular,theusualEuclideanvolumeelementontheunitspheremustbeinvariantunderbothleftandrightmultiplicationbySU(2)elements.
20.
8Remark.
Theright*leftactionofSU(2)*SU(2)onR4=H,wherebyα*βsendsq∈Htoαqβ1,givesahomomorphismh:SU(2)*SU(2)→SO(4).
(Theactionpreservesorientation,becausethegroupisconnected.
)Clearly,(Id,Id)actsastheidentity,sohfactorsthroughthequotientSU(2)*SU(2)/{±(Id,Id)},andindeedturnsouttogiveanisomorphismofthelatterwithSO(4).
WewilldiscussthisinLecture22.
Tounderstandtheinnerproductofcharacters,weareinterestedinintegratingclassfunctionsoverSU(2).
ThismustbeexpressibleintermsoftheirrestrictiontoU(1).
Thisismadeexplicitbythefollowingtheorem,whoseimportancecannotbeoverestimated:asweshallsee,itimpliesthecharacterformulaeforallirreduciblerepresentationsofSU(2).
LetdgbetheEuclideanvolumeformontheunitsphereinH,normalisedtototalvolume1,andlet(φ)=eiφeiφbetheWeyldenominator.
20.
9Theorem(WeylIntegrationFormula).
ForacontinuousclassfunctionfonSU(2),wehaveSU(2)f(g)dg=1212π2π0f(φ)·|(φ)|2dφ=1π2π0f(φ)sin2φdφ.
49Thus,theintegraloverSU(2)canbecomputedbyrestrictiontotheU(1)ofdiagonalmatrices,aftercorrectingthemeasurebythefactor12|(φ)|2.
Weareabusivelywritingf(φ)forthevalueoffateiφ00eiφ.
Proof.
InthepresentationofSU(2)astheunitsphereinH,thefunctionfbeingconstantonthespheresofconstantlatitude.
ThevolumeofasphericalsliceofwidthdφisC·sin2φdφ,withtheconstantCnormalisedbythe"totalvolume1"conditionπ0C·sin2φdφ=1,whenceC=2/π,inagreementwiththetheorem.
21IrreduciblecharactersofSU(2)Letuschecktheirreducibilityofsomerepresentations.
Thetrivial1-dimensionalrepresentationisobviouslyirreducible;itscharacterhasnorm1,duetoournormalisationofthemeasure.
ThecharacterofthestandardrepresentationonC2iseiφ+eiφ=2cosφ.
Itsnormis4π2π0cos2φ·sin2φdφ=1π2π0(2cosφsinφ)2dφ=1π2π0sin22φdφ=1,soC2isirreducible.
Ofcourse,thiscanalsobeseendirectly(noinvariantlines).
ThecharacterofthetensorsquareC2C2is4cos2φ,soitssquarenormis1π2π016cos4φsin2φdφ=1π2π04cos2φsin22φdφ=1π2π0(sin3φ+sinφ)2dφ=1π2π0sin23φ+sin2φ+2sinφsin3φdφ=1+1=2,sothisisreducible.
Indeed,wehave(C2)2=Sym2C2Λ2C2;thesemustbeirreducible.
Λ2C2isthetrivial1-dim.
representation,sinceitscharacteris1(theproductoftheeigen-values),andsoSym2C2isanew,3-dimensionalirreduciblerepresentation,withcharacter2cos2φ+1=z2+z2+1.
SofarwehavefoundtheirreduciblerepresentationsC=Sym0C2,C2=Sym1C2,Sym2C2,withcharacters1,z+z1,z2+1+z2.
Thissurelylendscredibilitytothefollowing.
21.
1Theorem.
ThecharacterχnofSymnC2iszn+zn2z2n+zn.
Itsnormis1,andsoeachSymnC2isanirreduciblerepresentationofSU(2).
Moreover,thisisthecompletelistofirreducibles,asn≥0.
21.
2Remark.
NotethatSymnC2hasdimensionn+1.
50Proof.
Thestandardbasisvectorse1,e2scaleunderg=z00z1bythefactorsz±1.
Now,thebasisvectorsofSymnC2arisebysymmetrisingthevectorsen1,e(n1)1e2,en2inthenthtensorpower(C2)n.
Thevectorsappearinginthesymmetrisationofep1e(np)2aretensorproductscontainingpfactorsofe1andnpfactorsofe2,insomeorder;eachoftheseisaneigenvectorsforg,witheigenvaluezp·znp.
Hence,theeigenvaluesofgonSymnare{zn,zn2,zn}andthecharacterformulafollows.
Wehaveχn(z)=zn+1zn1zz1,whenceχn(z)|2|(z)|2=|zn+1zn1|2=4sin2[(n+1)φ].
Thus,χn2=14π2π04sin2[(n+1)φ]dφ=1π·π=1,provingirreducibility.
Toseecompleteness,observethatthefunctions(z)χn(z)spanthevectorspaceofoddLaurentpolynomials(§20.
5).
Foranycharacterχ,thefunctionχ(z)(z)isanoddLaurentpolynomial.
Ifχwasanewirreduciblecharacter,χ(z)(z)wouldhavetobeorthogonaltoallanti-invariantLaurentpolynomials,inthestandardinnerproductonthecircle.
(Combinedwiththeweight||2,thisisthecorrectSU(2)innerproductuptoscale,aspertheintegrationformula).
Butthisisimpossible,astheorthogonalcomplementoftheoddpolynomialsisspannedbytheevenones.
(21.
3)AnotherlookattheirreduciblesThegroupSU(2)actslinearlyonthevectorspacePofpolynomialsinz1,z2bytransformingthevariablesinthestandardway,uvvu·z1z2=uz1+vz2vz1+uz2.
ThispreservesthedecompositionofPasthedirectsumofthespacesPnofhomogeneouspolynomialsofdegreen.
21.
4Proposition.
AsSU(2)-representations,SymnC2=Pn,thespaceofhomogeneouspoly-nomialsofdegreenintwovariables.
Proof.
AnisomorphismfromPntoSymnC2isdenedbysendingzp1znp2tothesymmetrisationofep1e(np)2.
Bylinearity,thiswillsend(uz1+vz2)p·(vz1+uz2)nptothesymmetrisationof(ue1+ve2)p(ve1+ue2)(np).
ThisshowsthatourisomorphismcommuteswiththeSU(2)-action.
21.
5Remark.
Equalityoftherepresentationsuptoisomorphismcanalsobeseenbycheckingthecharacterofadiagonalmatrixg,forwhichthezp1znp2areeigenvectors.
(21.
6)TensorproductofrepresentationsKnowledgeofthecharactersallowsustondthedecompositionoftensorproducts.
Obviously,tensoringwithCdoesnotchangearepresentation.
Thenextexampleisχ1·χn=(z+z1)zn+1zn1zz1=zn+2+znznz2nzz1=χn+1+χn1,providedn≥1;ifn=0ofcoursetheanswerisχ1.
Moregenerally,wehave5121.
7Theorem.
Ifp≥q,χp·χq=χp+q+χp+q2χpq.
Hence,SympC2SymqC2=qk=0Symp+q2kC2.
Proof.
χp(z)·χq(z)=zp+1zp1zz1·zq+zq2zq=qk=0zp+q+12kz2kpq1zz1=qk=0χp+q2k;theconditionp≥qensuresthattherearenocancellationsinthesum.
21.
8Remark.
Letusdeneformallyχn=χn2,a"virtualcharacter".
Thus,χ0=1,thetrivialcharacter,χ1=0andχ2=1.
Thedecompositionformulabecomesχp·χq=χp+q+χp+q2χpq,regardlesswhetherp≥qornot.
(21.
9)MultiplicitiesThemultiplicityoftheirreduciblerepresentationSymninarepresentationwithcharacterχ(z)canbecomputedastheinnerproductχ|χn,withtheintegration(20.
9).
Thefollowingrecipe,whichexploitsthesimpleformofthefunctions(z)χn(z)=zn+1zn1,issometimeshelpful.
21.
10Proposition.
χ|χnisthecoecientofzn+1in(z)χ(z).
22SomeSU(2)-relatedgroupsWenowdiscusstwogroupscloselyrelatedtoSU(2)anddescribetheirirreduciblerepresentationsandcharacters.
TheseareSO(3),SO(4)andU(2).
Westartwiththefollowing22.
1Proposition.
SO(3)=SU(2)/{±Id},SO(4)=SU(2)*SU(2)/{±(Id,Id)}andU(2)=U(1)*SU(2)/{±(Id,Id)}.
Proof.
Recalltheleft*rightmultiplicationactionofSU(2),viewedasthespaceofunitnormquaternions,onH=R4.
ThisgivesahomomorphismfromSU(2)*SU(2)→SO(4).
Notethatα*βsend1∈Htoαβ1,soα*βxes1iα=β.
Thepairα*αxeseveryotherquaternioniαiscentralinSU(2),thatis,α=±Id.
Sothekernelofourhomomorphismis{±(Id,Id)}.
WeseesurjectivityandconstructtheisomorphismSU(2)→SO(3)atthesametime.
Re-strictingourleft*rightactiontothediagonalcopyofSU(2)leadstotheconjugationactionofSU(2)onthespaceofpurequaternions,spannedbyi,j,k.
IclaimthatthisgeneratesthefullrotationgroupSO(3):indeed,rotationsinthei,j-planeareimplementedbyelementsa+bk,andsimilarlywithanypermutationofi,j,k,andtheserotationsgenerateSO(3).
ThisconstructsasurjectivehomomorphismfromSU(2)toSO(3),butwealreadyknowthatthekernelis{±Id}.
SowehavetheassertionaboutSO(3).
ReturningtoSO(4),wecantakeanyorthonormal,purequaternionframetoanyotheronebyaconjugation.
Wecanalsotake1∈Htoanyotherunitvectorbyaleftmultiplication.
52Combiningthese,itfollowsthatwecantakeanyorthonormal4-frametoanyotheronebyasuitableconjugation,followedbyaleftmultiplication.
Finally,theassertionaboutU(2)isclear,asbothU(1)andSU(2)sitnaturallyinU(2)(theformerasthescalarmatrices),andintersectat{±Id}.
Thegroupisomorphismsinthepropositionareinfacthomeomorphisms;thatmeans,theinversemapiscontinuous(usingthequotienttopologyonthequotientgroups).
Itisnotdicult(althoughabitpainful)toprovethisdirectlyfromtheconstruction,butthisfollowsmoreeasilyfromthefollowingtopologicalfactwhichisofindependentinterest.
22.
2Proposition.
AnycontinuousbijectionfromaHausdorspacetoacompactspaceisahomeomorphism.
(22.
3)RepresentationsItfollowsthatcontinuousrepresentationsofthethreegroupsinProposition22.
1arethesameascontinuousrepresentationsofSU(2),SU(2)*SU(2)andSU(2)*U(1),respectively,whichsendId,(Id,Id)and(Id,Id)totheidentitymatrix.
22.
4Corollary.
ThecompletelistofirreduciblerepresentationsofSO(3)isSym2nC2,asn≥0.
Thisformulationisslightlyabusive,asC2itselfisnotarepresentationofSO(3)butonlyofSU(2)(IdactsasId).
Butthesignproblemisxedonallevensymmetricpowers.
Forexample,Sym2C2isthestandard3-dimensionalrepresentationofSO(3).
(Thereislittlechoiceaboutit,asitistheonly3-dimensionalrepresentationonthelist).
Theimageofourcopy{diag[z,z1]}SU(2)ofU(1),inourhomomorphism,isthefamilyofmatrices1000cossin0sincos,with(caution!
)=2φ.
ThesecoveralltheconjugacyclassesinSO(3),andtheirreduciblecharactersofSO(3)restricttothisas1+2cos+2cos(2)2cos(n).
(22.
5)RepresentationsofaproductHandlingtheothertwogroups,SO(4)andU(2),requiresthefollowing22.
6Lemma.
FortheproductG*HoftwocompactgroupsGandH,thecompletelistofirreduciblerepresentationsconsistsofthetensorproductsVW,asVandWrangeovertheirreduciblesofGandH,independently.
Proofusingcharactertheory.
Fromthepropertiesofthetensorproductofmatrices,itfollowsthatthecharacterofVWattheelementg*hisχVW(g*h)=χV(g)·χW(h).
Now,aconjugacyclassinG*HisaCartesianproductofconjugacyclassesinGandH,andcharactertheoryensuresthattheχVandχWformHilbertspacebasesoftheL2classfunctionsonthetwogroups.
ItfollowsthattheχV(g)·χW(h)formaHilbertspacebasisoftheclassfunctionsonG*H,sothisisacompletelistofirreduciblecharacters.
Incidentally,theproofestablishesthecompletenessofcharactersfortheproductG*H,ifitwasknownonthefactors.
So,givenourknowledgeofU(1)andSU(2),itdoesgiveacompleteargument.
However,wealsoindicateanotherproofwhichdoesnotrelyoncharactertheory,butusescompletereducibilityinstead.
TheadvantageisthatweonlyneedtoknowcompletereducibilityunderoneofthefactorsG,H.
53Proofwithoutcharacters.
LetUbeanirreduciblerepresentationofG*H,anddecomposeitintoisotypicalcomponentsUiundertheactionofH.
BecausetheactionofGcommuteswithH,itmustbeblock-diagonalinthisdecomposition,andirreducibilityimpliesthatwehaveasingleblock.
LetthenWbetheuniqueirreducibleH-typeappearinginU,andletV:=HomH(W,U).
ThisinheritsandactionofGfromU,andwehaveanisomorphismU=WHomH(W,U)=VW(seeExampleSheet2),withGandHactingonthetwofactors.
Moreover,anyproperG-subrepresentationofVwouldleadtoaproperG*H-subrepresentationofU,andthisimpliesirreducibilityofV.
22.
7Corollary.
ThecompletelistofirreduciblerepresentationsofSO(4)isSymmC2SymnC2,withthetwoSU(2)factorsactingonthetwoSymfactors.
Here,m,n≥0andm=nmod2.
(22.
8)AcloserlookatU(2)ListingtherepresentationsofU(2)requiresacomment.
AsU(2)=U(1)*SU(2)/{±(Id,Id)},inprincipleweshouldtensortogetherpairsofrepresentationsofU(1)andSU(2)ofmatchingparity,sothat(Id,Id)actsas+Idintheproduct.
However,observethatU(2)actsnaturallyonC2,extendingtheSU(2)action,sotheactionofSU(2)onSymnC2alsoextendstoallofU(2).
Thereunder,thefactorU(1)ofscalarmatricesdoesnotacttrivially,butratherbythenthpowerofthenaturalrepresentation(sinceitactsnaturallyonC2).
Inaddition,U(2)hasa1-dimensionalrepresentationdet:U(2)→U(1).
Denotebydetmitsmthtensorpower;itrestrictstothetrivialrepresentationonSU(2)andtothe2mthpowerofthenaturalrepresentationonthescalarsubgroupU(1).
TheclassicationofrepresentationsofU(2)intermsoftheirrestrictionstothesubgroupsU(1)andSU(2)covertsthenintothe22.
9Proposition.
ThecompletelistofirreduciblerepresentationsofU(2)isdetmSymnC2,withm,n∈Z,n≥0.
(22.
10)CharactersofU(2)WesawearlierthattheconjugacyclassesofU(2)werelabelledbyunorderedpairs{z1,z2}ofeigenvalues.
Thesearedouble-coveredbythesubgroupofdiagonalmatricesdiag[z1,z2].
ThetraceofthismatrixonSymnC2iszn1+zn11z2+···+zn2,andsothecharacterofdetmSymnC2isthesymmetric8Laurentpolynomialzm+n1zm2+zm+n11zm+12zm1zm+n2.
(22.
11)Moreover,thesearealltheirreduciblecharactersofU(2).
Clearly,theyspanthespaceofsymmetricLaurentpolynomials.
GeneraltheorytellsusthattheyshouldformaHilbertspacebasisofthespaceofclassfunctions,withrespecttotheU(2)innerproduct;butwecancheckthisdirectlyfromthe22.
12Proposition(WeylintegrationformulaforU(2)).
ForaclassfunctionfonU(2),wehaveU(2)f(u)du=1214π22π*2π0*0f(φ1,φ2)·|eiφ1eiφ2|2dφ1dφ2.
Here,f(φ1,φ2)abusivelydenotesf(diag[eiφ1,eiφ2]).
Wecall(z1,z2):=z1z2=eiφ1eiφ2theWeyldenominatorforU(2).
ThepropositionisprovedbyliftingftothedoublecoverU(1)*SU(2)ofU(2)andapplyingtheintegrationformulaforSU(2);weomitthedetails.
Armedwiththeintegrationformula,wecouldhavediscoveredthecharacterformulae(22.
11)apriori,beforeconstructingtherepresentations.
Ofcourse,provingthatallformulaeareactuallyrealisedascharactersrequireseitheradirectconstruction(asgivenhere)orageneralexistenceargument,assecuredbythePeter-Weyltheorem.
8Undertheswitchz1z2.
5423TheunitarygroupTherepresentationtheoryofthegeneralunitarygroupsU(N)isverysimilartothatofU(2),withtheobviouschangethatthecharactersarenowsymmetricLaurentpolynomialsinNvariables,withintegercoecients;thevariablesrepresenttheeigenvaluesofaunitarymatrix.
Thesearepolynomialsintheziandz1iwhichareinvariantunderpermutationofthevariables.
Anysuchpolynomialcanbeexpressedas(z1z2···zN)n·f(z1,zN),wherefisagenuinesymmetricpolynomialwithintegercoecients.
ThefactthatallU(N)charactersareofthisformfollowsfromtheclassicationofconjugacyclassesinLecture20,andtherepresentationtheoryofthesubgroupU(1)N:representationsofthelatterarecompletelyreducible,withtheirreduciblebeingtensorproductsofNone-dimensionalrepresentationsofthefactors(Lemma22.
6).
(23.
1)Symmetryvs.
anti-symmetryLetusintroducesomenotation.
ForanN-tupleλ=λ1,λNofintegers,denotebyzλthe(Laurent)monomialzλ11zλ22···zλNN.
Givenσ∈SN,thesymmetricgrouponNletters,zσ(λ)willdenotethemonomialforthepermutedN-tupleσ(λ).
ThereisadistinguishedN-tupleδ=(N1,N2,0).
Theanti-symmetricLaurentpolynomialsaλ(z):=σ∈SNε(σ)·zσ(λ),whereε(σ)denotesthesignatureofσ,aredistinguishedbythefollowingsimpleobservation:23.
2Proposition.
AsλrangesoverthedecreasinglyorderedN-tuples,λ1≥λ2λN,theaλ+δformaZ-basisoftheintegralanti-symmetricLaurentpolynomials.
Thepolynomialaδ(z)isspecial,asthefollowingidentitiesshow:aδ(z)=det[zNqp]=p0,andtheyformabasisofthespaceofintegralsymmetricpolynomials.
Ourmaintheoremisnow23.
8Theorem.
TheSchurfunctionsarepreciselytheirreduciblecharactersofU(N).
FollowingthemodelofSU(2)intheearliersectionsandthegeneralorthogonalitytheoryofcharacters,thetheorembreaksupintotwostatements.
23.
9Proposition.
TheSchurfunctionsareorthonormalintheinnerproductdenedbyinte-grationoverU(N),withrespecttothenormalisedinvariantmeasure.
23.
10Proposition.
EverysλisthecharacterofsomerepresentationofU(N).
WeshallnotproveProposition23.
9here;asinthecaseofSU(2),itreducestothefollowingintegrationformula,whoseproof,however,isnowmoredicult,duetotheabsenceofaconcretegeometricmodelforU(N)withitsinvariantmeasure.
23.
11Theorem(WeylIntegrationforU(N)).
ForaclassfunctionfonU(N),wehaveU(N)f(u)du=1N!
1(2π)N2π0···2π0f(φ1,φN)·|(z)|2dφ1···dφN.
Wehaveusedthestandardconventionf(φ1,φN)=f(diag[z1,zN])andzp=eiφp.
(23.
12)ConstructionofrepresentationsMuchlikeinthecaseofSU(2),theirrepsofU(N)canbefoundintermsofthetensorpowersofitsstandardrepresentationonCN.
Formulatingthispreciselyrequiresacomment.
ArepresentationofU(N)willbecalledpolynomialiitscharacterisagenuine(asopposedtoLaurent)polynomialinthezp.
Forexample,thedeterminantrepresentationu→detuispolynomial,asitscharacterisz1···zN,butitsdualrepresentationhascharacter(z1···zN)1andisnotpolynomial.
23.
13Remark.
Asymmetricpolynomialinthezpisexpressibleintermsoftheelementarysymmetricpolynomials.
Ifthezparetheeigenvaluesofamatrixu,thesearethecoecientsofthecharacteristicpolynomialofu.
Assuch,theyhavepolynomialexpressionsintermsoftheentriesofu.
Thus,inanypolynomialrepresentationρ,thetraceofρ(u)isapolynomialintheentriesofu∈U(N).
Itturnsoutinfactthatallentriesofthematrixρ(u)arepolynomiallyexpressibleintermsofthoseofu,withoutinvolvingtheentriesofu1.
Now,thecharacterof(CN)disthepolynomial(z1zN)d,anditfollowsfromourdiscussionofSchurfunctionsandpolynomialsthatanyrepresentationofU(N)appearingintheirreducibledecompositionof(CN)dispolynomial.
ThekeystepinprovingtheexistenceofrepresentationnowconsistsinshowingthateverySchurpolynomialappearsintheirreducibledecompositionof(CN)d,forsomed.
ThisimpliesthatallSchurpolynomialsarecharactersofrepresentations.
However,anySchurfunctionconvertsintoaSchurpolynomialuponmulti-plicationbyalargepowerofdet;sothisdoesimplythatallSchurfunctionsarecharactersofU(N)-representations(apolynomialrepresentationtensoredwithalargeinversepowerofdet).
56NotethattheSchurfunctionsλishomogeneousofdegree|λ|=λ1+···+λN;soifitappearsinany(CN)d,itmustdosoford=|λ|.
Checkingitspresencerequiresusonlytoshowthatsλ|(z1zN)|λ|>0intheinnerproduct(23.
11).
Whilethereisareasonablysimpleargumentforthis,itturnsoutthatwecan(andwill)domuchmorewithlittleextraeort.
(23.
14)Schur-WeyldualityTostatethekeytheorem,observethatthespace(CN)dcarriesanactionofthesymmetricgroupSdondletters,whichpermutesthefactorsineveryvectorv1v2···vd.
Clearly,thiscommuteswiththeactionofU(N),inwhichamatrixu∈U(N)transformsalltensorfactorssimultaneously.
Thisway,(CN)dbecomesarepresentationoftheproductgroupU(N)*Sd.
23.
15Theorem(Schur-Weylduality).
UndertheactionofU(N)*Sd,(CN)ddecomposesasadirectsumofproductsλVλSλlabelledbypartitionsofdwithnomorethanNparts,inwhichVλistheirreduciblerepresentationofU(N)withcharactersλandSλisanirreduciblerepresentationofSd.
TherepresentationsSλ,forvariousλ's,dependonlyonλ(andnotonN),arepairwisenon-isomorphic.
ForN≥dtheyexhaustallirreduciblerepresentationsofSd.
Recallthatapartitionofdwithnpartsisasequenceλ1≥λ2≥.
.
.
λn>0ofintegerssummingtod.
OnceN≥d,allpartitionsofdarerepresentedinthesum.
AsthenumberofconjugacyclassesinSdequalsthenumberofpartitions,thelastsentenceinthetheoremisobvious.
Nothingelseinthetheoremisobvious;itwillbeprovedinthenextsection.
24ThesymmetricgroupandSchur-Weylduality(24.
1)ConjugacyclassedinthesymmetricgroupThedualitytheoremrelatestherepresentationsoftheunitarygroupsU(N)tothoseofthesymmetricgroupsSd,sowemustsayawordaboutthelatter.
Recallthateverypermutationhasauniquedecompositionasaproductofdisjointcycles,andthattwopermutationsareconjugateifandonlyiftheyhavethesamecycletype,whichisthecollectionofallcyclelengths,withmultiplicities.
Orderingthelengthsdecreasinglyleadstoapartition=1n>0ofd.
Assumethatthenumbers1,2,doccurm1,m2,mdtimes,respectivelyin;thatis,mkisthenumberofk-cyclesinourpermutation.
Weshallalsorefertothecycletypebythenotation(m).
Thus,theconjugacyclassesinSdarelabelledby(m)'ssuchthatkk·mk=d.
ItfollowsthatthenumberofirreduciblecharactersofSdisthenumberofpartitionsofd.
Whatisalsotruebutunexpectedisthatthereisanaturalwaytoassignirreduciblerepresentationstopartitions,asweshallexplain.
24.
2Proposition.
Theconjugacyclassofcycletype(m)hasorderd!
mk!
·kmk.
Proof.
Bytheorbit-stabilisertheorem,appliedtotheconjugationactionofSdonitself,itsucestoshowthatthecentraliserofapermutationofcycletype(m)ismk!
·kmk.
Butanelementofthecentralisermustpermutethek-cycles,forallk,whilepreservingthecyclicorderoftheelementswithin.
Wegetafactorofmk!
fromthepermutationsofthemkk-cycles,andkmkfromindependentcyclicpermutationswithineachk-cycle.
57(24.
3)TheirreduciblecharactersofSdChooseN>0anddenotebyporp(m)theproductofpowersumsp(z)=k(zk1+zk2zkN)=k>0(zk1zkN)mk;notethatifwearetoallowanumberoftrailingzeroesattheendof,theymustbeignoredintherstproduct.
ForanypartitionλofdwithnomorethanNparts,wedeneafunctiononconjugacyclassesofSdbyωλ(m)=coecientofzλ+δinp(m)(z)·(z).
Itisimportanttoobservethatωλ(m)doesnotdependonN,providedthelatterislargerthanthenumberofpartsofλ;indeed,anextravariablezN+1canbesettozero,p(m)isthenunchanged,whileboth(z)andzλ+δacquireafactorofz1z2···zN,leadingtothesamecoecientinthedenition.
Anti-symmetryofp(m)(z)·(z)andthedenitionoftheSchurfunctionsleadtothefollowing24.
4Proposition.
p(m)(z)=λωλ(m)·sλ(z),thesumrunningoverthepartitionsofdwithnomorethanNparts.
Indeed,thisisequivalenttotheidentityp(m)(z)·(z)=λωλ(m)·aλ+δ(z),whichjustrestatesthedenitionoftheωλ.
24.
5Theorem(Frobeniuscharacterformula).
TheωλaretheirreduciblecharactersofthesymmetricgroupSd.
WewillprovethattheωλareorthonormalintheinnerproductofclassfunctionsonSd.
WewillthenshowthattheyarecharactersofrepresentationsofSd,whichwillimplytheirirreducibility.
ThesecondpartwillbeprovedinconjunctionwithSchur-Weylduality.
Proofoforthonormality.
Wemustshowthatforanypartitionsλ,ν,(m)ωλ(m)·ων(m)mk!
·kmk=δλν.
(24.
6)Wewillprovetheseidentitiessimultaneouslyforalld,viatheidentity(m);λ,νωλ(m)·ων(m)mk!
·kmksλ(z)sν(w)≡λsλ(z)sλ(w)(24.
7)forvariablesz,w,with(m)rangingoverallcycletypesofallsymmetricgroupsSdandλ,νrangingoverallpartitionswithnomorethanNpartsofallintegersd.
IndependenceoftheSchurpolynomialsimplies(24.
6).
FromProposition24.
4,theleftsidein(24.
7)is(m)p(m)(z)·p(m)(w)mk!
·kmk,58whichisalso(m)k>0(zk1zkN)mk(wk1wkN)mkmk!
·kmk=(m)k>0(p,q(zpwq)k/k)mkmk!
=k>0expp,q(zpwq)k/k=expp,q;k(zpwq)k/k=expp,qlog(1zpwq)=p,q(1zpwq)1.
Wearethusreducedtoprovingtheidentityλsλ(z)sλ(w)=p,q(1zpwq)1.
(24.
8)Thereare(atleast)twowaystoproceed.
Wecanmultiplybothsidesby(z)(w)andshowthateachsideagreeswiththeN*NCauchydeterminantdet[(1zpwq)1].
Theequalitydet[(1zpwq)1]=(z)(w)p,q(1zpwq)1canbeprovedbycleverrowoperationsandinductiononN.
(SeeAppendixAofFulton-Harris,RepresentationTheory,forhelpifneeded.
)Ontheotherhand,ifweexpandeachentryinthematrixinageometricseries,multi-linearityofthedeterminantgivesdet[(1zpwq)1]=l1,.
.
.
,lN≥0det[(zpwq)lq]=l1,.
.
.
,lN≥0det[zlqp]·qwlqq=l1,.
.
.
,lN≥0al(z)wl=l1>l2>···>lN≥0al(z)al(w),thelastidentityfromtheanti-symmetryinloftheal.
Theresultistheleftsideof(24.
8)multipliedby(z)(w),asdesired.
Anotherproofof(24.
8)canbegivenbyexploitingtheorthogonalitypropertiesofSchurfunctionsandajudicioususeofCauchy'sintegrationformula.
Indeed,theleft-handsideΣ(z,w)oftheequationhasthepropertythat1N!
···Σ(z,w1)f(w)(w)(w1)dw12πiw1···dwN2πiwN=f(z)foranysymmetricpolynomialf(w),whileanyhomogeneoussymmetricLaurentpolynomialcontainingnegativepowersofthewqintegratestozero.
(Theintegralsareovertheunitcircles.
)AnN-foldapplicationofCauchy'sformulashowsthatthesameistrueforp,q(1zpw1q)1,thefunctionobtainedfromtheright-handsideof(24.
8)aftersubstitutingwqw1q,subjecttotheconvergencecondition|zp|0(zk1zkN)mk=p(m)(z),andourpropositionnowfollowsfromProp.
24.
4.
60
Forexample,thesymmetricgroupSnisthegroupofallpermutations(symmetries)of{1,n};thealternatinggroupAnisthesetofallsymmetriespreservingtheparityofthenumberoforderedpairs(didyoureallyrememberthatone);thedihedralgroupD2nisthegroupofsymmetriesoftheregularn-gonintheplane.
TheorthogonalgroupO(3)isthegroupofdistance-preservingtransformationsofEuclideanspacewhichxtheorigin.
Thereisalsothegroupofalldistance-preservingtransformations,whichincludesthetranslationsalongwithO(3).
1Theocialdenitionisofcoursemoreabstract,agroupisasetGwithabinaryoperationwhichisassociative,hasaunitelementeandforwhichinversesexist.
Associativityallowsaconvenientabuseofnotation,wherewewriteghforgh;wehaveghk=(gh)k=g(hk)andparenthesesareunnecessary.
Iwilloftenwrite1fore,butthisisdangerousonrareoccasions,suchaswhenstudyingthegroupZunderaddition;inthatcase,e=0.
Theabstractdenitionnotwithstanding,theinterestingsituationinvolvesagroup"acting"onaset.
Formally,anactionofagroupGonasetXisan"actionmap"a:G*X→Xwhichiscompatiblewiththegrouplaw,inthesensethata(h,a(g,x))=a(hg,x)anda(e,x)=x.
Thisjustiestheabusivenotationa(g,x)=g.
xorevengx,forwehaveh(gx)=(hg)x.
Fromthispointofview,geometryasks,"GivenageometricobjectX,whatisitsgroupofsymmetries"Representationtheoryreversesthequestionto"GivenagroupG,whatobjectsXdoesitacton"andattemptstoanswerthisquestionbyclassifyingsuchXuptoisomorphism.
Beforerestrictingtothelinearcase,ourmainconcern,letusrememberanotherwaytodescribeanactionofGonX.
Everyg∈Gdenesamapa(g):X→Xbyx→gx.
Thismapisabijection,withinversemapa(g1):indeed,a(g1)a(g)(x)=g1gx=ex=xfromthepropertiesoftheaction.
Hencea(g)belongstothesetPerm(X)ofbijectiveself-mapsofX.
Thissetformsagroupundercomposition,andthepropertiesofanactionimplythat1.
1Proposition.
AnactionofGonX"isthesameas"agrouphomomorphismα:G→Perm(X).
1.
2Remark.
Thereisalogicalabusehere,clearlyanaction,denedasamapa:G*X→XisnotthesameasthehomomorphismαintheProposition;youaremeanttoreadthatspecifyingoneiscompletelyequivalenttospecifyingtheother,unambiguously.
Butthedenitionsaredesignedtoallowsuchabusewithoutmuchdanger,andIwillfrequentlyindulgeinthat(infactIdenotedαbyainlecture).
21Thisgroupisisomorphictothesemi-directproductO(3)R3—butifyoudonotknowwhatthismeans,donotworry.
2Withrespecttoabuse,youmaywishtoerronthesideofcautionwhenwritingupsolutionsinyourexam!
1ThereformulationofProp.
1.
1leadstothefollowingobservation.
ForanyactionaHonXandgrouphomomorphism:G→H,thereisdenedarestrictedorpulled-backactionaofGonX,asa=a.
Intheoriginaldenition,theactionsends(g,x)to(g)(x).
(1.
3)Example:TautologicalactionofPerm(X)onXThisistheobviousaction,callitT,sending(f,x)tof(x),wheref:X→Xisabijectionandx∈X.
Checkthatitsatisesthepropertiesofanaction!
Inthislanguage,theactionaofGonXisαT,withthehomomorphismαoftheproposition—thepull-backunderαofthetautologicalaction.
(1.
4)Linearity.
ThequestionofclassifyingallpossibleXwithactionofGishopelessinsuchgenerality,butoneshouldrecallthat,inrstapproximation,mathematicsislinear.
SoweshalltakeourXtoavectorspaceoversomegroundeld,andaskthattheactionofGbelinear,aswell,inotherwords,thatitshouldpreservethevectorspacestructure.
OurinterestismostlyconnedtothecasewhentheeldofscalarsisC,althoughweshalloccasionalmentionhowthepicturechangeswhenothereldsarestudied.
1.
5Denition.
AlinearrepresentationρofGonacomplexvectorspaceVisaset-theoreticactiononVwhichpreservesthelinearstructure,thatis,ρ(g)(v1+v2)=ρ(g)v1+ρ(g)v2,v1,2∈V,ρ(g)(kv)=k·ρ(g)v,k∈C,v∈VUnlessotherwisementioned,representationwillmeannite-dimensionalcomplexrepresentation.
(1.
6)Example:ThegenerallineargroupLetVbeacomplexvectorspaceofdimensionnm.
Moregenerally,dimΛmV=1anddimΛkV=mk.
Notation:Inthesymmetricpower,weoftenuseu1untodenotethesymmetrisedvectorsinProp.
7.
4,whileintheexteriorpower,oneusesu1un.
8ThecharacterofarepresentationWenowturntothecoreresultsofthecourse,thecharactertheoryofgrouprepresentations.
Thisallowsaneectivecalculuswithgrouprepresentations,includingtheirtensorproducts,andtheirdecompositionintoirreducibles.
WewanttoattachinvariantstoarepresentationρofanitegroupGonV.
Thematrixcoecientsofρ(g)arebasis-dependent,hencenottrueinvariants.
Observe,however,thatggeneratesanitecyclicsubgroupofG;thisimpliesthefollowing(seeLecture2).
8.
1Proposition.
IfGanddimVarenite,theneveryρ(g)isdiagonalisable.
Moreprecisely,alleigenvaluesofρ(g)willberootsofunityofordersdividingthatofG.
(ApplyLagrange'stheoremtothecyclicsubgroupgeneratedbyg.
)Toeachg∈G,wecanassignitseigenvaluesonV,uptoorder.
Numericalinvariantsresultfromtheelementarysymmetricfunctionsoftheseeigenvalues,whichyoualsoknowasthecoecientsofthecharacteristicpolynomialdetV[λρ(g)].
Especiallymeaningfularetheconstantterm,detρ(g)(uptosign);thesub-leadingterm,Trρ(g)(uptosign).
Thefollowingisclearfrommultiplicativityofthedeterminant:8.
2Proposition.
Themapg→detVρ(g)∈Cdenesa1-dimensionalrepresentationofG.
Thisisanice,but"weak"invariant.
Forinstance,youmayknowthatthealternatinggroupA5issimple,thatis,ithasnopropernormalsubgroups.
Becauseitisnotabelian,anyhomomorphismtoCmustbetrivial,sothedeterminantofanyofitsrepresentationsis1.
Evenforabeliangroups,thedeterminantistooweaktodistinguishisomorphismclassesofrepresentations.
Thewinnerturnsouttobetheotherinterestinginvariant.
8.
3Denition.
Thecharacteroftherepresentationρisthecomplex-valuedfunctiononGdenedbyχρ(g):=TrV(ρ(g)).
TheBigTheoremofthecourseisthatthisisacompleteinvariant,inthesensethatitdeterminesρuptoisomorphism.
Forarepresentationρ:G→GL(V),wehavedenedχV:G→CbyχV(g)=TrV(ρ(g)).
8.
4Theorem(Firstproperties).
1.
χVisconjugation-invariant,χV(hgh1)=χV(g),g,h∈G;2.
χV(1)=dimV;3.
χV(g1)=χV(g);4.
FortworepresentationsV,W,χVW=χV+χW,andχVW=χV·χW;5.
ForthedualrepresentationV,χV(g)=χV(g1).
17Proof.
Parts(1)and(2)areclear.
Forpart(3),chooseaninvariantinnerproductonV;unitarityofρ(g)impliesthatρ(g1)=ρ(g)1=ρ(g)T,whence(3)followsbytakingthetrace.
4Part(4)isclearfromTrA00B=TrA+TrB,andρVW=ρV00ρW.
TheproductformulafollowsformtheidentityTr(AB)=TrA·TrBfromLecture6.
Finally,Part(5)followsformthefactthattheactionofgonalinearmapL∈Hom(V;C)=Viscompositionwithρ(g)1(Lecture5).
8.
5Remark.
conjugation-invariantfunctionsonGarealsocalledcentralfunctionsorclassfunc-tions.
Theirvalueatagroupelementgdependsonlyontheconjugacyclassofg.
Wecanthereforeviewthemasfunctionsonthesetofconjugacyclasses.
(8.
6)TherepresentationringWecanrewriteproperty(4)moreprofessionallybyintroducinganalgebraicconstruct.
8.
7Denition.
TherepresentationringRGofthenitegroupGisthefreeabeliangroupbasedonthesetofisomorphismclassesofirreduciblerepresentationsofG,withmultiplicationreectingthetensorproductdecompositionofirreducibles:[V]·[W]=knk·[Vk]iVW=kVnkk,wherewewrite[V]fortheconjugacyclassofanirreduciblerepresentationV,andthetensorproductVWhasbeendecomposedintoirreduciblesVk,withmultiplicitiesnk.
Thusdened,therepresentationringisassociativeandcommutative,withidentitythetrivial1-dimensionalrepresentation:[C]·[W]=[W]foranyW,becauseCW=W.
Example:G=Cn,thecyclicgroupofordernOurirreduciblesareL0,Ln1,wherethegeneratorofCnactsonLkasexp2πikn.
WehaveLpLq=Lp+q,subscriptsbeingtakenmodulon.
ItfollowsthatRCn=Z[X]/(Xn1),identifyingLkwithXk.
Theprofessionalrestatementofproperty(4)inTheorem8.
4isthefollowing.
8.
8Proposition.
ThecharacterisaringhomomorphismfromRGtotheclassfunctionsonG.
IttakestheinvolutionVVtocomplexconjugation.
Later,weshallreturntothishomomorphismandlistsomeothergoodproperties.
(8.
9)OrthogonalityofcharactersFortwocomplexfunctions,ψonG,welet|ψ:=1|G|g∈G(g)·ψ(g).
Inparticular,thisdenesaninnerproductonclassfunctions,ψ,wherewesumoverconjugacyclassesCG:|ψ=1|G||C|·(C)ψ(C).
Wearenowreadyfortherstpartofourmaintheorem.
Therewillbeacomplementinthenextlecture.
4Foranalternativeargument,recallthattheeigenvaluesofρ(g)arerootsofunity,andthoseofρ(g)1willbetheirinverses;butthesearealsotheirconjugates.
Recallnowthatthetraceisthesumoftheeigenvalues.
188.
10Theorem(Orthogonalityofcharacters).
1.
IfVisirreducible,thenχV2=1.
2.
IfV,Wareirreducibleandnotisomorphic,thenχV|χW=0.
Beforeprovingthetheorem,letuslistsomeconsequencestoillustrateitspower.
8.
11Corollary.
ThenumberoftimesanirreduciblerepresentationVappearsinanirreducibledecompositionofsomeWisχV|χW.
8.
12Corollary.
Theabovenumber(calledthemultiplicityofVinW)isindependentoftheirreducibledecompositionWehadalreadyprovedthisinLecture4,butwenowhaveasecondproof.
8.
13Corollary.
Tworepresentationsareisomorphicitheyhavethesamecharacter.
(Usecompletereducibilityandtherstcorollaryabove).
8.
14Corollary.
ThemultiplicityofthetrivialrepresentationinWis1|G|g∈GχW(g).
8.
15Corollary(Irreducibilitycriterion).
VisirreducibleiχV2=1.
Proof.
DecomposeVintoirreduciblesaskVnkk;then,χV=knk·χk,andχV2=kn2k.
SoχV2=1iallthenk'svanishbutone,whosevaluemustbe1.
Inpreparationfortheproofoforthogonality,weestablishthefollowingLemma.
FortworepresentationsV,WofGandanylinearmapφ:V→W,deneφ0=1|G|g∈GρW(g)φρV(g)1.
8.
16Lemma.
1.
φ0intertwinestheactionsG.
2.
IfVandWareirreducibleandnotisomorphic,thenφ0=0.
3.
IfV=WandρV=ρW,thenφ0=TrφdimV·Id.
Proof.
Forpart(1),wejustexaminetheresultofconjugatingbyh∈G:ρW(h)φ0ρV(h)1=1|G|g∈GρW(h)ρW(g)φρV(g)1ρV(h)1=1|G|g∈GρW(hg)φρV(hg)1=1|G|g∈GρW(g)φρV(g)1=φ0.
Part(2)nowfollowsfromSchur'slemma.
Forpart(3),Schur'slemmaagaintellsusthatφ0isascalar;tondit,itsucestotakethetraceoverV:then,φ0=Tr(φ0)/dimV.
ButwehaveTr(φ0)=1|G|g∈GTrρV(g)φρV(g)1=Tr(φ),asclaimed.
19Proofoforthogonality.
ChooseinvariantinnerproductsonV,Wandorthonormalbases{vi}and{wj}forthetwospaces.
WhenV=Wweshallusethesameinnerproductandbasisinboth.
Weshallusethe"bra·ket"vectornotationexplainedinthehandout.
ThenwehaveχW|χV=1|G|g∈GχW(g)χV(g)=1|G|g∈GχW(g1)χV(g)=1|G|i,jg∈Gwi|ρW(g)1|wivj|ρV(g)|vj.
Wenowinterpreteachsummandasfollows.
Recallrstthat|wivj|designatesthelinearmapV→Wwhichsendsavectorvtothevectorwi·vj|v.
Theproductwi|ρW(g)1|wivj|ρV(g)|vjistheninterpretableastheresultofapplyingthelinearmapρW(g)1|wivj|ρV(g)tothevector|vj,andthentakingdotproductwithwj.
Fixnowiandjandsumoverg∈G.
Lemma8.
16thenshowsthatthesumoflinearmapsρW(g)1|wivj|ρV(g)=0ifVW,Tr(|wivj|)/dimVifρV=ρW.
(8.
17)Intherstcase,summingoveri,jstillleadstozero,andwehaveprovedthatχV⊥χW.
Inthesecondcase,Tr(|wivj|)=1ifi=j,0otherwise,andsummingoveri,jleadstoafactorofdimV,cancellingthedenominatorinthesecondlineof(8.
17)andcompletingourproof.
8.
18Remark.
Theinnerproductofcharacterscanbedenedoveranygroundeldkofcharac-teristicnotdividing|G|,byusingχ(g1)inlieuofχ(g).
WehaveusedSchur'sLemmaoverCinestablishingPart(3)ofLemma8.
16,althoughnotforParts1and2.
Hence,theorthogonal-ityofcharactersofnon-isomorphicirreduciblesholdsoveranysucheldk,butorthonormalityχ2=1canfailifkisnotalgebraicallyclosed.
Thevalueofthesquaredependsonthedecompositionoftherepresentationinthealgebraicclosurek.
ThiscanbedeterminedfromthedivisionalgebraDV=EndGk(V)andtheGaloistheoryofitscentre,whichwillbeaniteextensioneldofk.
See,forinstance,Serre'sbookformoredetail.
(8.
19)TherepresentationringagainWedenedtherepresentationringRGofGasthefreeabeliangroupbasedontheisomorphismclassesofirreduciblerepresentationsofG.
Usingcompletereducibility,wecanidentifythelinearcombinationsofthesebasiselementswithnon-negativecoecientswithisomorphismclassesofG-representations:wesimplysendarepresentationiVnii,decomposedintoirreducibles,tothelinearcombinationini[Vi]∈RG(thebracketdenotestheisomorphismclass).
GeneralelementsofRGaresometimescalledvirtualrepresentationsofG.
WedenedthemultiplicationinRGusingthetensorproductofrepresentations.
Wesometimesdenotetheproductby,torememberitsorigin.
Theone-dimensionaltrivialrepresentation1istheunit.
WethendenedthecharacterχVofarepresentationρonVtobethecomplex-valuedfunctiononGwithχV(g)=TrVρ(g),theoperatortraceintherepresentationρ.
Thisfunctionisconjugation-invariant,oraclassfunctiononG.
WedenotethespaceofcomplexclassfunctionsonGbyC[G]G.
Bylinearity,χbecomesamapχ:RG→C[G]G.
WethencheckedthatχVW=χV+χW,χVW=χV·χW.
Thus,χ:RG→C[G]Gisaringhomomorphism.
Dualitycorrespondstocomplexconjugation:χ(V)=χV.
20ThelinearfunctionalInv:RG→Z,sendingavirtualrepresentationtothemultiplicity(=coecient)ofthetrivialrepresentation1correspondstoaveragingoverG:Inv(V)=1|G|g∈GχV(g).
WecandeneapositivedeniteinnerproductonRGbyV|W:=Inv(VW).
Thiscorrespondstotheobviousinnerproductoncharacters,1|G|g∈GχV(g)χW(g).
8.
20Remark.
ThestructuresdetailedaboveforRGisthatofaFrobeniusringwithinvolution.
(Inaringwithoutinvolution,wewouldjustrequirethatthebilinearpairingV*W→Inv(VW)shouldbenon-degenerate).
Similarly,C[G]GisacomplexFrobeniusalgebrawithinvolution,andwearesayingthatthecharacterχisahomomorphismofsuchstructures.
Itfailstobeanisomorphism"onlyintheobviousway":thetworingsshareabasisofirreduciblerepresentations(resp.
characters),buttheRGcoecientsareintegral,notcomplex.
9TheRegularRepresentationRecallthattheregularrepresentationofGhasbasis{eg},labelledbyg∈G;weletGpermutethebasisvectorsaccordingtoitsleftaction,λ(h)(eg)=ehg.
Thisdenesalinearactiononthespanoftheeg.
WecanidentifythespanoftheegwiththespaceC[G]ofcomplexfunctionsonG,bymatchingegwiththefunctionsendinggto1∈Candallothergroupelementstozero.
Underthiscorrespondence,elementsh∈Gact("ontheleft")onC[G],bysendingthefunctiontothefunctionλ(h)()(g):=(h1g).
Wecanseethatthecharacteroftheregularrepresentationisχreg(g)=|G|ifg=10otherwiseinparticular,χreg2=|G|2/|G|=|G|,sothisisfarfromirreducible.
Indeed,everyirreduciblerepresentationappearsintheregularrepresentation,asthefollowingpropositionshows.
9.
1Proposition.
Themultiplicityofanyirreduciblerepresentationintheregularrepresentationequalsitsdimension.
Proof.
χV|χreg=1|G|χV(1)χreg(1)=χV(1)=dimV.
Forinstance,thetrivialrepresentationappearsexactlyonce,whichwecanalsoconrmdirectly:theonlytranslation-invariantfunctionsonGareconstant.
9.
2Proposition.
Vdim2V=|G|,thesumrangingoverallirreducibleisomorphismclasses.
Proof.
Fromχreg=dimV·χVwegetχreg2=dim2V,asdesired.
Wenowproceedtocompleteourmainorthogonalitytheorem.
9.
3Theorem(Completenessofcharacters).
Theirreduciblecharactersformabasisforthespaceofcomplexclassfunctions.
Thus,theyformanorthonormalbasisforthatspace.
Theproofconsistsinshowingthatifaclassfunctionisorthogonaltoeveryirreduciblecharacter,thenitsvalueateverygroupelementiszero.
Forthis,weneedthefollowing.
21Construction.
Toeveryfunction:G→C,andtoeveryrepresentation(V,ρ)ofG,weassignthefollowinglinearself-mapρ()ofV:ρ()=g∈G(g)ρ(g).
Wenote,byapplyingρ(h)(h∈G)andrelabellingthesum,thatρ(h)ρ()=ρ(λ(h)).
9.
4Lemma.
isaclassfunctioniρ()commuteswithG,ineveryrepresentationV.
Proof.
Ifisaclassfunction,then(h1gh)=(g)forallg,handsoρ(h)ρ()ρ(h1)=g∈G(g)ρ(hgh1)=k∈G(h1kh)ρ(k)=k∈G(k)ρ(k).
Intheotherdirection,letVbetheregularrepresentation;thenρ()(e1)=g∈G(g)eg,andλ(h)ρ()(e1)=g∈G(g)ehg=g∈G(h1g)eg,whereasρ()λ(h)(e1)=ρ()(eh)=g∈G(g)egh=g∈G(gh1)eg;equatingthetwoshowsthat(h1g)=(gh1),asclaimed.
WhenisaclassfunctionandVisirreducible,Schur'sLemmaandthepreviousresultshowthatρ()isascalar.
Tondit,wecomputethetrace:Tr[ρ()]=g∈G(g)χV(g)=|G|·χV|(recallthatχV=χV).
Weobtainthat,whenVisirreducible,ρ()=|G|dimV·χV|·Id.
(9.
5)ProofofCompleteness.
Assumethattheclassfunctionisorthogonaltoallirreduciblechar-acters.
By(9.
5),ρ()=0ineveryirreduciblerepresentationV,hence(bycompletereducibil-ity)alsointheregularrepresentation.
But,aswesawintheproofofLemma9.
4,wehaveρ()(e1)=g∈G(g)egintheregularrepresentation;so=0,asdesired.
(9.
6)TheGroupalgebraWenowstudytheregularrepresentationingreaterdepth.
DeneamultiplicationonC[G]bysettingeg·eh=eghonthebasiselementsandextendingbylinearity:ggeg·hψheh=g,hgψhegh=ghgh1ψheg.
Thisisassociativeanddistributiveforaddition,butitisnotcommutativeunlessGwasso.
(Associativityfollowsfromthesamepropertyinthegroup.
)Itcontainse1asthemultiplicativeidentity,andthecopyCe1ofCcommuteswitheverything.
ThismakesC[G]intoaC-algebra.
ThegroupGembedsintothegroupofmultiplicativelyinvertibleelementsofC[G]byg→eg.
Forthisreason,wewilloftenreplaceegbyginournotation,andthinkofelementsofC[G]aslinearcombinationsofgroupelements,withtheobviousmultiplication.
229.
7Proposition.
ThereisanaturalbijectionbetweenmodulesoverC[G]andcomplexrepre-sentationsofG.
Proof.
Oneachrepresentation(V,ρ)ofG,weletthegroupalgebraactintheobviousway,ρgg·g(v)=ggρ(g)(v).
Conversely,givenaC[G]-moduleM,theactionofCe1makesitintoacomplexvectorspaceandagroupactionisdenedbyembeddingGinsideC[G]asexplainedabove.
Wecanreformulatethediscussionabove(perhapsmoreobscurely)bysayingthatagrouphomomorphismρ:G→GL(V)extendsnaturallytoanalgebrahomomorphismfromC[G]toEnd(V);VisamoduleoverEnd(V)andtheextendedhomomorphismmakesitintoaC[G]-module.
Conversely,anysuchhomomorphismdenesbyrestrictionarepresentationofG,fromwhichtheoriginalmapcanthenberecoveredbylinearity.
Choosingacompletelist(uptoisomorphism)ofirreduciblerepresentationsVofGgivesahomomorphismofalgebras,ρV:C[G]→VEnd(V),→(ρV()).
(9.
8)NoweachspaceEnd(V)carriestwocommutingactionsofG:theseareleftcompositionwithρV(g),andrightcompositionwithρV(g)1.
Foranalternativerealisation,End(V)isisomorphictoVVandGactsseparatelyonthetwofactors.
TherearealsotwocommutingactionsofGonC[G],bymultiplicationontheleftandontheright,respectively:(λ(h))(g)=(h1g),(ρ(h))(g)=(gh).
ItisclearfromourconstructionthatthetwoactionsofG*Gontheleftandrightsidesof(9.
8)correspond,henceouralgebrahomomorphismisalsoamapofG*G-representations.
ThemainresultofthissubsectionisthefollowingmuchmorepreciseversionofProposition9.
1.
9.
9Theorem.
Thehomomorphism(9.
8)isanisomorphismofalgebras,andhenceofG*G-representations.
Inparticular,wehaveanisomorphismofG*G-representations,C[G]=VVV,withthesumrangingovertheirreduciblerepresentationsofG.
Fortheproof,werequirethefollowing9.
10Lemma.
LetV,WbecomplexirreduciblerepresentationsofthenitegroupsGandH.
Then,VWisanirreduciblerepresentationofG*H.
Moreover,everyirrepofG*Hisofthatform.
Thereadershouldbecautionedthatthestatementcanfailiftheeldisnotalgebraicallyclosed.
Indeed,theproofusestheorthonormalityofcharacters.
Proof.
Directcalculationofthesquarenormshowsthatthecharacterχv(g)*χW(h)ofG*Hisirreducible.
(Homework:dothis!
).
Moreover,IclaimthatthesecharactersspantheclassfunctionsonG*H.
Indeed,conjugacyclassesinG*HareCartesianproductsofclassesinthefactors,andwecanwriteeveryindicatorclassfunctioninGandHasalinearcombinationofcharacters;sowecanwriteeveryindicatorclassfunctiononG*HasalinearcombinationoftheχV*χW.
23Inparticular,itfollowsthattherepresentationVVofG*Gisirreducible.
Moreover,notwosuchrepsfordistinctVcanbeisomorphic;indeed,onpairs(g,1)∈G*G,thecharacterreducestothatofV.
ProofofTheorem9.
8.
Bydimension-count,itsucestoshowthatourmapρVissurjective.
TheG*G-structurewillbeessentialforthis.
NotefromourLemmathatthedecompositionS:=VVVsplitsthesumSintopairwisenon-isomorphic,irreduciblerepresentations.
FromtheresultsinLecture4,weknowthatanyG*G-mapintoSmustbeblock-diagonalfortheisotypicaldecompositions.
Inparticular,ifamapsurjectsontoeachfactorseparately,itsurjectsontothesumS.
Sincethesummandsareirreducible,itsucesthentoshowthateachprojectiontoEnd(V)isnon-zero.
Butthegroupidentitye1mapstotheidentityineachEnd(V).
9.
11Remark.
(i)ItcanbeshownthattheinversemaptoρVassignstoφ∈End(V)thefunctiong→TrV(ρV(g)φ)/dimV.
(OptionalHomework.
)(ii)Schur'sLemmaensuresthattheinvariantsontheright-handsidefortheactionofthediagonalcopyGG*Garethemultiplesoftheidentityineachsummand.
Ontheleftside,wegettheconjugation-invariantfunctions,orclassfunctions.
10ThecharactertableInviewofourtheoremaboutcompletenessandorthogonalityofcharacters,thegoalofcomplexrepresentationtheoryforanitegroupistoproducethecharactertable.
Thisisthesquarematrixwhoserowsarelabelledbytheirreduciblecharacters,andwhosecolumnsarelabelledbytheconjugacyclassesinthegroup.
Theentriesinthetablearethevaluesofthecharacters.
Arepresentationcanberegardedas"known"assoonasitscharacterisknown.
Indeed,onecanextracttheirreduciblemultiplicitiesfromthedotproductswiththerowsofthechar-actertable.
Youwillseeinthehomework(Q.
2.
5)thatyoucanevenconstructtheisotypicaldecompositionofyourrepresentation,oncetheirreduciblecharactersareknown.
Orthonormalityofcharactersimpliesacertainorthonormalityoftherowsofthecharactertable,whenviewedasamatrixA.
"Acertain"reectstothefactthattheinnerproductbetweencharactersisnotthedotproductoftherows;rather,thecolumnofaclassCmustbeweighedbyafactor|C|/|G|inthedotproduct(asthesumgoesovergroupelementsandnotconjugacyclasses).
Inotherwords,ifBisthematrixobtainedfromAbymultiplyingeachcolumnby|C|/|G|,thentheorthonormalityrelationsimplythatBisaunitarymatrix.
Orthonormalityofitscolumnsleadstothe10.
1Proposition(Thesecondorthogonalityrelations).
ForanyconjugacyclassesC,C,wehave,summingovertheirreduciblecharactersχofG,χχ(C)·χ(C)=|G|/|C|ifC=C,0otherwise.
10.
2Remark.
Theorbit-stabilisertheorem,appliedtotheconjugationactionofGonitselfandsomeelementc∈C,showsthattheprefactor|G|/|C|istheorderofZG(c),thecentraliserofcinG(thesubgroupofG-elementscommutingwithc).
Asareformulation,wecanexpresstheindicatorfunctionECofaconjugacyclassCGintermsofcharacters:EC(g)=|C||G|χχ(C)·χ(g).
Notethatthecoecientsneednotbeintegral,sotheindicatorfunctionofaconjugacyclassisnotthecharacterofarepresentation.
24(10.
3)Example:ThecyclicgroupCnofordernCallgagenerator,andletLkbetheone-dimensionalrepresentationwheregactsasωk,whereω=exp2πin.
ThenweknowthatL0,Ln1arealltheirreduciblesandthecharactertableisthefollowing:{1}{g}gq}.
.
.
111.
.
.
1.
.
.
L01ω.
.
.
ωq.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
Lp1ωp.
.
.
ωpq.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
MovingontoD6,forwhichwehadfoundtheone-dimensionalirreducibles1,Sandthe2-dimensionalirreducibleV,wenotethat1+1+22=6sotherearenootherirreducibles.
Wethusexpect3conjugacyclassesand,indeed,wend{1},{g,g2},{r,rg,rg2},thethreereectionsbeingallconjugate(g1rg=rg2,etc).
Fromourmatrixconstructionoftherepresentationwellintheentriesofthecharactertable:{1}{g,g2}{rg,.
.
.
}1111S111V210Letuscheckoneoftheorthogonalityrelations:χ2V=(22+12)/6=5/6,whichdoesnotmatchthetheoremverywell;thatis,ofcourse,becauseweforgottoweightheconjugacyclassesbytheirsize.
Itishelpfultomakeanoteoftheweightssomewhereinthecharactertable.
Thecorrectcomputationisχ2V=(22+2·12)/6=1.
ThegeneraldihedralgroupD2nworksverysimilarly,butthereisadistinction,accordingtotheparityofn.
Letusrsttaken=2m+1,odd,andletω=exp(2πi/n).
Wecanagainseethetrivialandsignrepresentations1andS;additionally,wehavemtwo-dimensionalrepresentationsVk,labelledby0xvmus=0,wherexmodpisaxed,non-zeroresidueandmandsinthesumrangeoverallpossiblechoices.
12ThealternatinggroupA5InthislectureweconstructthecharactertableofthealternatinggroupA5ofevenpermutationsonveletters.
Unlikethepreviousexamples,wewillnowexploitthepropertiesofcharactersinndingrepresentationsandprovingtheirirreducibility.
Westartwiththeconjugacyclasses.
Recallthateverypermutationisaproductofdisjointcycles,uniquelyuptotheirorder,andthattwopermutationsareconjugateinthesymmetric27groupitheyhavethesamecycletype,meaningthecyclelengths(withtheirmultiplicities).
Thus,acompletesetofrepresentativesfortheconjugacyclassesinS5isId,(12),(12)(34),(123),(123)(45),(1234),(12345),sevenintotal.
Ofthese,onlyId,(12)(34),(123)and(12345)areinA5.
Now,aconjugacyclassinS5mayormaynotbreakupintoseveralclassesinA5.
ThereasonisthatwemayormaynotbeabletorelatetwogivenpermutationsinA5whichareconjugateinA5byanevenpermutation.
Nowtherstthreepermutationsinourlistallcommutewithsometransposition.
ThisensuresthatwecanimplementtheeectofanyconjugationinS5byaconjugationinA5:conjugatebytheextratranspositionifnecessary.
Wedonothavesuchanargumentforthe5-cycle,sothereisthepossibilitythatthecycles(12345)and(21345)arenotconjugateinA5.
Tomakesure,wecountthesizeoftheconjugacyclassbytheorbit-stabilisertheorem.
Thesizeoftheconjugacyclasscontainingg∈Gis|G|dividedbytheorderofthecentraliserofg.
Thecentraliserof(12345)inS5isthecyclicsubgroupoforder5generatedbythiscycle,andisthereforethesameasthecentraliserinA5.
SotheorderoftheconjugacyclassinS5is24,butinA5only12.
Sooursuspicionsareconrmed,andtherearetwoclassesof5-cyclesinA5.
12.
1Remark.
Thesameorbit-stabiliserargumentshowsthattheconjugacyclassinSnofanevenpermutationσisasingleconjugacyclassinAnpreciselywhenσcommuteswithsomeoddpermutation;else,itbreaksupintotwoclassesofequalsize.
Foruseincomputations,wenotethattheordersoftheconjugacyclassesare1forId,15for(12)(34),20for(123)and12eachfor(12345)and(21345).
Wehave,asweshould,1+15+20+12+12=60=|A5|.
Wenowstartproducingtherowsofthecharactertable,listingtheconjugacyclassesintheorderabove.
Thetrivialrep1givestherow1,1,1,1,1.
Next,weobservethatA5actsnaturallyonC5bypermutingthebasisvectors.
Thisrepresentationisnotirreducible;indeed,thelinespannedbythevectore1e5isinvariant.
ThecharacterχC5is5,1,2,0,0anditsdotproductχC5|χ1=5/60+1/4+2/3=1withthetrivialrepshowsthat1appearsinC5withmultiplicityone.
ThecomplementVhascharacterχV=4,0,1,1,1,whosesquarenormisχV2=1660+13+15+15=1,showingthatVisirreducible.
Weseekmorerepresentations,andconsiderforthatthetensorsquareV2.
Wealreadyknowthisisreducible,becauseitsplitsasSym2VΛ2V,butwetryextractirreduciblecomponents.
ForthisweneedthecharacterformulaeforSym2VandΛ2V.
12.
2Proposition.
Foranyg∈G,χSym2V(g)=χV(g)2+χV(g2)/2,χΛ2V(g)=χV(g)2χV(g2)/2.
Proof.
Havingxedg,wechooseabasisofVonwhichgactsdiagonally,witheigenvaluesλ1,λd,repeatedasnecessary.
FromourbasesofSym2V,Λ2V,weseethattheeigenvaluesofgonthetwospacesareλpλq,with1≤p≤q≤donSym2andwith1≤p1).
ProofoftheLemma.
Ifq∈U(1)isnotarootofunity,thenitspowersaredenseinU(1)(exercise,usingthepigeonholeprinciple).
So,anyclosed,propersubgroupofU(1)consistsonlyofrootsof1.
Amongthose,theremustbeoneofsmallestargument(inabsolutevalue)orelsetherewouldbeasequenceconvergingto1;thesewouldagaingenerateadensesubgroupofU(1).
Therootofunityofsmallestargumentisthenthegenerator.
ProofofTheorem19.
10.
Acontinuousmap:U(1)→C*hascompact,henceboundedimage.
Theimagemustlieontheunitcircle,becausetheintegralpowersofanyothercomplexnumberformanunboundedsequence.
SoisacontinuoushomomorphismfromU(1)itself.
Now,kerisaclosedsubgroupofU(1).
Ifkeristheentiregroup,then=1andthetheoremholdswithn=0.
Ifker=n(n≥1),wewillnowshowthat(z)=z±n,withthesamechoiceofsignforallz.
Toseethis,deneacontinuousfunctionψ:[0,2π/n]→R,ψ(0)=0,ψ(θ)=arg(eiθ);inotherwords,weparametriseU(1)bytheargumentθ,startwithψ(0)=0,whichisonevalueoftheargumentof(1)=1,choosetheargumentsoastomakethefunctioncontinuous.
Becauseker=n,ψmustbeinjectiveon[0,2π/n).
Bycontinuity,itmustbemonotonicallyincreasingordecreasing(IntermediateValueTheorem),andwemusthaveψ(2π/n)=±2π:thevaluezeroisruledoutbymonotonicityandanyothermultipleof2πwouldleadtoanintermediatevalueofθwith(eiθ)=1.
Henceforth,±denotesthesignofψ(2π/n).
Becauseisahomomorphismand(e2πi/n)=1,(e2πi/mn)mustbeanmthrootofunity,andsoψ({2πk/mn}){±2πk/m},k=0,m.
Bymonotonicity,thesem+1valuesmustbetakenexactlyonceandinthenaturalorder,soψ(2πk/mn)=±2πk/m,forallmandallk=0,m.
Butthen,ψ(θ)=±n·θ,bycontinuity,and(z)=z±n,asclaimed.
19.
12Remark.
Completereducibilitynowshowsthatcontinuousnite-dimensionalrepresenta-tionsofU(1)areinfactalgebraic:thatis,theentriesinamatrixrepresentationsareLaurentpolynomialsinz.
Thisisnotanaccident;itholdsforalargeclassoftopologicalgroups(thecompactLiegroups).
45(19.
13)CharactertheoryThehomomorphismsρn:U(1)→C*,ρn(z)=znformthecompletelistofirreduciblerepre-sentationsofU(1).
Clearly,theircharacters(whichweabusivelydenotebythesamesymbol)arelinearlyindependent;infact,theyareorthonormalintheinnerproduct|ψ=12π2π0(θ)ψ(θ)dθ,whichcorrespondsto"averagingoverU(1)"(z=eiθ).
ThefunctionsρnaresometimescalledFouriermodes,andtheirnitelinearcombinationsaretheFourierpolynomials.
AsU(1)isabelian,itcoincideswiththespaceofitsconjugacyclasses.
Wecannowstateourmaintheorem.
19.
14Theorem.
(i)ThefunctionsρnformacompletelistofirreduciblecharactersofU(1).
(ii)Everynite-dimensionalrepresentationVofU(1)isisomorphictoasumoftheρn.
ItscharacterχVisaFourierpolynomial.
ThemultiplicityofρninVistheinnerproductρn|χV.
RecallthatcompletereducibilityofrepresentationsfollowsbyWeyl'sunitarytrick,averaginganygiveninnerproductbyintegrationonU(1).
Asthespaceof(continuous)classfunctionsisinnite-dimensional,itrequiresabitofcaretostatethenalpartofourmaintheorem,thatthecharactersforma"basis"ofthespaceofclassfunctions.
ThegoodsettingforthatistheHilbertspaceofsquare-integrablefunctions,whichwediscussbelow.
Fornow,letusjustnoteanalgebraicversionoftheresult.
19.
15Proposition.
TheρnformabasisofthepolynomialfunctionsonU(1)R2.
Indeed,ontheunitcircle,z=z1sothetheFourierpolynomialsarealsothepolynomialsinzandz,whichcanalsobeexpressedaspolynomialsinx,y.
(19.
16)Digression:FourierseriesTheFouriermodesρnformacompleteorthonormalset(orthonormalbasis)oftheHilbertspaceL2(U(1)).
Thismeansthateverysquare-integrablefunctionf∈L2hasa(Fourier)seriesexpansionf(θ)=∞∞fn·einθ=∞∞fn·ρn,(*)whichconvergesinmeansquare;theFouriercoecientsfnaregivenbytheformulafn=ρn|f=12π2π0einθf(θ)dθ.
(**)Recallthatmean-squareconvergencesigniesthatthepartialsumsapproachf,inthedistancedenedbytheinnerproduct.
Theproofofmostofthisisfairlyeasy.
OrthonormalityoftheρnimpliestheFourierexpansionformulafortheFourierpolynomials,thenitelinearcombinationsoftheρn.
(Ofcourse,inthiscasetheFourierseriesisanitesum,andnoanalysisisneeded.
)Furthermore,foranygivennitecollectionSofindexesn,andforanyf∈L2,thesumoftermsin(*)withn∈Sistheorthogonalprojectionoffontothethespanoftheρn,n∈S.
Hence,anynitesumofnormsoftheFouriercoecientsfn2in(*)isboundedbyf2,andtheFourierseriesconverges.
Moreover,thelimitghasthepropertythatfgisorthogonaltoeachρn;inotherwords,gistheprojectionoffontothespanofalltheρn.
Themoredicultpartistoshowthatanyf∈L2whichisorthogonaltoallρnvanishes.
OnemethodistoderiveitfromapowerfultheoremofWeierstra',whichsaysthattheFourierpolynomialsaredenseinthespaceofcontinuousfunctions,inthesenseofuniformconvergence.
46(TheresultholdsforcontinuousfunctionsonanycompactsubsetofRN;here,N=2.
)Approx-imatingacandidatef,orthogonaltoallρn,byasequenceofFourierpolynomialspkleadstoacontradiction,becausefpk2=f2+pk2≥f2,byorthogonality,andyetuniformconvergencecertainlyimpliesconvergenceinmean-square.
Wesummarisethebasicfactsinthefollowing19.
17Theorem.
(i)AnycontinuousfunctiononU(1)canbeuniformlyapproximatedbynitelinearcombinationsoftheρn.
(ii)Anysquare-integrablefunctionf∈L2(U(1))hasaseriesexpansionf=fn·ρn,withFouriercoecientsfngivenby(**).
20ThegroupSU(2)WemoveontothegroupSU(2).
Wewilldescribeitsconjugacyclasses,ndthebi-invariantvolumeform,whoseexistenceimpliestheunitarisabilityandcompletereducibilityofitscon-tinuousnite-dimensionalrepresentations.
Inthenextlecture,wewilllisttheirreducibleswiththeircharacters.
Givingawaytheplot,observethatSU(2)actsonthespacePofpolynomialsintwovariablesz1,z2,byitsnaturallinearactiononthecoordinates.
WecandecomposePintothehomogeneouspiecesPn,n=0,1,2,preservedbytheactionofSU(2):thus,thespaceP0ofconstantsisthetrivialone-dimensionalrepresentation,thespaceP1oflinearfunctionsthestandard2-dimensionalone,etc.
Then,P0,P1,P2,.
.
.
isthecompletelistofirreduciblerepresentationsofSU(2),uptoisomorphism,andeverycontinuousnite-dimensionalrepresentationisisomorphictoadirectsumofthose.
(20.
1)SU(2)andthequaternionsBydenition,SU(2)isthegroupofcomplex2*2matricespreservingthecomplexinnerproductandwithdeterminant1;thegroupoperationismatrixmultiplication.
Explicitly,SU(2)=uvvu:u,v∈C,|u|2+|v|2=1.
Geometrically,thiscanbeidentiedwiththethree-dimensionalunitsphereinC2.
ItisusefultoreplaceC2withHamilton'squaternionsH,generatedoverRbyelementsi,jsatisfyingtherelationsi2=j2=1,ij=ji.
Thus,Hisfour-dimensional,spannedby1,i,jandk:=ij.
Theconjugateofaquaternionq=a+bi+cj+dkisq:=abicjdk,andthequaternionnormisq2=qq=qq=a2+b2+c2+d2.
Therelationq1q2=q2q1showsthat:H→Rismultiplicative,q1q2=q1q2·q1q2=q1q2·q2q1=q1q2inparticular,the"unitquaternions"(thequaternionsofunitnorm)formagroupundermulti-plication.
Directcalculationestablishesthefollowing.
20.
2Proposition.
Sendinguvvutoq=u+vjgivesanisomorphismofSU(2)withthemultiplicativegroupofunitquaternions.
47(20.
3)ConjugacyclassesAtheoremfromlinearalgebraassertsthatunitarymatricesarediagonalisableinanorthonormaleigenbasis.
Thediagonalisedmatrixisthenalsounitary,soitsdiagonalelementsarecomplexnumbersofunitnorm.
Thesenumbers,ofcourse,aretheeigenvaluesofthematrix.
Theyareonlydetermineduptoreordering.
WethereforegetabijectionofconjugacyclassesintheunitarygroupwithunorderedN-tuplesofcomplexnumbersofunitnorm:U(N)/U(N)U(1)N/SN,wherethesymmetricgroupSNactsbypermutingtheNfactorsofU(1)N.
ThemapfromrighttoleftisdenedbytheinclusionU(1)NU(N)andisthereforecontinuous;ageneraltheoremfromtopologyensuresthatitisinfactahomeomorphism.
7Clearly,restrictingtoSU(N)imposedthedet=1restrictionontherightsideU(1)N.
However,thereisapotentialprobleminsight,becauseitmighthappen,inprinciple,thattwomatricesinSU(N)willbeconjugateinU(N),butnotinSU(N).
ForageneralsituationofasubgroupHGthiswarrantsdeservescarefulconsideration.
Inthecaseathand,however,wearelucky.
ThescalarmatricesU(1)·IdU(N)arecentral,thatis,theirconjugationactionistrivial.
Now,foranymatrixA∈U(N)andanyNthrootrofdetA,wehaver1A∈SU(N),andconjugatingbythelatterhasthesameeectasconjugationbyA.
WethengetabijectionSU(N)/SU(N)SU(1)N/SN,whereU(1)NisidentiedwiththegroupofdiagonalunitaryN*NmatricesandtheSontheright,standingfor"special",indicatesitssubgroupofdeterminant1matrices.
20.
4Proposition.
Thenormalisedtrace12Tr:SU(2)→CgivesahomomorphismsofthesetofconjugacyclassesinSU(2)withtheinterval[1,1].
Thesetofconjugacyclassesisgiventhequotienttopology.
Proof.
Asdiscussed,matricesareconjugateinSU(2)itheireigenvaluesagreeuptoorder.
Theeigenvaluesformapair{z,z1},withzontheunitcircle,sotheyaretherootsofX2(z+z1)X+1,inwhichthemiddlecoecientrangesover[2,2].
Thequaternionpictureprovidesagoodgeometricmodelofthismap:thehalf-tracebecomestheprojectionq→aontherealaxisRH.
Theconjugacyclassesarethereforethe2-dimensionalspheresofconstantlatitudeontheunitsphere,plusthetwopoles,thesingletons{±1}.
Thelattercorrespondtothecentralelements±I2∈SU(2).
(20.
5)CharactersasLaurentpolynomialsTheprecedingdiscussionimpliesthatthecharactersofcontinuousSU(2)-representationsarecontinuousfunctionson[1,1].
Itwillbemoreprotable,however,toparametrisethatintervalbythe"latitude"φ∈[0,π],ratherthantherealpartcosφ.
Wecaninfactletφrangefrom[0,2π],providewerememberourfunctionsareinvariantunderφφandperiodicwithperiod2π.
WecallsuchfunctionsofφWeyl-invariant,oreven.
Functionswhichchangesignunderthatsamesymmetryareanti-invariantorodd.
7Weusethequotienttopologiesonbothsides.
TheresultIamalludingtoassertsthatacontinuousbijectionfromacompactspacetoaHausdorspaceisahomeomorphism.
48Noticethatφparametrisesthesubgroupofdiagonalmatrices,isomorphictoU(1),eiφ00eiφ=z00z1∈SU(2),andthetransformationφφisinducedbyconjugationbythematrix0ii0∈SU(2).
ThecharacterofanSU(2)-representationVwillbethefunctionofz∈U(1)χV(z)=TrVz00z1,invariantunderzz1.
Whenconvenient,weshallre-expressitintermsofφandabusivelywriteχV(φ).
ArepresentationofSU(2)restrictstooneofourU(1),andweknowfromlastlecturethatthecharactersofthelatterarepolynomialsinzandz1.
SuchfunctionsarecalledLaurentpolynomials.
Wethereforeobtainthe20.
6Proposition.
CharactersofSU(2)-representationsareevenLaurentpolynomialsinz.
(20.
7)ThevolumeformandWeyl'sIntegrationformulaforSU(2)Fornitegroups,averagingoverthegroupwasusedtoprovecompletereducibility,todenetheinnerproductofcharactersandprovetheorthogonalitytheorems.
Forcompactgroups(suchasU(1)),integrationoverthegroupmustbeusedinstead.
Inbothcases,theessentialpropertyoftheoperationisitsinvarianceunderleftandrighttranslationsonthegroup.
Tostatethismoreprecisely:thelinearfunctionalsendingacontinuousfunctiononGtoG(g)dgisinvariantunderleftandrighttranslationsof.
Asecondaryproperty(whichisarrangedbyappropriatescaling)isthattheaverageorintegraloftheconstantfunction1is1.
WethusneedavolumeformoverSU(2)whichisinvariantunderleftandrighttranslations.
OurgeometricmodelforSU(2)astheunitsphereinR4allowsustonditdirectly,withoutappealingtoHaar's(dicult)generalresult.
Note,indeed,thattheactionsofSU(2)onHbyleftandrightmultiplicationpreservethequaternionnorm,hencetheEuclideandistance.
Inparticular,theusualEuclideanvolumeelementontheunitspheremustbeinvariantunderbothleftandrightmultiplicationbySU(2)elements.
20.
8Remark.
Theright*leftactionofSU(2)*SU(2)onR4=H,wherebyα*βsendsq∈Htoαqβ1,givesahomomorphismh:SU(2)*SU(2)→SO(4).
(Theactionpreservesorientation,becausethegroupisconnected.
)Clearly,(Id,Id)actsastheidentity,sohfactorsthroughthequotientSU(2)*SU(2)/{±(Id,Id)},andindeedturnsouttogiveanisomorphismofthelatterwithSO(4).
WewilldiscussthisinLecture22.
Tounderstandtheinnerproductofcharacters,weareinterestedinintegratingclassfunctionsoverSU(2).
ThismustbeexpressibleintermsoftheirrestrictiontoU(1).
Thisismadeexplicitbythefollowingtheorem,whoseimportancecannotbeoverestimated:asweshallsee,itimpliesthecharacterformulaeforallirreduciblerepresentationsofSU(2).
LetdgbetheEuclideanvolumeformontheunitsphereinH,normalisedtototalvolume1,andlet(φ)=eiφeiφbetheWeyldenominator.
20.
9Theorem(WeylIntegrationFormula).
ForacontinuousclassfunctionfonSU(2),wehaveSU(2)f(g)dg=1212π2π0f(φ)·|(φ)|2dφ=1π2π0f(φ)sin2φdφ.
49Thus,theintegraloverSU(2)canbecomputedbyrestrictiontotheU(1)ofdiagonalmatrices,aftercorrectingthemeasurebythefactor12|(φ)|2.
Weareabusivelywritingf(φ)forthevalueoffateiφ00eiφ.
Proof.
InthepresentationofSU(2)astheunitsphereinH,thefunctionfbeingconstantonthespheresofconstantlatitude.
ThevolumeofasphericalsliceofwidthdφisC·sin2φdφ,withtheconstantCnormalisedbythe"totalvolume1"conditionπ0C·sin2φdφ=1,whenceC=2/π,inagreementwiththetheorem.
21IrreduciblecharactersofSU(2)Letuschecktheirreducibilityofsomerepresentations.
Thetrivial1-dimensionalrepresentationisobviouslyirreducible;itscharacterhasnorm1,duetoournormalisationofthemeasure.
ThecharacterofthestandardrepresentationonC2iseiφ+eiφ=2cosφ.
Itsnormis4π2π0cos2φ·sin2φdφ=1π2π0(2cosφsinφ)2dφ=1π2π0sin22φdφ=1,soC2isirreducible.
Ofcourse,thiscanalsobeseendirectly(noinvariantlines).
ThecharacterofthetensorsquareC2C2is4cos2φ,soitssquarenormis1π2π016cos4φsin2φdφ=1π2π04cos2φsin22φdφ=1π2π0(sin3φ+sinφ)2dφ=1π2π0sin23φ+sin2φ+2sinφsin3φdφ=1+1=2,sothisisreducible.
Indeed,wehave(C2)2=Sym2C2Λ2C2;thesemustbeirreducible.
Λ2C2isthetrivial1-dim.
representation,sinceitscharacteris1(theproductoftheeigen-values),andsoSym2C2isanew,3-dimensionalirreduciblerepresentation,withcharacter2cos2φ+1=z2+z2+1.
SofarwehavefoundtheirreduciblerepresentationsC=Sym0C2,C2=Sym1C2,Sym2C2,withcharacters1,z+z1,z2+1+z2.
Thissurelylendscredibilitytothefollowing.
21.
1Theorem.
ThecharacterχnofSymnC2iszn+zn2z2n+zn.
Itsnormis1,andsoeachSymnC2isanirreduciblerepresentationofSU(2).
Moreover,thisisthecompletelistofirreducibles,asn≥0.
21.
2Remark.
NotethatSymnC2hasdimensionn+1.
50Proof.
Thestandardbasisvectorse1,e2scaleunderg=z00z1bythefactorsz±1.
Now,thebasisvectorsofSymnC2arisebysymmetrisingthevectorsen1,e(n1)1e2,en2inthenthtensorpower(C2)n.
Thevectorsappearinginthesymmetrisationofep1e(np)2aretensorproductscontainingpfactorsofe1andnpfactorsofe2,insomeorder;eachoftheseisaneigenvectorsforg,witheigenvaluezp·znp.
Hence,theeigenvaluesofgonSymnare{zn,zn2,zn}andthecharacterformulafollows.
Wehaveχn(z)=zn+1zn1zz1,whenceχn(z)|2|(z)|2=|zn+1zn1|2=4sin2[(n+1)φ].
Thus,χn2=14π2π04sin2[(n+1)φ]dφ=1π·π=1,provingirreducibility.
Toseecompleteness,observethatthefunctions(z)χn(z)spanthevectorspaceofoddLaurentpolynomials(§20.
5).
Foranycharacterχ,thefunctionχ(z)(z)isanoddLaurentpolynomial.
Ifχwasanewirreduciblecharacter,χ(z)(z)wouldhavetobeorthogonaltoallanti-invariantLaurentpolynomials,inthestandardinnerproductonthecircle.
(Combinedwiththeweight||2,thisisthecorrectSU(2)innerproductuptoscale,aspertheintegrationformula).
Butthisisimpossible,astheorthogonalcomplementoftheoddpolynomialsisspannedbytheevenones.
(21.
3)AnotherlookattheirreduciblesThegroupSU(2)actslinearlyonthevectorspacePofpolynomialsinz1,z2bytransformingthevariablesinthestandardway,uvvu·z1z2=uz1+vz2vz1+uz2.
ThispreservesthedecompositionofPasthedirectsumofthespacesPnofhomogeneouspolynomialsofdegreen.
21.
4Proposition.
AsSU(2)-representations,SymnC2=Pn,thespaceofhomogeneouspoly-nomialsofdegreenintwovariables.
Proof.
AnisomorphismfromPntoSymnC2isdenedbysendingzp1znp2tothesymmetrisationofep1e(np)2.
Bylinearity,thiswillsend(uz1+vz2)p·(vz1+uz2)nptothesymmetrisationof(ue1+ve2)p(ve1+ue2)(np).
ThisshowsthatourisomorphismcommuteswiththeSU(2)-action.
21.
5Remark.
Equalityoftherepresentationsuptoisomorphismcanalsobeseenbycheckingthecharacterofadiagonalmatrixg,forwhichthezp1znp2areeigenvectors.
(21.
6)TensorproductofrepresentationsKnowledgeofthecharactersallowsustondthedecompositionoftensorproducts.
Obviously,tensoringwithCdoesnotchangearepresentation.
Thenextexampleisχ1·χn=(z+z1)zn+1zn1zz1=zn+2+znznz2nzz1=χn+1+χn1,providedn≥1;ifn=0ofcoursetheanswerisχ1.
Moregenerally,wehave5121.
7Theorem.
Ifp≥q,χp·χq=χp+q+χp+q2χpq.
Hence,SympC2SymqC2=qk=0Symp+q2kC2.
Proof.
χp(z)·χq(z)=zp+1zp1zz1·zq+zq2zq=qk=0zp+q+12kz2kpq1zz1=qk=0χp+q2k;theconditionp≥qensuresthattherearenocancellationsinthesum.
21.
8Remark.
Letusdeneformallyχn=χn2,a"virtualcharacter".
Thus,χ0=1,thetrivialcharacter,χ1=0andχ2=1.
Thedecompositionformulabecomesχp·χq=χp+q+χp+q2χpq,regardlesswhetherp≥qornot.
(21.
9)MultiplicitiesThemultiplicityoftheirreduciblerepresentationSymninarepresentationwithcharacterχ(z)canbecomputedastheinnerproductχ|χn,withtheintegration(20.
9).
Thefollowingrecipe,whichexploitsthesimpleformofthefunctions(z)χn(z)=zn+1zn1,issometimeshelpful.
21.
10Proposition.
χ|χnisthecoecientofzn+1in(z)χ(z).
22SomeSU(2)-relatedgroupsWenowdiscusstwogroupscloselyrelatedtoSU(2)anddescribetheirirreduciblerepresentationsandcharacters.
TheseareSO(3),SO(4)andU(2).
Westartwiththefollowing22.
1Proposition.
SO(3)=SU(2)/{±Id},SO(4)=SU(2)*SU(2)/{±(Id,Id)}andU(2)=U(1)*SU(2)/{±(Id,Id)}.
Proof.
Recalltheleft*rightmultiplicationactionofSU(2),viewedasthespaceofunitnormquaternions,onH=R4.
ThisgivesahomomorphismfromSU(2)*SU(2)→SO(4).
Notethatα*βsend1∈Htoαβ1,soα*βxes1iα=β.
Thepairα*αxeseveryotherquaternioniαiscentralinSU(2),thatis,α=±Id.
Sothekernelofourhomomorphismis{±(Id,Id)}.
WeseesurjectivityandconstructtheisomorphismSU(2)→SO(3)atthesametime.
Re-strictingourleft*rightactiontothediagonalcopyofSU(2)leadstotheconjugationactionofSU(2)onthespaceofpurequaternions,spannedbyi,j,k.
IclaimthatthisgeneratesthefullrotationgroupSO(3):indeed,rotationsinthei,j-planeareimplementedbyelementsa+bk,andsimilarlywithanypermutationofi,j,k,andtheserotationsgenerateSO(3).
ThisconstructsasurjectivehomomorphismfromSU(2)toSO(3),butwealreadyknowthatthekernelis{±Id}.
SowehavetheassertionaboutSO(3).
ReturningtoSO(4),wecantakeanyorthonormal,purequaternionframetoanyotheronebyaconjugation.
Wecanalsotake1∈Htoanyotherunitvectorbyaleftmultiplication.
52Combiningthese,itfollowsthatwecantakeanyorthonormal4-frametoanyotheronebyasuitableconjugation,followedbyaleftmultiplication.
Finally,theassertionaboutU(2)isclear,asbothU(1)andSU(2)sitnaturallyinU(2)(theformerasthescalarmatrices),andintersectat{±Id}.
Thegroupisomorphismsinthepropositionareinfacthomeomorphisms;thatmeans,theinversemapiscontinuous(usingthequotienttopologyonthequotientgroups).
Itisnotdicult(althoughabitpainful)toprovethisdirectlyfromtheconstruction,butthisfollowsmoreeasilyfromthefollowingtopologicalfactwhichisofindependentinterest.
22.
2Proposition.
AnycontinuousbijectionfromaHausdorspacetoacompactspaceisahomeomorphism.
(22.
3)RepresentationsItfollowsthatcontinuousrepresentationsofthethreegroupsinProposition22.
1arethesameascontinuousrepresentationsofSU(2),SU(2)*SU(2)andSU(2)*U(1),respectively,whichsendId,(Id,Id)and(Id,Id)totheidentitymatrix.
22.
4Corollary.
ThecompletelistofirreduciblerepresentationsofSO(3)isSym2nC2,asn≥0.
Thisformulationisslightlyabusive,asC2itselfisnotarepresentationofSO(3)butonlyofSU(2)(IdactsasId).
Butthesignproblemisxedonallevensymmetricpowers.
Forexample,Sym2C2isthestandard3-dimensionalrepresentationofSO(3).
(Thereislittlechoiceaboutit,asitistheonly3-dimensionalrepresentationonthelist).
Theimageofourcopy{diag[z,z1]}SU(2)ofU(1),inourhomomorphism,isthefamilyofmatrices1000cossin0sincos,with(caution!
)=2φ.
ThesecoveralltheconjugacyclassesinSO(3),andtheirreduciblecharactersofSO(3)restricttothisas1+2cos+2cos(2)2cos(n).
(22.
5)RepresentationsofaproductHandlingtheothertwogroups,SO(4)andU(2),requiresthefollowing22.
6Lemma.
FortheproductG*HoftwocompactgroupsGandH,thecompletelistofirreduciblerepresentationsconsistsofthetensorproductsVW,asVandWrangeovertheirreduciblesofGandH,independently.
Proofusingcharactertheory.
Fromthepropertiesofthetensorproductofmatrices,itfollowsthatthecharacterofVWattheelementg*hisχVW(g*h)=χV(g)·χW(h).
Now,aconjugacyclassinG*HisaCartesianproductofconjugacyclassesinGandH,andcharactertheoryensuresthattheχVandχWformHilbertspacebasesoftheL2classfunctionsonthetwogroups.
ItfollowsthattheχV(g)·χW(h)formaHilbertspacebasisoftheclassfunctionsonG*H,sothisisacompletelistofirreduciblecharacters.
Incidentally,theproofestablishesthecompletenessofcharactersfortheproductG*H,ifitwasknownonthefactors.
So,givenourknowledgeofU(1)andSU(2),itdoesgiveacompleteargument.
However,wealsoindicateanotherproofwhichdoesnotrelyoncharactertheory,butusescompletereducibilityinstead.
TheadvantageisthatweonlyneedtoknowcompletereducibilityunderoneofthefactorsG,H.
53Proofwithoutcharacters.
LetUbeanirreduciblerepresentationofG*H,anddecomposeitintoisotypicalcomponentsUiundertheactionofH.
BecausetheactionofGcommuteswithH,itmustbeblock-diagonalinthisdecomposition,andirreducibilityimpliesthatwehaveasingleblock.
LetthenWbetheuniqueirreducibleH-typeappearinginU,andletV:=HomH(W,U).
ThisinheritsandactionofGfromU,andwehaveanisomorphismU=WHomH(W,U)=VW(seeExampleSheet2),withGandHactingonthetwofactors.
Moreover,anyproperG-subrepresentationofVwouldleadtoaproperG*H-subrepresentationofU,andthisimpliesirreducibilityofV.
22.
7Corollary.
ThecompletelistofirreduciblerepresentationsofSO(4)isSymmC2SymnC2,withthetwoSU(2)factorsactingonthetwoSymfactors.
Here,m,n≥0andm=nmod2.
(22.
8)AcloserlookatU(2)ListingtherepresentationsofU(2)requiresacomment.
AsU(2)=U(1)*SU(2)/{±(Id,Id)},inprincipleweshouldtensortogetherpairsofrepresentationsofU(1)andSU(2)ofmatchingparity,sothat(Id,Id)actsas+Idintheproduct.
However,observethatU(2)actsnaturallyonC2,extendingtheSU(2)action,sotheactionofSU(2)onSymnC2alsoextendstoallofU(2).
Thereunder,thefactorU(1)ofscalarmatricesdoesnotacttrivially,butratherbythenthpowerofthenaturalrepresentation(sinceitactsnaturallyonC2).
Inaddition,U(2)hasa1-dimensionalrepresentationdet:U(2)→U(1).
Denotebydetmitsmthtensorpower;itrestrictstothetrivialrepresentationonSU(2)andtothe2mthpowerofthenaturalrepresentationonthescalarsubgroupU(1).
TheclassicationofrepresentationsofU(2)intermsoftheirrestrictionstothesubgroupsU(1)andSU(2)covertsthenintothe22.
9Proposition.
ThecompletelistofirreduciblerepresentationsofU(2)isdetmSymnC2,withm,n∈Z,n≥0.
(22.
10)CharactersofU(2)WesawearlierthattheconjugacyclassesofU(2)werelabelledbyunorderedpairs{z1,z2}ofeigenvalues.
Thesearedouble-coveredbythesubgroupofdiagonalmatricesdiag[z1,z2].
ThetraceofthismatrixonSymnC2iszn1+zn11z2+···+zn2,andsothecharacterofdetmSymnC2isthesymmetric8Laurentpolynomialzm+n1zm2+zm+n11zm+12zm1zm+n2.
(22.
11)Moreover,thesearealltheirreduciblecharactersofU(2).
Clearly,theyspanthespaceofsymmetricLaurentpolynomials.
GeneraltheorytellsusthattheyshouldformaHilbertspacebasisofthespaceofclassfunctions,withrespecttotheU(2)innerproduct;butwecancheckthisdirectlyfromthe22.
12Proposition(WeylintegrationformulaforU(2)).
ForaclassfunctionfonU(2),wehaveU(2)f(u)du=1214π22π*2π0*0f(φ1,φ2)·|eiφ1eiφ2|2dφ1dφ2.
Here,f(φ1,φ2)abusivelydenotesf(diag[eiφ1,eiφ2]).
Wecall(z1,z2):=z1z2=eiφ1eiφ2theWeyldenominatorforU(2).
ThepropositionisprovedbyliftingftothedoublecoverU(1)*SU(2)ofU(2)andapplyingtheintegrationformulaforSU(2);weomitthedetails.
Armedwiththeintegrationformula,wecouldhavediscoveredthecharacterformulae(22.
11)apriori,beforeconstructingtherepresentations.
Ofcourse,provingthatallformulaeareactuallyrealisedascharactersrequireseitheradirectconstruction(asgivenhere)orageneralexistenceargument,assecuredbythePeter-Weyltheorem.
8Undertheswitchz1z2.
5423TheunitarygroupTherepresentationtheoryofthegeneralunitarygroupsU(N)isverysimilartothatofU(2),withtheobviouschangethatthecharactersarenowsymmetricLaurentpolynomialsinNvariables,withintegercoecients;thevariablesrepresenttheeigenvaluesofaunitarymatrix.
Thesearepolynomialsintheziandz1iwhichareinvariantunderpermutationofthevariables.
Anysuchpolynomialcanbeexpressedas(z1z2···zN)n·f(z1,zN),wherefisagenuinesymmetricpolynomialwithintegercoecients.
ThefactthatallU(N)charactersareofthisformfollowsfromtheclassicationofconjugacyclassesinLecture20,andtherepresentationtheoryofthesubgroupU(1)N:representationsofthelatterarecompletelyreducible,withtheirreduciblebeingtensorproductsofNone-dimensionalrepresentationsofthefactors(Lemma22.
6).
(23.
1)Symmetryvs.
anti-symmetryLetusintroducesomenotation.
ForanN-tupleλ=λ1,λNofintegers,denotebyzλthe(Laurent)monomialzλ11zλ22···zλNN.
Givenσ∈SN,thesymmetricgrouponNletters,zσ(λ)willdenotethemonomialforthepermutedN-tupleσ(λ).
ThereisadistinguishedN-tupleδ=(N1,N2,0).
Theanti-symmetricLaurentpolynomialsaλ(z):=σ∈SNε(σ)·zσ(λ),whereε(σ)denotesthesignatureofσ,aredistinguishedbythefollowingsimpleobservation:23.
2Proposition.
AsλrangesoverthedecreasinglyorderedN-tuples,λ1≥λ2λN,theaλ+δformaZ-basisoftheintegralanti-symmetricLaurentpolynomials.
Thepolynomialaδ(z)isspecial,asthefollowingidentitiesshow:aδ(z)=det[zNqp]=p0,andtheyformabasisofthespaceofintegralsymmetricpolynomials.
Ourmaintheoremisnow23.
8Theorem.
TheSchurfunctionsarepreciselytheirreduciblecharactersofU(N).
FollowingthemodelofSU(2)intheearliersectionsandthegeneralorthogonalitytheoryofcharacters,thetheorembreaksupintotwostatements.
23.
9Proposition.
TheSchurfunctionsareorthonormalintheinnerproductdenedbyinte-grationoverU(N),withrespecttothenormalisedinvariantmeasure.
23.
10Proposition.
EverysλisthecharacterofsomerepresentationofU(N).
WeshallnotproveProposition23.
9here;asinthecaseofSU(2),itreducestothefollowingintegrationformula,whoseproof,however,isnowmoredicult,duetotheabsenceofaconcretegeometricmodelforU(N)withitsinvariantmeasure.
23.
11Theorem(WeylIntegrationforU(N)).
ForaclassfunctionfonU(N),wehaveU(N)f(u)du=1N!
1(2π)N2π0···2π0f(φ1,φN)·|(z)|2dφ1···dφN.
Wehaveusedthestandardconventionf(φ1,φN)=f(diag[z1,zN])andzp=eiφp.
(23.
12)ConstructionofrepresentationsMuchlikeinthecaseofSU(2),theirrepsofU(N)canbefoundintermsofthetensorpowersofitsstandardrepresentationonCN.
Formulatingthispreciselyrequiresacomment.
ArepresentationofU(N)willbecalledpolynomialiitscharacterisagenuine(asopposedtoLaurent)polynomialinthezp.
Forexample,thedeterminantrepresentationu→detuispolynomial,asitscharacterisz1···zN,butitsdualrepresentationhascharacter(z1···zN)1andisnotpolynomial.
23.
13Remark.
Asymmetricpolynomialinthezpisexpressibleintermsoftheelementarysymmetricpolynomials.
Ifthezparetheeigenvaluesofamatrixu,thesearethecoecientsofthecharacteristicpolynomialofu.
Assuch,theyhavepolynomialexpressionsintermsoftheentriesofu.
Thus,inanypolynomialrepresentationρ,thetraceofρ(u)isapolynomialintheentriesofu∈U(N).
Itturnsoutinfactthatallentriesofthematrixρ(u)arepolynomiallyexpressibleintermsofthoseofu,withoutinvolvingtheentriesofu1.
Now,thecharacterof(CN)disthepolynomial(z1zN)d,anditfollowsfromourdiscussionofSchurfunctionsandpolynomialsthatanyrepresentationofU(N)appearingintheirreducibledecompositionof(CN)dispolynomial.
ThekeystepinprovingtheexistenceofrepresentationnowconsistsinshowingthateverySchurpolynomialappearsintheirreducibledecompositionof(CN)d,forsomed.
ThisimpliesthatallSchurpolynomialsarecharactersofrepresentations.
However,anySchurfunctionconvertsintoaSchurpolynomialuponmulti-plicationbyalargepowerofdet;sothisdoesimplythatallSchurfunctionsarecharactersofU(N)-representations(apolynomialrepresentationtensoredwithalargeinversepowerofdet).
56NotethattheSchurfunctionsλishomogeneousofdegree|λ|=λ1+···+λN;soifitappearsinany(CN)d,itmustdosoford=|λ|.
Checkingitspresencerequiresusonlytoshowthatsλ|(z1zN)|λ|>0intheinnerproduct(23.
11).
Whilethereisareasonablysimpleargumentforthis,itturnsoutthatwecan(andwill)domuchmorewithlittleextraeort.
(23.
14)Schur-WeyldualityTostatethekeytheorem,observethatthespace(CN)dcarriesanactionofthesymmetricgroupSdondletters,whichpermutesthefactorsineveryvectorv1v2···vd.
Clearly,thiscommuteswiththeactionofU(N),inwhichamatrixu∈U(N)transformsalltensorfactorssimultaneously.
Thisway,(CN)dbecomesarepresentationoftheproductgroupU(N)*Sd.
23.
15Theorem(Schur-Weylduality).
UndertheactionofU(N)*Sd,(CN)ddecomposesasadirectsumofproductsλVλSλlabelledbypartitionsofdwithnomorethanNparts,inwhichVλistheirreduciblerepresentationofU(N)withcharactersλandSλisanirreduciblerepresentationofSd.
TherepresentationsSλ,forvariousλ's,dependonlyonλ(andnotonN),arepairwisenon-isomorphic.
ForN≥dtheyexhaustallirreduciblerepresentationsofSd.
Recallthatapartitionofdwithnpartsisasequenceλ1≥λ2≥.
.
.
λn>0ofintegerssummingtod.
OnceN≥d,allpartitionsofdarerepresentedinthesum.
AsthenumberofconjugacyclassesinSdequalsthenumberofpartitions,thelastsentenceinthetheoremisobvious.
Nothingelseinthetheoremisobvious;itwillbeprovedinthenextsection.
24ThesymmetricgroupandSchur-Weylduality(24.
1)ConjugacyclassedinthesymmetricgroupThedualitytheoremrelatestherepresentationsoftheunitarygroupsU(N)tothoseofthesymmetricgroupsSd,sowemustsayawordaboutthelatter.
Recallthateverypermutationhasauniquedecompositionasaproductofdisjointcycles,andthattwopermutationsareconjugateifandonlyiftheyhavethesamecycletype,whichisthecollectionofallcyclelengths,withmultiplicities.
Orderingthelengthsdecreasinglyleadstoapartition=1n>0ofd.
Assumethatthenumbers1,2,doccurm1,m2,mdtimes,respectivelyin;thatis,mkisthenumberofk-cyclesinourpermutation.
Weshallalsorefertothecycletypebythenotation(m).
Thus,theconjugacyclassesinSdarelabelledby(m)'ssuchthatkk·mk=d.
ItfollowsthatthenumberofirreduciblecharactersofSdisthenumberofpartitionsofd.
Whatisalsotruebutunexpectedisthatthereisanaturalwaytoassignirreduciblerepresentationstopartitions,asweshallexplain.
24.
2Proposition.
Theconjugacyclassofcycletype(m)hasorderd!
mk!
·kmk.
Proof.
Bytheorbit-stabilisertheorem,appliedtotheconjugationactionofSdonitself,itsucestoshowthatthecentraliserofapermutationofcycletype(m)ismk!
·kmk.
Butanelementofthecentralisermustpermutethek-cycles,forallk,whilepreservingthecyclicorderoftheelementswithin.
Wegetafactorofmk!
fromthepermutationsofthemkk-cycles,andkmkfromindependentcyclicpermutationswithineachk-cycle.
57(24.
3)TheirreduciblecharactersofSdChooseN>0anddenotebyporp(m)theproductofpowersumsp(z)=k(zk1+zk2zkN)=k>0(zk1zkN)mk;notethatifwearetoallowanumberoftrailingzeroesattheendof,theymustbeignoredintherstproduct.
ForanypartitionλofdwithnomorethanNparts,wedeneafunctiononconjugacyclassesofSdbyωλ(m)=coecientofzλ+δinp(m)(z)·(z).
Itisimportanttoobservethatωλ(m)doesnotdependonN,providedthelatterislargerthanthenumberofpartsofλ;indeed,anextravariablezN+1canbesettozero,p(m)isthenunchanged,whileboth(z)andzλ+δacquireafactorofz1z2···zN,leadingtothesamecoecientinthedenition.
Anti-symmetryofp(m)(z)·(z)andthedenitionoftheSchurfunctionsleadtothefollowing24.
4Proposition.
p(m)(z)=λωλ(m)·sλ(z),thesumrunningoverthepartitionsofdwithnomorethanNparts.
Indeed,thisisequivalenttotheidentityp(m)(z)·(z)=λωλ(m)·aλ+δ(z),whichjustrestatesthedenitionoftheωλ.
24.
5Theorem(Frobeniuscharacterformula).
TheωλaretheirreduciblecharactersofthesymmetricgroupSd.
WewillprovethattheωλareorthonormalintheinnerproductofclassfunctionsonSd.
WewillthenshowthattheyarecharactersofrepresentationsofSd,whichwillimplytheirirreducibility.
ThesecondpartwillbeprovedinconjunctionwithSchur-Weylduality.
Proofoforthonormality.
Wemustshowthatforanypartitionsλ,ν,(m)ωλ(m)·ων(m)mk!
·kmk=δλν.
(24.
6)Wewillprovetheseidentitiessimultaneouslyforalld,viatheidentity(m);λ,νωλ(m)·ων(m)mk!
·kmksλ(z)sν(w)≡λsλ(z)sλ(w)(24.
7)forvariablesz,w,with(m)rangingoverallcycletypesofallsymmetricgroupsSdandλ,νrangingoverallpartitionswithnomorethanNpartsofallintegersd.
IndependenceoftheSchurpolynomialsimplies(24.
6).
FromProposition24.
4,theleftsidein(24.
7)is(m)p(m)(z)·p(m)(w)mk!
·kmk,58whichisalso(m)k>0(zk1zkN)mk(wk1wkN)mkmk!
·kmk=(m)k>0(p,q(zpwq)k/k)mkmk!
=k>0expp,q(zpwq)k/k=expp,q;k(zpwq)k/k=expp,qlog(1zpwq)=p,q(1zpwq)1.
Wearethusreducedtoprovingtheidentityλsλ(z)sλ(w)=p,q(1zpwq)1.
(24.
8)Thereare(atleast)twowaystoproceed.
Wecanmultiplybothsidesby(z)(w)andshowthateachsideagreeswiththeN*NCauchydeterminantdet[(1zpwq)1].
Theequalitydet[(1zpwq)1]=(z)(w)p,q(1zpwq)1canbeprovedbycleverrowoperationsandinductiononN.
(SeeAppendixAofFulton-Harris,RepresentationTheory,forhelpifneeded.
)Ontheotherhand,ifweexpandeachentryinthematrixinageometricseries,multi-linearityofthedeterminantgivesdet[(1zpwq)1]=l1,.
.
.
,lN≥0det[(zpwq)lq]=l1,.
.
.
,lN≥0det[zlqp]·qwlqq=l1,.
.
.
,lN≥0al(z)wl=l1>l2>···>lN≥0al(z)al(w),thelastidentityfromtheanti-symmetryinloftheal.
Theresultistheleftsideof(24.
8)multipliedby(z)(w),asdesired.
Anotherproofof(24.
8)canbegivenbyexploitingtheorthogonalitypropertiesofSchurfunctionsandajudicioususeofCauchy'sintegrationformula.
Indeed,theleft-handsideΣ(z,w)oftheequationhasthepropertythat1N!
···Σ(z,w1)f(w)(w)(w1)dw12πiw1···dwN2πiwN=f(z)foranysymmetricpolynomialf(w),whileanyhomogeneoussymmetricLaurentpolynomialcontainingnegativepowersofthewqintegratestozero.
(Theintegralsareovertheunitcircles.
)AnN-foldapplicationofCauchy'sformulashowsthatthesameistrueforp,q(1zpw1q)1,thefunctionobtainedfromtheright-handsideof(24.
8)aftersubstitutingwqw1q,subjecttotheconvergencecondition|zp|0(zk1zkN)mk=p(m)(z),andourpropositionnowfollowsfromProp.
24.
4.
60
- cancellingxvm相关文档
- Incipientxvm
- HBKxvm
- provinciaxvm
- fiihrtxvm
- JUDICIALxvm
- resuitLinkxvm
恒创科技SonderCloud,美国VPS综合性能测评报告,美国洛杉矶机房,CN2+BGP优质线路,2核4G内存10Mbps带宽,适用于稳定建站业务需求
最近主机参考拿到了一台恒创科技的美国VPS云服务器测试机器,那具体恒创科技美国云服务器性能到底怎么样呢?主机参考进行了一番VPS测评,大家可以参考一下,总体来说还是非常不错的,是值得购买的。非常适用于稳定建站业务需求。恒创科技服务器怎么样?恒创科技服务器好不好?henghost怎么样?henghost值不值得购买?SonderCloud服务器好不好?恒创科技henghost值不值得购买?恒创科技是...
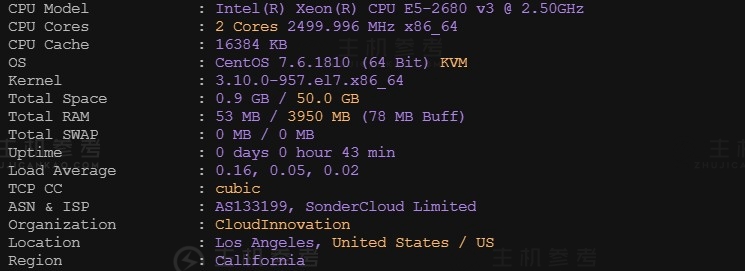
Sharktech10Gbps带宽,不限制流量,自带5个IPv4,100G防御
Sharktech荷兰10G带宽的独立服务器月付319美元起,10Gbps共享带宽,不限制流量,自带5个IPv4,免费60Gbps的 DDoS防御,可加到100G防御。CPU内存HDD价格购买地址E3-1270v216G2T$319/月链接E3-1270v516G2T$329/月链接2*E5-2670v232G2T$389/月链接2*E5-2678v364G2T$409/月链接这里我们需要注意,默...
月神科技:香港CN2/洛杉矶CN2/华中电信高防vps,月付20元起
月神科技怎么样?月神科技是由江西月神科技有限公司运营的一家自营云产品的IDC服务商,提供香港安畅、香港沙田、美国CERA、华中电信等机房资源,月神科技有自己的用户群和拥有创宇认证,并且也有电商企业将业务架设在月神科技的平台上。目前,香港CN2云服务器、洛杉矶CN2云主机、华中电信高防vps,月付20元起。点击进入:月神科技官方网站地址月神科技vps优惠信息:香港安畅CN2-GIA低至20元核心:2...

xvm为你推荐
-
盗版win8.1升级win10安装盗版windows10系统后怎么安装正版涡轮增压和自然吸气哪个好自然吸气与涡轮增压发动机哪个更好天气预报哪个好用哪个最准确分小时的那种天气预报app,哪个准确方便使用麒麟990和骁龙865哪个好5G手机芯片高通865对比麒麟990 5G SOC哪个好?红茶和绿茶哪个好红茶和绿茶,哪个好?播放器哪个好播放器哪个好用行车记录仪哪个好行车记录仪什么牌子好qq空间登录网址开通QZONe电信10000宽带测速怎样测试电信宽带的网速? 771212360云盘登陆首页怎样进入360的网络硬盘