linearwww.765.com
www.765.com 时间:2021-03-19 阅读:()
Yaoetal.
JournalofInequalitiesandApplications2014,2014:206http://www.
journalofinequalitiesandapplications.
com/content/2014/1/206RESEARCHOpenAccessConstructionofminimum-normxedpointsofpseudocontractionsinHilbertspacesYonghongYao1,GiuseppeMarino2,Hong-KunXu3andYeong-ChengLiou4,5**Correspondence:simplex_liou@hotmail.
com4DepartmentofInformationManagement,ChengShiuUniversity,Kaohsiung,833,Taiwan5CenterforGeneralEducation,KaohsiungMedicalUniversity,Kaohsiung,807,TaiwanFulllistofauthorinformationisavailableattheendofthearticleAbstractAniterativealgorithmisintroducedfortheconstructionoftheminimum-normxedpointofapseudocontractiononaHilbertspace.
Thealgorithmisprovedtobestronglyconvergent.
MSC:47H05;47H10;47H17Keywords:xedpoint;minimum-norm;pseudocontraction;nonexpansivemapping;projection1IntroductionConstructionofxedpointsofnonlinearmappingsisaclassicalandactiveareaofnonlin-earfunctionalanalysisduetothefactthatmanynonlinearproblemscanbereformulatedasxedpointequationsofnonlinearmappings.
TheresearchofthisareadatesbacktoPi-card'sandBanach'stime.
Asamatteroffact,thewell-knownBanachcontractionprinciplestatesthatthePicarditerates{Tnx}convergetotheuniquexedpointofTwheneverTisacontractionofacompletemetricspace.
However,ifTisnotacontraction(nonexpan-sive,say),thenthePicarditerates{Tnx}fail,ingeneral,toconverge;hence,otheriterativemethodsareneeded.
In,Mann[]introducedthenowcalledMann'siterativemethodwhichgeneratesasequence{xn}viatheaveragedalgorithmxn+=(–αn)xn+αnTxn,n≥,(.
)where{αn}isasequenceintheunitinterval[,],Tisaself-mappingofaclosedconvexsubsetCofaHilbertspaceH,andtheinitialguessxisanarbitrary(butxed)pointofC.
Mann'salgorithm(.
)hasextensivelybeenstudied[–],andinparticular,itisknownthatifTisnonexpansive(i.
e.
,Tx–Ty≤x–yforallx,y∈C)andifThasaxedpoint,thenthesequence{xn}generatedbyMann'salgorithm(.
)convergesweaklytoaxedpointofTprovidedthesequence{αn}satisesthecondition∞n=αn(–αn)=∞.
(.
)Thisalgorithm,however,doesnotconvergeinthestrongtopologyingeneral(see[,Corollary.
]).
2014Yaoetal.
;licenseeSpringer.
ThisisanOpenAccessarticledistributedunderthetermsoftheCreativeCommonsAttribu-tionLicense(http://creativecommons.
org/licenses/by/2.
0),whichpermitsunrestricteduse,distribution,andreproductioninanymedium,providedtheoriginalworkisproperlycited.
Yaoetal.
JournalofInequalitiesandApplications2014,2014:206Page2of14http://www.
journalofinequalitiesandapplications.
com/content/2014/1/206BrowderandPetryshyn[]studiedweakconvergenceofMann'salgorithm(.
)fortheclassofstrictpseudocontractions(inthecaseofconstantstepsizesαn=αforalln;see[]forthegeneralcaseofvariablestepsizes).
However,Mann'salgorithmfailstoconvergeforLipschitzianpseudocontractions(seethecounterexampleofChidumeandMutan-gadura[]).
ItisthereforeaninterestingquestionofinventingiterativealgorithmswhichgenerateasequenceconverginginthenormtopologytoaxedpointofaLipschitzianpseudocontraction(ifany).
Theinterestofpseudocontractionsliesintheirconnectionwithmonotoneoperators;namely,TisapseudocontractionifandonlyifthecomplementI–Tisamonotoneoperator.
Wealsonoticethatitisquiteusualtoseekaparticularsolutionofagivennonlinearproblem,inparticular,theminimum-normsolution.
Forinstance,givenaclosedconvexsubsetCofaHilbertspaceHandaboundedlinearoperatorA:H→H,whereHisanotherHilbertspace.
TheC-constrainedpseudoinverseofA,ACisthendenedastheminimum-normsolutionoftheconstrainedminimizationproblemAC(b):=argminx∈CAx–b(.
)whichisequivalenttothexedpointproblemx=PCx–λA(Ax–b),(.
)wherePCisthemetricprojectionfromHontoC,AistheadjointofA,λ>isaconstant,andb∈HissuchthatPA(C)(b)∈A(C).
Itisthereforeaninterestingproblemtoinventiterativealgorithmsthatcangeneratesequenceswhichconvergestronglytotheminimum-normsolutionofagivenxedpointproblem.
Thepurposeofthispaperistosolvesuchaproblemforpseudocontractions.
Moreprecisely,weshallintroduceaniterativealgorithmfortheconstructionofxedpointsofLipschitzianpseudocontractionsandprovethatouralgorithm(see(.
)inSec-tion)convergesinthestrongtopologytotheminimum-normxedpointofthemapping.
Fortheexistingliteratureoniterativemethodsforpseudocontractions,thereadercanconsult[,–];forndingminimum-normsolutionsofnonlinearxedpointandvariationalinequalityproblems,see[–];andforrelatediterativemethodsfornonex-pansivemappings,see[,,,]andthereferencestherein.
2PreliminariesLetHbearealHilbertspacewiththeinnerproduct·,·andthenorm·,respectively.
LetCbeanonemptyclosedconvexsubsetofH.
Theclassofnonlinearmappingswhichwewillstudyistheclassofpseudocontractions.
RecallthatamappingT:C→CisapseudocontractionifitsatisesthepropertyTx–Ty,x–y≤x–y,x,y∈C.
(.
)ItisnothardtondthatTisapseudocontractionifandonlyifTsatisesoneofthefollowingtwoequivalentproperties:(a)Tx–Ty≤x–y+(I–T)x–(I–T)yforallx,y∈C;or(b)I–TismonotoneonC:x–y,(I–T)x–(I–T)y≥forallx,y∈C.
Yaoetal.
JournalofInequalitiesandApplications2014,2014:206Page3of14http://www.
journalofinequalitiesandapplications.
com/content/2014/1/206RecallthatamappingT:C→CisnonexpansiveifTx–Ty≤x–y,x,y∈C.
Itisimmediatelyclearthatnonexpansivemappingsarepseudocontractions.
Recallalsothatthenearestpoint(ormetric)projectionfromHontoCisdenedasfollows:Foreachpointx∈H,PCxistheuniquepointinCwiththepropertyx–PCx≤x–y,y∈C.
NotethatPCischaracterizedbytheinequalityPCx∈C,x–PCx,y–PCx≤,y∈C.
(.
)Consequently,PCisnonexpansive.
Inthesequelweshallusethefollowingnotations:Fix(S)standsforthesetofxedpointsofS;xnxstandsfortheweakconvergenceof(xn)tox;xn→xstandsforthestrongconvergenceof(xn)tox.
Belowistheso-calleddemiclosednessprinciplefornonexpansivemappings.
Lemma.
(cf.
[])LetCbeanonemptyclosedconvexsubsetofarealHilbertspaceH,andletS:C→Cbeanonexpansivemappingwithxedpoints.
If{xn}isasequenceinCsuchthatxnxand(I–S)xn→y,then(I–S)x=y.
Wealsoneedthefollowinglemmawhoseproofcanbefoundinliterature(cf.
[]).
Lemma.
LetCbeanonemptyclosedconvexsubsetofarealHilbertspaceH.
As-sumethatamappingF:C→Hismonotoneandweaklycontinuousalongsegments(i.
e.
,F(x+ty)→F(x)weaklyast→,wheneverx+ty∈Cforx,y∈C).
Thenthevariationalinequalityx∈C,Fx,x–x≥,x∈C(.
)isequivalenttothedualvariationalinequalityx∈C,Fx,x–x≥,x∈C.
(.
)Finally,westatethefollowingelementaryresultonconvergenceofrealsequences.
Lemma.
([])Let{an}beasequenceofnonnegativerealnumberssatisfyingan+≤(–γn)an+γnσn,n≥,where{γn}(,)and{σn}satisfy(i)∞n=γn=∞;(ii)eitherlimsupn→∞σn≤or∞n=|γnσn|isaconstantsuchthatM>(–ρ)supf(xt)–xt:t∈(,).
Inparticular,wegetfrom(.
)xn–u≤–ρf(u)–u,xn–u+tnM,u∈Fix(S).
(.
)Yaoetal.
JournalofInequalitiesandApplications2014,2014:206Page6of14http://www.
journalofinequalitiesandapplications.
com/content/2014/1/206Since{xn}isbounded,withoutlossofgenerality,wemayassumethat{xn}convergesweaklytoapointx∈C.
Noticing(.
)wecanuseLemma.
togetx∈Fix(S).
Thereforewecansubstitutexforuin(.
)togetxn–x≤–ρfx–x,xn–x+tnM.
(.
)However,xnx.
Thistogetherwith(.
)guaranteesthatxn→x.
Thenet{xt}isthere-forerelativelycompact,ast→+,inthenormtopology.
Nowwereturnto(.
)andtakethelimitasn→∞togetx–u≤–ρf(u)–u,x–u,u∈Fix(S).
Inparticular,xsolvesthefollowingvariationalinequality:x∈Fix(S),(I–f)u,u–x≥,u∈Fix(S).
ByLemma.
,weseethatxsolvesthevariationalinequalityx∈Fix(S),(I–f)x,u–x≥,u∈Fix(S).
(.
)Therefore,x=(PFix(S)f)x.
Thatis,xistheuniquexedpointinFix(S)ofthecontractionPFix(S)f.
Clearlythisissucienttoconcludethattheentirenet{xt}convergesinnormtoxast→+.
Finally,ifwetakef=,thenvariationalinequality(.
)isreducedto≤x,u–x,u∈Fix(S).
Equivalently,x≤x,u,u∈Fix(S).
Thisclearlyimpliesthatx≤u,u∈Fix(S).
Therefore,xistheminimum-normxedpointofS.
Thiscompletestheproof.
Wearenowinapositiontoprovethestrongconvergenceofalgorithm(.
).
Theorem.
LetCbeanonemptyclosedconvexsubsetofarealHilbertspaceH,andletT:C→CbeL-LipschitzianandpseudocontractivewithFix(T)=.
Supposethatthefollowingconditionsaresatised:(i)limn→∞αn=and∞n=αn=∞;(ii)limn→∞αnβn=limn→∞βnαn=;(iii)limn→∞αnβn––αn–βnαnβn–=.
Yaoetal.
JournalofInequalitiesandApplications2014,2014:206Page7of14http://www.
journalofinequalitiesandapplications.
com/content/2014/1/206Thenthesequence{xn}generatedbyalgorithm(.
)convergesstronglytotheminimum-normxedpointofT.
ProofFirstweprovethatthesequence{xn}isbounded.
Wewillshowthisfactbyinduc-tion.
Accordingtoconditions(i)and(ii),thereexistsasucientlylargepositiveintegermsuchthat–(L+)(L+)αn+βn+βnαn>,n≥m.
(.
)Fixp∈Fix(T)andtakeaconstantM>suchthatmaxx–p,x–p,.
.
.
,xm–p,p≤M.
(.
)Next,weshowthatxm+–p≤M.
Setym=(–αm–βm)xm+βmTxm;thusxm+=PC[ym].
Then,byusingproperty(.
)ofthemetricprojection,wehavexm+–ym,xm+–p≤.
(.
)BythefactthatI–Tismonotone,wehave(I–T)xm+–(I–T)p,xm+–p≥.
(.
)From(.
),(.
)and(.
),weobtainxm+–p=xm+–p,xm+–p=xm+–ym,xm+–p+ym–p,xm+–p≤ym–p,xm+–p=xm–p,xm+–p–αmxm,xm+–p+βmTxm–xm,xm+–p=xm–p,xm+–p+αmxm+–xm,xm+–p–αmp,xm+–p–αmxm+–p,xm+–p+βmTxm–Txm+,xm+–p+βmxm+–xm,xm+–p–βmxm+–Txm+,xm+–p≤xm–pxm+–p+αmxm+–xmxm+–p+αmpxm+–p–αmxm+–p+βmTxm–Txm++xm+–xmxm+–p≤xm–pxm+–p+αmpxm+–p–αmxm+–p+(L+)(αm+βm)xm+–xmxm+–p.
Yaoetal.
JournalofInequalitiesandApplications2014,2014:206Page8of14http://www.
journalofinequalitiesandapplications.
com/content/2014/1/206Itfollowsthat(+αm)xm+–p≤xm–p+αmp+(L+)(αm+βm)xm+–xm.
(.
)By(.
),wehavexm+–xm=PC(–αm–βm)xm+βmTxm–PC[xm]≤(–αm–βm)xm+βmTxm–xm≤αmp+xm–p+βmTxm–p+xm–p≤αmp+xm–p+(L+)βmxm–p≤(L+)(αm+βm)xm–p+αmp≤(L+)(αm+βm)M.
(.
)Substitute(.
)into(.
)toobtain(+αm)xm+–p≤xm–p+αmp+(L+)(L+)(αm+βm)M≤+αmM+(L+)(L+)(αm+βm)M,thatis,xm+–p≤–(αm/)–(L+)(L+)(αm+βm)+αmM=–(αm/)[–(L+)(L+)(αm+βm+(βm/αm))]+αmM≤M.
Byinduction,wegetxn–p≤M,n≥,(.
)whichimpliesthat{xn}isboundedandsois{Txn}.
NowwetakeaconstantM>suchthatM=supnxn∨Txn–xn.
[Herea∨b=max{a,b}fora,b∈R.
]SetS=(I–T)–(i.
e.
,SisaresolventofthemonotoneoperatorI–T).
WethenhavethatSisanonexpansiveself-mappingofCandFix(S)=Fix(T)(cf.
Theoremof[]).
ByLemma.
,weknowthatwhenever{γn}(,)andγn→+,thesequence{zn}denedbyzn=SPC(–γn)zn(.
)Yaoetal.
JournalofInequalitiesandApplications2014,2014:206Page9of14http://www.
journalofinequalitiesandapplications.
com/content/2014/1/206convergesstronglytotheminimum-normxedpointxofS(andofTasFix(S)=Fix(T)).
Withoutlossofgenerality,wemayassumethatzn≤Mforalln.
Itsucestoprovethatxn+–zn→asn→∞(forsomeγn→+).
Tothisend,werewrite(.
)as(I–T)zn=PC(–γn)zn,n≥.
Byusingthepropertyofmetricprojection(.
),wehave(–γn)zn–(zn–Tzn),xn+–(zn–Tzn)≤–γnzn,xn+–zn–(zn–Tzn)+Tzn–zn,xn+–zn–(zn–Tzn)≤–γnzn+Tzn–zn,xn+–zn+zn–Tzn≤γnzn,Tzn–zn–γnzn+Tzn–zn,xn+–zn≤γnznTzn–zn–zn+Tzn–znγn,xn+–zn≤znTzn–zn.
Notethatzn–Tzn=PC(–γn)zn–zn≤(–γn)zn–zn=γnzn.
Hence,weget–zn+Tzn–znγn,xn+–zn≤γnzn.
(.
)From(.
)wehavezn+–zn=SPC(–γn+)zn+–SPC(–γn)zn≤(–γn+)zn+–(–γn)zn=(–γn+)(zn+–zn)+(γn–γn+)zn≤(–γn+)zn+–zn+|γn+–γn|zn.
Itfollowsthatzn+–zn≤|γn+–γn|γn+zn.
(.
)Setγn:=αnβn.
Yaoetal.
JournalofInequalitiesandApplications2014,2014:206Page10of14http://www.
journalofinequalitiesandapplications.
com/content/2014/1/206Bycondition(ii),γn→+andγn∈(,)fornlargeenough.
Hence,by(.
)and(.
)wehave–zn+βn(Tzn–zn)αn,xn+–zn≤αnβnzn≤αnβnM(.
)andzn–zn–≤αnβn––αn–βnαnβn–M.
(.
)By(.
)wehavexn+–xn=PC(–αn–βn)xn+βnTxn–PCxn≤αnxn+βnTxn–xn≤(αn+βn)M.
(.
)Next,weestimatexn+–zn+.
Sincexn+=PC[yn],xn+–yn,xn+–zn≤.
Using(.
)andbythefactthatTisL-Lipschitzianandpseudocontractive,weinferthatxn+–zn=xn+–zn,xn+–zn=xn+–yn,xn+–zn+yn–zn,xn+–zn≤yn–zn,xn+–zn=(–αn–βn)xn+βnTxn–zn,xn+–zn=(–αn–βn)xn–zn,xn+–zn+βnTxn–Txn+,xn+–zn+βnTxn+–Tzn,xn+–zn+–αnzn+βn(Tzn–zn),xn+–zn,whichleadstoxn+–zn≤(–αn–βn)xn–znxn+–zn+βnLxn–xn+xn+–zn+βnxn+–zn+αn–zn+βnαn(Tzn–zn),xn+–zn≤–αn–βnxn–zn+xn+–zn+βnxn+–zn+Lxn–xn++βnxn+–zn+αnβnzn.
Itfollowsthat,using(.
),(.
)and(.
),wegetxn+–zn≤–αn–βn+αn–βnxn–zn+L+αn–βnxn+–xn+αn(+αn–βn)βnzn+βn+αn–βnxn+–zn≤–αn+αn–βnxn–zn+(αn+βn)+αn–βnLMYaoetal.
JournalofInequalitiesandApplications2014,2014:206Page11of14http://www.
journalofinequalitiesandapplications.
com/content/2014/1/206+αn(+αn–βn)βnM+βn+αn–βnM≤–αn+αn–βnxn–zn–+zn–zn–+(αn+βn)+αn–βn+αn(+αn–βn)βn+βn+αn–βnM≤–αn+αn–βnxn–zn–++αn–βnzn–zn–xn–zn–+zn–zn–+(αn+βn)+αn–βn+αn(+αn–βn)βn+βn+αn–βnM≤–αn+αn–βnxn–zn–++αn–βnαnβn––αn–βnαnβn–M+(αn+βn)+αn–βn+αn(+αn–βn)βn+βn+αn–βnM,(.
)wheretheniteconstantM>isgivenbyM:=maxLM,M,Msupnxn–zn–+zn–zn–.
Letδn=αn+αn–βn≈αn(asn→∞)andnotethatby(.
)itfollowsthat{δn}(,).
Moreover,setθn=αnβn––αn–βnαnβn–+αn+βn+βnαn+αnβn+βnαnM.
Thenrelation(.
)isrewrittenasxn+–zn≤(–δn)xn–zn–+δnθn.
(.
)Byconditions(i),(ii)and(iii),itiseasilyfoundthatlimn→∞δn=,∞n=δn=∞,limn→∞θn=.
WecanthereforeapplyLemma.
to(.
)andconcludethatxn+–zn→asn→∞.
Thiscompletestheproof.
Remark.
Choosethesequences(αn)and(βn)suchthatαn=(n+)aandβn=(n+)b,n≥,Yaoetal.
JournalofInequalitiesandApplications2014,2014:206Page12of14http://www.
journalofinequalitiesandapplications.
com/content/2014/1/206whereItisclearthatconditions(i)and(ii)ofTheorem.
aresatised.
Toverifycondition(iii),wecomputeαnβn––αn–βnαnβn–=αn–αn–βnαnβn–=(n+)a–(n+)a–bna–b=(n+)a+na–b–≈a–bn(n+)a→.
Therefore,{αn}and{βn}satisfyallthreeconditions(i)-(iii)inTheorem.
.
4ApplicationToshowanapplicationofourresults,wedealwiththefollowingproblem.
Problem.
Let(.
)Atwhichvaluedoes{xn}approachasngoestoinnityWeclaimthatlimn→∞xn=anditcanbeeasilyderivedbyapplyingTheorem.
.
ProofInordertoapplyourresult,letH=R,C=[,]anddeneT:C→CbyTx:=x+x.
ObservethatTisLipschitzian,pseudocontractiveandthatFix(T)={}.
Moreover,ifwesetαn=n–/andβn=n–/,then(i)limn→∞αn=and∞n=αn=∞;(ii)limn→∞αnβn=limn→∞βnαn=;(iii)limn→∞αnβn––αn–βnαnβn–=.
ThenTheorem.
ensuresthatlimn→∞xn=.
CompetinginterestsTheauthorsdeclarethattheyhavenocompetinginterests.
Authors'contributionsAllauthorscontributedequallyandsignicantlyinwritingthispaper.
Allauthorsreadandapprovedthenalmanuscript.
Authordetails1DepartmentofMathematics,TianjinPolytechnicUniversity,Tianjin,300387,China.
2DipartimentodiMatematica,UniversitádellaCalabria,ArcavacatadiRende(CS),87036,Italy.
3DepartmentofAppliedMathematics,NationalSunYat-SenUniversity,Kaohsiung,80424,Taiwan.
4DepartmentofInformationManagement,ChengShiuUniversity,Kaohsiung,833,Taiwan.
5CenterforGeneralEducation,KaohsiungMedicalUniversity,Kaohsiung,807,Taiwan.
Yaoetal.
JournalofInequalitiesandApplications2014,2014:206Page13of14http://www.
journalofinequalitiesandapplications.
com/content/2014/1/206AcknowledgementsYonghongYaowassupportedinpartbyNSFC71161001-G0105.
Yeong-ChengLiouwassupportedinpartbyNSC101-2628-E-230-001-MY3andNSC101-2622-E-230-005-CC3.
Received:15February2014Accepted:9May2014Published:23May2014References1.
Mann,WR:Meanvaluemethodsiniteration.
Proc.
Am.
Math.
Soc.
4,506-510(1953)2.
Geobel,K,Kirk,WA:TopicsinMetricFixedPointTheory.
CambridgeStudiesinAdvancedMathematics,vol.
28.
CambridgeUniversityPress,Cambridge(1990)3.
Goebel,K,Reich,S:UniformConvexity,HyperbolicGeometryandNonexpansiveMappings.
Dekker,NewYork(1984)4.
Reich,S:WeakconvergencetheoremsfornonexpansivemappingsinBanachspaces.
J.
Math.
Anal.
Appl.
67,274-276(1979)5.
Reich,S,Zaslavski,AJ:ConvergenceofKrasnoselskii-Manniterationsofnonexpansiveoperators.
Math.
Comput.
Model.
32,1423-1431(2000)6.
Suzuki,T:StrongconvergenceofapproximatedsequencesfornonexpansivemappingsinBanachspaces.
Proc.
Am.
Math.
Soc.
135,99-106(2007)7.
Xu,HK:AvariableKrasnoselskii-Mannalgorithmandthemultiple-setsplitfeasibilityproblem.
InverseProbl.
22,2021-2034(2006)8.
Bauschke,HH,Matousková,E,Reich,S:Projectionandproximalpointmethods:convergenceresultsandcounterexamples.
NonlinearAnal.
,TheoryMethodsAppl.
56,715-738(2004)9.
Browder,FE,Petryshyn,WV:ConstructionofxedpointsofnonlinearmappingsinHilbertspaces.
J.
Math.
Anal.
Appl.
20,197-228(1967)10.
Marino,G,Xu,HK:Weakandstrongconvergencetheoremsforstrictpseudo-contractionsinHilbertspaces.
J.
Math.
Anal.
Appl.
329,336-346(2007)11.
Chidume,CE,Mutangadura,SA:AnexampleontheManniterationmethodforLipschitzpseudo-contractions.
Proc.
Am.
Math.
Soc.
129,2359-2363(2001)12.
Ceng,LC,Petrusel,A,Yao,JC:Strongconvergenceofmodiedimplicititerativealgorithmswithperturbedmappingsforcontinuouspseudocontractivemappings.
Appl.
Math.
Comput.
209,162-176(2009)13.
Chidume,CE,Abbas,M,Ali,B:ConvergenceoftheManniterationalgorithmforaclassofpseudocontractivemappings.
Appl.
Math.
Comput.
194,1-6(2007)14.
Chidume,CE,Zegeye,H:Iterativesolutionofnonlinearequationsofaccretiveandpseudocontractivetypes.
J.
Math.
Anal.
Appl.
282,756-765(2003)15.
Ciric,L,Raq,A,Cakic,N,Ume,JS:ImplicitMannxedpointiterationsforpseudo-contractivemappings.
Appl.
Math.
Lett.
22,581-584(2009)16.
Huang,NJ,Bai,MR:Aperturbediterativeprocedureformultivaluedpseudo-contractivemappingsandmultivaluedaccretivemappingsinBanachspaces.
Comput.
Math.
Appl.
37,7-15(1999)17.
Ishikawa,S:Fixedpointsbyanewiterationmethod.
Proc.
Am.
Math.
Soc.
44,147-150(1974)18.
Lan,KQ,Wu,JH:Convergenceofapproximantsfordemicontinuouspseudo-contractivemapsinHilbertspaces.
NonlinearAnal.
49,737-746(2002)19.
LopezAcedo,G,Xu,HK:Iterativemethodsforstrictpseudo-contractionsinHilbertspaces.
NonlinearAnal.
67,2258-2271(2007)20.
Moore,C,Nnoli,BVC:StrongconvergenceofaveragedapproximantsforLipschitzpseudocontractivemaps.
J.
Math.
Anal.
Appl.
260,269-278(2001)21.
Qin,X,Cho,YJ,Kang,SM,Zhou,H:ConvergencetheoremsofcommonxedpointsforafamilyofLipschitzquasi-pseudocontractions.
NonlinearAnal.
71,685-690(2009)22.
Udomene,A:Pathconvergence,approximationofxedpointsandvariationalsolutionsofLipschitzpseudo-contractionsinBanachspaces.
NonlinearAnal.
67,2403-2414(2007)23.
Yao,Y,Liou,YC,Marino,G:Ahybridalgorithmforpseudo-contractivemappings.
NonlinearAnal.
71,4997-5002(2009)24.
Yao,Y,Liou,YC,Marino,G:StrongconvergenceoftwoiterativealgorithmsfornonexpansivemappingsinHilbertspaces.
FixedPointTheoryAppl.
(2009).
doi:10.
1155/2009/27905825.
Zegeye,H,Shahzad,N,Mekonen,T:ViscosityapproximationmethodsforpseudocontractivemappingsinBanachspaces.
Appl.
Math.
Comput.
185,538-546(2007)26.
Zhang,Q,Cheng,C:StrongconvergencetheoremforafamilyofLipschitzpseudocontractivemappingsinaHilbertspace.
Math.
Comput.
Model.
48,480-485(2008)27.
Cui,YL,Liu,X:NotesonBrowder'sandHalpern'smethodsfornonexpansivemaps.
FixedPointTheory10(1),89-98(2009)28.
Yao,Y,Chen,R,Xu,HK:Schemesforndingminimum-normsolutionsofvariationalinequalities.
NonlinearAnal.
72,3447-3456(2010)29.
Yao,Y,Xu,HK:Iterativemethodsforndingminimum-normxedpointsofnonexpansivemappingswithapplications.
Optimization60,645-658(2011)30.
Xu,HK:Viscosityapproximationmethodsfornonexpansivemappings.
J.
Math.
Anal.
Appl.
298,279-291(2004)31.
Yao,Y,Yao,JC:Onmodiediterativemethodfornonexpansivemappingsandmonotonemappings.
Appl.
Math.
Comput.
186,1551-1558(2007)32.
Browder,FE:SemicontractionandsemiaccretivenonlinearmappingsinBanachspaces.
Bull.
Am.
Math.
Soc.
74,660-665(1968)33.
Lu,X,Xu,HK,Yin,X:Hybridmethodsforaclassofmonotonevariationalinequalities.
NonlinearAnal.
71,1032-1041(2009)34.
Zhou,H:Strongconvergenceofanexplicititerativealgorithmforcontinuouspseudo-contractionsinBanachspaces.
NonlinearAnal.
70,4039-4046(2009)Yaoetal.
JournalofInequalitiesandApplications2014,2014:206Page14of14http://www.
journalofinequalitiesandapplications.
com/content/2014/1/20610.
1186/1029-242X-2014-206Citethisarticleas:Yaoetal.
:Constructionofminimum-normxedpointsofpseudocontractionsinHilbertspaces.
JournalofInequalitiesandApplications2014,2014:206
JournalofInequalitiesandApplications2014,2014:206http://www.
journalofinequalitiesandapplications.
com/content/2014/1/206RESEARCHOpenAccessConstructionofminimum-normxedpointsofpseudocontractionsinHilbertspacesYonghongYao1,GiuseppeMarino2,Hong-KunXu3andYeong-ChengLiou4,5**Correspondence:simplex_liou@hotmail.
com4DepartmentofInformationManagement,ChengShiuUniversity,Kaohsiung,833,Taiwan5CenterforGeneralEducation,KaohsiungMedicalUniversity,Kaohsiung,807,TaiwanFulllistofauthorinformationisavailableattheendofthearticleAbstractAniterativealgorithmisintroducedfortheconstructionoftheminimum-normxedpointofapseudocontractiononaHilbertspace.
Thealgorithmisprovedtobestronglyconvergent.
MSC:47H05;47H10;47H17Keywords:xedpoint;minimum-norm;pseudocontraction;nonexpansivemapping;projection1IntroductionConstructionofxedpointsofnonlinearmappingsisaclassicalandactiveareaofnonlin-earfunctionalanalysisduetothefactthatmanynonlinearproblemscanbereformulatedasxedpointequationsofnonlinearmappings.
TheresearchofthisareadatesbacktoPi-card'sandBanach'stime.
Asamatteroffact,thewell-knownBanachcontractionprinciplestatesthatthePicarditerates{Tnx}convergetotheuniquexedpointofTwheneverTisacontractionofacompletemetricspace.
However,ifTisnotacontraction(nonexpan-sive,say),thenthePicarditerates{Tnx}fail,ingeneral,toconverge;hence,otheriterativemethodsareneeded.
In,Mann[]introducedthenowcalledMann'siterativemethodwhichgeneratesasequence{xn}viatheaveragedalgorithmxn+=(–αn)xn+αnTxn,n≥,(.
)where{αn}isasequenceintheunitinterval[,],Tisaself-mappingofaclosedconvexsubsetCofaHilbertspaceH,andtheinitialguessxisanarbitrary(butxed)pointofC.
Mann'salgorithm(.
)hasextensivelybeenstudied[–],andinparticular,itisknownthatifTisnonexpansive(i.
e.
,Tx–Ty≤x–yforallx,y∈C)andifThasaxedpoint,thenthesequence{xn}generatedbyMann'salgorithm(.
)convergesweaklytoaxedpointofTprovidedthesequence{αn}satisesthecondition∞n=αn(–αn)=∞.
(.
)Thisalgorithm,however,doesnotconvergeinthestrongtopologyingeneral(see[,Corollary.
]).
2014Yaoetal.
;licenseeSpringer.
ThisisanOpenAccessarticledistributedunderthetermsoftheCreativeCommonsAttribu-tionLicense(http://creativecommons.
org/licenses/by/2.
0),whichpermitsunrestricteduse,distribution,andreproductioninanymedium,providedtheoriginalworkisproperlycited.
Yaoetal.
JournalofInequalitiesandApplications2014,2014:206Page2of14http://www.
journalofinequalitiesandapplications.
com/content/2014/1/206BrowderandPetryshyn[]studiedweakconvergenceofMann'salgorithm(.
)fortheclassofstrictpseudocontractions(inthecaseofconstantstepsizesαn=αforalln;see[]forthegeneralcaseofvariablestepsizes).
However,Mann'salgorithmfailstoconvergeforLipschitzianpseudocontractions(seethecounterexampleofChidumeandMutan-gadura[]).
ItisthereforeaninterestingquestionofinventingiterativealgorithmswhichgenerateasequenceconverginginthenormtopologytoaxedpointofaLipschitzianpseudocontraction(ifany).
Theinterestofpseudocontractionsliesintheirconnectionwithmonotoneoperators;namely,TisapseudocontractionifandonlyifthecomplementI–Tisamonotoneoperator.
Wealsonoticethatitisquiteusualtoseekaparticularsolutionofagivennonlinearproblem,inparticular,theminimum-normsolution.
Forinstance,givenaclosedconvexsubsetCofaHilbertspaceHandaboundedlinearoperatorA:H→H,whereHisanotherHilbertspace.
TheC-constrainedpseudoinverseofA,ACisthendenedastheminimum-normsolutionoftheconstrainedminimizationproblemAC(b):=argminx∈CAx–b(.
)whichisequivalenttothexedpointproblemx=PCx–λA(Ax–b),(.
)wherePCisthemetricprojectionfromHontoC,AistheadjointofA,λ>isaconstant,andb∈HissuchthatPA(C)(b)∈A(C).
Itisthereforeaninterestingproblemtoinventiterativealgorithmsthatcangeneratesequenceswhichconvergestronglytotheminimum-normsolutionofagivenxedpointproblem.
Thepurposeofthispaperistosolvesuchaproblemforpseudocontractions.
Moreprecisely,weshallintroduceaniterativealgorithmfortheconstructionofxedpointsofLipschitzianpseudocontractionsandprovethatouralgorithm(see(.
)inSec-tion)convergesinthestrongtopologytotheminimum-normxedpointofthemapping.
Fortheexistingliteratureoniterativemethodsforpseudocontractions,thereadercanconsult[,–];forndingminimum-normsolutionsofnonlinearxedpointandvariationalinequalityproblems,see[–];andforrelatediterativemethodsfornonex-pansivemappings,see[,,,]andthereferencestherein.
2PreliminariesLetHbearealHilbertspacewiththeinnerproduct·,·andthenorm·,respectively.
LetCbeanonemptyclosedconvexsubsetofH.
Theclassofnonlinearmappingswhichwewillstudyistheclassofpseudocontractions.
RecallthatamappingT:C→CisapseudocontractionifitsatisesthepropertyTx–Ty,x–y≤x–y,x,y∈C.
(.
)ItisnothardtondthatTisapseudocontractionifandonlyifTsatisesoneofthefollowingtwoequivalentproperties:(a)Tx–Ty≤x–y+(I–T)x–(I–T)yforallx,y∈C;or(b)I–TismonotoneonC:x–y,(I–T)x–(I–T)y≥forallx,y∈C.
Yaoetal.
JournalofInequalitiesandApplications2014,2014:206Page3of14http://www.
journalofinequalitiesandapplications.
com/content/2014/1/206RecallthatamappingT:C→CisnonexpansiveifTx–Ty≤x–y,x,y∈C.
Itisimmediatelyclearthatnonexpansivemappingsarepseudocontractions.
Recallalsothatthenearestpoint(ormetric)projectionfromHontoCisdenedasfollows:Foreachpointx∈H,PCxistheuniquepointinCwiththepropertyx–PCx≤x–y,y∈C.
NotethatPCischaracterizedbytheinequalityPCx∈C,x–PCx,y–PCx≤,y∈C.
(.
)Consequently,PCisnonexpansive.
Inthesequelweshallusethefollowingnotations:Fix(S)standsforthesetofxedpointsofS;xnxstandsfortheweakconvergenceof(xn)tox;xn→xstandsforthestrongconvergenceof(xn)tox.
Belowistheso-calleddemiclosednessprinciplefornonexpansivemappings.
Lemma.
(cf.
[])LetCbeanonemptyclosedconvexsubsetofarealHilbertspaceH,andletS:C→Cbeanonexpansivemappingwithxedpoints.
If{xn}isasequenceinCsuchthatxnxand(I–S)xn→y,then(I–S)x=y.
Wealsoneedthefollowinglemmawhoseproofcanbefoundinliterature(cf.
[]).
Lemma.
LetCbeanonemptyclosedconvexsubsetofarealHilbertspaceH.
As-sumethatamappingF:C→Hismonotoneandweaklycontinuousalongsegments(i.
e.
,F(x+ty)→F(x)weaklyast→,wheneverx+ty∈Cforx,y∈C).
Thenthevariationalinequalityx∈C,Fx,x–x≥,x∈C(.
)isequivalenttothedualvariationalinequalityx∈C,Fx,x–x≥,x∈C.
(.
)Finally,westatethefollowingelementaryresultonconvergenceofrealsequences.
Lemma.
([])Let{an}beasequenceofnonnegativerealnumberssatisfyingan+≤(–γn)an+γnσn,n≥,where{γn}(,)and{σn}satisfy(i)∞n=γn=∞;(ii)eitherlimsupn→∞σn≤or∞n=|γnσn|isaconstantsuchthatM>(–ρ)supf(xt)–xt:t∈(,).
Inparticular,wegetfrom(.
)xn–u≤–ρf(u)–u,xn–u+tnM,u∈Fix(S).
(.
)Yaoetal.
JournalofInequalitiesandApplications2014,2014:206Page6of14http://www.
journalofinequalitiesandapplications.
com/content/2014/1/206Since{xn}isbounded,withoutlossofgenerality,wemayassumethat{xn}convergesweaklytoapointx∈C.
Noticing(.
)wecanuseLemma.
togetx∈Fix(S).
Thereforewecansubstitutexforuin(.
)togetxn–x≤–ρfx–x,xn–x+tnM.
(.
)However,xnx.
Thistogetherwith(.
)guaranteesthatxn→x.
Thenet{xt}isthere-forerelativelycompact,ast→+,inthenormtopology.
Nowwereturnto(.
)andtakethelimitasn→∞togetx–u≤–ρf(u)–u,x–u,u∈Fix(S).
Inparticular,xsolvesthefollowingvariationalinequality:x∈Fix(S),(I–f)u,u–x≥,u∈Fix(S).
ByLemma.
,weseethatxsolvesthevariationalinequalityx∈Fix(S),(I–f)x,u–x≥,u∈Fix(S).
(.
)Therefore,x=(PFix(S)f)x.
Thatis,xistheuniquexedpointinFix(S)ofthecontractionPFix(S)f.
Clearlythisissucienttoconcludethattheentirenet{xt}convergesinnormtoxast→+.
Finally,ifwetakef=,thenvariationalinequality(.
)isreducedto≤x,u–x,u∈Fix(S).
Equivalently,x≤x,u,u∈Fix(S).
Thisclearlyimpliesthatx≤u,u∈Fix(S).
Therefore,xistheminimum-normxedpointofS.
Thiscompletestheproof.
Wearenowinapositiontoprovethestrongconvergenceofalgorithm(.
).
Theorem.
LetCbeanonemptyclosedconvexsubsetofarealHilbertspaceH,andletT:C→CbeL-LipschitzianandpseudocontractivewithFix(T)=.
Supposethatthefollowingconditionsaresatised:(i)limn→∞αn=and∞n=αn=∞;(ii)limn→∞αnβn=limn→∞βnαn=;(iii)limn→∞αnβn––αn–βnαnβn–=.
Yaoetal.
JournalofInequalitiesandApplications2014,2014:206Page7of14http://www.
journalofinequalitiesandapplications.
com/content/2014/1/206Thenthesequence{xn}generatedbyalgorithm(.
)convergesstronglytotheminimum-normxedpointofT.
ProofFirstweprovethatthesequence{xn}isbounded.
Wewillshowthisfactbyinduc-tion.
Accordingtoconditions(i)and(ii),thereexistsasucientlylargepositiveintegermsuchthat–(L+)(L+)αn+βn+βnαn>,n≥m.
(.
)Fixp∈Fix(T)andtakeaconstantM>suchthatmaxx–p,x–p,.
.
.
,xm–p,p≤M.
(.
)Next,weshowthatxm+–p≤M.
Setym=(–αm–βm)xm+βmTxm;thusxm+=PC[ym].
Then,byusingproperty(.
)ofthemetricprojection,wehavexm+–ym,xm+–p≤.
(.
)BythefactthatI–Tismonotone,wehave(I–T)xm+–(I–T)p,xm+–p≥.
(.
)From(.
),(.
)and(.
),weobtainxm+–p=xm+–p,xm+–p=xm+–ym,xm+–p+ym–p,xm+–p≤ym–p,xm+–p=xm–p,xm+–p–αmxm,xm+–p+βmTxm–xm,xm+–p=xm–p,xm+–p+αmxm+–xm,xm+–p–αmp,xm+–p–αmxm+–p,xm+–p+βmTxm–Txm+,xm+–p+βmxm+–xm,xm+–p–βmxm+–Txm+,xm+–p≤xm–pxm+–p+αmxm+–xmxm+–p+αmpxm+–p–αmxm+–p+βmTxm–Txm++xm+–xmxm+–p≤xm–pxm+–p+αmpxm+–p–αmxm+–p+(L+)(αm+βm)xm+–xmxm+–p.
Yaoetal.
JournalofInequalitiesandApplications2014,2014:206Page8of14http://www.
journalofinequalitiesandapplications.
com/content/2014/1/206Itfollowsthat(+αm)xm+–p≤xm–p+αmp+(L+)(αm+βm)xm+–xm.
(.
)By(.
),wehavexm+–xm=PC(–αm–βm)xm+βmTxm–PC[xm]≤(–αm–βm)xm+βmTxm–xm≤αmp+xm–p+βmTxm–p+xm–p≤αmp+xm–p+(L+)βmxm–p≤(L+)(αm+βm)xm–p+αmp≤(L+)(αm+βm)M.
(.
)Substitute(.
)into(.
)toobtain(+αm)xm+–p≤xm–p+αmp+(L+)(L+)(αm+βm)M≤+αmM+(L+)(L+)(αm+βm)M,thatis,xm+–p≤–(αm/)–(L+)(L+)(αm+βm)+αmM=–(αm/)[–(L+)(L+)(αm+βm+(βm/αm))]+αmM≤M.
Byinduction,wegetxn–p≤M,n≥,(.
)whichimpliesthat{xn}isboundedandsois{Txn}.
NowwetakeaconstantM>suchthatM=supnxn∨Txn–xn.
[Herea∨b=max{a,b}fora,b∈R.
]SetS=(I–T)–(i.
e.
,SisaresolventofthemonotoneoperatorI–T).
WethenhavethatSisanonexpansiveself-mappingofCandFix(S)=Fix(T)(cf.
Theoremof[]).
ByLemma.
,weknowthatwhenever{γn}(,)andγn→+,thesequence{zn}denedbyzn=SPC(–γn)zn(.
)Yaoetal.
JournalofInequalitiesandApplications2014,2014:206Page9of14http://www.
journalofinequalitiesandapplications.
com/content/2014/1/206convergesstronglytotheminimum-normxedpointxofS(andofTasFix(S)=Fix(T)).
Withoutlossofgenerality,wemayassumethatzn≤Mforalln.
Itsucestoprovethatxn+–zn→asn→∞(forsomeγn→+).
Tothisend,werewrite(.
)as(I–T)zn=PC(–γn)zn,n≥.
Byusingthepropertyofmetricprojection(.
),wehave(–γn)zn–(zn–Tzn),xn+–(zn–Tzn)≤–γnzn,xn+–zn–(zn–Tzn)+Tzn–zn,xn+–zn–(zn–Tzn)≤–γnzn+Tzn–zn,xn+–zn+zn–Tzn≤γnzn,Tzn–zn–γnzn+Tzn–zn,xn+–zn≤γnznTzn–zn–zn+Tzn–znγn,xn+–zn≤znTzn–zn.
Notethatzn–Tzn=PC(–γn)zn–zn≤(–γn)zn–zn=γnzn.
Hence,weget–zn+Tzn–znγn,xn+–zn≤γnzn.
(.
)From(.
)wehavezn+–zn=SPC(–γn+)zn+–SPC(–γn)zn≤(–γn+)zn+–(–γn)zn=(–γn+)(zn+–zn)+(γn–γn+)zn≤(–γn+)zn+–zn+|γn+–γn|zn.
Itfollowsthatzn+–zn≤|γn+–γn|γn+zn.
(.
)Setγn:=αnβn.
Yaoetal.
JournalofInequalitiesandApplications2014,2014:206Page10of14http://www.
journalofinequalitiesandapplications.
com/content/2014/1/206Bycondition(ii),γn→+andγn∈(,)fornlargeenough.
Hence,by(.
)and(.
)wehave–zn+βn(Tzn–zn)αn,xn+–zn≤αnβnzn≤αnβnM(.
)andzn–zn–≤αnβn––αn–βnαnβn–M.
(.
)By(.
)wehavexn+–xn=PC(–αn–βn)xn+βnTxn–PCxn≤αnxn+βnTxn–xn≤(αn+βn)M.
(.
)Next,weestimatexn+–zn+.
Sincexn+=PC[yn],xn+–yn,xn+–zn≤.
Using(.
)andbythefactthatTisL-Lipschitzianandpseudocontractive,weinferthatxn+–zn=xn+–zn,xn+–zn=xn+–yn,xn+–zn+yn–zn,xn+–zn≤yn–zn,xn+–zn=(–αn–βn)xn+βnTxn–zn,xn+–zn=(–αn–βn)xn–zn,xn+–zn+βnTxn–Txn+,xn+–zn+βnTxn+–Tzn,xn+–zn+–αnzn+βn(Tzn–zn),xn+–zn,whichleadstoxn+–zn≤(–αn–βn)xn–znxn+–zn+βnLxn–xn+xn+–zn+βnxn+–zn+αn–zn+βnαn(Tzn–zn),xn+–zn≤–αn–βnxn–zn+xn+–zn+βnxn+–zn+Lxn–xn++βnxn+–zn+αnβnzn.
Itfollowsthat,using(.
),(.
)and(.
),wegetxn+–zn≤–αn–βn+αn–βnxn–zn+L+αn–βnxn+–xn+αn(+αn–βn)βnzn+βn+αn–βnxn+–zn≤–αn+αn–βnxn–zn+(αn+βn)+αn–βnLMYaoetal.
JournalofInequalitiesandApplications2014,2014:206Page11of14http://www.
journalofinequalitiesandapplications.
com/content/2014/1/206+αn(+αn–βn)βnM+βn+αn–βnM≤–αn+αn–βnxn–zn–+zn–zn–+(αn+βn)+αn–βn+αn(+αn–βn)βn+βn+αn–βnM≤–αn+αn–βnxn–zn–++αn–βnzn–zn–xn–zn–+zn–zn–+(αn+βn)+αn–βn+αn(+αn–βn)βn+βn+αn–βnM≤–αn+αn–βnxn–zn–++αn–βnαnβn––αn–βnαnβn–M+(αn+βn)+αn–βn+αn(+αn–βn)βn+βn+αn–βnM,(.
)wheretheniteconstantM>isgivenbyM:=maxLM,M,Msupnxn–zn–+zn–zn–.
Letδn=αn+αn–βn≈αn(asn→∞)andnotethatby(.
)itfollowsthat{δn}(,).
Moreover,setθn=αnβn––αn–βnαnβn–+αn+βn+βnαn+αnβn+βnαnM.
Thenrelation(.
)isrewrittenasxn+–zn≤(–δn)xn–zn–+δnθn.
(.
)Byconditions(i),(ii)and(iii),itiseasilyfoundthatlimn→∞δn=,∞n=δn=∞,limn→∞θn=.
WecanthereforeapplyLemma.
to(.
)andconcludethatxn+–zn→asn→∞.
Thiscompletestheproof.
Remark.
Choosethesequences(αn)and(βn)suchthatαn=(n+)aandβn=(n+)b,n≥,Yaoetal.
JournalofInequalitiesandApplications2014,2014:206Page12of14http://www.
journalofinequalitiesandapplications.
com/content/2014/1/206where
aresatised.
Toverifycondition(iii),wecomputeαnβn––αn–βnαnβn–=αn–αn–βnαnβn–=(n+)a–(n+)a–bna–b=(n+)a+na–b–≈a–bn(n+)a→.
Therefore,{αn}and{βn}satisfyallthreeconditions(i)-(iii)inTheorem.
.
4ApplicationToshowanapplicationofourresults,wedealwiththefollowingproblem.
Problem.
Let
)Atwhichvaluedoes{xn}approachasngoestoinnityWeclaimthatlimn→∞xn=anditcanbeeasilyderivedbyapplyingTheorem.
.
ProofInordertoapplyourresult,letH=R,C=[,]anddeneT:C→CbyTx:=x+x.
ObservethatTisLipschitzian,pseudocontractiveandthatFix(T)={}.
Moreover,ifwesetαn=n–/andβn=n–/,then(i)limn→∞αn=and∞n=αn=∞;(ii)limn→∞αnβn=limn→∞βnαn=;(iii)limn→∞αnβn––αn–βnαnβn–=.
ThenTheorem.
ensuresthatlimn→∞xn=.
CompetinginterestsTheauthorsdeclarethattheyhavenocompetinginterests.
Authors'contributionsAllauthorscontributedequallyandsignicantlyinwritingthispaper.
Allauthorsreadandapprovedthenalmanuscript.
Authordetails1DepartmentofMathematics,TianjinPolytechnicUniversity,Tianjin,300387,China.
2DipartimentodiMatematica,UniversitádellaCalabria,ArcavacatadiRende(CS),87036,Italy.
3DepartmentofAppliedMathematics,NationalSunYat-SenUniversity,Kaohsiung,80424,Taiwan.
4DepartmentofInformationManagement,ChengShiuUniversity,Kaohsiung,833,Taiwan.
5CenterforGeneralEducation,KaohsiungMedicalUniversity,Kaohsiung,807,Taiwan.
Yaoetal.
JournalofInequalitiesandApplications2014,2014:206Page13of14http://www.
journalofinequalitiesandapplications.
com/content/2014/1/206AcknowledgementsYonghongYaowassupportedinpartbyNSFC71161001-G0105.
Yeong-ChengLiouwassupportedinpartbyNSC101-2628-E-230-001-MY3andNSC101-2622-E-230-005-CC3.
Received:15February2014Accepted:9May2014Published:23May2014References1.
Mann,WR:Meanvaluemethodsiniteration.
Proc.
Am.
Math.
Soc.
4,506-510(1953)2.
Geobel,K,Kirk,WA:TopicsinMetricFixedPointTheory.
CambridgeStudiesinAdvancedMathematics,vol.
28.
CambridgeUniversityPress,Cambridge(1990)3.
Goebel,K,Reich,S:UniformConvexity,HyperbolicGeometryandNonexpansiveMappings.
Dekker,NewYork(1984)4.
Reich,S:WeakconvergencetheoremsfornonexpansivemappingsinBanachspaces.
J.
Math.
Anal.
Appl.
67,274-276(1979)5.
Reich,S,Zaslavski,AJ:ConvergenceofKrasnoselskii-Manniterationsofnonexpansiveoperators.
Math.
Comput.
Model.
32,1423-1431(2000)6.
Suzuki,T:StrongconvergenceofapproximatedsequencesfornonexpansivemappingsinBanachspaces.
Proc.
Am.
Math.
Soc.
135,99-106(2007)7.
Xu,HK:AvariableKrasnoselskii-Mannalgorithmandthemultiple-setsplitfeasibilityproblem.
InverseProbl.
22,2021-2034(2006)8.
Bauschke,HH,Matousková,E,Reich,S:Projectionandproximalpointmethods:convergenceresultsandcounterexamples.
NonlinearAnal.
,TheoryMethodsAppl.
56,715-738(2004)9.
Browder,FE,Petryshyn,WV:ConstructionofxedpointsofnonlinearmappingsinHilbertspaces.
J.
Math.
Anal.
Appl.
20,197-228(1967)10.
Marino,G,Xu,HK:Weakandstrongconvergencetheoremsforstrictpseudo-contractionsinHilbertspaces.
J.
Math.
Anal.
Appl.
329,336-346(2007)11.
Chidume,CE,Mutangadura,SA:AnexampleontheManniterationmethodforLipschitzpseudo-contractions.
Proc.
Am.
Math.
Soc.
129,2359-2363(2001)12.
Ceng,LC,Petrusel,A,Yao,JC:Strongconvergenceofmodiedimplicititerativealgorithmswithperturbedmappingsforcontinuouspseudocontractivemappings.
Appl.
Math.
Comput.
209,162-176(2009)13.
Chidume,CE,Abbas,M,Ali,B:ConvergenceoftheManniterationalgorithmforaclassofpseudocontractivemappings.
Appl.
Math.
Comput.
194,1-6(2007)14.
Chidume,CE,Zegeye,H:Iterativesolutionofnonlinearequationsofaccretiveandpseudocontractivetypes.
J.
Math.
Anal.
Appl.
282,756-765(2003)15.
Ciric,L,Raq,A,Cakic,N,Ume,JS:ImplicitMannxedpointiterationsforpseudo-contractivemappings.
Appl.
Math.
Lett.
22,581-584(2009)16.
Huang,NJ,Bai,MR:Aperturbediterativeprocedureformultivaluedpseudo-contractivemappingsandmultivaluedaccretivemappingsinBanachspaces.
Comput.
Math.
Appl.
37,7-15(1999)17.
Ishikawa,S:Fixedpointsbyanewiterationmethod.
Proc.
Am.
Math.
Soc.
44,147-150(1974)18.
Lan,KQ,Wu,JH:Convergenceofapproximantsfordemicontinuouspseudo-contractivemapsinHilbertspaces.
NonlinearAnal.
49,737-746(2002)19.
LopezAcedo,G,Xu,HK:Iterativemethodsforstrictpseudo-contractionsinHilbertspaces.
NonlinearAnal.
67,2258-2271(2007)20.
Moore,C,Nnoli,BVC:StrongconvergenceofaveragedapproximantsforLipschitzpseudocontractivemaps.
J.
Math.
Anal.
Appl.
260,269-278(2001)21.
Qin,X,Cho,YJ,Kang,SM,Zhou,H:ConvergencetheoremsofcommonxedpointsforafamilyofLipschitzquasi-pseudocontractions.
NonlinearAnal.
71,685-690(2009)22.
Udomene,A:Pathconvergence,approximationofxedpointsandvariationalsolutionsofLipschitzpseudo-contractionsinBanachspaces.
NonlinearAnal.
67,2403-2414(2007)23.
Yao,Y,Liou,YC,Marino,G:Ahybridalgorithmforpseudo-contractivemappings.
NonlinearAnal.
71,4997-5002(2009)24.
Yao,Y,Liou,YC,Marino,G:StrongconvergenceoftwoiterativealgorithmsfornonexpansivemappingsinHilbertspaces.
FixedPointTheoryAppl.
(2009).
doi:10.
1155/2009/27905825.
Zegeye,H,Shahzad,N,Mekonen,T:ViscosityapproximationmethodsforpseudocontractivemappingsinBanachspaces.
Appl.
Math.
Comput.
185,538-546(2007)26.
Zhang,Q,Cheng,C:StrongconvergencetheoremforafamilyofLipschitzpseudocontractivemappingsinaHilbertspace.
Math.
Comput.
Model.
48,480-485(2008)27.
Cui,YL,Liu,X:NotesonBrowder'sandHalpern'smethodsfornonexpansivemaps.
FixedPointTheory10(1),89-98(2009)28.
Yao,Y,Chen,R,Xu,HK:Schemesforndingminimum-normsolutionsofvariationalinequalities.
NonlinearAnal.
72,3447-3456(2010)29.
Yao,Y,Xu,HK:Iterativemethodsforndingminimum-normxedpointsofnonexpansivemappingswithapplications.
Optimization60,645-658(2011)30.
Xu,HK:Viscosityapproximationmethodsfornonexpansivemappings.
J.
Math.
Anal.
Appl.
298,279-291(2004)31.
Yao,Y,Yao,JC:Onmodiediterativemethodfornonexpansivemappingsandmonotonemappings.
Appl.
Math.
Comput.
186,1551-1558(2007)32.
Browder,FE:SemicontractionandsemiaccretivenonlinearmappingsinBanachspaces.
Bull.
Am.
Math.
Soc.
74,660-665(1968)33.
Lu,X,Xu,HK,Yin,X:Hybridmethodsforaclassofmonotonevariationalinequalities.
NonlinearAnal.
71,1032-1041(2009)34.
Zhou,H:Strongconvergenceofanexplicititerativealgorithmforcontinuouspseudo-contractionsinBanachspaces.
NonlinearAnal.
70,4039-4046(2009)Yaoetal.
JournalofInequalitiesandApplications2014,2014:206Page14of14http://www.
journalofinequalitiesandapplications.
com/content/2014/1/20610.
1186/1029-242X-2014-206Citethisarticleas:Yaoetal.
:Constructionofminimum-normxedpointsofpseudocontractionsinHilbertspaces.
JournalofInequalitiesandApplications2014,2014:206
- linearwww.765.com相关文档
- Sleevewww.765.com
- FREQUENCYwww.765.com
- FEAwww.765.com
- 中国科学引文数据库来源期刊列表(2017—2018
- 8.1www.765.com
- 讯号www.765.com
LOCVPS:美国XEN架构VPS七折,全场八折,日本/新加坡XEN架构月付29.6元起
LOCVPS发来了针对XEN架构VPS的促销方案,其中美国洛杉矶机房7折,其余日本/新加坡/中国香港等机房全部8折,优惠后日本/新加坡机房XEN VPS月付仅29.6元起。这是成立较久的一家国人VPS服务商,目前提供美国洛杉矶(MC/C3)、和中国香港(邦联、沙田电信、大埔)、日本(东京、大阪)、新加坡、德国和荷兰等机房VPS主机,基于XEN或者KVM虚拟架构,均选择国内访问线路不错的机房,适合建...

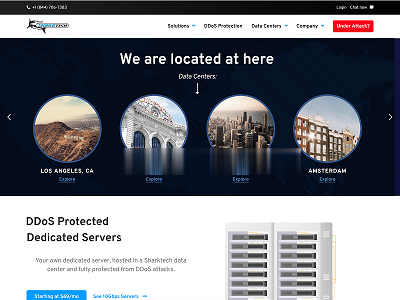
sharktech:老牌高防服务器商,跳楼价,1G独享$70、10G共享$240、10G独享$800
不知道大家是否注意到sharktech的所有服务器的带宽价格全部跳楼跳水,降幅简直不忍直视了,还没有见过这么便宜的独立服务器。根据不同的机房,价格也是不一样的。大带宽、不限流量比较适合建站、数据备份、做下载、做流媒体、做CDN等多种业务。 官方网站:https://www.sharktech.net 付款方式:比特币、信用卡、PayPal、支付宝、西联汇款 以最贵的洛杉矶机器为例,配置表如...
快云科技:夏季大促销,香港VPS7.5折特惠,CN2 GIA线路; 年付仅不到五折巨惠,续费永久同价
快云科技怎么样?快云科技是一家成立于2020年的新起国内主机商,资质齐全 持有IDC ICP ISP等正规商家。我们秉承着服务于客户服务于大众的理念运营,机器线路优价格低。目前已注册用户达到5000+!主营产品有:香港弹性云服务器,美国vps和日本vps,香港物理机,国内高防物理机以及美国日本高防物理机!产品特色:全配置均20M带宽,架构采用KVM虚拟化技术,全盘SSD硬盘,RAID10阵列, 国...

www.765.com为你推荐
-
对对塔今儿老师给推荐了一个叫对对塔的学习网站,看起来挺不错的,有用过的人吗?管不管用?哪些功能比较好啊?怎么查询商标手机上能查询商标吗?怎么查?咏春大师被ko练咏春拳的杨师傅对阵散打冠军,注:是高龄级别被冠军级别打败了,那如果是咏春冠军叶问呢?更别说是李小地图应用哪个手机定位软件最好用?甲骨文不满赔偿公司倒闭员工不满一年怎么赔偿冯媛甑尸城女主角叫什么名字porndao单词prondao的汉语是什么www.44ri.comwww.yydcsjw.comwww.7788dy.com回家的诱惑 哪个网站更新的最快啊m.kan84.net经常使用http://www.feikan.cc看电影的进来帮我下啊