1.5174xx53xx.com
xx53xx.com 时间:2021-04-07 阅读:()
CaspianJournalofAppliedSciencesResearch2(7),pp.
36-43,2013JournalHomepage:www.
cjasr.
comISSN:2251-9114EstimationofParametersforFrechetDistributionBasedonType-IICensoredSamplesKamranAbbas1,2,*,YincaiTang11SchoolofFinanceandStatistics,EastChinaNormalUniversity,Shanghai200241,China2DepartmentofStatistics,UniversityofAzadJammuandKashmir,Muzaffrabad,PakistanInthispaperweconsidermaximumlikelihoodestimatorsandleastsquaresestimatorsoftwo-parameterFrechetdistributionbasedontype-IIcensoredsample.
Themaximumlikelihoodestimatorsandleastsquaresestimatorsaredevelopedforestimatingtheunknownparameters.
TheobservedFisherinformationmatrixandconfidenceintervalsoftheparametersbasedonasymptoticnormalityarealsoderived.
Anextensivesimulationstudyiscarriedouttocomparetheperformancesofdifferentmethods.
2013CaspianJournalofAppliedSciencesResearch.
Allrightsreserved.
Keywords:Maximumlikelihoodestimator;Leastsquaresestimator;Rootmeansquarederror;Frechetdistribution;Type-IIcensoring1.
IntroductionThelengthofthelifetestsofitemscannotbeobservedfailuretimesexactly.
Generallythereareconstraintsonthelengthoflifetestsorotherreliabilitystudies.
Duringtheanalysisofhighlyreliableitems,thetestinghastobestoppedbeforealloftheitemshavefailedasthereislimitedavailabilityoftesttime.
Lifeteststerminatedafteraspecifiednumberoffailuresareknownastype-IIcensoringorfailurecensoring(MeekerandEscober1998).
Intype-IIcensoring,weobserve1,2,.
.
.
,rxxx,whererisspecifiedinadvance.
ThetestendsattimerXX=and()nrunitshavebeensurvived.
Inthisarticleweconsidertype-IIcensoredlifetimedata,whenthelifetimeoftheexperimentalunitfollowsaFrechetdistribution.
FrechetdistributionwasintroducedbyFrenchmathematicianMauriceFrechet(1878-1973)whoidentifiedpossiblelimitdistributionforthelargestorderstatisticduring1927.
TheFrechetdistributionhavebeenusedasanusefulmethodformodelingand*Correspondingaddress:SchoolofFinanceandStatistics,EastChinaNormalUniversity,Shanghai200241,ChinaE-mailaddress:kamiuajk@gmail.
com(KamranAbbas)2013CaspianJournalofAppliedSciencesResearch;www.
cjasr.
com.
Allrightsreserved.
analyzingseveralextremeeventssuchasacceleratedlifetesting,earthquake,flood,rainfall,seacurrentandwindspeed.
ThereforeFrechetdistributioniswellsuitedtocharacterizerandomvariablesoflargefeatures.
Inthispaper,thelifetimesofthetestitemsareassumedtofollowaFrechetdistributionwiththecumulativedistributionfunction(CDF)asfollows:()exp,0,,0Fxxxαβαβ=>>(1)Therefore,probabilitydensityfunction(PDF)oftheFrechetdistributionisgivenby1(,,)exp,0fxxxxααββααββ+=>(2)Wheretheparameterαdeterminestheshapeofthedistributionandβisthescaleparameter.
Thisdistributiondoesnotseemtohavereceivedenoughattention.
ItisworthnotingthatFrechetdistributionisequivalenttotakingthereciprocalofvaluesfromastandardWeibulldistribution.
ApplicationsoftheFrechetdistributioninvariousfieldsaregiveninKamranAbbas;YincaiTang/EstimationofParametersforFrechetDistributionBasedonType-IICensoredSamples2(7),pp.
36-43,201337Harlow(2002)reportedthatitisimportantformodelingthestatisticalbehaviorofmaterialspropertiesforavarietyofengineeringapplications.
NadarajahandKotz(2008)discussedthesociologicalmodelsbasedonFrechetrandomvariables.
Further,applicationsofFrechetdistributionaregiveninZaharimetal.
(2009),andMubarak(2012).
Severalestimationmethodshavebeenproposedtoestimatetheparametersofdistributions.
Amethodofestimationmustbechosenwhichminimizessamplingerrors.
Amethodissuitabletoestimatetheparametersofonedistributionmightnotnecessarilybeasefficientforanotherdistribution.
Moreover,amethodisefficientinestimatingtheparametersmaynotbeefficientinpredictingisgivenbyAl-BaidhaniandSinclair(1987).
Themethodofmaximumlikelihood(ML)isthemostpopularintermsofthetheoriticalprospectiveandtheleastsquares(LS)methodiscomputationallyeasier.
HossainandZimmer(2003)carriedoutastudyonthecomparisonofestimationmethodforcompleteandcensoredsamplebasedonWeibulldistribution.
Similarly,HossainandHowlader(1996)comparedLSEandMLEforcompletesamples.
Moreover,Gumbel(1965)estimatedtheparameterofFrechetdistribution.
Further,AbbasandTang(2012)studieddifferentestimationmethodsforFrechetdistributionwithknownshape.
Moreover,Mann(1984)discussedtheestimationproceduresfortheFrechetandthethree-parameterWeibulldistribution.
TherelationshipsbetweenFrechet,WeibullandtheGumbeldistributionwerealsodiscussed.
Further,themaximum-likelihoodandmomentestimatorsaswellaslinearlybasedestimatorsinvolvingonlyafeworderstatisticsandpropertiesforlargeandsmallsampleswerealsodiscussed.
Inthispaper,comparisonamongtheMLEandLSEaremadeforthecaseofcensoreddataintermsofthebiasandtherootmeansquarederror(RMSE)oftheestimates.
Theplanofthepaperisasfollows.
InSection2,theMLEsandtheobservedFisherinformationmatrixfortheparametersundertype-IIcensoredarederived.
InSection3ofthisarticle,wederivetheLSEs.
InSection4,simulationstudyisdiscussedandfinallyconclusionsaregiveninSection5.
2.
MaximumLikelihoodEstimationLet(),.
.
.
,12XXXXr<=<Thelikelihoodfunctionofrfailuresand()nrcensoredvaluesisgivenby11(,)exp1expnrriLiirXXXαααααβββββ+==∏Then,()11lnlnln(1)ln1explnrriiiLrrnrirXXXααααβαββ==TheMLE'sofαandβsayαandβcanbeobtainedasthesolutionsof()11explnlnlnln,1explnrrriiiinrrrLrrirXXXXXXXααααβββααββββ==(3)()11lnexp.
1expriLrnrirrrXXXXαααααααββββββββ=(4)KamranAbbas;YincaiTang/EstimationofParametersforFrechetDistributionBasedonType-IICensoredSamples2(7),pp.
36-43,201338From(3)and(4),themaximumlikelihoodestimatesare()11,explnlnln1explnrrriiiirnrrrrirXXXXXXXαααααβββββββ===+()11.
1expexprriirXrrXXnrXαααβαβββ==However,itisnoteasytoobtainaclosedformsolutionfortheaboveequations;thereforeweuseLaplaceapproximationtocomputeMLEs.
TheobservedFisherinformationmatrixisobtainedbytakingthesecondandmixedpartialderivativesoflnLlnLwithrespecttoαandβ.
So,theobservedFisherinformationmatrixcanbewrittenas:()222,222lnlnlnlnLLLLIαβααββαβ=Where22221expln11explnrirrLrUiirrXXXXXXαααααααββββββ=12()expln1expUnrrrrrXXXXαααββββ=And()221expln1(1)1exprirLVrWWWirXXXααααααααβββαβββ=KamranAbbas;YincaiTang/EstimationofParametersforFrechetDistributionBasedonType-IICensoredSamples2(7),pp.
36-43,201339()12,exp1expVWnrrrrXXXααααββββ==2111111lnln1expln11lnln1exp11rriiirrrrrLriirrWrrXXXXXXXXXXXXααααααααβααββββαβαββββββββ===+Atwosided()1001%γapproximateconfidencelimitsforαandβbasedontheasymptoticnormalitycanbeconstructedas,,LLzUUzLLzUUzαασααασαββσβββσβ==+==+3.
LeastSquaresEstimationFromtheFrechetCDFin(1),onecaneasilywrite[]lnln()lnlnyFxxαβαWhichislinearmodelinyversuslnxlnxwithaslopeofαandaninterceptoflnαβ.
Let()iXbetheithorderfailureandiYbetheestimateof()()iFX.
Theleastsquareestimatesarethenexpressedas()()()lnln122ln1()lnirYrXYirrXiiXXα===Where()()1lnlnriiXrX==and1riiYrY==,subsequentlyβisexplnYXβα=Inthepresentstudy,HerdJohnsonmethod(Nelson,1982)isusedtoestimatethefailureprobability()()iFXandiYcanbeestimatedas(1),1,2,.
.
.
,1iiiirRRirr==+and01,R=isthereliabilityattime0.
Moreover,iristhereverserankfortheithfailure.
Therefore,()()lnln1iiRY=4.
SimulationStudySimulationstudyisconductedinordertocomparetheperformanceofpresentedMLEsandLSEsusingvarioussamplesize()nandfailureofthefirstindividuals()r.
IncomputingtheestimatessamplesaregeneratedfromtheFrechetdistributionusingthetransformation1(ln)iiXUαβ=,whereiUisuniformlydistributedrandomvariableandwereplicatedtheprocess5000times.
Inthepresentstudysimulationswerecarriedoutfordifferentchoicesofthevaluesofparametersineachcase.
Onlyonevalueofscaleparameter()βneedstobeconsidered,becausechangingthevalueofβisequivalenttomultiplythesamplevaluesbyaconstant.
ComparisonaremadeintermsofmeansandRMSEs(withinparenthesis)andresultsarepresentedinTable1forcomparisonpurpose.
Further,confidenceintervalsofαandβbasedonmaximumlikelihoodestimatorsalongwithcoverageprobabilitiesareconstructedusingtheasymptoticnormality.
TheresultsaresummarizedinTables2-3.
CaspianJournalofAppliedSciencesResearch2(7),pp.
36-43,2013JournalHomepage:www.
cjasr.
comISSN:2251-9114Table1:AverageestimatesandRMSEs(withinparenthesis)ofαandβnrMLLSαβαβ1032.
4335(1.
5174)1.
9210(0.
7077)0.
9315(0.
8191)3.
4047(1.
9930)51.
4585(0.
5528)2.
0995(0.
6088)1.
1547(0.
4771)1.
9585(0.
7555)71.
2779(0.
3758)2.
1587(0.
5794)1.
4251(0.
3191)1.
4938(0.
6800)91.
1980(0.
2948)2.
1957(0.
5538)1.
8071(0.
2944)0.
9344(1.
0826)1532.
5265(1.
5998)1.
7451(0.
6976)0.
7716(0.
3917)4.
2614(2.
6135)51.
4873(0.
5821)1.
9736(0.
5531)0.
9167(0.
3233)2.
5364(0.
8996)71.
2786(0.
3744)2.
0482(0.
4922)1.
0689(0.
2989)2.
0389(0.
4919)91.
1871(0.
2837)2.
0958(0.
4798)1.
2627(0.
2344)1.
4786(0.
6209)2032.
5335(1.
6130)1.
7013(0.
7377)0.
6945(0.
3305)4.
7459(3.
0448)51.
4875(0.
5807)1.
9263(0.
5464)0.
8084(0.
2519)2.
9055(1.
1511)71.
2902(0.
3830)1.
9869(0.
4734)0.
9164(0.
1980)2.
4215(0.
7350)91.
1984(0.
2937)2.
0458(0.
4350)1.
0521(0.
1936)1.
8252(0.
4993)3032.
5633(1.
6423)1.
6239(0.
7817)0.
6029(0.
4039)5.
4807(3.
6743)51.
5095(0.
6029)1.
8462(0.
5608)0.
6851(0.
3219)3.
4519(1.
6455)71.
3019(0.
3955)1.
9403(0.
4756)0.
7670(0.
2561)2.
9275(1.
0522)91.
2164(0.
3074)1.
9640(0.
4067)0.
8503(0.
1966)2.
2920(0.
5826)5032.
8013(1.
6623)1.
5085(0.
8987)0.
5290(0.
4814)6.
5922(4.
3206)51.
5426(0.
6285)1.
7677(0.
6186)0.
5800(0.
4201)4.
1993(2.
2008)71.
3006(0.
3977)1.
8813(0.
5023)0.
6302(0.
3683)3.
5238(1.
5686)91.
2237(0.
3117)1.
9035(0.
4268)0.
6880(0.
3106)2.
8340(0.
9530)8032.
7524(1.
8364)1.
5585(0.
9706)0.
4592(0.
5417)7.
7469(5.
3026)51.
5543(0.
6502)1.
7729(0.
7015)0.
5084(0.
4941)4.
8078(2.
9205)71.
3126(0.
4064)1.
8413(0.
5508)0.
5464(0.
4531)4.
1234(2.
1406)91.
2322(0.
3239)1.
8748(0.
4736)0.
5868(0.
4058)3.
3405(1.
4061)10032.
5542(1.
6326)1.
5316(0.
9829)0.
4362(0.
5602)7.
5373(5.
7916)51.
5647(0.
6553)1.
7322(0.
7157)0.
4797(0.
5241)5.
1514(3.
0976)71.
3210(0.
4170)1.
8294(0.
5723)0.
5140(0.
4849)4.
3783(2.
4050)91.
2251(0.
3208)1.
8793(0.
4891)0.
5494(0.
4475)3.
5626(1.
6036)Table2:Confidenceintervalsandcoverageprobabilities(CP)forαKamranAbbas;YincaiTang/EstimationofParametersforFrechetDistributionBasedonType-IICensoredSamples2(7),pp.
36-43,201341nr90%CICP95%CICP103(0.
4832,4.
0239)0.
7760(0.
5385,4.
3285)0.
90645(0.
6871,2.
2299)0.
8520(0.
5393,2.
3776)0.
93387(0.
6946,1.
8612)0.
8654(0.
5828,1.
9729)0.
93809(0.
7089,1.
6870)0.
8728(0.
6152,1.
7807)0.
9378153(1.
1117,3.
4914)0.
6290(0.
8400,4.
2130)0.
77725(0.
7666,2.
2080)0.
7860(0.
6286,2.
3460)0.
89607(0.
7271,1.
8301)0.
8244(0.
6215,1.
9358)0.
92549(0.
7231,1.
6511)0.
8726(0.
6342,1.
7400)0.
9366203(1.
3038,3.
7632)0.
5144(1.
0682,3.
9987)0.
63505(0.
8367,2.
1382)0.
7240(0.
7120,2.
2629)0.
83537(0.
7710,1.
8095)0.
7992(0.
6715,1.
9090)0.
89369(0.
7526,1.
6441)0.
8382(0.
6672,1.
7295)0.
9128303(1.
5640,3.
5627)0.
3922(1.
7326,3.
7541)0.
48745(0.
9597,2.
0594)0.
5992(0.
8543,2.
1648)0.
71007(0.
8500,1.
7539)0.
7156(0.
7634,1.
8405)0.
81329(0.
8128,1.
6200)0.
7690(0.
7355,1.
6974)0.
8614503(1.
8145,3.
3485)0.
2700(1.
6675,3.
4955)0.
32005(1.
1099,1.
9752)0.
4544(1.
0271,2.
0581)0.
53727(0.
9479,1.
6533)0.
5544(0.
8803,1.
7208)0.
65069(0.
8976,1.
5498)0.
6336(0.
8351,1.
6123)0.
7306803(2.
1014,3.
4035)0.
2198(1.
9767,3.
5282)0.
27065(1.
2118,1.
8968)0.
3368(1.
1462,1.
9624)0.
40627(1.
0326,1.
5926)0.
4404(0.
9790,1.
6463)0.
52049(0.
9729,1.
4915)0.
5060(0.
9232,1.
5412)0.
59281003(2.
0109,3.
0975)0.
2088(1.
9068,3.
2016)0.
25205(1.
2550,1.
8743)0.
3070(1.
1957,1.
9337)0.
36727(1.
0689,1.
5731)0.
3790(1.
0206,1.
6214)0.
45069(0.
9955,1.
4547)0.
4448(0.
9515,1.
4987)0.
5292Table3:Confidenceintervalsandcoverageprobabilities(CP)forβnr90%CICP95%CICP103(1.
3164,2.
5256)0.
5032(1.
2006,2.
6415)0.
60065(1.
7683,2.
4306)0.
6777(1.
7049,2.
4941)0.
77407(1.
4463,2.
8712)0.
6912(1.
3098,3.
0076)0.
78909(1.
5342,2.
8573)0.
7358(1.
4074,2.
9841)0.
8054153(1.
0203,2.
4700)0.
5450(0.
8814,2.
6089)0.
63585(1.
4399,2.
5073)0.
5594(1.
3377,2.
6096)0.
64027(1.
7774,2.
3081)0.
5694(1.
7266,2.
3590)0.
65889(1.
6328,2.
5588)0.
6030(1.
5441,2.
6475)0.
6812203(1.
0225,2.
3801)0.
4996(0.
8925,2.
5106)0.
60865(1.
4220,2.
4306)0.
5372(1.
3254,2.
5272)0.
6324KamranAbbas;YincaiTang/EstimationofParametersforFrechetDistributionBasedonType-IICensoredSamples2(7),pp.
36-43,2013427(1.
3034,2.
6704)0.
6396(1.
1724,2.
8013)0.
72489(1.
5749,2.
5167)0.
7834(1.
4847,2.
6069)0.
8646303(1.
3566,1.
8911)0.
2186(1.
3054,1.
9423)0.
26705(1.
5278,2.
1646)0.
3234(1.
4668,2.
2256)0.
38547(1.
5464,2.
3342)0.
5162(1.
4709,2.
4097)0.
59889(1.
5143,2.
4137)0.
6334(1.
4281,2.
4998)0.
7224503(0.
3216,2.
7500)0.
2680(0.
0939,2.
2822)0.
34205(1.
1636,2.
3717)0.
5346(1.
0479,2.
4874)0.
64007(0.
9596,2.
8031)0.
5415(0.
7830,2.
9796)0.
57009(1.
5547,2.
2524)0.
8946(1.
4879,2.
3192)0.
9452803(0.
6953,2.
4218)0.
4448(0.
5299,2.
5872)0.
58185(1.
4257,2.
1201)0.
2684(1.
3592,2.
1866)0.
31907(1.
6525,2.
0300)0.
3140(1.
6164,2.
0661)0.
36849(1.
3686,2.
3966)0.
6178(1.
2701,2.
4951)0.
72001003(1.
3164,1.
7468)0.
1946(1.
2752,1.
7880)0.
23185(0.
8458,2.
6185)0.
2182(0.
6760,2.
7883)0.
26047(1.
6568,2.
0020)0.
2406(1.
6238,2.
0350)0.
28749(1.
6926,2.
0659)0.
2634(1.
6569,2.
1016)0.
31505.
ConclusionFromtheresultsofthesimulationstudypresentedinTables1-3,weobservethefollowing:1.
ItcanbeseenthatLSmethodtendstounderestimateαandMLmethodtendstooverestimateαforvarioussamplesizesandoveralllevelsofcensoring.
Forfixedleveloftype-IIcensoring,MLEsofαdecreasewithsamplesizesothatthebiastendstobeworseforthelargersamplesizes.
Withinfixedlevelsofcensoring,theRMSEdecreaseswithsamplesizeforallmethodsofestimationdiscussedinthisstudy.
ItisalsoobservedthatCPsareincreasingwithinthefixedlevelofcensoring.
Moreover,thelengthofconfidenceintervalsdecreaseassamplesizeincreasesforfixedlevelofcensoring.
2.
Forestimatingαincaseoftype-IIcensoringitisrecommendedthatoneshouldusetheLS(HerdJohnson)estimationbecauseitprovidesthesmallestRMSEforallsamplesizesandalllevelsoftype-IIcensoring.
3.
Forβ,MLEshavesmallerRMSEthanLSEsforallsamplesizesinfixedleveloftype-IIcensoring.
IncaseofMLEsofβwithinthefixedleveloftype-IIcensoring,assamplesizeincreasesthebiasesandRMSEoftheestimatesdecrease.
Thisindicatedthattheestimatorsareconsistentandapproachestrueparametervalueassamplesizeincreases.
IntermsoftheRMSE,theMLEisslightlybetterforalllevelsoftype-IIcensoringandallsamplesizes.
Finally,inthepresentstudyweconsiderMLandLSestimationofFrechetdistributionbasedontype-IIcensoredsamplesandMLestimatescannotbeobtainedinexplicitform.
ReferencesAbbasK,TangY(2012).
ComparisonofestimationmethodsforFrechetdistributionwithknownshape.
CaspianJournalofAppliedSciencesResearch.
1(10):58-64.
Al-BaidhaniFA,SinclairCD(1987).
ComparisonofmethodsofestimationofparametersoftheWeibulldistribution.
Commun.
Statist.
Simula.
16:373-384.
FrechetM(1927).
Surlaloideprobabilitedelecartmaximum.
Ann.
Soc.
Polon.
Math,6(93).
GumbelEJ(1965).
AquickestimationoftheparametersinFrechet'sdistribution.
ReviewoftheInternationalStatisticalInstitute.
33(3).
HarlowDG(2002).
ApplicationsoftheFrechetdistributionfunction.
InternationalJournalofMaterialandProductTechnology.
5(17):482-495.
KamranAbbas;YincaiTang/EstimationofParametersforFrechetDistributionBasedonType-IICensoredSamples2(7),pp.
36-43,201343HossainA,HowladerHA(1996).
UnweightedleastsquaresestimationofWeibullparameters.
JournalofStatisticalComputationandSimulation.
54:265-271.
HossainAM,ZimmerWJ(2003).
ComparisonofestimationmethodsforWeibullparameters:completeandcensoredsamples.
JournalofStatisticalComputationandSimulation.
73(2):145-153.
MannNR(1984).
StatisticalestimationofparametersoftheWeibullandFrechetdistributions.
StatisticalExtremesandApplication.
NATOASISeries,131,81-89.
MeekerWQ,EscobarLA(1998).
StatisticalMethodsforReliabilityData.
JohnWileyandSons,INC.
MubarakM(2012).
ParameterestimationbasedontheFrechetprogressivetype-IIcensoreddatawithbinomialremovals.
InternationalJournalofQuality,StatisticsandReliability,2012,ArticleID245910.
NadarajahS,KotzS(2008).
SociologicalmodelsbasedonFrechetrandomvariables.
QualityandQuantity.
42:89-95.
NelsonW(1982).
AppliedLifeDataAnalysis.
JohnWileyandSons,NewYork.
ZaharimA,NajidiSK,RazaliAM,SopianK(2009,February,24-26).
AnalyzingMalaysianwindspeeddatausingstatisticaldistribution.
Proceedingsofthe4thIASME/WSEASInternationalConferenceonEnergyandEnvironment.
UniversityofCambridge.
36-43,2013JournalHomepage:www.
cjasr.
comISSN:2251-9114EstimationofParametersforFrechetDistributionBasedonType-IICensoredSamplesKamranAbbas1,2,*,YincaiTang11SchoolofFinanceandStatistics,EastChinaNormalUniversity,Shanghai200241,China2DepartmentofStatistics,UniversityofAzadJammuandKashmir,Muzaffrabad,PakistanInthispaperweconsidermaximumlikelihoodestimatorsandleastsquaresestimatorsoftwo-parameterFrechetdistributionbasedontype-IIcensoredsample.
Themaximumlikelihoodestimatorsandleastsquaresestimatorsaredevelopedforestimatingtheunknownparameters.
TheobservedFisherinformationmatrixandconfidenceintervalsoftheparametersbasedonasymptoticnormalityarealsoderived.
Anextensivesimulationstudyiscarriedouttocomparetheperformancesofdifferentmethods.
2013CaspianJournalofAppliedSciencesResearch.
Allrightsreserved.
Keywords:Maximumlikelihoodestimator;Leastsquaresestimator;Rootmeansquarederror;Frechetdistribution;Type-IIcensoring1.
IntroductionThelengthofthelifetestsofitemscannotbeobservedfailuretimesexactly.
Generallythereareconstraintsonthelengthoflifetestsorotherreliabilitystudies.
Duringtheanalysisofhighlyreliableitems,thetestinghastobestoppedbeforealloftheitemshavefailedasthereislimitedavailabilityoftesttime.
Lifeteststerminatedafteraspecifiednumberoffailuresareknownastype-IIcensoringorfailurecensoring(MeekerandEscober1998).
Intype-IIcensoring,weobserve1,2,.
.
.
,rxxx,whererisspecifiedinadvance.
ThetestendsattimerXX=and()nrunitshavebeensurvived.
Inthisarticleweconsidertype-IIcensoredlifetimedata,whenthelifetimeoftheexperimentalunitfollowsaFrechetdistribution.
FrechetdistributionwasintroducedbyFrenchmathematicianMauriceFrechet(1878-1973)whoidentifiedpossiblelimitdistributionforthelargestorderstatisticduring1927.
TheFrechetdistributionhavebeenusedasanusefulmethodformodelingand*Correspondingaddress:SchoolofFinanceandStatistics,EastChinaNormalUniversity,Shanghai200241,ChinaE-mailaddress:kamiuajk@gmail.
com(KamranAbbas)2013CaspianJournalofAppliedSciencesResearch;www.
cjasr.
com.
Allrightsreserved.
analyzingseveralextremeeventssuchasacceleratedlifetesting,earthquake,flood,rainfall,seacurrentandwindspeed.
ThereforeFrechetdistributioniswellsuitedtocharacterizerandomvariablesoflargefeatures.
Inthispaper,thelifetimesofthetestitemsareassumedtofollowaFrechetdistributionwiththecumulativedistributionfunction(CDF)asfollows:()exp,0,,0Fxxxαβαβ=>>(1)Therefore,probabilitydensityfunction(PDF)oftheFrechetdistributionisgivenby1(,,)exp,0fxxxxααββααββ+=>(2)Wheretheparameterαdeterminestheshapeofthedistributionandβisthescaleparameter.
Thisdistributiondoesnotseemtohavereceivedenoughattention.
ItisworthnotingthatFrechetdistributionisequivalenttotakingthereciprocalofvaluesfromastandardWeibulldistribution.
ApplicationsoftheFrechetdistributioninvariousfieldsaregiveninKamranAbbas;YincaiTang/EstimationofParametersforFrechetDistributionBasedonType-IICensoredSamples2(7),pp.
36-43,201337Harlow(2002)reportedthatitisimportantformodelingthestatisticalbehaviorofmaterialspropertiesforavarietyofengineeringapplications.
NadarajahandKotz(2008)discussedthesociologicalmodelsbasedonFrechetrandomvariables.
Further,applicationsofFrechetdistributionaregiveninZaharimetal.
(2009),andMubarak(2012).
Severalestimationmethodshavebeenproposedtoestimatetheparametersofdistributions.
Amethodofestimationmustbechosenwhichminimizessamplingerrors.
Amethodissuitabletoestimatetheparametersofonedistributionmightnotnecessarilybeasefficientforanotherdistribution.
Moreover,amethodisefficientinestimatingtheparametersmaynotbeefficientinpredictingisgivenbyAl-BaidhaniandSinclair(1987).
Themethodofmaximumlikelihood(ML)isthemostpopularintermsofthetheoriticalprospectiveandtheleastsquares(LS)methodiscomputationallyeasier.
HossainandZimmer(2003)carriedoutastudyonthecomparisonofestimationmethodforcompleteandcensoredsamplebasedonWeibulldistribution.
Similarly,HossainandHowlader(1996)comparedLSEandMLEforcompletesamples.
Moreover,Gumbel(1965)estimatedtheparameterofFrechetdistribution.
Further,AbbasandTang(2012)studieddifferentestimationmethodsforFrechetdistributionwithknownshape.
Moreover,Mann(1984)discussedtheestimationproceduresfortheFrechetandthethree-parameterWeibulldistribution.
TherelationshipsbetweenFrechet,WeibullandtheGumbeldistributionwerealsodiscussed.
Further,themaximum-likelihoodandmomentestimatorsaswellaslinearlybasedestimatorsinvolvingonlyafeworderstatisticsandpropertiesforlargeandsmallsampleswerealsodiscussed.
Inthispaper,comparisonamongtheMLEandLSEaremadeforthecaseofcensoreddataintermsofthebiasandtherootmeansquarederror(RMSE)oftheestimates.
Theplanofthepaperisasfollows.
InSection2,theMLEsandtheobservedFisherinformationmatrixfortheparametersundertype-IIcensoredarederived.
InSection3ofthisarticle,wederivetheLSEs.
InSection4,simulationstudyisdiscussedandfinallyconclusionsaregiveninSection5.
2.
MaximumLikelihoodEstimationLet(),.
.
.
,12XXXXr<=<
1expriLrnrirrrXXXXαααααααββββββββ=(4)KamranAbbas;YincaiTang/EstimationofParametersforFrechetDistributionBasedonType-IICensoredSamples2(7),pp.
36-43,201338From(3)and(4),themaximumlikelihoodestimatesare()11,explnlnln1explnrrriiiirnrrrrirXXXXXXXαααααβββββββ===+()11.
1expexprriirXrrXXnrXαααβαβββ==However,itisnoteasytoobtainaclosedformsolutionfortheaboveequations;thereforeweuseLaplaceapproximationtocomputeMLEs.
TheobservedFisherinformationmatrixisobtainedbytakingthesecondandmixedpartialderivativesoflnLlnLwithrespecttoαandβ.
So,theobservedFisherinformationmatrixcanbewrittenas:()222,222lnlnlnlnLLLLIαβααββαβ=Where22221expln11explnrirrLrUiirrXXXXXXαααααααββββββ=12()expln1expUnrrrrrXXXXαααββββ=And()221expln1(1)1exprirLVrWWWirXXXααααααααβββαβββ=KamranAbbas;YincaiTang/EstimationofParametersforFrechetDistributionBasedonType-IICensoredSamples2(7),pp.
36-43,201339()12,exp1expVWnrrrrXXXααααββββ==2111111lnln1expln11lnln1exp11rriiirrrrrLriirrWrrXXXXXXXXXXXXααααααααβααββββαβαββββββββ===+Atwosided()1001%γapproximateconfidencelimitsforαandβbasedontheasymptoticnormalitycanbeconstructedas,,LLzUUzLLzUUzαασααασαββσβββσβ==+==+3.
LeastSquaresEstimationFromtheFrechetCDFin(1),onecaneasilywrite[]lnln()lnlnyFxxαβαWhichislinearmodelinyversuslnxlnxwithaslopeofαandaninterceptoflnαβ.
Let()iXbetheithorderfailureandiYbetheestimateof()()iFX.
Theleastsquareestimatesarethenexpressedas()()()lnln122ln1()lnirYrXYirrXiiXXα===Where()()1lnlnriiXrX==and1riiYrY==,subsequentlyβisexplnYXβα=Inthepresentstudy,HerdJohnsonmethod(Nelson,1982)isusedtoestimatethefailureprobability()()iFXandiYcanbeestimatedas(1),1,2,.
.
.
,1iiiirRRirr==+and01,R=isthereliabilityattime0.
Moreover,iristhereverserankfortheithfailure.
Therefore,()()lnln1iiRY=4.
SimulationStudySimulationstudyisconductedinordertocomparetheperformanceofpresentedMLEsandLSEsusingvarioussamplesize()nandfailureofthefirstindividuals()r.
IncomputingtheestimatessamplesaregeneratedfromtheFrechetdistributionusingthetransformation1(ln)iiXUαβ=,whereiUisuniformlydistributedrandomvariableandwereplicatedtheprocess5000times.
Inthepresentstudysimulationswerecarriedoutfordifferentchoicesofthevaluesofparametersineachcase.
Onlyonevalueofscaleparameter()βneedstobeconsidered,becausechangingthevalueofβisequivalenttomultiplythesamplevaluesbyaconstant.
ComparisonaremadeintermsofmeansandRMSEs(withinparenthesis)andresultsarepresentedinTable1forcomparisonpurpose.
Further,confidenceintervalsofαandβbasedonmaximumlikelihoodestimatorsalongwithcoverageprobabilitiesareconstructedusingtheasymptoticnormality.
TheresultsaresummarizedinTables2-3.
CaspianJournalofAppliedSciencesResearch2(7),pp.
36-43,2013JournalHomepage:www.
cjasr.
comISSN:2251-9114Table1:AverageestimatesandRMSEs(withinparenthesis)ofαandβnrMLLSαβαβ1032.
4335(1.
5174)1.
9210(0.
7077)0.
9315(0.
8191)3.
4047(1.
9930)51.
4585(0.
5528)2.
0995(0.
6088)1.
1547(0.
4771)1.
9585(0.
7555)71.
2779(0.
3758)2.
1587(0.
5794)1.
4251(0.
3191)1.
4938(0.
6800)91.
1980(0.
2948)2.
1957(0.
5538)1.
8071(0.
2944)0.
9344(1.
0826)1532.
5265(1.
5998)1.
7451(0.
6976)0.
7716(0.
3917)4.
2614(2.
6135)51.
4873(0.
5821)1.
9736(0.
5531)0.
9167(0.
3233)2.
5364(0.
8996)71.
2786(0.
3744)2.
0482(0.
4922)1.
0689(0.
2989)2.
0389(0.
4919)91.
1871(0.
2837)2.
0958(0.
4798)1.
2627(0.
2344)1.
4786(0.
6209)2032.
5335(1.
6130)1.
7013(0.
7377)0.
6945(0.
3305)4.
7459(3.
0448)51.
4875(0.
5807)1.
9263(0.
5464)0.
8084(0.
2519)2.
9055(1.
1511)71.
2902(0.
3830)1.
9869(0.
4734)0.
9164(0.
1980)2.
4215(0.
7350)91.
1984(0.
2937)2.
0458(0.
4350)1.
0521(0.
1936)1.
8252(0.
4993)3032.
5633(1.
6423)1.
6239(0.
7817)0.
6029(0.
4039)5.
4807(3.
6743)51.
5095(0.
6029)1.
8462(0.
5608)0.
6851(0.
3219)3.
4519(1.
6455)71.
3019(0.
3955)1.
9403(0.
4756)0.
7670(0.
2561)2.
9275(1.
0522)91.
2164(0.
3074)1.
9640(0.
4067)0.
8503(0.
1966)2.
2920(0.
5826)5032.
8013(1.
6623)1.
5085(0.
8987)0.
5290(0.
4814)6.
5922(4.
3206)51.
5426(0.
6285)1.
7677(0.
6186)0.
5800(0.
4201)4.
1993(2.
2008)71.
3006(0.
3977)1.
8813(0.
5023)0.
6302(0.
3683)3.
5238(1.
5686)91.
2237(0.
3117)1.
9035(0.
4268)0.
6880(0.
3106)2.
8340(0.
9530)8032.
7524(1.
8364)1.
5585(0.
9706)0.
4592(0.
5417)7.
7469(5.
3026)51.
5543(0.
6502)1.
7729(0.
7015)0.
5084(0.
4941)4.
8078(2.
9205)71.
3126(0.
4064)1.
8413(0.
5508)0.
5464(0.
4531)4.
1234(2.
1406)91.
2322(0.
3239)1.
8748(0.
4736)0.
5868(0.
4058)3.
3405(1.
4061)10032.
5542(1.
6326)1.
5316(0.
9829)0.
4362(0.
5602)7.
5373(5.
7916)51.
5647(0.
6553)1.
7322(0.
7157)0.
4797(0.
5241)5.
1514(3.
0976)71.
3210(0.
4170)1.
8294(0.
5723)0.
5140(0.
4849)4.
3783(2.
4050)91.
2251(0.
3208)1.
8793(0.
4891)0.
5494(0.
4475)3.
5626(1.
6036)Table2:Confidenceintervalsandcoverageprobabilities(CP)forαKamranAbbas;YincaiTang/EstimationofParametersforFrechetDistributionBasedonType-IICensoredSamples2(7),pp.
36-43,201341nr90%CICP95%CICP103(0.
4832,4.
0239)0.
7760(0.
5385,4.
3285)0.
90645(0.
6871,2.
2299)0.
8520(0.
5393,2.
3776)0.
93387(0.
6946,1.
8612)0.
8654(0.
5828,1.
9729)0.
93809(0.
7089,1.
6870)0.
8728(0.
6152,1.
7807)0.
9378153(1.
1117,3.
4914)0.
6290(0.
8400,4.
2130)0.
77725(0.
7666,2.
2080)0.
7860(0.
6286,2.
3460)0.
89607(0.
7271,1.
8301)0.
8244(0.
6215,1.
9358)0.
92549(0.
7231,1.
6511)0.
8726(0.
6342,1.
7400)0.
9366203(1.
3038,3.
7632)0.
5144(1.
0682,3.
9987)0.
63505(0.
8367,2.
1382)0.
7240(0.
7120,2.
2629)0.
83537(0.
7710,1.
8095)0.
7992(0.
6715,1.
9090)0.
89369(0.
7526,1.
6441)0.
8382(0.
6672,1.
7295)0.
9128303(1.
5640,3.
5627)0.
3922(1.
7326,3.
7541)0.
48745(0.
9597,2.
0594)0.
5992(0.
8543,2.
1648)0.
71007(0.
8500,1.
7539)0.
7156(0.
7634,1.
8405)0.
81329(0.
8128,1.
6200)0.
7690(0.
7355,1.
6974)0.
8614503(1.
8145,3.
3485)0.
2700(1.
6675,3.
4955)0.
32005(1.
1099,1.
9752)0.
4544(1.
0271,2.
0581)0.
53727(0.
9479,1.
6533)0.
5544(0.
8803,1.
7208)0.
65069(0.
8976,1.
5498)0.
6336(0.
8351,1.
6123)0.
7306803(2.
1014,3.
4035)0.
2198(1.
9767,3.
5282)0.
27065(1.
2118,1.
8968)0.
3368(1.
1462,1.
9624)0.
40627(1.
0326,1.
5926)0.
4404(0.
9790,1.
6463)0.
52049(0.
9729,1.
4915)0.
5060(0.
9232,1.
5412)0.
59281003(2.
0109,3.
0975)0.
2088(1.
9068,3.
2016)0.
25205(1.
2550,1.
8743)0.
3070(1.
1957,1.
9337)0.
36727(1.
0689,1.
5731)0.
3790(1.
0206,1.
6214)0.
45069(0.
9955,1.
4547)0.
4448(0.
9515,1.
4987)0.
5292Table3:Confidenceintervalsandcoverageprobabilities(CP)forβnr90%CICP95%CICP103(1.
3164,2.
5256)0.
5032(1.
2006,2.
6415)0.
60065(1.
7683,2.
4306)0.
6777(1.
7049,2.
4941)0.
77407(1.
4463,2.
8712)0.
6912(1.
3098,3.
0076)0.
78909(1.
5342,2.
8573)0.
7358(1.
4074,2.
9841)0.
8054153(1.
0203,2.
4700)0.
5450(0.
8814,2.
6089)0.
63585(1.
4399,2.
5073)0.
5594(1.
3377,2.
6096)0.
64027(1.
7774,2.
3081)0.
5694(1.
7266,2.
3590)0.
65889(1.
6328,2.
5588)0.
6030(1.
5441,2.
6475)0.
6812203(1.
0225,2.
3801)0.
4996(0.
8925,2.
5106)0.
60865(1.
4220,2.
4306)0.
5372(1.
3254,2.
5272)0.
6324KamranAbbas;YincaiTang/EstimationofParametersforFrechetDistributionBasedonType-IICensoredSamples2(7),pp.
36-43,2013427(1.
3034,2.
6704)0.
6396(1.
1724,2.
8013)0.
72489(1.
5749,2.
5167)0.
7834(1.
4847,2.
6069)0.
8646303(1.
3566,1.
8911)0.
2186(1.
3054,1.
9423)0.
26705(1.
5278,2.
1646)0.
3234(1.
4668,2.
2256)0.
38547(1.
5464,2.
3342)0.
5162(1.
4709,2.
4097)0.
59889(1.
5143,2.
4137)0.
6334(1.
4281,2.
4998)0.
7224503(0.
3216,2.
7500)0.
2680(0.
0939,2.
2822)0.
34205(1.
1636,2.
3717)0.
5346(1.
0479,2.
4874)0.
64007(0.
9596,2.
8031)0.
5415(0.
7830,2.
9796)0.
57009(1.
5547,2.
2524)0.
8946(1.
4879,2.
3192)0.
9452803(0.
6953,2.
4218)0.
4448(0.
5299,2.
5872)0.
58185(1.
4257,2.
1201)0.
2684(1.
3592,2.
1866)0.
31907(1.
6525,2.
0300)0.
3140(1.
6164,2.
0661)0.
36849(1.
3686,2.
3966)0.
6178(1.
2701,2.
4951)0.
72001003(1.
3164,1.
7468)0.
1946(1.
2752,1.
7880)0.
23185(0.
8458,2.
6185)0.
2182(0.
6760,2.
7883)0.
26047(1.
6568,2.
0020)0.
2406(1.
6238,2.
0350)0.
28749(1.
6926,2.
0659)0.
2634(1.
6569,2.
1016)0.
31505.
ConclusionFromtheresultsofthesimulationstudypresentedinTables1-3,weobservethefollowing:1.
ItcanbeseenthatLSmethodtendstounderestimateαandMLmethodtendstooverestimateαforvarioussamplesizesandoveralllevelsofcensoring.
Forfixedleveloftype-IIcensoring,MLEsofαdecreasewithsamplesizesothatthebiastendstobeworseforthelargersamplesizes.
Withinfixedlevelsofcensoring,theRMSEdecreaseswithsamplesizeforallmethodsofestimationdiscussedinthisstudy.
ItisalsoobservedthatCPsareincreasingwithinthefixedlevelofcensoring.
Moreover,thelengthofconfidenceintervalsdecreaseassamplesizeincreasesforfixedlevelofcensoring.
2.
Forestimatingαincaseoftype-IIcensoringitisrecommendedthatoneshouldusetheLS(HerdJohnson)estimationbecauseitprovidesthesmallestRMSEforallsamplesizesandalllevelsoftype-IIcensoring.
3.
Forβ,MLEshavesmallerRMSEthanLSEsforallsamplesizesinfixedleveloftype-IIcensoring.
IncaseofMLEsofβwithinthefixedleveloftype-IIcensoring,assamplesizeincreasesthebiasesandRMSEoftheestimatesdecrease.
Thisindicatedthattheestimatorsareconsistentandapproachestrueparametervalueassamplesizeincreases.
IntermsoftheRMSE,theMLEisslightlybetterforalllevelsoftype-IIcensoringandallsamplesizes.
Finally,inthepresentstudyweconsiderMLandLSestimationofFrechetdistributionbasedontype-IIcensoredsamplesandMLestimatescannotbeobtainedinexplicitform.
ReferencesAbbasK,TangY(2012).
ComparisonofestimationmethodsforFrechetdistributionwithknownshape.
CaspianJournalofAppliedSciencesResearch.
1(10):58-64.
Al-BaidhaniFA,SinclairCD(1987).
ComparisonofmethodsofestimationofparametersoftheWeibulldistribution.
Commun.
Statist.
Simula.
16:373-384.
FrechetM(1927).
Surlaloideprobabilitedelecartmaximum.
Ann.
Soc.
Polon.
Math,6(93).
GumbelEJ(1965).
AquickestimationoftheparametersinFrechet'sdistribution.
ReviewoftheInternationalStatisticalInstitute.
33(3).
HarlowDG(2002).
ApplicationsoftheFrechetdistributionfunction.
InternationalJournalofMaterialandProductTechnology.
5(17):482-495.
KamranAbbas;YincaiTang/EstimationofParametersforFrechetDistributionBasedonType-IICensoredSamples2(7),pp.
36-43,201343HossainA,HowladerHA(1996).
UnweightedleastsquaresestimationofWeibullparameters.
JournalofStatisticalComputationandSimulation.
54:265-271.
HossainAM,ZimmerWJ(2003).
ComparisonofestimationmethodsforWeibullparameters:completeandcensoredsamples.
JournalofStatisticalComputationandSimulation.
73(2):145-153.
MannNR(1984).
StatisticalestimationofparametersoftheWeibullandFrechetdistributions.
StatisticalExtremesandApplication.
NATOASISeries,131,81-89.
MeekerWQ,EscobarLA(1998).
StatisticalMethodsforReliabilityData.
JohnWileyandSons,INC.
MubarakM(2012).
ParameterestimationbasedontheFrechetprogressivetype-IIcensoreddatawithbinomialremovals.
InternationalJournalofQuality,StatisticsandReliability,2012,ArticleID245910.
NadarajahS,KotzS(2008).
SociologicalmodelsbasedonFrechetrandomvariables.
QualityandQuantity.
42:89-95.
NelsonW(1982).
AppliedLifeDataAnalysis.
JohnWileyandSons,NewYork.
ZaharimA,NajidiSK,RazaliAM,SopianK(2009,February,24-26).
AnalyzingMalaysianwindspeeddatausingstatisticaldistribution.
Proceedingsofthe4thIASME/WSEASInternationalConferenceonEnergyandEnvironment.
UniversityofCambridge.
- 1.5174xx53xx.com相关文档
- HOLExx53xx.com
- 3.326xx53xx.com
- dataxx53xx.com
- obtainxx53xx.com
- Autoxx53xx.com
- 电话xx53xx.com
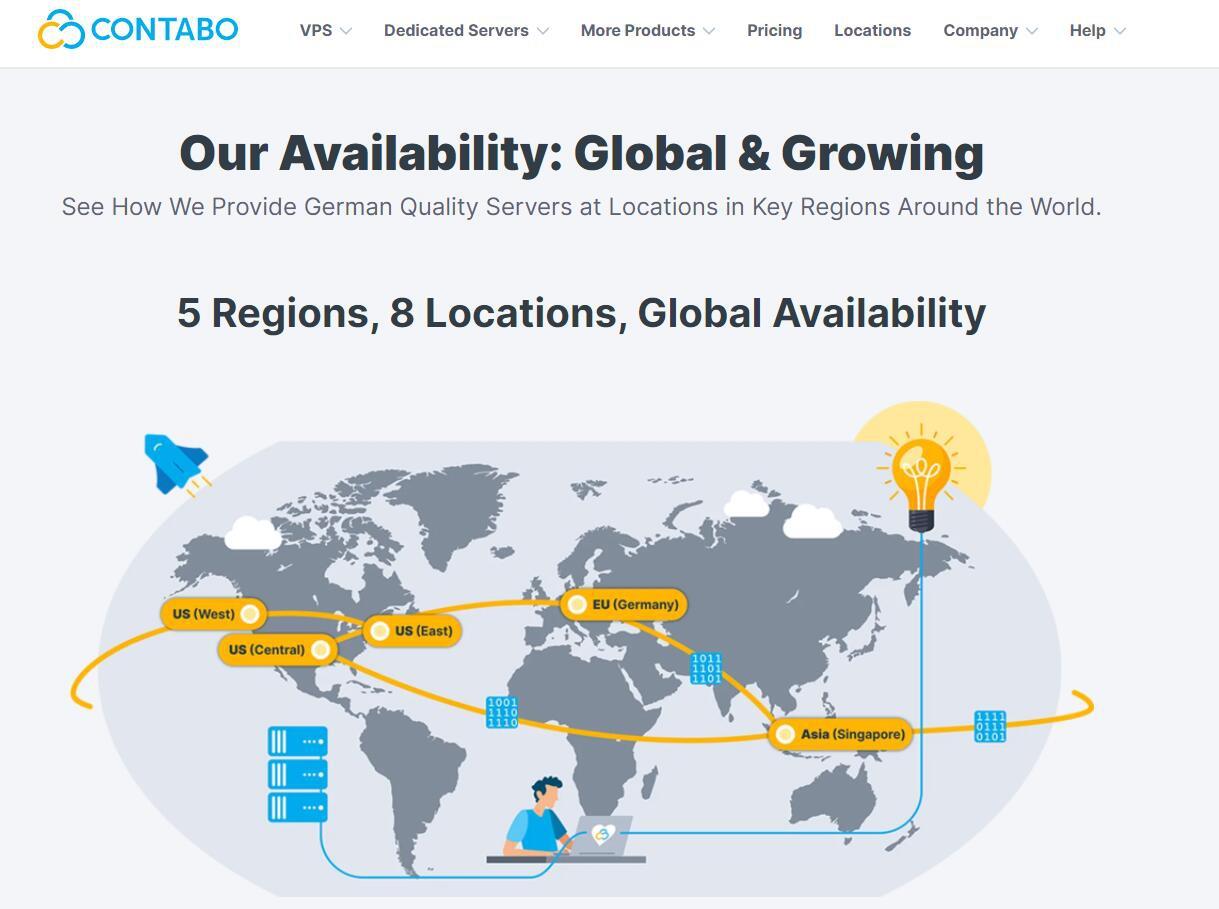
€4.99/月Contabo云服务器,美国高性价比VPS/4核8G内存200G SSD存储
Contabo是一家运营了20多年的欧洲老牌主机商,之前主要是运营德国数据中心,Contabo在今年4月份增设新加坡数据中心,近期同时新增了美国纽约和西雅图数据中心。全球布局基本完成,目前可选的数据中心包括:德国本土、美国东部(纽约)、美国西部(西雅图)、美国中部(圣路易斯)和亚洲的新加坡数据中心。Contabo的之前国外主机测评网站有多次介绍,他们家的特点就是性价比高,而且这个高不是一般的高,是...
EtherNetservers年付仅10美元,美国洛杉矶VPS/1核512M内存10GB硬盘1Gpbs端口月流量500GB/2个IP
EtherNetservers是一家成立于2013年的英国主机商,提供基于OpenVZ和KVM架构的VPS,数据中心包括美国洛杉矶、新泽西和杰克逊维尔,商家支持使用PayPal、支付宝等付款方式,提供 60 天退款保证,这在IDC行业来说很少见,也可见商家对自家产品很有信心。有需要便宜VPS、多IP VPS的朋友可以关注一下。优惠码SUMMER-VPS-15 (终身 15% 的折扣)SUMMER-...
CloudCone闪购优惠洛杉矶MC机房VPS月$1.99 便宜可随意删除重开
CloudCone商家我们很多喜欢低价便宜VPS主机的肯定是熟悉的,个人不是特别喜欢他。因为我之前测试过几次,开通的机器IP都是不通的,需要删除且开通好几次才能得到一个可用的IP地址。当然他们家的优势也是有的,就是价格确实便宜,而且还支持删除重新开通,而且机房只有一个洛杉矶MC。实话,如果他们家能多几个机房,保持现在的特点,还是有很多市场的。CloudCone是来自美国的主机销售商,成立于2017...

xx53xx.com为你推荐
-
易烊千玺弟弟创魔方世界纪录易烊千玺带弟弟参加的那个节目是什么哈利波特罗恩升级当爸电影哈利波特中罗恩一家的红头发为什么后来变成金色的了johncusack约翰·库萨克好看的的恐怖片全集嘀动网动网和爱动网各自的优势是什么?psbc.comwww.psbc.com怎样注册同ip网站12306怎么那么多同IP网站啊?这么重要的一个网站我感觉应该是超强配置的独立服务器才对啊,求高人指点8090lu.com8090看看电影网怎么打不开了mole.61.com摩尔庄园的米米号和密码我都忘了 只记得注册的邮箱 怎么办-_-www.zjs.com.cn中通快递投诉网站网址是什么?103838.com39052.com这电影网支持网页观看吗?