mediumwww.236jj.com
www.236jj.com 时间:2021-04-06 阅读:()
Wangetal.
AdvancesinDierenceEquations2013,2013:280http://www.
advancesindifferenceequations.
com/content/2013/1/280RESEARCHOpenAccessExistenceresultsfornonlinearfractionaldifferentialequationsinvolvingdifferentRiemann-LiouvillefractionalderivativesGuotaoWang1,SanyangLiu1*,DumitruBaleanu2,3,4andLihongZhang5*Correspondence:liusanyang@126.
com1DepartmentofAppliedMathematics,XidianUniversity,Xi'an,Shaanxi710071,People'sRepublicofChinaFulllistofauthorinformationisavailableattheendofthearticleAbstractByapplyinganiterativetechnique,anecessaryandsucientconditionisobtainedfortheexistenceoftheuniquesolutionofnonlinearfractionaldierentialequationsinvolvingtwoRiemann-Liouvillederivativesofdierentfractionalorders.
Finally,anexampleisalsogiventoillustratetheavailabilityofourmainresults.
Keywords:dierentfractional-order;nonlinearfractionaldierentialequations;Riemann-Liouvillederivative;monotoneiterativetechnique1IntroductionRecently,thestudyoffractionaldierentialequationshasacquiredpopularity,seebooks[–]formoreinformation.
Inthispaper,weconsiderthefollowingnonlinearfractionaldierentialequations:Dαu(t)=f(t,Dαu(t),Dβu(t),u(t)),Dβu()=,u()=,(.
)wheret∈J=[,T](Itisworthwhiletoindicatethatthenonlineartermfinvolvestheunknownfunction'sRiemann-Liouvillefractionalderivativeswithdierentorders.
Themethodofupperandlowersolutionscoupledwiththemonotoneiterativetech-niqueisaninterestingandpowerfulmechanism.
Theimportanceandadvantageofthemethodneedsnospecialemphasis[,].
TherehaveappearedsomepapersdealingwiththeexistenceofthesolutionofnonlinearRiemann-Liouville-typefractionaldier-entialequations[–]ornonlinearCaputo-typefractionaldierentialequations[–]byusingthemethod.
Forexample,byemployingthemethodoflowerandupperso-lutionscombinedwiththemonotoneiterativetechnique,LakshmikanthanandVatsala[],McRae[]andZhang[]successfullyinvestigatedtheinitialvalueproblemsofRiemann-LiouvillefractionaldierentialequationDαu(t)=f(t,u(t)),where<α≤.
However,intheexistingliterature[–],onlyonecasewhenα∈(,]isconsidered.
Theresearch,involvingRiemann-Liouvillefractionalderivativeoforder<α≤,pro-ceedsslowlyandthereappearsomenewdicultiesinemployingthemonotoneiterativemethod.
Toovercomethesediculties,weapplyasubstitutionDαu(t)=y(t).
Notethat2013Wangetal.
;licenseeSpringer.
ThisisanOpenAccessarticledistributedunderthetermsoftheCreativeCommonsAttribu-tionLicense(http://creativecommons.
org/licenses/by/2.
0),whichpermitsunrestricteduse,distribution,andreproductioninanymedium,providedtheoriginalworkisproperlycited.
Wangetal.
AdvancesinDierenceEquations2013,2013:280Page2of7http://www.
advancesindifferenceequations.
com/content/2013/1/280thetechniquehasbeendiscussedforfractionalproblemsinpapers[,].
Tothebestofourknowledge,itistherstpaper,inwhichthemonotoneiterativemethodisappliedtononlinearRiemann-Liouville-typefractionaldierentialequations,involvingtwodier-entfractionalderivativesDαandDβ.
Weorganizetherestofthispaperasfollows.
InSection,byusingthemonotoneiter-ativetechniqueandthemethodofupperandlowersolutions,theminimalandmaximalsolutionsofanequivalentproblemof(.
)areinvestigatedandtwoexplicitmonotoneit-erativesequences,convergingtothecorrespondingminimalandmaximalsolution,aregiven.
Inaddition,theuniquenessofthesolutionforfractionaldierentialequations(.
)isdiscussed.
InSection,anexampleisgiventoillustrateourresults.
2ExistenceresultsLemma.
Foragivenfunctiony∈C(J,R),thefollowingproblemDαu(t)=y(t),Dβu()=u()=,(.
)hasauniquesolutionu(t)=Iαy(t),whereIisthefractionalintegralandIαy(t)=t(t–s)α–(α)y(s)ds,<α≤,<β≤and<α–β≤.
ProofOnecanreduceequationDαu(t)=y(t)toanequivalentintegralequationu(t)=Iαy(t)+ctα–+ctα–(.
)forsomec,c∈R.
Byu()=,itfollowsc=.
Consequently,thegeneralsolutionof(.
)isu(t)=Iαy(t)+ctα–.
(.
)Thus,wehaveDβu(t)=Iα–βy(t)+c(α)(α–β)tα–β–=t(t–s)α–β–(α–β)y(s)ds+c(α)(α–β)tα–β–.
(.
)BytheconditionDβu()=,itfollowsthatc=.
Therefore,wehaveu(t)=Iαy(t).
Conversely,byadirectcomputation,wecangetDαu(t)=y(t)andDβu(t)=Iα–βy(t).
Itiseasytoverifyu(t)=Iαy(t)satises(.
).
Thiscompletestheproof.
CombinedwithLemma.
,weseethat(.
)canbetranslatedintothefollowingsystemy(t)=ft,y(t),Iα–βy(t),Iαy(t),(.
)wherey(t)=Dαu(t),t∈JandIα,Iα–βarethestandardfractionalintegrals.
Wangetal.
AdvancesinDierenceEquations2013,2013:280Page3of7http://www.
advancesindifferenceequations.
com/content/2013/1/280Now,welistforconveniencethefollowingcondition:(H)Thereexisty,z∈C(J,R)satisfyingy≤zsuchthaty(t)≤f(t,y(t),Iα–βy(t),Iαy(t)),z(t)≥f(t,z(t),Iα–βz(t),Iαz(t)).
(H)ThereexistsafunctionM∈C(J,(–,+∞))suchthatft,u(t),Iα–βu(t),Iαu(t)–ft,v(t),Iα–βv(t),Iαv(t)≥–M(t)(u–v)(t),wherey≤v≤u≤z,t∈J.
(H)ThereexistfunctionsN,K,L∈C(J,[,+∞))suchthatft,u(t),Iα–βu(t),Iαu(t)–ft,v(t),Iα–βv(t),Iαv(t)≤N(t)(u–v)(t)+K(t)Iα–β(u–v)(t)+L(t)Iα(u–v)(t),wherey≤v≤u≤z,t∈J.
Theorem.
Assumethat(H)and(H)hold.
Thenproblem(.
)hastheminimalandmaximalsolutiony,zintheorderedinterval[y,z].
Moreover,thereexistex-plicitmonotoneiterativesequences{yn},{zn}[y,z]suchthatlimn→∞yn(t)=y(t)andlimn→∞zn(t)=z(t),whereyn(t),zn(t)aredenedasyn(t)=+M(t)ft,yn–(t),Iα–βyn–(t),Iαyn–(t)+M(t)yn–(t),t∈J,n=,,.
.
.
,zn(t)=+M(t)ft,zn–(t),Iα–βzn–(t),Iαzn–(t)+M(t)zn–(t),t∈J,n=,,.
.
.
,(.
)andy≤y≤···≤yn≤···≤y≤z≤···≤zn≤···≤z≤z.
(.
)ProofDeneanoperatorQ:[y,z]→C(J,R)byx=Qη,wherexistheuniquesolutionofthecorrespondinglinearproblemcorrespondingtoη∈[y,z]andQη=+M(t)ft,η(t),Iα–βη(t),Iαη(t)+M(t)η(t).
(.
)Then,theoperatorQhasthefollowingproperties:(a)y≤Qy,Qz≤z;(b)Qh≤Qh,h,h∈[y,z],h≤h.
(.
)Wangetal.
AdvancesinDierenceEquations2013,2013:280Page4of7http://www.
advancesindifferenceequations.
com/content/2013/1/280Firstly,weshowthat(a)holds.
Lety=Qy,p=y–y.
By(H)andthedenitionofQ,weknowthatp(t)=+M(t)ft,y(t),Iα–βy(t),Iαy(t)+M(t)y(t)–y(t)≥+M(t)y(t)+M(t)y(t)–y(t)=.
Thus,wecanobtainp(t)≥,t∈J.
Thatis,y≤Qy.
Similarly,wecanprovethatQz≤z.
Then,(a)holds.
Secondly,letq=Qh–Qh,by(.
)and(H),wehaveq(t)=+M(t)ft,h(t),Iα–βh(t),Iαh(t)+M(t)h(t)–+M(t)ft,h(t),Iα–βh(t),Iαh(t)+M(t)h(t)≥+M(t)–M(t)(h–h)(t)+M(t)(h–h)(t)=.
Hence,wehaveq(t)≥,t∈J.
Thatis,Qh≥Qh.
Then,(b)holds.
Now,putyn=Qyn–,zn=Qzn–,n=,,.
.
.
.
(.
)By(.
),wecangety≤y≤···≤yn≤···≤zn≤···≤z≤z.
Obviously,yn,znsatisfyyn(t)=ft,yn–(t),Iα–βyn–(t),Iαyn–(t)–M(t)(un–yn–)(t),zn(t)=ft,zn–(t),Iα–βzn–(t),Iαzn–(t)–M(t)(zn–zn–)(t).
(.
)EmployingthesameargumentsusedinRef.
[],weseethat{yn},{zn}convergetotheirlimitfunctionsy,z,respectively.
Thatis,limn→∞yn(t)=y(t)andlimn→∞zn(t)=z(t).
Moreover,y(t),z(t)aresolutionsof(.
)in[y,z].
(.
)istrue.
Finally,weprovethaty(t),z(t)aretheminimalandthemaximalsolutionof(.
)in[y,z].
Letw∈[y,z]beanysolutionof(.
),thenQw=w.
Byy≤w≤z,(.
)and(.
),wecanobtainyn≤w≤zn,n=,,.
.
.
.
(.
)Thus,takinglimitin(.
)asn→+∞,wehavey≤w≤z.
Thatis,y,zaretheminimalandmaximalsolutionof(.
)intheorderedinterval[y,z],respectively.
Thiscompletestheproof.
Wangetal.
AdvancesinDierenceEquations2013,2013:280Page5of7http://www.
advancesindifferenceequations.
com/content/2013/1/280Theorem.
LetN(t)≥–M(t).
Assumeconditions(H)-(H)hold.
Ifλ(t)=N(t)+K(t)tα–β(α–β+)+L(t)tα(α+)<,thenproblem(.
)hasauniquesolutionx(t)∈[y,z].
ProofByTheorem.
,wehaveprovedthaty,zaretheminimalandmaximalsolutionof(.
)andy(t)≤y(t)≤z(t)≤z(t),t∈J.
Now,wearegoingtoshowthatproblem(.
)hasauniquesolutionx,i.
e.
,y(t)=z(t)=x(t).
Letp(t)=z(t)–y(t),by(H),wehave≤p(t)≤ft,z(t),Iα–βz(t),Iαz(t)–ft,y(t),Iα–βy(t),Iαy(t)≤N(t)z–y(t)+K(t)Iα–βz–y(t)+L(t)Iαz–y(t)=N(t)p(t)+K(t)t(t–s)α–β–(α–β)p(s)ds+L(t)t(t–s)α–(α)p(s)ds≤N(t)+K(t)tα–β(α–β+)+L(t)tα(α+)maxt∈Jp(t)λ(t)maxt∈Jp(t),whichimpliesthatmaxt∈Jp(t)≤.
Sincep(t)≥,thenitholdsp(t)=.
Thatis,y(t)=z(t).
Therefore,problem(.
)hasauniquesolutionx∈[y,z].
Letx(t)betheuniquesolutionof(.
).
Notingthatx∈[y,z]andu(t)=Iαx(t),wecaneasilyobtainthefollowingtheorem.
Theorem.
LetallconditionsofTheorem.
hold.
Thenproblem(.
)hasauniquesolutionu∈[Iαy,Iαz],t∈J.
3ExampleConsiderthefollowingproblem:Du(t)=t[–Du(t)]+tDu(t)+t[–Du(t)]+tu(t),Du()=,u()=,(.
)wheret∈[,].
LetDu(t)=y(t),thenDu(t)=Iy(t),u(t)=Iy(t).
So,(.
)canbetranslatedintothefollowingproblemy(t)=t–y(t)+ty(t)+t–Iy(t)+tIy(t),(.
)Wangetal.
AdvancesinDierenceEquations2013,2013:280Page6of7http://www.
advancesindifferenceequations.
com/content/2013/1/280Notingthatα=,β=,thenft,y,Iα–βy,Iαy=t[–y]+ty+t–Iy+tIy.
Takey(t)=,z(t)=,wehavey(t)=≤t+t=f(t,y(t),Iα–βy(t),Iαy(t)),z(t)=≥ty+t(–t)+tπ=f(t,z(t),Iα–βz(t),Iαz(t)).
Hence,condition(H)holds.
Fory≤y≤z≤z,wehaveft,z,Iα–βz,Iαz–ft,y,Iα–βy,Iαy=t(–z)–(–y)+t(z–y)+t–Iz––Iy+tIz–Iy≥–t–t(z–y)andft,z,Iα–βz,Iαz–ft,y,Iα–βy,Iαy≤–t(z–y)+tI(z–y)+t√πI(z–y).
TakeM(t)=t–t,N(t)=K(t)=t,L(t)=t√π.
Throughasimplecalculation,wehaveλ(t)=t+t+tπ<.
Then,allconditionsofTheorem.
aresatised.
Inconsequence,theproblem(.
)hasauniquesolutionu∈[,t√π].
CompetinginterestsTheauthorsdeclarethattheyhavenocompetinginterests.
Authors'contributionsAllauthorshaveequalcontributions.
Authordetails1DepartmentofAppliedMathematics,XidianUniversity,Xi'an,Shaanxi710071,People'sRepublicofChina.
2DepartmentofMathematics,FacultyofArtandSciences,Balgat,06530,Turkey.
3InstituteofSpaceSciences,Magurele-Bucharest,Romania.
4DepartmentofChemicalandMaterialsEngineering,FacultyofEngineering,KingAbdulazizUniversity,P.
O.
Box80204,Jeddah,21589,SaudiArabia.
5SchoolofMathematicsandComputerScience,ShanxiNormalUniversity,Linfen,Shanxi041004,People'sRepublicofChina.
AcknowledgementsTheauthorswouldliketothanktherefereesfortheirusefulcommentsandremarks.
ThisworkissupportedbytheNNSFofChina(No.
61373174)andtheNaturalScienceFoundationforYoungScientistsofShanxiProvince,China(No.
2012021002-3).
Received:9May2013Accepted:15August2013Published:4October2013Wangetal.
AdvancesinDierenceEquations2013,2013:280Page7of7http://www.
advancesindifferenceequations.
com/content/2013/1/280References1.
Podlubny,I:FractionalDierentialEquations.
AcademicPress,SanDiego(1999)2.
Kilbas,AA,Srivastava,HM,Trujillo,JJ:TheoryandApplicationsofFractionalDierentialEquations.
North-HollandMathematicsStudies,vol.
204.
Elsevier,Amsterdam(2006)3.
Lakshmikantham,V,Leela,S,Devi,JV:TheoryofFractionalDynamicSystems.
CambridgeScienticPublishers,Cambridge(2009)4.
Sabatier,J,Agrawal,OP,Machado,JAT(eds.
):AdvancesinFractionalCalculus:TheoreticalDevelopmentsandApplicationsinPhysicsandEngineering.
Springer,Dordrecht(2007)5.
Baleanu,D,Diethelm,K,Scalas,E,Trujillo,JJ:FractionalCalculusModelsandNumericalMethods.
SeriesonComplexity,NonlinearityandChaos.
WorldScientic,Boston(2012)6.
Ladde,GS,Lakshmikantham,V,Vatsala,AS:MonotoneIterativeTechniquesforNonlinearDierentialEquations.
Pitman,Boston(1985)7.
Nieto,JJ:Anabstractmonotoneiterativetechnique.
NonlinearAnal.
TMA28(12),1923-1933(1997)8.
Wang,G:Monotoneiterativetechniqueforboundaryvalueproblemsofanonlinearfractionaldierentialequationswithdeviatingarguments.
J.
Comput.
Appl.
Math.
236,2425-2430(2012)9.
Wang,G,Agarwal,RP,Cabada,A:Existenceresultsandthemonotoneiterativetechniqueforsystemsofnonlinearfractionaldierentialequations.
Appl.
Math.
Lett.
25,1019-1024(2012)10.
Wang,G,Baleanu,D,Zhang,L:Monotoneiterativemethodforaclassofnonlinearfractionaldierentialequations.
Fract.
Calc.
Appl.
Anal.
15,244-252(2012)11.
Jankowski,T:InitialvalueproblemsforneutralfractionaldierentialequationsinvolvingaRiemann-Liouvillederivative.
Appl.
Math.
Comput.
219,7772-7776(2013)12.
Jankowski,T:FractionalequationsofVolterratypeinvolvingaRiemann-Liouvillederivative.
Appl.
Math.
Lett.
26,344-350(2013)13.
Lakshmikanthan,V,Vatsala,AS:Generaluniquenessandmonotoneiterativetechniqueforfractionaldierentialequations.
Appl.
Math.
Lett.
21,828-834(2008)14.
McRae,FA:Monotoneiterativetechniqueandexistenceresultsforfractionaldierentialequations.
NonlinearAnal.
71,6093-6096(2009)15.
Wei,Z,Li,G,Che,J:InitialvalueproblemsforfractionaldierentialequationsinvolvingRiemann-Liouvillesequentialfractionalderivative.
J.
Math.
Anal.
Appl.
367,260-272(2010)16.
Zhang,L,Wang,G,Ahmad,B,Agarwal,RP:Nonlinearfractionalintegro-dierentialequationsonunboundeddomainsinaBanachspace.
J.
Comput.
Appl.
Math.
249,51-56(2013)17.
Zhang,S:MonotoneiterativemethodforinitialvalueprobleminvolvingRiemann-Liouvillefractionalderivatives.
NonlinearAnal.
71,2087-2093(2009)18.
Liu,Z,Sun,J,Szanto,I:MonotoneiterativetechniqueforRiemann-Liouvillefractionalintegro-dierentialequationswithadvancedarguments.
ResultsMath.
(2012).
doi:10.
1007/s00025-012-0268-419.
Zhang,S,Su,X:Theexistenceofasolutionforafractionaldierentialequationwithnonlinearboundaryconditionsconsideredusingupperandlowersolutionsinreversedorder.
Comput.
Math.
Appl.
62,1269-1274(2011)20.
Al-Refai,M,Hajji,MA:Monotoneiterativesequencesfornonlinearboundaryvalueproblemsoffractionalorder.
NonlinearAnal.
74,3531-3539(2011)21.
Ramirez,JD,Vatsala,AS:Monotoneiterativetechniqueforfractionaldierentialequationswithperiodicboundaryconditions.
Opusc.
Math.
29,289-304(2009)22.
Lin,L,Liu,X,Fang,H:Methodofupperandlowersolutionsforfractionaldierentialequations.
Electron.
J.
Dier.
Equ.
2012,1-13(2012)doi:10.
1186/1687-1847-2013-280Citethisarticleas:Wangetal.
:ExistenceresultsfornonlinearfractionaldifferentialequationsinvolvingdifferentRiemann-Liouvillefractionalderivatives.
AdvancesinDierenceEquations20132013:280.
AdvancesinDierenceEquations2013,2013:280http://www.
advancesindifferenceequations.
com/content/2013/1/280RESEARCHOpenAccessExistenceresultsfornonlinearfractionaldifferentialequationsinvolvingdifferentRiemann-LiouvillefractionalderivativesGuotaoWang1,SanyangLiu1*,DumitruBaleanu2,3,4andLihongZhang5*Correspondence:liusanyang@126.
com1DepartmentofAppliedMathematics,XidianUniversity,Xi'an,Shaanxi710071,People'sRepublicofChinaFulllistofauthorinformationisavailableattheendofthearticleAbstractByapplyinganiterativetechnique,anecessaryandsucientconditionisobtainedfortheexistenceoftheuniquesolutionofnonlinearfractionaldierentialequationsinvolvingtwoRiemann-Liouvillederivativesofdierentfractionalorders.
Finally,anexampleisalsogiventoillustratetheavailabilityofourmainresults.
Keywords:dierentfractional-order;nonlinearfractionaldierentialequations;Riemann-Liouvillederivative;monotoneiterativetechnique1IntroductionRecently,thestudyoffractionaldierentialequationshasacquiredpopularity,seebooks[–]formoreinformation.
Inthispaper,weconsiderthefollowingnonlinearfractionaldierentialequations:Dαu(t)=f(t,Dαu(t),Dβu(t),u(t)),Dβu()=,u()=,(.
)wheret∈J=[,T](
Themethodofupperandlowersolutionscoupledwiththemonotoneiterativetech-niqueisaninterestingandpowerfulmechanism.
Theimportanceandadvantageofthemethodneedsnospecialemphasis[,].
TherehaveappearedsomepapersdealingwiththeexistenceofthesolutionofnonlinearRiemann-Liouville-typefractionaldier-entialequations[–]ornonlinearCaputo-typefractionaldierentialequations[–]byusingthemethod.
Forexample,byemployingthemethodoflowerandupperso-lutionscombinedwiththemonotoneiterativetechnique,LakshmikanthanandVatsala[],McRae[]andZhang[]successfullyinvestigatedtheinitialvalueproblemsofRiemann-LiouvillefractionaldierentialequationDαu(t)=f(t,u(t)),where<α≤.
However,intheexistingliterature[–],onlyonecasewhenα∈(,]isconsidered.
Theresearch,involvingRiemann-Liouvillefractionalderivativeoforder<α≤,pro-ceedsslowlyandthereappearsomenewdicultiesinemployingthemonotoneiterativemethod.
Toovercomethesediculties,weapplyasubstitutionDαu(t)=y(t).
Notethat2013Wangetal.
;licenseeSpringer.
ThisisanOpenAccessarticledistributedunderthetermsoftheCreativeCommonsAttribu-tionLicense(http://creativecommons.
org/licenses/by/2.
0),whichpermitsunrestricteduse,distribution,andreproductioninanymedium,providedtheoriginalworkisproperlycited.
Wangetal.
AdvancesinDierenceEquations2013,2013:280Page2of7http://www.
advancesindifferenceequations.
com/content/2013/1/280thetechniquehasbeendiscussedforfractionalproblemsinpapers[,].
Tothebestofourknowledge,itistherstpaper,inwhichthemonotoneiterativemethodisappliedtononlinearRiemann-Liouville-typefractionaldierentialequations,involvingtwodier-entfractionalderivativesDαandDβ.
Weorganizetherestofthispaperasfollows.
InSection,byusingthemonotoneiter-ativetechniqueandthemethodofupperandlowersolutions,theminimalandmaximalsolutionsofanequivalentproblemof(.
)areinvestigatedandtwoexplicitmonotoneit-erativesequences,convergingtothecorrespondingminimalandmaximalsolution,aregiven.
Inaddition,theuniquenessofthesolutionforfractionaldierentialequations(.
)isdiscussed.
InSection,anexampleisgiventoillustrateourresults.
2ExistenceresultsLemma.
Foragivenfunctiony∈C(J,R),thefollowingproblemDαu(t)=y(t),Dβu()=u()=,(.
)hasauniquesolutionu(t)=Iαy(t),whereIisthefractionalintegralandIαy(t)=t(t–s)α–(α)y(s)ds,<α≤,<β≤and<α–β≤.
ProofOnecanreduceequationDαu(t)=y(t)toanequivalentintegralequationu(t)=Iαy(t)+ctα–+ctα–(.
)forsomec,c∈R.
Byu()=,itfollowsc=.
Consequently,thegeneralsolutionof(.
)isu(t)=Iαy(t)+ctα–.
(.
)Thus,wehaveDβu(t)=Iα–βy(t)+c(α)(α–β)tα–β–=t(t–s)α–β–(α–β)y(s)ds+c(α)(α–β)tα–β–.
(.
)BytheconditionDβu()=,itfollowsthatc=.
Therefore,wehaveu(t)=Iαy(t).
Conversely,byadirectcomputation,wecangetDαu(t)=y(t)andDβu(t)=Iα–βy(t).
Itiseasytoverifyu(t)=Iαy(t)satises(.
).
Thiscompletestheproof.
CombinedwithLemma.
,weseethat(.
)canbetranslatedintothefollowingsystemy(t)=ft,y(t),Iα–βy(t),Iαy(t),(.
)wherey(t)=Dαu(t),t∈JandIα,Iα–βarethestandardfractionalintegrals.
Wangetal.
AdvancesinDierenceEquations2013,2013:280Page3of7http://www.
advancesindifferenceequations.
com/content/2013/1/280Now,welistforconveniencethefollowingcondition:(H)Thereexisty,z∈C(J,R)satisfyingy≤zsuchthaty(t)≤f(t,y(t),Iα–βy(t),Iαy(t)),z(t)≥f(t,z(t),Iα–βz(t),Iαz(t)).
(H)ThereexistsafunctionM∈C(J,(–,+∞))suchthatft,u(t),Iα–βu(t),Iαu(t)–ft,v(t),Iα–βv(t),Iαv(t)≥–M(t)(u–v)(t),wherey≤v≤u≤z,t∈J.
(H)ThereexistfunctionsN,K,L∈C(J,[,+∞))suchthatft,u(t),Iα–βu(t),Iαu(t)–ft,v(t),Iα–βv(t),Iαv(t)≤N(t)(u–v)(t)+K(t)Iα–β(u–v)(t)+L(t)Iα(u–v)(t),wherey≤v≤u≤z,t∈J.
Theorem.
Assumethat(H)and(H)hold.
Thenproblem(.
)hastheminimalandmaximalsolutiony,zintheorderedinterval[y,z].
Moreover,thereexistex-plicitmonotoneiterativesequences{yn},{zn}[y,z]suchthatlimn→∞yn(t)=y(t)andlimn→∞zn(t)=z(t),whereyn(t),zn(t)aredenedasyn(t)=+M(t)ft,yn–(t),Iα–βyn–(t),Iαyn–(t)+M(t)yn–(t),t∈J,n=,,.
.
.
,zn(t)=+M(t)ft,zn–(t),Iα–βzn–(t),Iαzn–(t)+M(t)zn–(t),t∈J,n=,,.
.
.
,(.
)andy≤y≤···≤yn≤···≤y≤z≤···≤zn≤···≤z≤z.
(.
)ProofDeneanoperatorQ:[y,z]→C(J,R)byx=Qη,wherexistheuniquesolutionofthecorrespondinglinearproblemcorrespondingtoη∈[y,z]andQη=+M(t)ft,η(t),Iα–βη(t),Iαη(t)+M(t)η(t).
(.
)Then,theoperatorQhasthefollowingproperties:(a)y≤Qy,Qz≤z;(b)Qh≤Qh,h,h∈[y,z],h≤h.
(.
)Wangetal.
AdvancesinDierenceEquations2013,2013:280Page4of7http://www.
advancesindifferenceequations.
com/content/2013/1/280Firstly,weshowthat(a)holds.
Lety=Qy,p=y–y.
By(H)andthedenitionofQ,weknowthatp(t)=+M(t)ft,y(t),Iα–βy(t),Iαy(t)+M(t)y(t)–y(t)≥+M(t)y(t)+M(t)y(t)–y(t)=.
Thus,wecanobtainp(t)≥,t∈J.
Thatis,y≤Qy.
Similarly,wecanprovethatQz≤z.
Then,(a)holds.
Secondly,letq=Qh–Qh,by(.
)and(H),wehaveq(t)=+M(t)ft,h(t),Iα–βh(t),Iαh(t)+M(t)h(t)–+M(t)ft,h(t),Iα–βh(t),Iαh(t)+M(t)h(t)≥+M(t)–M(t)(h–h)(t)+M(t)(h–h)(t)=.
Hence,wehaveq(t)≥,t∈J.
Thatis,Qh≥Qh.
Then,(b)holds.
Now,putyn=Qyn–,zn=Qzn–,n=,,.
.
.
.
(.
)By(.
),wecangety≤y≤···≤yn≤···≤zn≤···≤z≤z.
Obviously,yn,znsatisfyyn(t)=ft,yn–(t),Iα–βyn–(t),Iαyn–(t)–M(t)(un–yn–)(t),zn(t)=ft,zn–(t),Iα–βzn–(t),Iαzn–(t)–M(t)(zn–zn–)(t).
(.
)EmployingthesameargumentsusedinRef.
[],weseethat{yn},{zn}convergetotheirlimitfunctionsy,z,respectively.
Thatis,limn→∞yn(t)=y(t)andlimn→∞zn(t)=z(t).
Moreover,y(t),z(t)aresolutionsof(.
)in[y,z].
(.
)istrue.
Finally,weprovethaty(t),z(t)aretheminimalandthemaximalsolutionof(.
)in[y,z].
Letw∈[y,z]beanysolutionof(.
),thenQw=w.
Byy≤w≤z,(.
)and(.
),wecanobtainyn≤w≤zn,n=,,.
.
.
.
(.
)Thus,takinglimitin(.
)asn→+∞,wehavey≤w≤z.
Thatis,y,zaretheminimalandmaximalsolutionof(.
)intheorderedinterval[y,z],respectively.
Thiscompletestheproof.
Wangetal.
AdvancesinDierenceEquations2013,2013:280Page5of7http://www.
advancesindifferenceequations.
com/content/2013/1/280Theorem.
LetN(t)≥–M(t).
Assumeconditions(H)-(H)hold.
Ifλ(t)=N(t)+K(t)tα–β(α–β+)+L(t)tα(α+)<,thenproblem(.
)hasauniquesolutionx(t)∈[y,z].
ProofByTheorem.
,wehaveprovedthaty,zaretheminimalandmaximalsolutionof(.
)andy(t)≤y(t)≤z(t)≤z(t),t∈J.
Now,wearegoingtoshowthatproblem(.
)hasauniquesolutionx,i.
e.
,y(t)=z(t)=x(t).
Letp(t)=z(t)–y(t),by(H),wehave≤p(t)≤ft,z(t),Iα–βz(t),Iαz(t)–ft,y(t),Iα–βy(t),Iαy(t)≤N(t)z–y(t)+K(t)Iα–βz–y(t)+L(t)Iαz–y(t)=N(t)p(t)+K(t)t(t–s)α–β–(α–β)p(s)ds+L(t)t(t–s)α–(α)p(s)ds≤N(t)+K(t)tα–β(α–β+)+L(t)tα(α+)maxt∈Jp(t)λ(t)maxt∈Jp(t),whichimpliesthatmaxt∈Jp(t)≤.
Sincep(t)≥,thenitholdsp(t)=.
Thatis,y(t)=z(t).
Therefore,problem(.
)hasauniquesolutionx∈[y,z].
Letx(t)betheuniquesolutionof(.
).
Notingthatx∈[y,z]andu(t)=Iαx(t),wecaneasilyobtainthefollowingtheorem.
Theorem.
LetallconditionsofTheorem.
hold.
Thenproblem(.
)hasauniquesolutionu∈[Iαy,Iαz],t∈J.
3ExampleConsiderthefollowingproblem:Du(t)=t[–Du(t)]+tDu(t)+t[–Du(t)]+tu(t),Du()=,u()=,(.
)wheret∈[,].
LetDu(t)=y(t),thenDu(t)=Iy(t),u(t)=Iy(t).
So,(.
)canbetranslatedintothefollowingproblemy(t)=t–y(t)+ty(t)+t–Iy(t)+tIy(t),(.
)Wangetal.
AdvancesinDierenceEquations2013,2013:280Page6of7http://www.
advancesindifferenceequations.
com/content/2013/1/280Notingthatα=,β=,thenft,y,Iα–βy,Iαy=t[–y]+ty+t–Iy+tIy.
Takey(t)=,z(t)=,wehavey(t)=≤t+t=f(t,y(t),Iα–βy(t),Iαy(t)),z(t)=≥ty+t(–t)+tπ=f(t,z(t),Iα–βz(t),Iαz(t)).
Hence,condition(H)holds.
Fory≤y≤z≤z,wehaveft,z,Iα–βz,Iαz–ft,y,Iα–βy,Iαy=t(–z)–(–y)+t(z–y)+t–Iz––Iy+tIz–Iy≥–t–t(z–y)andft,z,Iα–βz,Iαz–ft,y,Iα–βy,Iαy≤–t(z–y)+tI(z–y)+t√πI(z–y).
TakeM(t)=t–t,N(t)=K(t)=t,L(t)=t√π.
Throughasimplecalculation,wehaveλ(t)=t+t+tπ<.
Then,allconditionsofTheorem.
aresatised.
Inconsequence,theproblem(.
)hasauniquesolutionu∈[,t√π].
CompetinginterestsTheauthorsdeclarethattheyhavenocompetinginterests.
Authors'contributionsAllauthorshaveequalcontributions.
Authordetails1DepartmentofAppliedMathematics,XidianUniversity,Xi'an,Shaanxi710071,People'sRepublicofChina.
2DepartmentofMathematics,FacultyofArtandSciences,Balgat,06530,Turkey.
3InstituteofSpaceSciences,Magurele-Bucharest,Romania.
4DepartmentofChemicalandMaterialsEngineering,FacultyofEngineering,KingAbdulazizUniversity,P.
O.
Box80204,Jeddah,21589,SaudiArabia.
5SchoolofMathematicsandComputerScience,ShanxiNormalUniversity,Linfen,Shanxi041004,People'sRepublicofChina.
AcknowledgementsTheauthorswouldliketothanktherefereesfortheirusefulcommentsandremarks.
ThisworkissupportedbytheNNSFofChina(No.
61373174)andtheNaturalScienceFoundationforYoungScientistsofShanxiProvince,China(No.
2012021002-3).
Received:9May2013Accepted:15August2013Published:4October2013Wangetal.
AdvancesinDierenceEquations2013,2013:280Page7of7http://www.
advancesindifferenceequations.
com/content/2013/1/280References1.
Podlubny,I:FractionalDierentialEquations.
AcademicPress,SanDiego(1999)2.
Kilbas,AA,Srivastava,HM,Trujillo,JJ:TheoryandApplicationsofFractionalDierentialEquations.
North-HollandMathematicsStudies,vol.
204.
Elsevier,Amsterdam(2006)3.
Lakshmikantham,V,Leela,S,Devi,JV:TheoryofFractionalDynamicSystems.
CambridgeScienticPublishers,Cambridge(2009)4.
Sabatier,J,Agrawal,OP,Machado,JAT(eds.
):AdvancesinFractionalCalculus:TheoreticalDevelopmentsandApplicationsinPhysicsandEngineering.
Springer,Dordrecht(2007)5.
Baleanu,D,Diethelm,K,Scalas,E,Trujillo,JJ:FractionalCalculusModelsandNumericalMethods.
SeriesonComplexity,NonlinearityandChaos.
WorldScientic,Boston(2012)6.
Ladde,GS,Lakshmikantham,V,Vatsala,AS:MonotoneIterativeTechniquesforNonlinearDierentialEquations.
Pitman,Boston(1985)7.
Nieto,JJ:Anabstractmonotoneiterativetechnique.
NonlinearAnal.
TMA28(12),1923-1933(1997)8.
Wang,G:Monotoneiterativetechniqueforboundaryvalueproblemsofanonlinearfractionaldierentialequationswithdeviatingarguments.
J.
Comput.
Appl.
Math.
236,2425-2430(2012)9.
Wang,G,Agarwal,RP,Cabada,A:Existenceresultsandthemonotoneiterativetechniqueforsystemsofnonlinearfractionaldierentialequations.
Appl.
Math.
Lett.
25,1019-1024(2012)10.
Wang,G,Baleanu,D,Zhang,L:Monotoneiterativemethodforaclassofnonlinearfractionaldierentialequations.
Fract.
Calc.
Appl.
Anal.
15,244-252(2012)11.
Jankowski,T:InitialvalueproblemsforneutralfractionaldierentialequationsinvolvingaRiemann-Liouvillederivative.
Appl.
Math.
Comput.
219,7772-7776(2013)12.
Jankowski,T:FractionalequationsofVolterratypeinvolvingaRiemann-Liouvillederivative.
Appl.
Math.
Lett.
26,344-350(2013)13.
Lakshmikanthan,V,Vatsala,AS:Generaluniquenessandmonotoneiterativetechniqueforfractionaldierentialequations.
Appl.
Math.
Lett.
21,828-834(2008)14.
McRae,FA:Monotoneiterativetechniqueandexistenceresultsforfractionaldierentialequations.
NonlinearAnal.
71,6093-6096(2009)15.
Wei,Z,Li,G,Che,J:InitialvalueproblemsforfractionaldierentialequationsinvolvingRiemann-Liouvillesequentialfractionalderivative.
J.
Math.
Anal.
Appl.
367,260-272(2010)16.
Zhang,L,Wang,G,Ahmad,B,Agarwal,RP:Nonlinearfractionalintegro-dierentialequationsonunboundeddomainsinaBanachspace.
J.
Comput.
Appl.
Math.
249,51-56(2013)17.
Zhang,S:MonotoneiterativemethodforinitialvalueprobleminvolvingRiemann-Liouvillefractionalderivatives.
NonlinearAnal.
71,2087-2093(2009)18.
Liu,Z,Sun,J,Szanto,I:MonotoneiterativetechniqueforRiemann-Liouvillefractionalintegro-dierentialequationswithadvancedarguments.
ResultsMath.
(2012).
doi:10.
1007/s00025-012-0268-419.
Zhang,S,Su,X:Theexistenceofasolutionforafractionaldierentialequationwithnonlinearboundaryconditionsconsideredusingupperandlowersolutionsinreversedorder.
Comput.
Math.
Appl.
62,1269-1274(2011)20.
Al-Refai,M,Hajji,MA:Monotoneiterativesequencesfornonlinearboundaryvalueproblemsoffractionalorder.
NonlinearAnal.
74,3531-3539(2011)21.
Ramirez,JD,Vatsala,AS:Monotoneiterativetechniqueforfractionaldierentialequationswithperiodicboundaryconditions.
Opusc.
Math.
29,289-304(2009)22.
Lin,L,Liu,X,Fang,H:Methodofupperandlowersolutionsforfractionaldierentialequations.
Electron.
J.
Dier.
Equ.
2012,1-13(2012)doi:10.
1186/1687-1847-2013-280Citethisarticleas:Wangetal.
:ExistenceresultsfornonlinearfractionaldifferentialequationsinvolvingdifferentRiemann-Liouvillefractionalderivatives.
AdvancesinDierenceEquations20132013:280.
- mediumwww.236jj.com相关文档
- TS12A4514www.236jj.com
- Windowswww.236jj.com
- mTORC2www.236jj.com
- milswww.236jj.com
- D0www.236jj.com
- digitalwww.236jj.com
捷锐数据399/年、60元/季 ,香港CN2云服务器 4H4G10M
捷锐数据官网商家介绍捷锐数据怎么样?捷锐数据好不好?捷锐数据是成立于2018年一家国人IDC商家,早期其主营虚拟主机CDN,现在主要有香港云服、国内物理机、腾讯轻量云代理、阿里轻量云代理,自营香港为CN2+BGP线路,采用KVM虚拟化而且单IP提供10G流量清洗并且免费配备天机盾可达到屏蔽UDP以及无视CC效果。这次捷锐数据给大家带来的活动是香港云促销,总共放量40台点击进入捷锐数据官网优惠活动内...

hostyun评测香港原生IPVPS
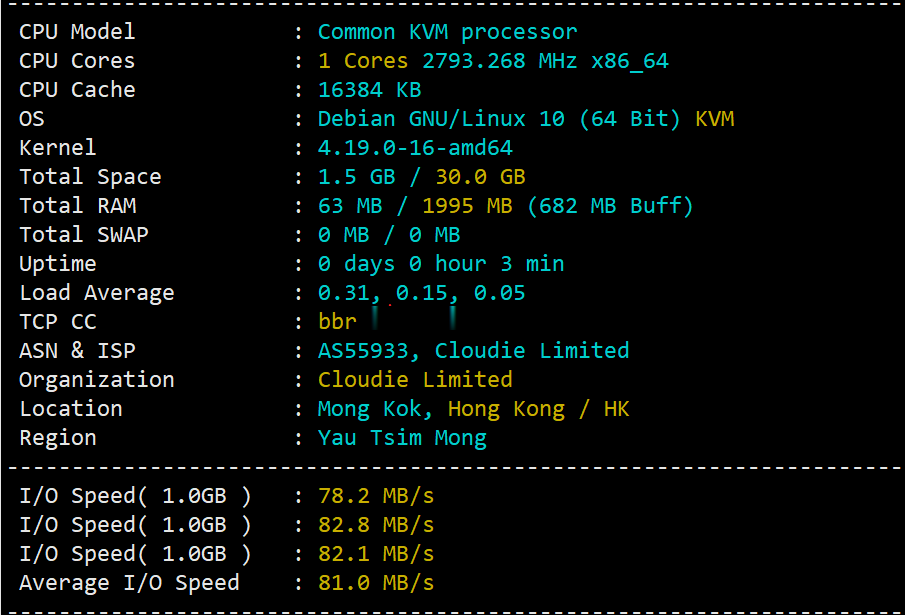
hostyun新上了香港cloudie机房的香港原生IP的VPS,写的是默认接入200Mbps带宽(共享),基于KVM虚拟,纯SSD RAID10,三网直连,混合超售的CN2网络,商家对VPS的I/O有大致100MB/S的限制。由于是原生香港IP,所以这个VPS还是有一定的看头的,这里给大家弄个测评,数据仅供参考!9折优惠码:hostyun,循环优惠内存CPUSSD流量带宽价格购买1G1核10G3...
HostKvm($4.25/月)俄罗斯/香港高防VPS
HostKvm又上新了,这次上架了2个线路产品:俄罗斯和香港高防VPS,其中俄罗斯经测试电信CN2线路,而香港高防VPS提供30Gbps攻击防御。HostKvm是一家成立于2013年的国外主机服务商,主要提供基于KVM架构的VPS主机,可选数据中心包括日本、新加坡、韩国、美国、中国香港等多个地区机房,均为国内直连或优化线路,延迟较低,适合建站或者远程办公等。俄罗斯VPSCPU:1core内存:2G...
www.236jj.com为你推荐
-
12306崩溃亲们,为什么12306手机订票系统打不开,显示网络异常,硬盘工作原理高人指点:电子存储器(U盘,储存卡,硬盘等)的工作原理广东GDP破10万亿在已披露的2017年GDP经济数据中,以下哪个省份GDP总量排名第一?地陷裂口天上顿时露出一个大窟窿地上也裂开了,一到黑幽幽的深沟可以用什么四字词语来?同ip站点同IP网站具体是什么意思,能换独立的吗www.kanav001.com翻译为日文: 主人,请你收养我一天吧. 带上罗马音标会更好wwwwww.toutoulu.com老板强大的外包装还是被快递弄断了dpscycle寻求LR 高输出宏干支论坛2018天干地支数值是多少?蚕食嫩妻求都市超宠文、温馨、轻松、无虐、无小三